From Wikipedia, the free encyclopedia

Typical aerodynamic teardrop shape, assuming a viscous medium passing from left to right, the diagram shows the pressure distribution as the thickness of the black line and shows the velocity in the boundary layer as the violet triangles. The green vortex generators prompt the transition to turbulent flow and prevent back-flow also called flow separation from the high pressure region in the back. The surface in front is as smooth as possible or even employs shark-like skin, as any turbulence here will reduce the energy of the airflow. The truncation on the right, known as a Kammback, also prevents backflow from the high pressure region in the back across the spoilers to the convergent part.
In physics, fluid dynamics is a subdiscipline of fluid mechanics that deals with fluid flow—the natural science of fluids (liquids and gases) in motion. It has several subdisciplines itself, including aerodynamics (the study of air and other gases in motion) and hydrodynamics (the study of liquids in motion). Fluid dynamics has a wide range of applications, including calculating forces and moments on aircraft, determining the mass flow rate of petroleum through pipelines, predicting weather patterns, understanding nebulae in interstellar space and modelling fission weapon detonation. Some of its principles are even used in traffic engineering, where traffic is treated as a continuous fluid, and crowd dynamics.
Fluid dynamics offers a systematic structure—which underlies these practical disciplines—that embraces empirical and semi-empirical laws derived from flow measurement and used to solve practical problems. The solution to a fluid dynamics problem typically involves calculating various properties of the fluid, such as flow velocity, pressure, density, and temperature, as functions of space and time.
Before the twentieth century, hydrodynamics was synonymous with fluid dynamics. This is still reflected in names of some fluid dynamics topics, like magnetohydrodynamics and hydrodynamic stability, both of which can also be applied to gases.[1]
Equations of fluid dynamics
The foundational axioms of fluid dynamics are the conservation laws, specifically, conservation of mass, conservation of linear momentum (also known as Newton's Second Law of Motion), and conservation of energy (also known as First Law of Thermodynamics). These are based on classical mechanics and are modified in quantum mechanics and general relativity. They are expressed using the Reynolds Transport Theorem.In addition to the above, fluids are assumed to obey the continuum assumption. Fluids are composed of molecules that collide with one another and solid objects. However, the continuum assumption considers fluids to be continuous, rather than discrete. Consequently, properties such as density, pressure, temperature, and flow velocity are taken to be well-defined at infinitesimally small points, and are assumed to vary continuously from one point to another. The fact that the fluid is made up of discrete molecules is ignored.
For fluids which are sufficiently dense to be a continuum, do not contain ionized species, and have flow velocities small in relation to the speed of light, the momentum equations for Newtonian fluids are the Navier–Stokes equations, which is a non-linear set of differential equations that describes the flow of a fluid whose stress depends linearly on flow velocity gradients and pressure. The unsimplified equations do not have a general closed-form solution, so they are primarily of use in Computational Fluid Dynamics. The equations can be simplified in a number of ways, all of which make them easier to solve. Some of them allow appropriate fluid dynamics problems to be solved in closed form.[citation needed]
In addition to the mass, momentum, and energy conservation equations, a thermodynamical equation of state giving the pressure as a function of other thermodynamic variables for the fluid is required to completely specify the problem. An example of this would be the perfect gas equation of state:
Conservation laws
Three conservation laws are used to solve fluid dynamics problems, and may be written in integral or differential form. Mathematical formulations of these conservation laws may be interpreted by considering the concept of a control volume. A control volume is a specified volume in space through which air can flow in and out. Integral formulations of the conservation laws consider the change in mass, momentum, or energy within the control volume. Differential formulations of the conservation laws apply Stokes' theorem to yield an expression which may be interpreted as the integral form of the law applied to an infinitesimal volume at a point within the flow.- Mass continuity (conservation of mass): The rate of change of fluid mass inside a control volume must be equal to the net rate of fluid flow into the volume. Physically, this statement requires that mass is neither created nor destroyed in the control volume,[2] and can be translated into the integral form of the continuity equation:
- Above,
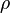 is the fluid density, u is the flow velocity vector, and t is time. The left-hand side of the above expression contains a triple integral over the control volume, whereas the right-hand side contains a surface integral over the surface of the control volume. The differential form of the continuity equation is, by the divergence theorem:
is the fluid density, u is the flow velocity vector, and t is time. The left-hand side of the above expression contains a triple integral over the control volume, whereas the right-hand side contains a surface integral over the surface of the control volume. The differential form of the continuity equation is, by the divergence theorem:
- Conservation of momentum: This equation applies Newton's second law of motion to the control volume, requiring that any change in momentum of the air within a control volume be due to the net flow of air into the volume and the action of external forces on the air within the volume. In the integral formulation of this equation, body forces here are represented by fbody, the body force per unit mass. Surface forces, such as viscous forces, are represented by
 , the net force due to stresses on the control volume surface.
, the net force due to stresses on the control volume surface.
- The differential form of the momentum conservation equation is as follows. Here, both surface and body forces are accounted for in one total force, F. For example, F may be expanded into an expression for the frictional and gravitational forces acting on an internal flow.
- In aerodynamics, air is assumed to be a Newtonian fluid, which posits a linear relationship between the shear stress (due to internal friction forces) and the rate of strain of the fluid. The equation above is a vector equation: in a three-dimensional flow, it can be expressed as three scalar equations. The conservation of momentum equations for the compressible, viscous flow case are called the Navier–Stokes equations.[citation needed]
- Conservation of energy: Although energy can be converted from one form to another, the total energy in a given closed system remains constant.
- Above, h is enthalpy, k is the thermal conductivity of the fluid, T is temperature, and
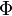 is the viscous dissipation function. The viscous dissipation function governs the rate at which mechanical energy of the flow is converted to heat. The second law of thermodynamics requires that the dissipation term is always positive: viscosity cannot create energy within the control volume.[3] The expression on the left side is a material derivative.
is the viscous dissipation function. The viscous dissipation function governs the rate at which mechanical energy of the flow is converted to heat. The second law of thermodynamics requires that the dissipation term is always positive: viscosity cannot create energy within the control volume.[3] The expression on the left side is a material derivative.
Compressible vs incompressible flow
All fluids are compressible to some extent, that is, changes in pressure or temperature will result in changes in density. However, in many situations the changes in pressure and temperature are sufficiently small that the changes in density are negligible. In this case the flow can be modelled as an incompressible flow. Otherwise the more general compressible flow equations must be used.Mathematically, incompressibility is expressed by saying that the density ρ of a fluid parcel does not change as it moves in the flow field, i.e.,
For flow of gases, to determine whether to use compressible or incompressible fluid dynamics, the Mach number of the flow is to be evaluated. As a rough guide, compressible effects can be ignored at Mach numbers below approximately 0.3. For liquids, whether the incompressible assumption is valid depends on the fluid properties (specifically the critical pressure and temperature of the fluid) and the flow conditions (how close to the critical pressure the actual flow pressure becomes). Acoustic problems always require allowing compressibility, since sound waves are compression waves involving changes in pressure and density of the medium through which they propagate.
Inviscid vs Newtonian and non-Newtonian fluids
Viscous problems are those in which fluid friction has significant effects on the fluid motion.
The Reynolds number, which is a ratio between inertial and viscous forces, can be used to evaluate whether viscous or inviscid equations are appropriate to the problem.
Stokes flow is flow at very low Reynolds numbers, Re << 1, such that inertial forces can be neglected compared to viscous forces.
On the contrary, high Reynolds numbers indicate that the inertial forces are more significant than the viscous (friction) forces. Therefore, we may assume the flow to be an inviscid flow, an approximation in which we neglect viscosity completely, compared to inertial terms.
This idea can work fairly well when the Reynolds number is high. However, certain problems such as those involving solid boundaries, may require that the viscosity be included. Viscosity often cannot be neglected near solid boundaries because the no-slip condition can generate a thin region of large strain rate (known as Boundary layer) which enhances the effect of even a small amount of viscosity, and thus generating vorticity. Therefore, to calculate net forces on bodies (such as wings) we should use viscous flow equations. As illustrated by d'Alembert's paradox, a body in an inviscid fluid will experience no drag force. The standard equations of inviscid flow are the Euler equations. Another often used model, especially in computational fluid dynamics, is to use the Euler equations away from the body and the boundary layer equations, which incorporates viscosity, in a region close to the body.
The Euler equations can be integrated along a streamline to get Bernoulli's equation. When the flow is everywhere irrotational and inviscid, Bernoulli's equation can be used throughout the flow field. Such flows are called potential flows.
Sir Isaac Newton showed how stress and the rate of strain are very close to linearly related for many familiar fluids, such as water and air. These Newtonian fluids are modelled by a viscosity that is independent of strain rate, depending primarily on the specific fluid.
However, some of the other materials, such as emulsions and slurries and some visco-elastic materials (e.g. blood, some polymers), have more complicated non-Newtonian stress-strain behaviours. These materials include sticky liquids such as latex, honey, and lubricants which are studied in the sub-discipline of rheology.
Steady vs unsteady flow
When all the time derivatives of a flow field vanish, the flow is considered to be a steady flow. Steady-state flow refers to the condition where the fluid properties at a point in the system do not change over time. Otherwise, flow is called unsteady (also called transient[5]). Whether a particular flow is steady or unsteady, can depend on the chosen frame of reference. For instance, laminar flow over a sphere is steady in the frame of reference that is stationary with respect to the sphere. In a frame of reference that is stationary with respect to a background flow, the flow is unsteady.
Turbulent flows are unsteady by definition. A turbulent flow can, however, be statistically stationary. According to Pope:[6]
The random field U(x,t) is statistically stationary if all statistics are invariant under a shift in time.This roughly means that all statistical properties are constant in time. Often, the mean field is the object of interest, and this is constant too in a statistically stationary flow.
Steady flows are often more tractable than otherwise similar unsteady flows. The governing equations of a steady problem have one dimension fewer (time) than the governing equations of the same problem without taking advantage of the steadiness of the flow field.
Laminar vs turbulent flow
Turbulence is flow characterized by recirculation, eddies, and apparent randomness. Flow in which turbulence is not exhibited is called laminar. It should be noted, however, that the presence of eddies or recirculation alone does not necessarily indicate turbulent flow—these phenomena may be present in laminar flow as well. Mathematically, turbulent flow is often represented via a Reynolds decomposition, in which the flow is broken down into the sum of an average component and a perturbation component.It is believed that turbulent flows can be described well through the use of the Navier–Stokes equations. Direct numerical simulation (DNS), based on the Navier–Stokes equations, makes it possible to simulate turbulent flows at moderate Reynolds numbers. Restrictions depend on the power of the computer used and the efficiency of the solution algorithm. The results of DNS have been found to agree well with experimental data for some flows.[7]
Most flows of interest have Reynolds numbers much too high for DNS to be a viable option,[8] given the state of computational power for the next few decades. Any flight vehicle large enough to carry a human (L > 3 m), moving faster than 72 km/h (20 m/s) is well beyond the limit of DNS simulation (Re = 4 million). Transport aircraft wings (such as on an Airbus A300 or Boeing 747) have Reynolds numbers of 40 million (based on the wing chord). In order to solve these real-life flow problems, turbulence models will be a necessity for the foreseeable future. Reynolds-averaged Navier–Stokes equations (RANS) combined with turbulence modelling provides a model of the effects of the turbulent flow. Such a modelling mainly provides the additional momentum transfer by the Reynolds stresses, although the turbulence also enhances the heat and mass transfer. Another promising methodology is large eddy simulation (LES), especially in the guise of detached eddy simulation (DES)—which is a combination of RANS turbulence modelling and large eddy simulation.
Subsonic vs transonic, supersonic and hypersonic flows
While many terrestrial flows (e.g. flow of water through a pipe) occur at low mach numbers, many flows of practical interest (e.g. in aerodynamics) occur at high fractions of the Mach Number M=1 or in excess of it (supersonic flows). New phenomena occur at these Mach number regimes (e.g. shock waves for supersonic flow, transonic instability in a regime of flows with M nearly equal to 1, non-equilibrium chemical behaviour due to ionization in hypersonic flows) and it is necessary to treat each of these flow regimes separately.Magnetohydrodynamics
Magnetohydrodynamics is the multi-disciplinary study of the flow of electrically conducting fluids in electromagnetic fields. Examples of such fluids include plasmas, liquid metals, and salt water. The fluid flow equations are solved simultaneously with Maxwell's equations of electromagnetism.Other approximations
There are a large number of other possible approximations to fluid dynamic problems. Some of the more commonly used are listed below.- The Boussinesq approximation neglects variations in density except to calculate buoyancy forces. It is often used in free convection problems where density changes are small.
- Lubrication theory and Hele–Shaw flow exploits the large aspect ratio of the domain to show that certain terms in the equations are small and so can be neglected.
- Slender-body theory is a methodology used in Stokes flow problems to estimate the force on, or flow field around, a long slender object in a viscous fluid.
- The shallow-water equations can be used to describe a layer of relatively inviscid fluid with a free surface, in which surface gradients are small.
- The Boussinesq equations are applicable to surface waves on thicker layers of fluid and with steeper surface slopes.
- Darcy's law is used for flow in porous media, and works with variables averaged over several pore-widths.
- In rotating systems, the Quasi-geostrophic equations assume an almost perfect balance between pressure gradients and the Coriolis force. It is useful in the study of atmospheric dynamics.
Terminology in fluid dynamics
The concept of pressure is central to the study of both fluid statics and fluid dynamics. A pressure can be identified for every point in a body of fluid, regardless of whether the fluid is in motion or not. Pressure can be measured using an aneroid, Bourdon tube, mercury column, or various other methods.Some of the terminology that is necessary in the study of fluid dynamics is not found in other similar areas of study. In particular, some of the terminology used in fluid dynamics is not used in fluid statics.
Terminology in incompressible fluid dynamics
The concepts of total pressure and dynamic pressure arise from Bernoulli's equation and are significant in the study of all fluid flows. (These two pressures are not pressures in the usual sense—they cannot be measured using an aneroid, Bourdon tube or mercury column.) To avoid potential ambiguity when referring to pressure in fluid dynamics, many authors use the term static pressure to distinguish it from total pressure and dynamic pressure. Static pressure is identical to pressure and can be identified for every point in a fluid flow field.In Aerodynamics, L.J. Clancy writes:[9] To distinguish it from the total and dynamic pressures, the actual pressure of the fluid, which is associated not with its motion but with its state, is often referred to as the static pressure, but where the term pressure alone is used it refers to this static pressure.
A point in a fluid flow where the flow has come to rest (i.e. speed is equal to zero adjacent to some solid body immersed in the fluid flow) is of special significance. It is of such importance that it is given a special name—a stagnation point. The static pressure at the stagnation point is of special significance and is given its own name—stagnation pressure. In incompressible flows, the stagnation pressure at a stagnation point is equal to the total pressure throughout the flow field.
Terminology in compressible fluid dynamics
In a compressible fluid, such as air, the temperature and density are essential when determining the state of the fluid. In addition to the concept of total pressure (also known as stagnation pressure), the concepts of total (or stagnation) temperature and total (or stagnation) density are also essential in any study of compressible fluid flows. To avoid potential ambiguity when referring to temperature and density, many authors use the terms static temperature and static density. Static temperature is identical to temperature; and static density is identical to density; and both can be identified for every point in a fluid flow field.The temperature and density at a stagnation point are called stagnation temperature and stagnation density.
A similar approach is also taken with the thermodynamic properties of compressible fluids. Many authors use the terms total (or stagnation) enthalpy and total (or stagnation) entropy. The terms static enthalpy and static entropy appear to be less common, but where they are used they mean nothing more than enthalpy and entropy respectively, and the prefix "static" is being used to avoid ambiguity with their 'total' or 'stagnation' counterparts. Because the 'total' flow conditions are defined by isentropically bringing the fluid to rest, the total (or stagnation) entropy is by definition always equal to the "static" entropy.




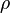 is the fluid density, u is the
is the fluid density, u is the 
 , the net force due to
, the net force due to 






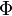 is the viscous dissipation function. The viscous dissipation function governs the rate at which mechanical energy of the flow is converted to heat. The
is the viscous dissipation function. The viscous dissipation function governs the rate at which mechanical energy of the flow is converted to heat. The 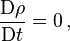






 is infinite for a ≥ −1 but finite for a < −1. So if L(r) is proportional to r−2, then for
is infinite for a ≥ −1 but finite for a < −1. So if L(r) is proportional to r−2, then for 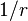 in addition to its rest-frame
in addition to its rest-frame  Coulomb field. This radiation electric field has an accompanying magnetic field, and the whole oscillating electromagnetic radiation field propagates independently of the accelerated charge, carrying away momentum and energy. The energy in the radiation is provided by the work that accelerates the charge. We understand a
Coulomb field. This radiation electric field has an accompanying magnetic field, and the whole oscillating electromagnetic radiation field propagates independently of the accelerated charge, carrying away momentum and energy. The energy in the radiation is provided by the work that accelerates the charge. We understand a 
 is the speed of light,
is the speed of light,  is proper time,
is proper time, 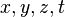 are the usual coordinates of space and time,
are the usual coordinates of space and time,  is the acceleration of the gravitational field, and
is the acceleration of the gravitational field, and  is an arbitrary function of the coordinate but must approach the observed Newtonian value of
is an arbitrary function of the coordinate but must approach the observed Newtonian value of 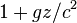 . This is the metric for the gravitational field measured by the supported observer.
. This is the metric for the gravitational field measured by the supported observer.
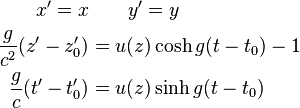
 uniformly accelerated at rate
uniformly accelerated at rate 