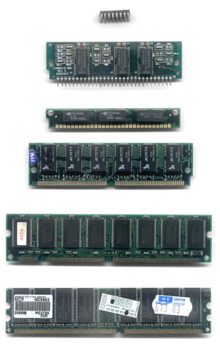
Semiconductor memory

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Semiconductor_memory 

Semiconductor memory is a digital electronic semiconductor device used for digital data storage, such as computer memory. It typically refers to MOS memory, where data is stored within metal–oxide–semiconductor (MOS) memory cells on a silicon integrated circuit memory chip. There are numerous different types using different semiconductor technologies. The two main types of random-access memory (RAM) are static RAM (SRAM), which uses several MOS transistors per memory cell, and dynamic RAM (DRAM), which uses a MOS transistor and a MOS capacitor per cell. Non-volatile memory (such as EPROM, EEPROM and flash memory) uses floating-gate memory cells, which consist of a single floating-gate MOS transistor per cell.

Most types of semiconductor memory have the property of random access, which means that it takes the same amount of time to access any memory location, so data can be efficiently accessed in any random order. This contrasts with data storage media such as hard disks and CDs which read and write data consecutively and therefore the data can only be accessed in the same sequence it was written. Semiconductor memory also has much faster access times than other types of data storage; a byte of data can be written to or read from semiconductor memory within a few nanoseconds, while access time for rotating storage such as hard disks is in the range of milliseconds. For these reasons it is used for primary storage, to hold the program and data the computer is currently working on, among other uses.

As of 2017, semiconductor memory chips sell $124 billion annually, accounting for 30% of the semiconductor industry. Shift registers, processor registers, data buffers and other small digital registers that have no memory address decoding mechanism are typically not referred to as memory although they also store digital data.

Description

See also: Computer memory

In a semiconductor memory chip, each bit of binary data is stored in a tiny circuit called a memory cell consisting of one to several transistors. The memory cells are laid out in rectangular arrays on the surface of the chip. The 1-bit memory cells are grouped in small units called words which are accessed together as a single memory address. Memory is manufactured in word length that is usually a power of two, typically N=1, 2, 4 or 8 bits.

Data is accessed by means of a binary number called a memory address applied to the chip's address pins, which specifies which word in the chip is to be accessed. If the memory address consists of M bits, the number of addresses on the chip is 2^M, each containing an N bit word. Consequently, the amount of data stored in each chip is N2^M bits. The memory storage capacity for M number of address lines is given by 2^M, which is usually in power of two: 2, 4, 8, 16, 32, 64, 128, 256 and 512 and measured in kilobits, megabits, gigabits or terabits, etc. As of 2014 the largest semiconductor memory chips hold a few gigabits of data, but higher capacity memory is constantly being developed. By combining several integrated circuits, memory can be arranged into a larger word length and/or address space than what is offered by each chip, often but not necessarily a power of two.

The two basic operations performed by a memory chip are "read", in which the data contents of a memory word is read out (nondestructively), and "write" in which data is stored in a memory word, replacing any data that was previously stored there. To increase data rate, in some of the latest types of memory chips such as DDR SDRAM multiple words are accessed with each read or write operation.

In addition to standalone memory chips, blocks of semiconductor memory are integral parts of many computer and data processing integrated circuits. For example, the microprocessor chips that run computers contain cache memory to store instructions awaiting execution.

Types

Volatile memory

RAM chips for computers usually come on removable memory modules like these. Additional memory can be added to the computer by plugging in additional modules.

Volatile memory loses its stored data when the power to the memory chip is turned off. However it can be faster and less expensive than non-volatile memory. This type is used for the main memory in most computers, since data is stored on the hard disk while the computer is off. Major types are:

RAM (Random-access memory) – This has become a generic term for any semiconductor memory that can be written to, as well as read from, in contrast to ROM (below), which can only be read. All semiconductor memory, not just RAM, has the property of random access.

• DRAM (Dynamic random-access memory) – This uses metal–oxide–semiconductor (MOS) memory cells consisting of one MOSFET (MOS field-effect transistor) and one MOS capacitor to store each bit. This type of RAM is the cheapest and highest in density, so it is used for the main memory in computers. However, the electric charge that stores the data in the memory cells slowly leaks out, so the memory cells must be periodically refreshed (rewritten) which requires additional circuitry. The refresh process is handled internally by the computer and is transparent to its user.
  • FPM DRAM (Fast page mode DRAM) – An older type of asynchronous DRAM that improved on previous types by allowing repeated accesses to a single "page" of memory to occur at a faster rate. Used in the mid-1990s.
  • EDO DRAM (Extended data out DRAM) – An older type of asynchronous DRAM which had faster access time than earlier types by being able to initiate a new memory access while data from the previous access was still being transferred. Used in the later part of the 1990s.
  • VRAM (Video random access memory) – An older type of dual-ported memory once used for the frame buffers of video adapters (video cards).
  • SDRAM (Synchronous dynamic random-access memory) – This added circuitry to the DRAM chip which synchronizes all operations with a clock signal added to the computer's memory bus. This allowed the chip to process multiple memory requests simultaneously using pipelining, to increase the speed. The data on the chip is also divided into banks which can each work on a memory operation simultaneously. This became the dominant type of computer memory by about the year 2000.
    • DDR SDRAM (Double data rate SDRAM) – This could transfer twice the data (two consecutive words) on each clock cycle by double pumping (transferring data on both the rising and falling edges of the clock pulse). Extensions of this idea are the current (2012) technique being used to increase memory access rate and throughput. Since it is proving difficult to further increase the internal clock speed of memory chips, these chips increase the transfer rate by transferring more data words on each clock cycle
      • DDR2 SDRAM – Transfers 4 consecutive words per internal clock cycle
      • DDR3 SDRAM – Transfers 8 consecutive words per internal clock cycle.
      • DDR4 SDRAM – Transfers 16 consecutive words per internal clock cycle.
    • RDRAM (Rambus DRAM) – An alternate double data rate memory standard that was used on some Intel systems but ultimately lost out to DDR SDRAM.
      • XDR DRAM (Extreme data rate DRAM)
    • SGRAM (Synchronous graphics RAM) – A specialized type of SDRAM made for graphics adaptors (video cards). It can perform graphics-related operations such as bit masking and block write, and can open two pages of memory at once.
      • GDDR SDRAM (Graphics DDR SDRAM)
        • GDDR2
        • GDDR3 SDRAM
        • GDDR4 SDRAM
        • GDDR5 SDRAM
        • GDDR6 SDRAM
    • HBM (High Bandwidth Memory) – A development of SDRAM used in graphics cards that can transfer data at a faster rate. It consists of multiple memory chips stacked on top of one another, with a wider data bus.
  • PSRAM (Pseudostatic RAM) – This is DRAM which has circuitry to perform memory refresh on the chip, so that it acts like SRAM, allowing the external memory controller to be shut down to save energy. It is used in a few game consoles such as the Wii.
• SRAM (Static random-access memory) – This stores each bit of data in a circuit called a flip-flop, made of 4 to 6 transistors. SRAM is less dense and more expensive per bit than DRAM, but faster and does not require memory refresh. It is used for smaller cache memories in computers.
• CAM (Content-addressable memory) – This is a specialized type in which, instead of accessing data using an address, a data word is applied and the memory returns the location if the word is stored in the memory. It is mostly incorporated in other chips such as microprocessors where it is used for cache memory.

Non-volatile memory

Non-volatile memory (NVM) preserves the data stored in it during periods when the power to the chip is turned off. Therefore, it is used for the memory in portable devices, which don't have disks, and for removable memory cards among other uses. Major types are:

• ROM (Read-only memory) – This is designed to hold permanent data, and in normal operation is only read from, not written to. Although many types can be written to, the writing process is slow and usually all the data in the chip must be rewritten at once. It is usually used to store system software which must be immediately accessible to the computer, such as the BIOS program which starts the computer, and the software (microcode) for portable devices and embedded computers such as microcontrollers.
  • MROM (Mask programmed ROM or Mask ROM) – In this type the data is programmed into the chip when the chip is manufactured, so it is only used for large production runs. It cannot be rewritten with new data.
  • PROM (Programmable read-only memory) – In this type the data is written into an existing PROM chip before it is installed in the circuit, but it can only be written once. The data is written by plugging the chip into a device called a PROM programmer.
  • EPROM (Erasable programmable read-only memory) – In this type the data in it can be rewritten by removing the chip from the circuit board, exposing it to an ultraviolet light to erase the existing data, and plugging it into a PROM programmer. The IC package has a small transparent "window" in the top to admit the UV light. It is often used for prototypes and small production run devices, where the program in it may have to be changed at the factory.
    

• • 4M EPROM, showing transparent window used to erase the chip
  • EEPROM (Electrically erasable programmable read-only memory) – In this type the data can be rewritten electrically, while the chip is on the circuit board, but the writing process is slow. This type is used to hold firmware, the low level microcode which runs hardware devices, such as the BIOS program in most computers, so that it can be updated.
• NVRAM (Non-volatile random-access memory)
  • FRAM (Ferroelectric RAM) – One type of nonvolatile RAM.
• Flash memory – In this type the writing process is intermediate in speed between EEPROMS and RAM memory; it can be written to, but not fast enough to serve as main memory. It is often used as a semiconductor version of a hard disk, to store files. It is used in portable devices such as PDAs, USB flash drives, and removable memory cards used in digital cameras and cellphones.

History

See also: Computer memory and Memory cell (computing)

Early computer memory consisted of magnetic-core memory, as early solid-state electronic semiconductors, including transistors such as the bipolar junction transistor (BJT), were impractical for use as digital storage elements (memory cells). The earliest semiconductor memory dates back to the early 1960s, with bipolar memory, which used bipolar transistors. Bipolar semiconductor memory made from discrete devices was first shipped by Texas Instruments to the United States Air Force in 1961. The same year, the concept of solid-state memory on an integrated circuit (IC) chip was proposed by applications engineer Bob Norman at Fairchild Semiconductor. The first bipolar semiconductor memory IC chip was the SP95 introduced by IBM in 1965. While bipolar memory offered improved performance over magnetic-core memory, it could not compete with the lower price of magnetic-core memory, which remained dominant up until the late 1960s. Bipolar memory failed to replace magnetic-core memory because bipolar flip-flop circuits were too large and expensive.

MOS memory

See also: MOSFET

The advent of the metal–oxide–semiconductor field-effect transistor (MOSFET), invented by Mohamed M. Atalla and Dawon Kahng at Bell Labs in 1959, enabled the practical use of metal–oxide–semiconductor (MOS) transistors as memory cell storage elements, a function previously served by magnetic cores in computer memory. MOS memory was developed by John Schmidt at Fairchild Semiconductor in 1964. In addition to higher performance, MOS memory was cheaper and consumed less power than magnetic-core memory. This led to MOSFETs eventually replacing magnetic cores as the standard storage elements in computer memory.

In 1965, J. Wood and R. Ball of the Royal Radar Establishment proposed digital storage systems that use CMOS (complementary MOS) memory cells, in addition to MOSFET power devices for the power supply, switched cross-coupling, switches and delay-line storage. The development of silicon-gate MOS integrated circuit (MOS IC) technology by Federico Faggin at Fairchild in 1968 enabled the production of MOS memory chips. NMOS memory was commercialized by IBM in the early 1970s. MOS memory overtook magnetic core memory as the dominant memory technology in the early 1970s.

The term "memory" when used with reference to computers most often refers to volatile random-access memory (RAM). The two main types of volatile RAM are static random-access memory (SRAM) and dynamic random-access memory (DRAM). Bipolar SRAM was invented by Robert Norman at Fairchild Semiconductor in 1963, followed by the development of MOS SRAM by John Schmidt at Fairchild in 1964. SRAM became an alternative to magnetic-core memory, but required six MOS transistors for each bit of data. Commercial use of SRAM began in 1965, when IBM introduced their SP95 SRAM chip for the System/360 Model 95.

Toshiba introduced bipolar DRAM memory cells for its Toscal BC-1411 electronic calculator in 1965.  While it offered improved performance over magnetic-core memory, bipolar DRAM could not compete with the lower price of the then dominant magnetic-core memory. MOS technology is the basis for modern DRAM. In 1966, Dr. Robert H. Dennard at the IBM Thomas J. Watson Research Center was working on MOS memory. While examining the characteristics of MOS technology, he found it was capable of building capacitors, and that storing a charge or no charge on the MOS capacitor could represent the 1 and 0 of a bit, while the MOS transistor could control writing the charge to the capacitor. This led to his development of a single-transistor DRAM memory cell. In 1967, Dennard filed a patent under IBM for a single-transistor DRAM memory cell, based on MOS technology. This led to the first commercial DRAM IC chip, the Intel 1103, in October 1970. Synchronous dynamic random-access memory (SDRAM) later debuted with the Samsung KM48SL2000 chip in 1992.

The term "memory" is also often used to refer to non-volatile memory, specifically flash memory. It has origins in read-only memory (ROM). Programmable read-only memory (PROM) was invented by Wen Tsing Chow in 1956, while working for the Arma Division of the American Bosch Arma Corporation. In 1967, Dawon Kahng and Simon Sze of Bell Labs proposed that the floating gate of a MOS semiconductor device could be used for the cell of a reprogrammable read-only memory (ROM), which led to Dov Frohman of Intel inventing EPROM (erasable PROM) in 1971. EEPROM (electrically erasable PROM) was developed by Yasuo Tarui, Yutaka Hayashi and Kiyoko Naga at the Electrotechnical Laboratory in 1972. Flash memory was invented by Fujio Masuoka at Toshiba in the early 1980s. Masuoka and colleagues presented the invention of NOR flash in 1984, and then NAND flash in 1987. Toshiba commercialized NAND flash memory in 1987.

Applications

See also: List of MOSFET applications

 

MOS memory applications

MOS memory type	Abbr.	MOS memory cell	Applications
Static random-access memory	SRAM	MOSFETs	Cache memory, cell phones, eSRAM, mainframes, multimedia computers, networking, personal computers, servers, supercomputers, telecommunications, workstations, DVD disk buffer, data buffer, nonvolatile BIOS memory
Dynamic random-access memory	DRAM	MOSFET, MOS capacitor	Camcorders, embedded logic, eDRAM, graphics card, hard disk drive (HDD), networks, personal computers, personal digital assistants, printers, main computer memory, desktop computers, servers, solid-state drives, video memory, framebuffer memory
Ferroelectric random-access memory	FRAM	MOSFET, MOS capacitor	Non-volatile memory, radio-frequency identification (RF identification), smart cards
Read-only memory	ROM	MOSFET	Character generators, electronic musical instruments, laser printer fonts, video game ROM cartridges, word processor dictionary data
Erasable programmable read-only memory	EPROM	Floating-gate MOSFET	CD-ROM drives, embedded memory, code storage, modems
Electrically erasable programmable read-only memory	EEPROM	Floating-gate MOSFET	Anti-lock braking systems, air bags, car radios, cell phones, consumer electronics, cordless telephones, disk drives, embedded memory, flight controllers, military technology, modems, pagers, printers, set-top box, smart cards
Flash memory	Flash	Floating-gate MOSFET	ATA controllers, battery-powered applications, telecommunications, code storage, digital cameras, MP3 players, portable media players, BIOS memory, USB flash drive, digital TV, e-books, memory cards, mobile devices, set-top box, smartphones, solid-state drives, tablet computers
Non-volatile random-access memory	NVRAM	Floating-gate MOSFETs	Medical equipment, spacecraft

Optical resolution

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Optical_resolution 

Optical resolution describes the ability of an imaging system to resolve detail, in the object that is being imaged.

An imaging system may have many individual components, including one or more lenses, and/or recording and display components. Each of these contributes (given suitable design, and adequate alignment) to the optical resolution of the system; the environment in which the imaging is done often is a further important factor.

Lateral resolution

Resolution depends on the distance between two distinguishable radiating points. The sections below describe the theoretical estimates of resolution, but the real values may differ. The results below are based on mathematical models of Airy discs, which assumes an adequate level of contrast. In low-contrast systems, the resolution may be much lower than predicted by the theory outlined below. Real optical systems are complex, and practical difficulties often increase the distance between distinguishable point sources.

The resolution of a system is based on the minimum distance ${\displaystyle r}$ at which the points can be distinguished as individuals. Several standards are used to determine, quantitatively, whether or not the points can be distinguished. One of the methods specifies that, on the line between the center of one point and the next, the contrast between the maximum and minimum intensity be at least 26% lower than the maximum. This corresponds to the overlap of one Airy disk on the first dark ring in the other. This standard for separation is also known as the Rayleigh criterion. In symbols, the distance is defined as follows:

${\displaystyle r={\frac {1.22\lambda }{2n\sin {\theta }}}={\frac {0.61\lambda }{\mathrm {NA} }}}$

where

${\displaystyle r}$ is the minimum distance between resolvable points, in the same units as ${\displaystyle \lambda }$ is specified

${\displaystyle \lambda }$ is the wavelength of light, emission wavelength, in the case of fluorescence,

${\displaystyle n}$ is the index of refraction of the media surrounding the radiating points,

${\displaystyle \theta }$ is the half angle of the pencil of light that enters the objective, and

${\displaystyle \mathrm {NA} }$ is the numerical aperture

This formula is suitable for confocal microscopy, but is also used in traditional microscopy. In confocal laser-scanned microscopes, the full-width half-maximum (FWHM) of the point spread function is often used to avoid the difficulty of measuring the Airy disc. This, combined with the rastered illumination pattern, results in better resolution, but it is still proportional to the Rayleigh-based formula given above.

${\displaystyle r={\frac {0.4\lambda }{\mathrm {NA} }}}$

Also common in the microscopy literature is a formula for resolution that treats the above-mentioned concerns about contrast differently. The resolution predicted by this formula is proportional to the Rayleigh-based formula, differing by about 20%. For estimating theoretical resolution, it may be adequate.

${\displaystyle r={\frac {\lambda }{2n\sin {\theta }}}={\frac {\lambda }{2\mathrm {NA} }}}$

When a condenser is used to illuminate the sample, the shape of the pencil of light emanating from the condenser must also be included.

${\displaystyle r={\frac {1.22\lambda }{\mathrm {NA} _{obj}+\mathrm {NA} _{cond}}}}$

In a properly configured microscope, ${\displaystyle \mathrm {NA} _{obj}+\mathrm {NA} _{cond}=2\mathrm {NA} _{obj}}$.

The above estimates of resolution are specific to the case in which two identical very small samples that radiate incoherently in all directions. Other considerations must be taken into account if the sources radiate at different levels of intensity, are coherent, large, or radiate in non-uniform patterns.

Lens resolution

The ability of a lens to resolve detail is usually determined by the quality of the lens, but is ultimately limited by diffraction. Light coming from a point source in the object diffracts through the lens aperture such that it forms a diffraction pattern in the image, which has a central spot and surrounding bright rings, separated by dark nulls; this pattern is known as an Airy pattern, and the central bright lobe as an Airy disk. The angular radius of the Airy disk (measured from the center to the first null) is given by:

${\displaystyle \theta =1.22{\frac {\lambda }{D}}}$		where θ is the angular resolution in radians, λ is the wavelength of light in meters, and D is the diameter of the lens aperture in meters.

Two adjacent points in the object give rise to two diffraction patterns. If the angular separation of the two points is significantly less than the Airy disk angular radius, then the two points cannot be resolved in the image, but if their angular separation is much greater than this, distinct images of the two points are formed and they can therefore be resolved. Rayleigh defined the somewhat arbitrary "Rayleigh criterion" that two points whose angular separation is equal to the Airy disk radius to first null can be considered to be resolved. It can be seen that the greater the diameter of the lens or its aperture, the greater the resolution. Astronomical telescopes have increasingly large lenses so they can 'see' ever finer detail in the stars.

Only the very highest quality lenses have diffraction limited resolution, however, and normally the quality of the lens limits its ability to resolve detail. This ability is expressed by the Optical Transfer Function which describes the spatial (angular) variation of the light signal as a function of spatial (angular) frequency. When the image is projected onto a flat plane, such as photographic film or a solid state detector, spatial frequency is the preferred domain, but when the image is referred to the lens alone, angular frequency is preferred. OTF may be broken down into the magnitude and phase components as follows:

${\displaystyle \mathbf {OTF(\xi ,\eta )} =\mathbf {MTF(\xi ,\eta )} \cdot \mathbf {PTF(\xi ,\eta )} }$

where

${\displaystyle \mathbf {MTF(\xi ,\eta )} =|\mathbf {OTF(\xi ,\eta )} |}$

${\displaystyle \mathbf {PTF(\xi ,\eta )} =e^{-i2\cdot \pi \cdot \lambda (\xi ,\eta )}}$

and ${\displaystyle (\xi ,\eta )}$ are spatial frequency in the x- and y-plane, respectively.

The OTF accounts for aberration, which the limiting frequency expression above does not. The magnitude is known as the Modulation Transfer Function (MTF) and the phase portion is known as the Phase Transfer Function (PTF).

In imaging systems, the phase component is typically not captured by the sensor. Thus, the important measure with respect to imaging systems is the MTF.

Phase is critically important to adaptive optics and holographic systems.

Sensor resolution (spatial)

Some optical sensors are designed to detect spatial differences in electromagnetic energy. These include photographic film, solid-state devices (CCD, CMOS detectors, and infrared detectors like PtSi and InSb), tube detectors (vidicon, plumbicon, and photomultiplier tubes used in night-vision devices), scanning detectors (mainly used for IR), pyroelectric detectors, and microbolometer detectors. The ability of such a detector to resolve those differences depends mostly on the size of the detecting elements.

Spatial resolution is typically expressed in line pairs per millimeter (lppmm), lines (of resolution, mostly for analog video), contrast vs. cycles/mm, or MTF (the modulus of OTF). The MTF may be found by taking the two-dimensional Fourier transform of the spatial sampling function. Smaller pixels result in wider MTF curves and thus better detection of higher frequency energy.

This is analogous to taking the Fourier transform of a signal sampling function; as in that case, the dominant factor is the sampling period, which is analogous to the size of the picture element (pixel).

Other factors include pixel noise, pixel cross-talk, substrate penetration, and fill factor.

A common problem among non-technicians is the use of the number of pixels on the detector to describe the resolution. If all sensors were the same size, this would be acceptable. Since they are not, the use of the number of pixels can be misleading. For example, a 2-megapixel camera of 20-micrometre-square pixels will have worse resolution than a 1-megapixel camera with 8-micrometre pixels, all else being equal.

For resolution measurement, film manufacturers typically publish a plot of Response (%) vs. Spatial Frequency (cycles per millimeter). The plot is derived experimentally. Solid state sensor and camera manufacturers normally publish specifications from which the user may derive a theoretical MTF according to the procedure outlined below. A few may also publish MTF curves, while others (especially intensifier manufacturers) will publish the response (%) at the Nyquist frequency, or, alternatively, publish the frequency at which the response is 50%.

To find a theoretical MTF curve for a sensor, it is necessary to know three characteristics of the sensor: the active sensing area, the area comprising the sensing area and the interconnection and support structures ("real estate"), and the total number of those areas (the pixel count). The total pixel count is almost always given. Sometimes the overall sensor dimensions are given, from which the real estate area can be calculated. Whether the real estate area is given or derived, if the active pixel area is not given, it may be derived from the real estate area and the fill factor, where fill factor is the ratio of the active area to the dedicated real estate area.

${\displaystyle FF={\frac {a\cdot b}{c\cdot d}}}$

where

• the active area of the pixel has dimensions a×b
• the pixel real estate has dimensions c×d

In Gaskill's notation, the sensing area is a 2D comb(x, y) function of the distance between pixels (the pitch), convolved with a 2D rect(x, y) function of the active area of the pixel, bounded by a 2D rect(x, y) function of the overall sensor dimension. The Fourier transform of this is a ${\displaystyle \operatorname {comb} (\xi ,\eta )}$ function governed by the distance between pixels, convolved with a ${\displaystyle \operatorname {sinc} (\xi ,\eta )}$ function governed by the number of pixels, and multiplied by the ${\displaystyle \operatorname {sinc} (\xi ,\eta )}$ function corresponding to the active area. That last function serves as an overall envelope to the MTF function; so long as the number of pixels is much greater than one (1), then the active area size dominates the MTF.

Sampling function:

${\displaystyle \mathbf {S} (x,y)=\left[\operatorname {comb} \left({\frac {x}{c}},{\frac {y}{d}}\right)*\operatorname {rect} \left({\frac {x}{a}},{\frac {y}{b}}\right)\right]\cdot \operatorname {rect} \left({\frac {x}{M\cdot c}},{\frac {y}{N\cdot d}}\right)}$

where the sensor has M×N pixels

${\displaystyle \mathbf {MTF_{sensor}} (\xi ,\eta )}$	${\displaystyle ={\mathcal {FF}}(\mathbf {S} (x,y))}$
	${\displaystyle =[\operatorname {sinc} ((M\cdot c)\cdot \xi ,(N\cdot d)\cdot \eta )*\operatorname {comb} (c\cdot \xi ,d\cdot \eta )]\cdot \operatorname {sinc} (a\cdot \xi ,b\cdot \eta )}$

Sensor resolution (temporal)

An imaging system running at 24 frames per second is essentially a discrete sampling system that samples a 2D area. The same limitations described by Nyquist apply to this system as to any signal sampling system.

All sensors have a characteristic time response. Film is limited at both the short resolution and the long resolution extremes by reciprocity breakdown. These are typically held to be anything longer than 1 second and shorter than 1/10,000 second. Furthermore, film requires a mechanical system to advance it through the exposure mechanism, or a moving optical system to expose it. These limit the speed at which successive frames may be exposed.

CCD and CMOS are the modern preferences for video sensors. CCD is speed-limited by the rate at which the charge can be moved from one site to another. CMOS has the advantage of having individually addressable cells, and this has led to its advantage in the high speed photography industry.

Vidicons, Plumbicons, and image intensifiers have specific applications. The speed at which they can be sampled depends upon the decay rate of the phosphor used. For example, the P46 phosphor has a decay time of less than 2 microseconds, while the P43 decay time is on the order of 2-3 milliseconds. The P43 is therefore unusable at frame rates above 1000 frames per second (frame/s). See § External links for links to phosphor information.

Pyroelectric detectors respond to changes in temperature. Therefore, a static scene will not be detected, so they require choppers. They also have a decay time, so the pyroelectric system temporal response will be a bandpass, while the other detectors discussed will be a lowpass.

If objects within the scene are in motion relative to the imaging system, the resulting motion blur will result in lower spatial resolution. Short integration times will minimize the blur, but integration times are limited by sensor sensitivity. Furthermore, motion between frames in motion pictures will impact digital movie compression schemes (e.g. MPEG-1, MPEG-2). Finally, there are sampling schemes that require real or apparent motion inside the camera (scanning mirrors, rolling shutters) that may result in incorrect rendering of image motion. Therefore, sensor sensitivity and other time-related factors will have a direct impact on spatial resolution.

Analog bandwidth effect on resolution

The spatial resolution of digital systems (e.g. HDTV and VGA) are fixed independently of the analog bandwidth because each pixel is digitized, transmitted, and stored as a discrete value. Digital cameras, recorders, and displays must be selected so that the resolution is identical from camera to display. However, in analog systems, the resolution of the camera, recorder, cabling, amplifiers, transmitters, receivers, and display may all be independent and the overall system resolution is governed by the bandwidth of the lowest performing component.

In analog systems, each horizontal line is transmitted as a high-frequency analog signal. Each picture element (pixel) is therefore converted to an analog electrical value (voltage), and changes in values between pixels therefore become changes in voltage. The transmission standards require that the sampling be done in a fixed time (outlined below), so more pixels per line becomes a requirement for more voltage changes per unit time, i.e. higher frequency. Since such signals are typically band-limited by cables, amplifiers, recorders, transmitters, and receivers, the band-limitation on the analog signal acts as an effective low-pass filter on the spatial resolution. The difference in resolutions between VHS (240 discernible lines per scanline), Betamax (280 lines), and the newer ED Beta format (500 lines) is explained primarily by the difference in the recording bandwidth.

In the NTSC transmission standard, each field contains 262.5 lines, and 59.94 fields are transmitted every second. Each line must therefore take 63 microseconds, 10.7 of which are for reset to the next line. Thus, the retrace rate is 15.734 kHz. For the picture to appear to have approximately the same horizontal and vertical resolution (see Kell factor), it should be able to display 228 cycles per line, requiring a bandwidth of 4.28 MHz. If the line (sensor) width is known, this may be converted directly into cycles per millimeter, the unit of spatial resolution.

B/G/I/K television system signals (usually used with PAL colour encoding) transmit frames less often (50 Hz), but the frame contains more lines and is wider, so bandwidth requirements are similar.

Note that a "discernible line" forms one half of a cycle (a cycle requires a dark and a light line), so "228 cycles" and "456 lines" are equivalent measures.

System resolution

There are two methods by which to determine "system resolution" (in the sense that omits the eye, or other final reception of the optical information). The first is to perform a series of two-dimensional convolutions, first with the image and the lens, and then, with that procedure's result and a sensor (and so on through all of the components of the system). Not only is this computationally expensive, but normally it also requires repetition of the process, for each additional object that is to be imaged.

${\displaystyle \mathbf {Image(x,y)=} }$	${\displaystyle \mathbf {Object(x,y)*PSF_{atmosphere}(x,y)*} }$
	${\displaystyle \mathbf {PSF_{lens}(x,y)*PSF_{sensor}(x,y)*} }$
	${\displaystyle \mathbf {PSF_{transmission}(x,y)*PSF_{display}(x,y)} }$

The other method is to transform each of the components of the system into the spatial frequency domain, and then to multiply the 2-D results. A system response may be determined without reference to an object. Although this method is considerably more difficult to comprehend conceptually, it becomes easier to use computationally, especially when different design iterations or imaged objects are to be tested.

The transformation to be used is the Fourier transform.

${\displaystyle \mathbf {MTF_{sys}(\xi ,\eta )=} }$	${\displaystyle \mathbf {MTF_{atmosphere}(\xi ,\eta )\cdot MTF_{lens}(\xi ,\eta )\cdot } }$
	${\displaystyle \mathbf {MTF_{sensor}(\xi ,\eta )\cdot MTF_{transmission}(\xi ,\eta )\cdot } }$
	${\displaystyle \mathbf {MTF_{display}(\xi ,\eta )} }$

Ocular resolution

The human eye is a limiting feature of many systems, when the goal of the system is to present data to humans for processing.

For example, in a security or air traffic control function, the display and work station must be constructed so that average humans can detect problems and direct corrective measures. Other examples are when a human is using eyes to carry out a critical task such as flying (piloting by visual reference), driving a vehicle, and so forth.

The best visual acuity of the human eye at its optical centre (the fovea) is less than 1 arc minute per line pair, reducing rapidly away from the fovea.

The human brain requires more than just a line pair to understand what the eye is imaging. Johnson's criteria defines the number of line pairs of ocular resolution, or sensor resolution, needed to recognize or identify an item.

Atmospheric resolution

Systems looking through long atmospheric paths may be limited by turbulence. A key measure of the quality of atmospheric turbulence is the seeing diameter, also known as Fried's seeing diameter. A path which is temporally coherent is known as an isoplanatic patch.

Large apertures may suffer from aperture averaging, the result of several paths being integrated into one image.

Turbulence scales with wavelength at approximately a 6/5 power. Thus, seeing is better at infrared wavelengths than at visible wavelengths.

Short exposures suffer from turbulence less than longer exposures due to the "inner" and "outer" scale turbulence; short is considered to be much less than 10 ms for visible imaging (typically, anything less than 2 ms). Inner scale turbulence arises due to the eddies in the turbulent flow, while outer scale turbulence arises from large air mass flow. These masses typically move slowly, and so are reduced by decreasing the integration period.

A system limited only by the quality of the optics is said to be diffraction-limited. However, since atmospheric turbulence is normally the limiting factor for visible systems looking through long atmospheric paths, most systems are turbulence-limited. Corrections can be made by using adaptive optics or post-processing techniques.

${\displaystyle \operatorname {MTF} _{s}(\nu )=e^{-3.44\cdot (\lambda f\nu /r_{0})^{5/3}\cdot [1-b\cdot (\lambda f\nu /D)^{1/3}]}}$

where

${\displaystyle \nu }$ is the spatial frequency

${\displaystyle \lambda }$ is the wavelength

f is the focal length

D is the aperture diameter

b is a constant (1 for far-field propagation)

and ${\displaystyle r_{0}}$ is Fried's seeing diameter

Measuring optical resolution

A variety of measurement systems are available, and use may depend upon the system being tested.

Typical test charts for Contrast Transfer Function (CTF) consist of repeated bar patterns (see Discussion below). The limiting resolution is measured by determining the smallest group of bars, both vertically and horizontally, for which the correct number of bars can be seen. By calculating the contrast between the black and white areas at several different frequencies, however, points of the CTF can be determined with the contrast equation.

${\displaystyle {\text{contrast}}={\frac {C_{\max }-C_{\min }}{C_{\max }+C_{\min }}}}$

where

${\displaystyle C_{\max }}$ is the normalized value of the maximum (for example, the voltage or grey value of the white area)

${\displaystyle C_{\min }}$ is the normalized value of the minimum (for example, the voltage or grey value of the black area)

When the system can no longer resolve the bars, the black and white areas have the same value, so Contrast = 0. At very low spatial frequencies, C_max = 1 and C_min = 0 so Modulation = 1. Some modulation may be seen above the limiting resolution; these may be aliased and phase-reversed.

When using other methods, including the interferogram, sinusoid, and the edge in the ISO 12233 target, it is possible to compute the entire MTF curve. The response to the edge is similar to a step response, and the Fourier Transform of the first difference of the step response yields the MTF.

Interferogram

An interferogram created between two coherent light sources may be used for at least two resolution-related purposes. The first is to determine the quality of a lens system (see LUPI), and the second is to project a pattern onto a sensor (especially photographic film) to measure resolution.

NBS 1010a/ ISO #2 target

This 5 bar resolution test chart is often used for evaluation of microfilm systems and scanners. It is convenient for a 1:1 range (typically covering 1-18 cycles/mm) and is marked directly in cycles/mm. Details can be found in ISO-3334.

USAF 1951 target

SilverFast Resolution Target USAF 1951 for determining a scanner's optimum resolution

The USAF 1951 resolution test target consists of a pattern of 3 bar targets. Often found covering a range of 0.25 to 228 cycles/mm. Each group consists of six elements. The group is designated by a group number (-2, -1, 0, 1, 2, etc.) which is the power to which 2 should be raised to obtain the spatial frequency of the first element (e.g., group -2 is 0.25 line pairs per millimeter). Each element is the 6th root of 2 smaller than the preceding element in the group (e.g. element 1 is 2^0, element 2 is 2^(-1/6), element 3 is 2(-1/3), etc.). By reading off the group and element number of the first element which cannot be resolved, the limiting resolution may be determined by inspection. The complex numbering system and use of a look-up chart can be avoided by use of an improved but not standardized layout chart, which labels the bars and spaces directly in cycles/mm using OCR-A extended font.

${\displaystyle Resolution=2^{{group}+{\frac {element-1}{6}}}}$

NBS 1952 target

The NBS 1952 target is a 3 bar pattern (long bars). The spatial frequency is printed alongside each triple bar set, so the limiting resolution may be determined by inspection. This frequency is normally only as marked after the chart has been reduced in size (typically 25 times). The original application called for placing the chart at a distance 26 times the focal length of the imaging lens used. The bars above and to the left are in sequence, separated by approximately the square root of two (12, 17, 24, etc.), while the bars below and to the left have the same separation but a different starting point (14, 20, 28, etc.)

EIA 1956 video resolution target

EIA 1956 video resolution target

The EIA 1956 resolution chart was specifically designed to be used with television systems. The gradually expanding lines near the center are marked with periodic indications of the corresponding spatial frequency. The limiting resolution may be determined by inspection. The most important measure is the limiting horizontal resolution, since the vertical resolution is typically determined by the applicable video standard (I/B/G/K/NTSC/NTSC-J).

IEEE Std 208-1995 target

The IEEE 208-1995 resolution target is similar to the EIA target. Resolution is measured in horizontal and vertical TV lines.

ISO 12233 target

The ISO 12233 target was developed for digital camera applications, since modern digital camera spatial resolution may exceed the limitations of the older targets. It includes several knife-edge targets for the purpose of computing MTF by Fourier transform. They are offset from the vertical by 5 degrees so that the edges will be sampled in many different phases, which allow estimation of the spatial frequency response beyond the Nyquist frequency of the sampling.

Random test patterns

The idea is analogous to the use of a white noise pattern in acoustics to determine system frequency response.

Monotonically increasing sinusoid patterns

The interferogram used to measure film resolution can be synthesized on personal computers and used to generate a pattern for measuring optical resolution. See especially Kodak MTF curves.

Multiburst

A multiburst signal is an electronic waveform used to test analog transmission, recording, and display systems. The test pattern consists of several short periods of specific frequencies. The contrast of each may be measured by inspection and recorded, giving a plot of attenuation vs. frequency. The NTSC3.58 multiburst pattern consists of 500 kHz, 1 MHz, 2 MHz, 3 MHz, and 3.58 MHz blocks. 3.58 MHz is important because it is the chrominance frequency for NTSC video.

Discussion

Using a bar target that the resulting measure is the contrast transfer function (CTF) and not the MTF. The difference arises from the subharmonics of the square waves and can be easily computed.