Shape of the universe

From Wikipedia, the free encyclopedia

The shape of the Universe is the local and global geometry of the universe, in terms of both curvature and topology (though, strictly speaking, the concept goes beyond both). When physicists describe the Universe as being flat or nearly flat, they're talking geometry: how space and time are warped according to general relativity. When they talk about whether it is open or closed, they're referring to its topology.^[1] Although the shape of the Universe is still a matter of debate in physical cosmology, the recent Wilkinson Microwave Anisotropy Probe (WMAP) measurements allow the statement that "We now know that the universe is flat with only a 0.4% margin of error", according to NASA scientists. ^{[2] [3]} Theorists have been trying to construct a formal mathematical model of the shape of the Universe. In formal terms, this is a 3-manifold model corresponding to the spatial section (in comoving coordinates) of the 4-dimensional space-time of the Universe. The model most theorists currently use is the so-called Friedmann–Lemaître–Robertson–Walker (FLRW) model. According to cosmologists, on this model the observational data best fit with the conclusion that the shape of the Universe is infinite and flat,^[4] but the data are also consistent with other possible shapes, such as the so-called Poincaré dodecahedral space^[5][6] and the Picard horn.^[7]

Two aspects of shape

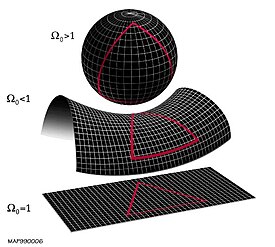
The local geometry of the Universe is determined by whether the density parameter Ω is greater than, less than, or equal to 1.
From top to bottom: a spherical universe with Ω > 1, a hyperbolic universe with Ω < 1, and a flat universe with Ω = 1. Note that these depictions of two-dimensional surfaces are merely easily visualizable analogs to the 3-dimensional structure of (local) space.

Describing the shape of the Universe requires a consideration of two aspects:

1. its local geometry, which mostly concerns the curvature of the Universe, particularly the observable universe, and
2. its global geometry, which concerns the topology of the Universe as a whole.

If the observable universe encompasses the entire universe, we may be able to determine the global structure of the entire universe by observation. However, if the observable universe is smaller than the entire universe, our observations will be limited to only a part of the whole, and we may not be able to determine its global geometry through measurement. It is possible to construct different mathematical models of the global geometry of the entire universe all of which are consistent with current observational data. For example, the observable universe may be many orders of magnitude smaller than the entire universe. The Universe may be small in some dimensions and not in others (analogous to the way a cuboid is longer in the dimension of length than it is in the dimensions of width and depth). To test whether a given mathematical model describes the Universe accurately, scientists look for the model's novel implications—what are some phenomena in the Universe that we have not yet observed, but that must exist if the model is correct—and they devise experiments to test whether those phenomena occur or not. For example, if the Universe is a small closed loop, one would expect to see multiple images of an object in the sky, although not necessarily images of the same age.

Cosmologists normally work with a given space-like slice of spacetime called the comoving coordinates, the existence of a preferred set of which is possible and widely accepted in present-day physical cosmology. The section of spacetime that can be observed is the backward light cone (all points within the cosmic light horizon, given time to reach a given observer), while the related term Hubble volume can be used to describe either the past light cone or comoving space up to the surface of last scattering. To speak of "the shape of the universe (at a point in time)" is ontologically naive from the point of view of special relativity alone: due to the relativity of simultaneity we cannot speak of different points in space as being "at the same point in time" nor, therefore, of "the shape of the universe at a point in time".

Local geometry (spatial curvature)

The local geometry is the curvature describing any arbitrary point in the observable universe (averaged on a sufficiently large scale). Many astronomical observations, such as those from supernovae and the Cosmic Microwave Background (CMB) radiation, show the observable universe to be very close to homogeneous and isotropic and infer it to be accelerating.

FLRW model of the universe

In General Relativity, this is modelled by the Friedmann–Lemaître–Robertson–Walker (FLRW) model. This model, which can be represented by the Friedmann equations, provides a curvature (often referred to as geometry) of the Universe based on the mathematics of fluid dynamics, i.e. it models the matter within the Universe as a perfect fluid. Although stars and structures of mass can be introduced into an "almost FLRW" model, a strictly FLRW model is used to approximate the local geometry of the observable universe.

Another way of saying this is that if all forms of dark energy are ignored, then the curvature of the Universe can be determined by measuring the average density of matter within it, assuming that all matter is evenly distributed (rather than the distortions caused by 'dense' objects such as galaxies).

This assumption is justified by the observations that, while the Universe is "weakly" inhomogeneous and anisotropic (see the large-scale structure of the cosmos), it is on average homogeneous and isotropic.

The homogeneous and isotropic universe allows for a spatial geometry with a constant curvature. One aspect of local geometry to emerge from General Relativity and the FLRW model is that the density parameter, Omega (Ω), is related to the curvature of space. Omega is the average density of the Universe divided by the critical energy density, i.e. that required for the Universe to be flat (zero curvature).

The curvature of space is a mathematical description of whether or not the Pythagorean theorem is valid for spatial coordinates. In the latter case, it provides an alternative formula for expressing local relationships between distances:

• If the curvature is zero, then Ω = 1, and the Pythagorean theorem is correct;
• If Ω < 1, there is positive curvature; and
• if Ω < 1 there is negative curvature.

In the last two cases, the Pythagorean theorem is invalid (but discrepancies are only detectable in triangles whose sides' lengths are of cosmological scale).

If you measure the circumferences of circles of steadily larger diameters and divide the former by the latter, all three geometries give a value very close to π for small enough diameters but the ratio departs from π for larger diameters unless Ω = 1:

• For Ω < 1 (the sphere, see diagram) the ratio falls below π: indeed, a great circle on a sphere has circumference only twice its diameter.
• For Ω < 1 the ratio rises above π.

Astronomical measurements of both matter-energy density of the Universe and spacetime intervals using supernova events constrain the spatial curvature to be very close to zero, although they do not constrain its sign. This means that although the local geometries of spacetime are generated by the theory of relativity based on spacetime intervals, we can approximate 3-space by the familiar Euclidean geometry.

Possible local geometries

There are three categories for the possible spatial geometries of constant curvature, depending on the sign of the curvature. If the curvature is exactly zero, then the local geometry is flat; if it is positive, then the local geometry is spherical, and if it is negative then the local geometry is hyperbolic.

The geometry of the Universe is usually represented in the system of comoving coordinates, according to which the expansion of the Universe can be ignored. Comoving coordinates form a single frame of reference according to which the Universe has a static geometry of three spatial dimensions.

Under the assumption that the Universe is homogeneous and isotropic, the curvature of the observable universe, or the local geometry, is described by one of the three "primitive" geometries (in mathematics these are called the model geometries):

• 3-dimensional Flat Euclidean geometry, generally notated as E³
• 3-dimensional spherical geometry with a small curvature, often notated as S³
• 3-dimensional hyperbolic geometry with a small curvature

Even if the Universe is not exactly spatially flat, the spatial curvature is close enough to zero to place the radius at approximately the horizon of the observable universe or beyond.

Global structure: geometry and topology

Global structure covers the geometry and the topology of the whole universe—both the observable universe and beyond. While the local geometry does not determine the global geometry completely, it does limit the possibilities, particularly a geometry of a constant curvature. For this discussion, the Universe is taken to be a geodesic manifold, free of topological defects; relaxing either of these complicates the analysis considerably.

A global geometry is a local geometry plus a topology. It follows that a topology alone does not give a global geometry: for instance, Euclidean 3-space and hyperbolic 3-space have the same topology but different global geometries.

Investigations within the study of global structure of include

• Whether the Universe is infinite or finite in extent
• The scale or size of the entire universe (if it is finite)
• Whether the geometry is flat, positively curved, or negatively curved
• Whether the topology is simply connected like a sphere or multiply connected like a torus

Infinite or finite

One of the presently unanswered questions about the Universe is whether it is infinite or finite in extent.
Mathematically, the question of whether the Universe is infinite or finite is referred to as boundedness. An infinite universe (unbounded metric space) means that there are points arbitrarily far apart: for any distance d, there are points that are of a distance at least d apart. A finite universe is a bounded metric space, where there is some distance d such that all points are within distance d of each other. The smallest such d is called the diameter of the Universe, in which case the Universe has a well-defined "volume" or "scale."

Closed manifolds

Many finite mathematical spaces, e.g. a disc, have an edge or boundary. Spaces that have an edge are difficult to treat, both conceptually and mathematically. Namely, it is very difficult to state what would happen at the edge of such a universe. For this reason, spaces that have an edge are typically excluded from consideration. However, there exist many finite spaces, such as the 3-sphere and 3-torus, which have no edges. Mathematically, these spaces are referred to as being compact without boundary. The term compact basically means that it is finite in extent ("bounded") and is a closed set. The term "without boundary" means that the space has no edges. Moreover, so that calculus can be applied, the Universe is typically assumed to be a differentiable manifold. A mathematical object that possess all these properties, compact without boundary and differentiable, is termed a closed manifold. The 3-sphere and 3-torus are both closed manifolds.

Scale

For spherical and hyperbolic spatial geometries, the curvature gives a scale (either by using the radius of curvature or its inverse), a fact noted by Carl Friedrich Gauss in an 1824 letter to Franz Taurinus.^[8]

For a flat spatial geometry, the scale of any properties of the topology is arbitrary and may or may not be directly detectable.

The probability of detection of the topology by direct observation depends on the spatial curvature: a small curvature of the local geometry, with a corresponding radius of curvature greater than the observable horizon, makes the topology difficult or impossible to detect if the curvature is hyperbolic. A spherical geometry with a small curvature (large radius of curvature) does not make detection difficult.

Analysis of data from WMAP implies that on the scale to the surface of last scattering, the density parameter of the Universe is within about 0.5% of the value representing spatial flatness.^[9]

Curvature

The curvature of the Universe places constraints on the topology. If the spatial geometry is spherical, i.e. possess positive curvature, the topology is compact. For a flat (zero curvature) or a hyperbolic (negative curvature) spatial geometry, the topology can be either compact or infinite.^[10] Many textbooks erroneously state that a flat universe implies an infinite universe; however, the correct statement is that a flat universe that is also simply connected implies an infinite universe.^[10] For example, Euclidean space is flat, simply connected and infinite, but the torus is flat, multiply connected, finite and compact.

In general, local to global theorems in Riemannian geometry relate the local geometry to the global geometry. If the local geometry has constant curvature, the global geometry is very constrained, as described in Thurston geometries.

The latest research shows that even the most powerful future experiments (like SKA, Planck..) will not be able to distinguish between flat, open and closed universe if the true value of cosmological curvature parameter is smaller than 10⁻⁴. If the true value of the cosmological curvature parameter is larger than 10⁻³ we will be able to distinguish between these three models even now.^[11]

Results of the Planck mission released in 2015 show the cosmological curvature parameter, Ω_K, to be 0.000±0.005, coincident with a flat Universe.^[12]

Universe with zero curvature

In a universe with zero curvature, the local geometry is flat. The most obvious global structure is that of Euclidean space, which is infinite in extent. Flat universes that are finite in extent include the torus and Klein bottle. Moreover, in three dimensions, there are 10 finite closed flat 3-manifolds, of which 6 are orientable and 4 are non-orientable. The most familiar is the aforementioned 3-Torus universe.

In the absence of dark energy, a flat universe expands forever but at a continually decelerating rate, with expansion asymptotically approaching zero. With dark energy, the expansion rate of the Universe initially slows down, due to the effect of gravity, but eventually increases. The ultimate fate of the universe is the same as that of an open universe.

A flat universe can have zero total energy.

Universe with positive curvature

A positively curved universe is described by spherical geometry, and can be thought of as a three-dimensional hypersphere, or some other spherical 3-manifold (such as the Poincaré dodecahedral space), all of which are quotients of the 3-sphere.

Poincaré dodecahedral space, a positively curved space, colloquially described as "soccerball-shaped", as it is the quotient of the 3-sphere by the binary icosahedral group, which is very close to icosahedral symmetry, the symmetry of a soccer ball. This was proposed by Jean-Pierre Luminet and colleagues in 2003^[5][13] and an optimal orientation on the sky for the model was estimated in 2008.^[6]

Universe with negative curvature

A hyperbolic universe, one of a negative spatial curvature, is described by hyperbolic geometry, and can be thought of locally as a three-dimensional analog of an infinitely extended saddle shape. There are a great variety of hyperbolic 3-manifolds, and their classification is not completely understood. For hyperbolic local geometry, many of the possible three-dimensional spaces are informally called horn topologies, so called because of the shape of the pseudosphere, a canonical model of hyperbolic geometry. An example is the Picard horn, a negatively curved space, colloquially described as "funnel-shaped".^[7]

Curvature: Open or closed

When cosmologists speak of the Universe as being "open" or "closed", they most commonly are referring to whether the curvature is negative or positive. These meanings of open and closed are different from the mathematical meaning of open and closed used for sets in metric spaces and for the mathematical meaning of open and closed manifolds, which gives rise to ambiguity and confusion. In mathematics, there are definitions for a closed manifold (i.e. compact without boundary) and open manifold (i.e. one that is not compact and without boundary,^[14]). A "closed universe" is necessarily a closed manifold. An "open universe" can be either a closed or open manifold. For example, the Friedmann–Lemaître–Robertson–Walker (FLRW) model the Universe is considered to be without boundaries, in which case "compact universe" could describe a universe that is a closed manifold.

Milne model ("spherical" expanding)

Universe in an expanding sphere. The galaxies farthest away are moving fastest and hence experience length contraction and so become smaller to an observer in the centre.

If one applies Minkowski space-based Special Relativity to expansion of the Universe, without resorting to the concept of a curved spacetime, then one obtains the Milne model. Any spatial section of the Universe of a constant age (the proper time elapsed from the Big Bang) will have a negative curvature; this is merely a pseudo-Euclidean geometric fact analogous to one that concentric spheres in the flat Euclidean space are nevertheless curved. Spacial geometry of this model is an unbounded hyperbolic space. The entire universe is contained within a light cone, namely the future cone of the Big Bang. For any given moment t > 0 of coordinate time (assuming the Big Bang has t = 0), the entire universe is bounded by a sphere of radius exactly c t. The apparent paradox of an infinite universe contained within a sphere is explained with length contraction: the galaxies farther away, which are travelling away from the observer the fastest, will appear thinner.

This model is essentially a degenerate FLRW for Ω = 0. It is incompatible with observations that definitely rule out such a large negative spatial curvature. However, as a background in which gravitational fields (or gravitons) can operate, due to diffeomorphism invariance, the space on the macroscopic scale, is equivalent to any other (open) solution of Einstein's field equations.

rigins of Complex Life Uncovered in the Ocean Deep

• PBS.org
• Video
• Programs
• TV Schedules
• Shop
• Donate

Posted by R.A. Becker on Thu, 07 May 2015

Original link:  http://www.pbs.org/wgbh/nova/next/evolution/origins-of-complex-life-uncovered-in-the-ocean-deep/

Scientists have just discovered a missing link between two branches on the evolutionary tree of life hiding deep under the sea, one that we’ve been looking for since 1977.

Ever since microbiologist Carl Woese, the greatest scientist you’ve never heard of, added a third branch, archaea, to the existing two of prokaryotes (bacteria) and eukaryotes (which have more complex cells like our own), scientists have searched for the evolutionary connection between archaea and eukaryotes. While the reigning theory is that eukaryotes evolved from the simpler archaea, the ancestral parent of eukaryotes has remained elusive.

Scientists discovered the closest evolutionary relative to eukaryotic cells near a hydrothermal vent.

But just this week, a group of scientists announced that they found that connection buried under the Arctic Ocean near a hydrothermal vent called Loki’s Castle. They named it Lokiarchaeum, and published their findings in the journal Nature.

Here’s Carl Zimmer, reporting for the New York Times:

Analyzing the DNA, the researchers found that Lokiarchaeum is far more closely related to eukaryotes than any other known species of archaea. But even more surprising was that it had genes for many traits only found before in eukaryotes.Among these genes were many that build special compartments inside eukaryote cells. Inside these compartments, called lysosomes, eukaryote cells can destroy defective proteins.

All eukaryotes also share a cellular skeleton that they constantly build and tear down to change their shape. Dr. Ettema and his colleagues found many genes in Lokiarchaeum that encode the proteins required to build the skeleton.

The scientists are now trying to grow these cells in the lab to understand more about them, but it’s difficult to replicate the conditions of deep ocean hydrothermal vents. In the meantime they’re looking for more archaea that might be even closer relatives of our eukaryotic ancestors, and in turn telling us more about our own origins.

Eternal inflation

From Wikipedia, the free encyclopedia

The eternal nature of new inflation was discovered independently by Paul Steinhardt and Alexander Vilenkin in 1983.^[1] Eternal inflation is an inflationary universe model, which is itself an outgrowth or extension of the Big Bang theory. In theories of eternal inflation, the inflationary phase of the universe's expansion lasts forever in at least some regions of the universe. Because these regions expand exponentially rapidly, most of the volume of the universe at any given time is inflating. All models of eternal inflation produce an infinite multiverse, typically a fractal.

Eternal inflation is predicted by many different models of cosmic inflation. MIT professor Alan H. Guth proposed an inflation model involving a "false vacuum" phase with positive vacuum energy. Parts of the universe in that phase inflate, and only occasionally decay to lower-energy, non-inflating phases or the ground state. In chaotic inflation, proposed by physicist Andrei Linde, the peaks in the evolution of a scalar field (determining the energy of the vacuum) correspond to regions of rapid inflation which dominate. Chaotic inflation usually eternally inflates,^[2] since the expansions of the inflationary peaks exhibit positive feedback and come to dominate the large-scale dynamics of the universe.

Alan Guth's 2007 paper, "Eternal inflation and its implications",^[2] details what is now known on the subject, and demonstrates that this particular flavor of inflationary universe theory is relatively current, or is still considered viable, more than 20 years after its inception.^{[3] [4][5]}

Inflation and the multiverse

Both Linde and Guth believe that inflationary models of the early universe most likely lead to a multiverse but more proof is required.

It's hard to build models of inflation that don't lead to a multiverse. It's not impossible, so I think there's still certainly research that needs to be done. But most models of inflation do lead to a multiverse, and evidence for inflation will be pushing us in the direction of taking [the idea of a] multiverse seriously. Alan Guth^[6]

It's possible to invent models of inflation that do not allow [a] multiverse, but it's difficult. Every experiment that brings better credence to inflationary theory brings us much closer to hints that the multiverse is real. Andrei Linde ^[6]

Polarization in the cosmic microwave background radiation suggests inflationary models for the early universe are more likely but confirmation is needed.^[6]

History

Inflation, or the inflationary universe theory, was developed as a way to overcome the few remaining problems with what was otherwise considered a successful theory of cosmology, the Big Bang model. Although Alexei Starobinsky of the L.D. Landau Institute of Theoretical Physics in Moscow developed the first realistic inflation theory in 1979^[7][8] he did not articulate its relevance to modern cosmological problems.

In 1979, Alan Guth developed an inflationary model independently, which offered a mechanism for inflation to begin: the decay of a so-called false vacuum into "bubbles" of "true vacuum" that expanded at the speed of light. Guth coined the term "inflation", and he was the first to discuss the theory with other scientists worldwide. But this formulation was problematic, as there was no consistent way to bring an end to the inflationary epoch and end up with the isotropic, homogeneous universe observed today. (See False vacuum: Development of theories). In 1982, this "graceful exit problem" was solved by Andrei Linde in the new inflationary scenario. A few months later, the same result was also obtained by Andreas Albrecht and Paul J. Steinhardt.

In 1986, Linde published an alternative model of inflation that also reproduced the same successes of new inflation entitled "Eternally Existing Self-Reproducing Chaotic Inflationary Universe",^[9] which provides a detailed description of what has become known as the Chaotic Inflation theory or eternal inflation. The Chaotic Inflation theory is in some ways similar to Fred Hoyle’s steady state theory, as it employs the concept of a universe that is eternally existing, and thus does not require a unique beginning or an ultimate end of the cosmos.

Quantum fluctuations of the inflation field

Chaotic Inflation theory models quantum fluctuations in the rate of inflation.^[10] Those regions with a higher rate of inflation expand faster and dominate the universe, despite the natural tendency of inflation to end in other regions. This allows inflation to continue forever, to produce future-eternal inflation.

Within the framework of established knowledge of physics and cosmology, our universe could be one of many in a super-universe or multiverse. Linde (1990, 1994) has proposed that a background space-time "foam" empty of matter and radiation will experience local quantum fluctuations in curvature, forming many bubbles of false vacuum that individually inflate into mini-universes with random characteristics. Each universe within the multiverse can have a different set of constants and physical laws. Some might have life of a form different from ours; others might have no life at all or something even more complex or so different that we cannot even imagine it. Obviously we are in one of those universes with life.^[11]

—Victor J. Stenger

Past-eternal models have been proposed which adhere to the perfect cosmological principle and have features of the steady state cosmos.^[12][13][14]

A recent paper by Kohli and Haslam^{[when?] [15]} analyzed Linde's chaotic inflation theory in which the quantum fluctuations are modelled as Gaussian white noise. They showed that in this popular scenario, eternal inflation in fact cannot be eternal, and the random noise leads to spacetime being filled with singularities. This was demonstrated by showing that solutions to the Einstein field equations diverge in a finite time. Their paper therefore concluded that the theory of eternal inflation based on random quantum fluctuations would not be a viable theory, and the resulting existence of a multiverse is "still very much an open question that will require much deeper investigation".

Differential decay

In standard inflation, inflationary expansion occurred while the universe was in a false vacuum state, halting when the universe decayed to a true vacuum state and became a general and inclusive phenomenon with homogeneity throughout, yielding a single expanding universe which is "our general reality" wherein the laws of physics are consistent throughout. In this case, the physical laws "just happen" to be compatible with the evolution of life.

The bubble universe model proposes that different regions of this inflationary universe (termed a multiverse) decayed to a true vacuum state at different times, with decaying regions corresponding to "sub"- universes not in causal contact with each other and existing in discrete regions that are subject to truly random "selection", determining each region's components based upon the persistence of the quantum components within that region. The end result will be a finite number of universes with physical laws consistent within each region of spacetime.

False vacuum and true vacuum

Variants of the bubble universe model postulate multiple false vacuum states, which result in lower-energy false-vacuum "progeny" universes spawned, which in turn produce true vacuum state progeny universes within themselves.

Evidence from the fluctuation level in our universe

New inflation does not produce a perfectly symmetric universe; tiny quantum fluctuations in the inflaton are created. These tiny fluctuations form the primordial seeds for all structure created in the later universe. These fluctuations were first calculated by Viatcheslav Mukhanov and G. V. Chibisov in the Soviet Union in analyzing Starobinsky's similar model.^[16][17][18] In the context of inflation, they were worked out independently of the work of Mukhanov and Chibisov at the three-week 1982 Nuffield Workshop on the Very Early Universe at Cambridge University.^[19] The fluctuations were calculated by four groups working separately over the course of the workshop: Stephen Hawking;^[20] Starobinsky;^[21] Guth and So-Young Pi;^[22] and James M. Bardeen, Paul Steinhardt and Michael Turner.^[23]

The fact that these models are consistent with WMAP data adds weight to the idea that the universe could be created in such a way. As a result, many physicists in the field agree it is possible, but needs further support to be accepted.^[24]

Graviton

From Wikipedia, the free encyclopedia

Graviton

Composition	Elementary particle
Statistics	Bose–Einstein statistics
Interactions	Gravitation
Status	Theoretical
Symbol	G[1]
Antiparticle	Self
Theorized	1930s[2] The name is attributed to Dmitrii Blokhintsev and F. M. Gal'perin in 1934[3]
Discovered	Hypothetical
Mass	0
Mean lifetime	Stable
Electric charge	0 e
Spin	2

In physics, the graviton is a hypothetical elementary particle that mediates the force of gravitation in the framework of quantum field theory. If it exists, the graviton is expected to be massless (because the gravitational force appears to have unlimited range) and must be a spin-2 boson. The spin follows from the fact that the source of gravitation is the stress–energy tensor, a second-rank tensor (compared to electromagnetism's spin-1 photon, the source of which is the four-current, a first-rank tensor). Additionally, it can be shown that any massless spin-2 field would give rise to a force indistinguishable from gravitation, because a massless spin-2 field must couple to (interact with) the stress–energy tensor in the same way that the gravitational field does. Seeing as the graviton is hypothetical, its discovery would unite quantum theory with gravity.^[4] This result suggests that, if a massless spin-2 particle is discovered, it must be the graviton, so that the only experimental verification needed for the graviton may simply be the discovery of a massless spin-2 particle.^[5]

Theory

The four other known forces of nature are mediated by elementary particles: electromagnetism by the photon, the strong interaction by the gluons, the Higgs field by the Higgs Boson, and the weak interaction by the W and Z bosons. The hypothesis is that the gravitational interaction is likewise mediated by an – as yet undiscovered – elementary particle, dubbed as the graviton. In the classical limit, the theory would reduce to general relativity and conform to Newton's law of gravitation in the weak-field limit.^[6][7][8]

Gravitons and renormalization

When describing graviton interactions, the classical theory (i.e., the tree diagrams) and semiclassical corrections (one-loop diagrams) behave normally, but Feynman diagrams with two (or more) loops lead to ultraviolet divergences; that is, infinite results that cannot be removed because the quantized general relativity is not renormalizable, unlike quantum electrodynamics.^{[dubious – discuss]} That is, the usual ways physicists calculate the probability that a particle will emit or absorb a graviton give nonsensical answers and the theory loses its predictive power. These problems, together with some conceptual puzzles, led many physicists^[who?] to believe that a theory more complete than quantized general relativity must describe the behavior near the Planck scale.^{[citation needed]}

Comparison with other forces

Unlike the force carriers of the other forces, gravitation plays a special role in general relativity in defining the spacetime in which events take place. In some descriptions, matter modifies the 'shape' of spacetime itself, and gravity is a result of this shape, an idea which at first glance may appear hard to match with the idea of a force acting between particles.^[9] Because the diffeomorphism invariance of the theory does not allow any particular space-time background to be singled out as the "true" space-time background, general relativity is said to be background independent. In contrast, the Standard Model is not background independent, with Minkowski space enjoying a special status as the fixed background space-time.^[10] A theory of quantum gravity is needed in order to reconcile these differences.^[11] Whether this theory should be background independent is an open question. The answer to this question will determine our understanding of what specific role gravitation plays in the fate of the universe.^[12]

Gravitons in speculative theories

String theory predicts the existence of gravitons and their well-defined interactions. A graviton in perturbative string theory is a closed string in a very particular low-energy vibrational state. The scattering of gravitons in string theory can also be computed from the correlation functions in conformal field theory, as dictated by the AdS/CFT correspondence, or from matrix theory.^{[citation needed]}

An interesting feature of gravitons in string theory is that, as closed strings without endpoints, they would not be bound to branes and could move freely between them. If we live on a brane (as hypothesized by brane theories) this "leakage" of gravitons from the brane into higher-dimensional space could explain why gravitation is such a weak force, and gravitons from other branes adjacent to our own could provide a potential explanation for dark matter. See brane cosmology.^{[citation needed]}

A theory by Ali and Das adds quantum mechanical corrections (using Bohm trajectories) to general relativistic geodesics. If gravitons are given a small but non-zero mass, it could explain the cosmological constant without need for dark energy and solve the smallness problem.^[13]

Experimental observation

Unambiguous detection of individual gravitons, though not prohibited by any fundamental law, is impossible with any physically reasonable detector.^[14] The reason is the extremely low cross section for the interaction of gravitons with matter. For example, a detector with the mass of Jupiter and 100% efficiency, placed in close orbit around a neutron star, would only be expected to observe one graviton every 10 years, even under the most favorable conditions. It would be impossible to discriminate these events from the background of neutrinos, since the dimensions of the required neutrino shield would ensure collapse into a black hole.^[14]

However, experiments to detect gravitational waves, which may be viewed as coherent states of many gravitons, are underway (e.g., LIGO and VIRGO). Although these experiments cannot detect individual gravitons, they might provide information about certain properties of the graviton.^[15] For example, if gravitational waves were observed to propagate slower than c (the speed of light in a vacuum), that would imply that the graviton has mass (however, gravitational waves must propagate slower than "c" in a region with non-zero mass density if they are to be detectable).^[16] Astronomical observations of the kinematics of galaxies, especially the galaxy rotation problem and modified Newtonian dynamics, might point toward gravitons having non-zero mass.^[17]

Difficulties and outstanding issues

Most theories containing gravitons suffer from severe problems. Attempts to extend the Standard Model or other quantum field theories by adding gravitons run into serious theoretical difficulties at high energies (processes involving energies close to or above the Planck scale) because of infinities arising due to quantum effects (in technical terms, gravitation is nonrenormalizable). Since classical general relativity and quantum mechanics seem to be incompatible at such energies, from a theoretical point of view, this situation is not tenable. One possible solution is to replace particles with strings. String theories are quantum theories of gravity in the sense that they reduce to classical general relativity plus field theory at low energies, but are fully quantum mechanical, contain a graviton, and are believed to be mathematically consistent.^[18]

Seafloor Volcano Pulses May Alter Climate

An ocean-bottom seismometer (sailboat-like object) was trapped amid
erupting magma in 2006 at the East Pacific Rise. Such instruments
are providing new insights into the timing of eruptions.
(Dan Fornari/Woods Hole Oceanographic Institution)

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Original link:  http://www.ldeo.columbia.edu/news-events/seafloor-volcano-pulses-may-alter-climate
 

New Data Show Strikingly Regular Patterns, From Weeks to Eons

Vast ranges of volcanoes hidden under the oceans are presumed by scientists to be the gentle giants of the planet, oozing lava at slow, steady rates along mid-ocean ridges. But a new study shows that they flare up on strikingly regular cycles, ranging from two weeks to 100,000 years—and, that they erupt almost exclusively during the first six months of each year. The pulses—apparently tied to short- and long-term changes in earth’s orbit, and to sea levels--may help trigger natural climate swings. Scientists have already speculated that volcanic cycles on land emitting large amounts of carbon dioxide might influence climate; but up to now there was no evidence from submarine volcanoes. The findings suggest that models of earth’s natural climate dynamics, and by extension human-influenced climate change, may have to be adjusted. The study appears this week in the journal Geophysical Research Letters.

 

“People have ignored seafloor volcanoes on the idea that their influence is small—but that’s because they are assumed to be in a steady state, which they’re not,” said the study’s author, marine geophysicist Maya Tolstoy of Columbia University’s Lamont-Doherty Earth Observatory. “They respond to both very large forces, and to very small ones, and that tells us that we need to look at them much more closely.” A related study by a separate team this week in the journal Science bolsters Tolstoy’s case by showing similar long-term patterns of submarine volcanism in an Antarctic region Tolstoy did not study.

 

Volcanically active mid-ocean ridges crisscross earth’s seafloors like stitching on a baseball, stretching some 37,000 miles. They are the growing edges of giant tectonic plates; as lavas push out, they form new areas of seafloor, which comprise some 80 percent of the planet’s crust. Conventional wisdom holds that they erupt at a fairly constant rate--but Tolstoy finds that the ridges are actually now in a languid phase. Even at that, they produce maybe eight times more lava annually than land volcanoes. Due to the chemistry of their magmas, the carbon dioxide they are thought to emit is currently about the same as, or perhaps a little less than, from land volcanoes—about 88 million metric tons a year. But were the undersea chains to stir even a little bit more, their CO2 output would shoot up, says Tolstoy.

 

Magma from undersea eruptions congealed into forms known as pillow basalts
on the Juan De Fuca Ridge, off the U.S. Pacific Northwest. A new study
shows such eruptions wax and wane on regular schedules.
(Deborah Kelley/University of Washington)

Some scientists think volcanoes may act in concert with Milankovitch cycles--repeating changes in the shape of earth’s solar orbit, and the tilt and direction of its axis—to produce suddenly seesawing hot and cold periods. The major one is a 100,000-year cycle in which the planet’s orbit around the sun changes from more or less an annual circle into an ellipse that annually brings it closer or farther from the sun. Recent ice ages seem to build up through most of the cycle; but then things suddenly warm back up near the orbit’s peak eccentricity. The causes are not clear.

 

Enter volcanoes. Researchers have suggested that as icecaps build on land, pressure on underlying volcanoes also builds, and eruptions are suppressed. But when warming somehow starts and the ice begins melting, pressure lets up, and eruptions surge. They belch CO2 that produces more warming, which melts more ice, which creates a self-feeding effect that tips the planet suddenly into a warm period. A 2009 paper from Harvard University says that land volcanoes worldwide indeed surged six to eight times over background levels during the most recent deglaciation, 12,000 to 7,000 years ago. The corollary would be that undersea volcanoes do the opposite: as earth cools, sea levels may drop 100 meters, because so much water gets locked into ice. This relieves pressure on submarine volcanoes, and they erupt more. At some point, could the increased CO2 from undersea eruptions start the warming that melts the ice covering volcanoes on land?  

 

That has been a mystery, partly because undersea eruptions are almost impossible to observe. However, Tolstoy and other researchers recently have been able to closely monitor 10 submarine eruption sites using sensitive new seismic instruments. They have also produced new high-resolution maps showing outlines of past lava flows. Tolstoy analyzed some 25 years of seismic data from ridges in the Pacific, Atlantic and Arctic oceans, plus maps showing past activity in the south Pacific.

	Alternating ridges and valleys formed by volcanism near the East Pacific Rise, a mid-ocean ridge in the Pacific Ocean. Such formations indicate ancient highs and lows of volcanic activity. (Haymon et al., NOAA-OE, WHOI)

The long-term eruption data, spread over more than 700,000 years, showed that during the coldest times, when sea levels are low, undersea volcanism surges, producing visible bands of hills. When things warm up and sea levels rise to levels similar to the present, lava erupts more slowly, creating bands of lower topography. Tolstoy attributes this not only to the varying sea level, but to closely related changes in earth’s orbit. When the orbit is more elliptical, Earth gets squeezed and unsqueezed by the sun’s gravitational pull at a rapidly varying rate as it spins daily—a process that she thinks tends to massage undersea magma upward, and help open the tectonic cracks that let it out. When the orbit is fairly (though not completely) circular, as it is now, the squeezing/unsqueezing effect is minimized, and there are fewer eruptions.

The idea that remote gravitational forces influence volcanism is mirrored by the short-term data, says Tolstoy. She says the seismic data suggest that today, undersea volcanoes pulse to life mainly during periods that come every two weeks. That is the schedule upon which combined gravity from the moon and sun cause ocean tides to reach their lowest points, thus subtly relieving pressure on volcanoes below. Seismic signals interpreted as eruptions followed fortnightly low tides at eight out of nine study sites. Furthermore, Tolstoy found that all known modern eruptions occur from January through June. January is the month when Earth is closest to the sun, July when it is farthest—a period similar to the squeezing/unsqueezing effect Tolstoy sees in longer-term cycles. “If you look at the present-day eruptions, volcanoes respond even to much smaller forces than the ones that might drive climate,” she said.

 

Daniel Fornari, a senior scientist at Woods Hole Oceanographic Institution not involved in the research, called the study “a very important contribution.”  He said it was unclear whether the contemporary seismic measurements signal actual lava flows or just seafloor rumbles and cracking. But, he said, the study “clearly could have important implications for better quantifying and characterizing our assessment of climate variations over decadal to tens to hundreds of thousands of years cycles.”

 

Edward Baker, a senior ocean scientist at the National Oceanic and Atmospheric Administration, said, “The most interesting takeaway from this paper is that it provides further evidence that the solid Earth, and the air and water all operate as a single system.”

 

The research for this paper was funded in large part by the U.S. National Science Foundation.