The Written History of a Neuron

Brain cells record their activity in gene expression, new study finds.

Image: jxfzsy/iStock

By STEPHANIE DUTCHEN

April 26, 2018

Original link:  https://hms.harvard.edu/news/written-history-neuron?utm_source=facebook&utm_medium=social&utm_campaign=hms-facebook-general

From burning your palm on a hot pan handle to memorizing the name of a new acquaintance, “anytime you experience something, your neurons are active,” says Kelsey Tyssowski, a graduate student in genetics at Harvard Medical School.

Different experiences stimulate different patterns of activity in brain cells. Researchers want to track these activity patterns to better understand how the brain makes sense of the world, but they’ve been limited by the transient nature of the activity and by the tiny fraction of neurons they’re able to study at once—only a few thousand out of an estimated 100 billion.

A new study by Tyssowski, HMS graduate student Nicholas DeStefino and colleagues promises to change that.

Studying cells in a dish and lab mice, the researchers reported April 19 in Neuron that bits of a neuron’s activity record can be reconstructed by analyzing its gene expression pattern—the particular genes that are active in the cell.
Specifically, the researchers found that expression patterns reflect how long a neuron fired in response to a stimulus.

“The longer a neuron’s activity persists, the more genes are turned on by it,” explained Jesse Gray, assistant professor of genetics at HMS and co-senior author of the paper, along with Ramendra Saha of the University of California, Merced, and Serena Dudek of the National Institute of Environmental Health Sciences.

Because gene expression is easier to measure across many neurons than neuronal activity, linking the two should now allow researchers to “analyze the activity patterns of tens of thousands of neurons in a single experiment,” said Gray.

That, in turn, promises to enable more comprehensive research into how the brain works, particularly how it forms memories.

An illuminating experiment

Tyssowski made her first discoveries in the Gray lab using neurons in a dish.

As she investigated how neuronal activity leads to gene activation, she found that exposing the cells to a brief stimulus turned on genes that responded quickly, while a sustained stimulus turned on both fast-response and slow-response genes.

“There’s an elegance to what Kelsey found, an unexpected simplicity to nature,” said Gray. “The faster a gene is able to be turned on, the more likely it is to be turned on by brief activity. That makes intuitive sense, but we hadn’t known that that’s how it works.”

The results were intriguing, but a critical question loomed, said Tyssowski: “Does this happen in an actual brain?”

Together with Jin Hyung Cho, a research fellow in genetics in the Gray lab, Tyssowski teamed up with Mark Andermann, HMS associate professor of medicine, and research technician Crista Carty, both at Beth Israel Deaconess Medical Center, to confirm the findings in mice.

The scientists used an established experimental method in which mice are housed in the dark for a while to clear out any residual gene expression related to light exposure; then they turned on lights near the cages for either a few minutes or a longer time.

The team recorded the activity of light-sensing neurons in the mice’s visual cortex, a brain region that handles vision. To the researchers’ delight, Tyssowski’s initial findings remained true: Brief light exposure turned on fast-response genes, and longer exposure turned on both fast- and slow-response genes.

Given this consistent outcome, the researchers wondered: Would it be possible to estimate the duration of an earlier exposure simply by looking at a neuron’s gene expression?

The answer, it seemed, was yes. Tyssowski successfully trained a computer to look at gene expression patterns in neurons from the mouse experiment and guess whether they had undergone brief or sustained light exposure.

Two-thirds of the puzzle still remain. Now that they’ve figured out the relationship between the duration of neuronal activity and changes in gene expression, researchers can explore the dynamics of two other major types of neuronal activity variation: frequency of firing and “burstiness,” periods of rapid firing with long gaps in between.

Thinking, fast and slow

The group’s findings could improve understanding of the connection between what Gray calls the “fast computers” and “slow computers” inside neurons that convert sensory experiences into thoughts and actions.

“The fast computer, which performs electrical and chemical computations in milliseconds, acts in the moment to elicit rapid actions that determine whether we get eaten or not,” said Gray.

The slower computer uses the genome to perform computations over hours or days.

“It helps store memories that make it easier to avoid getting eaten the next time we encounter the same predator,” said Gray.

Decoding the relationship between these computers could help solve the puzzle of how the slow computer completes two of its major tasks: storing information following new experiences and reining in neuronal overactivity.

“One of my hopes is that our study sparks more interest in the connection between fast and slow, which we call the coupling map,” he said. “We hope our fellow neuroscientists will think about this map more, because we think it’s important for understanding how the brain operates.”

Artist’s rendition of how the “slow computer” uses geneexpression to process electrochemical information fromthe “fast computer.” Illustrations: Anastasia Nizhnik andKelsey Tyssowski





This research was supported by the NIH (grants R01 MH101528-01, R01 MH116223-01, R01 DK109930, Z01 ES100221, R00 MH096941 and New Innovator Award DP2 DK105570), the Canadian Institute of Health Research, the Giovanni Armenise-Harvard Foundation, the National Science Foundation Graduate Research Fellowship Program, a McKnight Scholar Award, a Harvard Brain Science Initiative Bipolar Disorder Seed Grant, the Kaneb family and Kent and Liz Dauten.

Chronobiology

From Wikipedia, the free encyclopedia

 

Overview, including some physiological parameters, of the human circadian rhythm ("biological clock").

Chronobiology is a field of biology that examines periodic (cyclic) phenomena in living organisms and their adaptation to solar- and lunar-related rhythms.^[1] These cycles are known as biological rhythms. Chronobiology comes from the ancient Greek χρόνος (chrónos, meaning "time"), and biology, which pertains to the study, or science, of life. The related terms chronomics and chronome have been used in some cases to describe either the molecular mechanisms involved in chronobiological phenomena or the more quantitative aspects of chronobiology, particularly where comparison of cycles between organisms is required.

Chronobiological studies include but are not limited to comparative anatomy, physiology, genetics, molecular biology and behavior of organisms within biological rhythms mechanics.^[1] Other aspects include epigenetics, development, reproduction, ecology and evolution.

Description

The variations of the timing and duration of biological activity in living organisms occur for many essential biological processes. These occur (a) in animals (eating, sleeping, mating, hibernating, migration, cellular regeneration, etc.), (b) in plants (leaf movements, photosynthetic reactions, etc.), and in microbial organisms such as fungi and protozoa. They have even been found in bacteria, especially among the cyanobacteria (aka blue-green algae, see bacterial circadian rhythms). The most important rhythm in chronobiology is the circadian rhythm, a roughly 24-hour cycle shown by physiological processes in all these organisms. The term circadian comes from the Latin circa, meaning "around" and dies, "day", meaning "approximately a day." It is regulated by circadian clocks.

The circadian rhythm can further be broken down into routine cycles during the 24-hour day:^[2]

• Diurnal, which describes organisms active during daytime
• Nocturnal, which describes organisms active in the night
• Crepuscular, which describes animals primarily active during the dawn and dusk hours (ex: white-tailed deer, some bats)

While circadian rhythms are defined as endogenously regulated, other biological cycles may be regulated by exogenous signals. In some cases, multi-trophic systems may exhibit rhythms driven by the circadian clock of one of the members (which may also be influenced or reset by external factors). The endogenous plant cycles may regulate the activity of the bacterium by controlling availability of plant-produced photosynthate.

Many other important cycles are also studied, including:

• Infradian rhythms, which are cycles longer than a day. Examples include circannual or annual cycles that govern migration or reproduction cycles in many plants and animals, or the human menstrual cycle.
• Ultradian rhythms, which are cycles shorter than 24 hours, such as the 90-minute REM cycle, the 4-hour nasal cycle, or the 3-hour cycle of growth hormone production.
• Tidal rhythms, commonly observed in marine life, which follow the roughly 12.4-hour transition from high to low tide and back.
• Lunar rhythms, which follow the lunar month (29.5 days). They are relevant e.g. for marine life, as the level of the tides is modulated across the lunar cycle.
• Gene oscillations – some genes are expressed more during certain hours of the day than during other hours.

Within each cycle, the time period during which the process is more active is called the acrophase.^[3] When the process is less active, the cycle is in its bathyphase or trough phase. The particular moment of highest activity is the peak or maximum; the lowest point is the nadir. How high (or low) the process gets is measured by the amplitude.

History

A circadian cycle was first observed in the 18th century in the movement of plant leaves by the French scientist Jean-Jacques d'Ortous de Mairan.^[4] In 1751 Swedish botanist and naturalist Carl Linnaeus (Carl von Linné) designed a flower clock using certain species of flowering plants. By arranging the selected species in a circular pattern, he designed a clock that indicated the time of day by the flowers that were open at each given hour. For example, among members of the daisy family, he used the hawk's beard plant which opened its flowers at 6:30 am and the hawkbit which did not open its flowers until 7 am.^[5]

The 1960 symposium at Cold Spring Harbor Laboratory laid the groundwork for the field of chronobiology.^[6]

It was also in 1960 that Patricia DeCoursey invented the phase response curve, one of the major tools used in the field since.

Franz Halberg of the University of Minnesota, who coined the word circadian, is widely considered the "father of American chronobiology." However, it was Colin Pittendrigh and not Halberg who was elected to lead the Society for Research in Biological Rhythms in the 1970s. Halberg wanted more emphasis on the human and medical issues while Pittendrigh had his background more in evolution and ecology. With Pittendrigh as leader, the Society members did basic research on all types of organisms, plants as well as animals. More recently it has been difficult to get funding for such research on any other organisms than mice, rats, humans^[7][8] and fruit flies.

Recent developments

More recently, light therapy and melatonin administration have been explored by Alfred J. Lewy (OHSU), Josephine Arendt (University of Surrey, UK) and other researchers as a means to reset animal and human circadian rhythms. Additionally, the presence of low-level light at night accelerates circadian re-entrainment of hamsters of all ages by 50%; this is thought to be related to simulation of moonlight.^[9]

Humans can have a propensity to be morning people or evening people; these behavioral preferences are called chronotypes for which there are various assessment questionnaires and biological marker correlations.^[10]

In the second half of 20th century, substantial contributions and formalizations have been made by Europeans such as Jürgen Aschoff and Colin Pittendrigh, who pursued different but complementary views on the phenomenon of entrainment of the circadian system by light (parametric, continuous, tonic, gradual vs. nonparametric, discrete, phasic, instantaneous, respectively^[11]).

There is also a food-entrainable biological clock, which is not confined to the suprachiasmatic nucleus. The location of this clock has been disputed. Working with mice, however, Fuller et al. concluded that the food-entrainable clock seems to be located in the dorsomedial hypothalamus. During restricted feeding, it takes over control of such functions as activity timing, increasing the chances of the animal successfully locating food resources.^[12]

Other fields

Chronobiology is an interdisciplinary field of investigation. It interacts with medical and other research fields such as sleep medicine, endocrinology, geriatrics, sports medicine, space medicine and photoperiodism.^[13][14][15]

In spite of the similarity of the name to legitimate biological rhythms, the theory and practice of biorhythms is a classic example of pseudoscience. It attempts to describe a set of cyclic variations in human behavior based on a person's birth date. It is not a part of chronobiology.^[16]

Pineal gland

From Wikipedia, the free encyclopedia

 

Pineal gland
Diagram of pituitary and pineal glands in the human brain
Details
Precursor	Neural ectoderm, roof of diencephalon
Artery	posterior cerebral artery
Identifiers
Latin	glandula pinealis
MeSH	D010870
NeuroNames	297
NeuroLex ID	birnlex_1184
TA	A11.2.00.001
FMA	62033

Pineal gland or epiphysis (in red in back of the brain). Expand the image to an animated version

The pineal gland, also known as the conarium or epiphysis cerebri, is a small endocrine gland in the vertebrate brain. The pineal gland produces melatonin, a serotonin-derived hormone which modulates sleep patterns in both circadian and seasonal cycles. The shape of the gland resembles a pine cone, hence its name. The pineal gland is located in the epithalamus, near the center of the brain, between the two hemispheres, tucked in a groove where the two halves of the thalamus join.^[1][2]

Nearly all vertebrate species possess a pineal gland. The most important exception is a primitive vertebrate, the hagfish. Even in the hagfish, however, there may be a "pineal equivalent" structure in the dorsal diencephalon.^[3] The lancelet Branchiostoma lanceolatum, the nearest existing relative to vertebrates, also lacks a recognizable pineal gland.^[4] The lamprey (another primitive vertebrate), however, does possess one.^[4] A few more developed vertebrates lost pineal glands over the course of their evolution.^[5]

The results of various scientific research in evolutionary biology, comparative neuroanatomy and neurophysiology, have explained the phylogeny of the pineal gland in different vertebrate species. From the point of view of biological evolution, the pineal gland represents a kind of atrophied photoreceptor. In the epithalamus of some species of amphibians and reptiles, it is linked to a light-sensing organ, known as the parietal eye, which is also called the pineal eye or third eye.^[6]

René Descartes believed the pineal gland to be the "principal seat of the soul". Academic philosophy among his contemporaries considered the pineal gland as a neuroanatomical structure without special metaphysical qualities; science studied it as one endocrine gland among many. However, the pineal gland continues to have an exalted status in the realm of pseudoscience.^[7]

Structure

The pineal gland is a midline brain structure that is unpaired. It takes its name from its pine-cone shape.^[8] The gland is reddish-gray and about the size of a grain of rice (5–8 mm) in humans. The pineal gland, also called the pineal body, is part of the epithalamus, and lies between the laterally positioned thalamic bodies and behind the habenular commissure. It is located in the quadrigeminal cistern near to the corpora quadrigemina.^[9] It is also located behind the third ventricle and is bathed in cerebrospinal fluid supplied through a small pineal recess of the third ventricle which projects into the stalk of the gland.^[10]

Blood supply

Unlike most of the mammalian brain, the pineal gland is not isolated from the body by the blood–brain barrier system;^[11] it has profuse blood flow, second only to the kidney,^[12] supplied from the choroidal branches of the posterior cerebral artery.

Nerve supply

The pineal gland receives a sympathetic innervation from the superior cervical ganglion. A parasympathetic innervation from the pterygopalatine and otic ganglia is also present.^[13] Further, some nerve fibers penetrate into the pineal gland via the pineal stalk (central innervation). Also, neurons in the trigeminal ganglion innervate the gland with nerve fibers containing the neuropeptide PACAP.

Microanatomy

Pineal gland parenchyma with calcifications.

Micrograph of a normal pineal gland – very high magnification.

Micrograph of a normal pineal gland – intermediate magnification.

The pineal body consists in humans of a lobular parenchyma of pinealocytes surrounded by connective tissue spaces. The gland's surface is covered by a pial capsule.

The pineal gland consists mainly of pinealocytes, but four other cell types have been identified. As it is quite cellular (in relation to the cortex and white matter), it may be mistaken for a neoplasm.^[14]

Cell type	Description
Pinealocytes	The pinealocytes consist of a cell body with 4–6 processes emerging. They produce and secrete melatonin. The pinealocytes can be stained by special silver impregnation methods. Their cytoplasm is lightly basophilic. With special stains, pinealocytes exhibit lengthy, branched cytoplasmic processes that extend to the connective septa and its blood vessels.
Interstitial cells	Interstitial cells are located between the pinealocytes. They have elongated nuclei and a cytoplasm that is stained darker than that of the pinealocytes.
Perivascular phagocyte	Many capillaries are present in the gland, and perivascular phagocytes are located close to these blood vessels. The perivascular phagocytes are antigen presenting cells.
Pineal neurons	In higher vertebrates neurons are usually located in the pineal gland. However, this is not the case in rodents.
Peptidergic neuron-like cells	In some species, neuronal-like peptidergic cells are present. These cells might have a paracrine regulatory function.

In some parts of the brain and in particular the pineal gland, there are calcium structures, the number of which increases with age, called corpora arenacea (or "acervuli," or "brain sand"). Chemical analysis shows that they are composed of calcium phosphate, calcium carbonate, magnesium phosphate, and ammonium phosphate.^[15] In 2002, deposits of the calcite form of calcium carbonate were described.^[16] Calcium and phosphorus^[17] deposits in the pineal gland have been linked with aging.

Development

The human pineal gland grows in size until about 1–2 years of age, remaining stable thereafter,^[18][19] although its weight increases gradually from puberty onwards.^[20][21] The abundant melatonin levels in children are believed to inhibit sexual development, and pineal tumors have been linked with precocious puberty. When puberty arrives, melatonin production is reduced.^{[citation needed]}

Symmetry

In the zebrafish the pineal gland does not straddle the midline but shows a left-sided bias. In humans, functional cerebral dominance is accompanied by subtle anatomical asymmetry.^[22][23][24]

Function

The primary function of the pineal gland is to produce melatonin. Melatonin has various functions in the central nervous system, the most important of which is to help modulate sleep patterns. Melatonin production is stimulated by darkness and inhibited by light.^[25][26] Light sensitive nerve cells in the retina detect light and send this signal to the suprachiasmatic nucleus (SCN), synchronizing the SCN to the day-night cycle. Nerve fibers then relay the daylight information from the SCN to the paraventricular nuclei (PVN), then to the spinal cord and via the sympathetic system to superior cervical ganglia (SCG), and from there into the pineal gland.

The compound pinoline is also claimed to be produced in the pineal gland; it is one of the beta-carbolines.^[27] This claim is subject to some controversy.

Regulation of the pituitary gland

Studies on rodents suggest that the pineal gland influences the pituitary gland's secretion of the sex hormones, follicle-stimulating hormone (FSH), and luteinizing hormone (LH). Pinealectomy performed on rodents produced no change in pituitary weight, but caused an increase in the concentration of FSH and LH within the gland.^[28] Administration of melatonin did not return the concentrations of FSH to normal levels, suggesting that the pineal gland influences pituitary gland secretion of FSH and LH through an undescribed transmitting molecule.^[28]

The pineal gland contains receptors for the regulatory neuropeptide, endothelin-1,^[29] which, when injected in picomolar quantities into the lateral cerebral ventricle, causes a calcium-mediated increase in pineal glucose metabolism.^[30]

Drug metabolism

Studies on rodents suggest that the pineal gland may influence the actions of recreational drugs, such as cocaine,^[31] and antidepressants, such as fluoxetine (Prozac),^[32] and that its hormone melatonin can protect against neurodegeneration.^[33]

Regulation of bone metabolism

Studies in mice suggest that the pineal-derived melatonin regulates new bone deposition. Pineal-derived melatonin mediates its action on the bone cells through MT2 receptors. This pathway could be a potential new target for osteoporosis treatment as the study shows the curative effect of oral melatonin treatment in a postmenopausal osteoporosis mouse model.^[34]

Clinical significance

Calcification

Calcification of the pineal gland is typical in young adults, and has been observed in children as young as two years of age.^[35] Calcium and phosphorus deposits in the pineal gland have been correlated with aging.^[17] By old age, the pineal gland contains about the same amount of fluoride as teeth.^[36] Pineal fluoride and pineal calcium are correlated.^[36]

The calcified gland is often seen in skull X-Rays.^[35] Calcification rates vary widely by country and correlate with an increase in age, with calcification occurring in an estimated 40% of Americans by age seventeen.^[35] Calcification of the pineal gland is largely associated with corpora arenacea, also known as "brain sand".

It seems that the internal secretions of the pineal gland inhibit the development of the reproductive glands, because, in cases where it is severely damaged in children, the result is accelerated development of the sexual organs and the skeleton.^[37]

Some studies show that the degree of pineal gland calcification is significantly higher in patients with Alzheimer's disease vs. other types of dementia.^[38] Pineal gland calcification may contribute to the pathogenesis of Alzheimer's disease and may reflect an absence of crystallization inhibitors.^[38] Calcification of the pineal gland has also been found to be closely associated to certain types of migraines as well as cluster headaches.^[39][36]

Tumours

Tumours of the pineal gland are called pinealomas. These tumours are rare and 50% to 70% are germinomas that arise from sequestered embryonic germ cells. Histologically they are similar to testicular seminomas and ovarian dysgerminomas.^[40]

A pineal tumour can compress the superior colliculi and pretectal area of the dorsal midbrain, producing Parinaud's syndrome. Pineal tumours also can cause compression of the cerebral aqueduct, resulting in a noncommunicating hydrocephalus. Other manifestations are the consequence of their pressure effects and consist of visual disturbances, headache, mental deterioration, and sometimes dementia-like behaviour.^[41]

These neoplasms are divided into three categories, pineoblastomas, pineocytomas, and mixed tumours, based on their level of differentiation, which, in turn, correlates with their neoplastic aggressiveness.^[42] The clinical course of patients with pineocytomas is prolonged, averaging up to several years.^[43] The position of these tumours makes them very difficult to remove surgically.

Other animals

Most living vertebrates have pineal glands. It's likely that the common ancestor of all vertebrates had a pair of photosensory organs on the top of its head, similar to the arrangement in modern lampreys.^[44] Some extinct Devonian fishes have two parietal foramina in their skulls,^[45][46] suggesting an ancestral bilaterality of parietal eyes. The parietal eye and the pineal gland of living tetrapods are probably the descendants of the left and right parts of this organ, respectively.^[47]

During embryonic development, the parietal eye and the pineal organ of modern lizards^[48] and tuataras^[49] form together from a pocket formed in the brain ectoderm. The loss of parietal eyes in many living tetrapods is supported by developmental formation of a paired structure that subsequently fuses into a single pineal gland in developing embryos of turtles, snakes, birds, and mammals.^[50]

The pineal organs of mammals fall into one of three categories based on shape. Rodents have more structurally-complex pineal glands than other mammals.^[51]

Crocodilians and some tropical lineages of mammals (some xenarthrans [sloths], pangolins, sirenians [manatees & dugongs], and some marsupials [sugar gliders]) have lost both their parietal eye and their pineal organ.^[52][53][51] Polar mammals, such as walruses and some seals, possess unusually large pineal glands.^[52]

All amphibians have a pineal organ, but some frogs and toads also have what is called a "frontal organ", which is essentially a parietal eye.^[54]

Pinealocytes in many non-mammalian vertebrates have a strong resemblance to the photoreceptor cells of the eye. Evidence from morphology and developmental biology suggests that pineal cells possess a common evolutionary ancestor with retinal cells.^[55]

Pineal cytostructure seems to have evolutionary similarities to the retinal cells of the lateral eyes.^[55] Modern birds and reptiles express the phototransducing pigment melanopsin in the pineal gland. Avian pineal glands are thought to act like the suprachiasmatic nucleus in mammals.^[56] The structure of the pineal eye in modern lizards and tuatara is analogous to the cornea, lens, and retina of the lateral eyes of vertebrates.^[50]

In most vertebrates, exposure to light sets off a chain reaction of enzymatic events within the pineal gland that regulates circadian rhythms.^[57] In humans and other mammals, the light signals necessary to set circadian rhythms are sent from the eye through the retinohypothalamic system to the suprachiasmatic nuclei (SCN) and the pineal gland.

The fossilized skulls of many extinct vertebrates have a pineal foramen (opening), which in some cases is larger than that of any living vertebrate.^[58] Although fossils seldom preserve deep-brain soft anatomy, the brain of the Russian fossil bird Cerebavis cenomanica from Melovatka, about 90 million years old, shows a relatively large parietal eye and pineal gland.^[59]

Society and culture

Diagram of the operation of the pineal gland for Descartes in the Treatise of Man (figure published in the edition of 1664)

Seventeenth-century philosopher and scientist René Descartes was highly interested in anatomy and physiology. He discussed the pineal gland both in his first book, the Treatise of Man (written before 1637, but only published posthumously 1662/1664), and in his last book, The Passions of the Soul (1649) and he regarded it as "the principal seat of the soul and the place in which all our thoughts are formed."^[7] In the Treatise of Man, Descartes described conceptual models of man, namely creatures created by God, which consist of two ingredients, a body and a soul.^[7][60] In the Passions, Descartes split man up into a body and a soul and emphasized that the soul is joined to the whole body by "a certain very small gland situated in the middle of the brain's substance and suspended above the passage through which the spirits in the brain's anterior cavities communicate with those in its posterior cavities". Descartes attached significance to the gland because he believed it to be the only section of the brain to exist as a single part rather than one-half of a pair. Most of Descartes's basic anatomical and physiological assumptions were totally mistaken, not only by modern standards, but also in light of what was already known in his time.^[7][61]

The notion of a "pineal-eye" is central to the philosophy of the French writer Georges Bataille, which is analyzed at length by literary scholar Denis Hollier in his study Against Architecture. In this work Hollier discusses how Bataille uses the concept of a "pineal-eye" as a reference to a blind-spot in Western rationality, and an organ of excess and delirium.^[62] This conceptual device is explicit in his surrealist texts, The Jesuve and The Pineal Eye.^[63]

In the late 19th century Madame Blavatsky (who founded theosophy) identified the pineal gland with the Hindu concept of the third eye, or the Ajna chakra. This association is still popular today.^[7]

Rick Strassman, an author and Clinical Associate Professor of Psychiatry at the University of New Mexico School of Medicine, has theorised that the human pineal gland is capable of producing the hallucinogen N,N-dimethyltryptamine (DMT) under certain circumstances.^[64] In 2013 he and other researchers first reported DMT in the pineal gland microdialysate of rodents.^[65]

In the short story "From Beyond" by H. P. Lovecraft, a scientist creates an electronic device that emits a resonance wave, which stimulates an affected person's pineal gland, thereby allowing her or him to perceive planes of existence outside the scope of accepted reality, a translucent, alien environment that overlaps our own recognized reality. It was adapted as a film of the same name in 1986. The 2013 horror film, Banshee Chapter is heavily influenced by this short story.

History

The secretory activity of the pineal gland is only partially understood. Its location deep in the brain suggested to philosophers throughout history that it possesses particular importance. This combination led to its being regarded as a "mystery" gland with mystical, metaphysical, and occult theories surrounding its perceived functions.

The pineal gland was originally believed to be a "vestigial remnant" of a larger organ. In 1917, it was known that extract of cow pineals lightened frog skin. Dermatology professor Aaron B. Lerner and colleagues at Yale University, hoping that a substance from the pineal might be useful in treating skin diseases, isolated and named the hormone melatonin in 1958.^[66] The substance did not prove to be helpful as intended, but its discovery helped solve several mysteries such as why removing the rat's pineal accelerated ovary growth, why keeping rats in constant light decreased the weight of their pineals, and why pinealectomy and constant light affect ovary growth to an equal extent; this knowledge gave a boost to the then new field of chronobiology.^[67]

Square root of 2

From Wikipedia, the free encyclopedia

Binary	1.01101010000010011110…
Decimal	1.4142135623730950488…
Hexadecimal	1.6A09E667F3BCC908B2F…
Continued fraction	${\displaystyle 1+{\cfrac {1}{2+{\cfrac {1}{2+{\cfrac {1}{2+{\cfrac {1}{2+\ddots }}}}}}}}}$

The square root of 2 is equal to the length of the hypotenuse of a right triangle with legs of length 1

The square root of 2, or the (1/2)th power of 2, written in mathematics as √2 or 2^​1⁄₂, is the positive algebraic number that, when multiplied by itself, gives the number 2. Technically, it is called the principal square root of 2, to distinguish it from the negative number with the same property.

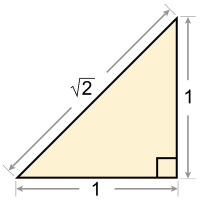
Geometrically the square root of 2 is the length of a diagonal across a square with sides of one unit of length; this follows from the Pythagorean theorem. It was probably the first number known to be irrational.

The rational approximation of the square root of two, 665,857/470,832, derived from the fourth step in the Babylonian algorithm starting with a₀ = 1, is too large by approx. 1.6×10⁻¹²: its square is 2.0000000000045…

The rational approximation 99/70 (≈ 1.4142857) is frequently used. Despite having a denominator of only 70, it differs from the correct value by less than 1/10,000 (approx. +0.72×10⁻⁴). Since it is a convergent of the continued fraction representation of the square root of two, any better rational approximation has a denominator not less than 169, since 239/169 (≈ 1.4142012) is the next convergent with an error of approx. −0.12×10⁻⁴.

The numerical value for the square root of two, truncated to 65 decimal places, is:

1.41421356237309504880168872420969807856967187537694807317667973799... (sequence A002193 in the OEIS).

History

Babylonian clay tablet YBC 7289 with annotations. Besides showing the square root of 2 in sexagesimal (1 24 51 10), the tablet also gives an example where one side of the square is 30 and the diagonal then is 42 25 35. The sexagesimal digit 30 can also stand for 0 30 = 1/2, in which case 0 42 25 35 is approximately 0.7071065.

The Babylonian clay tablet YBC 7289 (c. 1800–1600 BC) gives an approximation of √2 in four sexagesimal figures, 1 24 51 10, which is accurate to about six decimal digits,^[1] and is the closest possible three-place sexagesimal representation of √2:

${\displaystyle 1+{\frac {24}{60}}+{\frac {51}{60^{2}}}+{\frac {10}{60^{3}}}={\frac {30547}{21600}}=1.41421{\overline {296}}.}$

Another early close approximation is given in ancient Indian mathematical texts, the Sulbasutras (c. 800–200 BC) as follows: Increase the length [of the side] by its third and this third by its own fourth less the thirty-fourth part of that fourth.^[2] That is,

${\displaystyle 1+{\frac {1}{3}}+{\frac {1}{3\times 4}}-{\frac {1}{3\times 4\times 34}}={\frac {577}{408}}=1.41421{\overline {56862745098039}}.}$

This approximation is the seventh in a sequence of increasingly accurate approximations based on the sequence of Pell numbers, which can be derived from the continued fraction expansion of √2. Despite having a smaller denominator, it is only slightly less accurate than the Babylonian approximation.

Pythagoreans discovered that the diagonal of a square is incommensurable with its side, or in modern language, that the square root of two is irrational. Little is known with certainty about the time or circumstances of this discovery, but the name of Hippasus of Metapontum is often mentioned. For a while, the Pythagoreans treated as an official secret the discovery that the square root of two is irrational, and, according to legend, Hippasus was murdered for divulging it.^[3][4][5] The square root of two is occasionally called "Pythagoras' number" or "Pythagoras' constant", for example by Conway & Guy (1996).^[6]

Computation algorithms

There are a number of algorithms for approximating √2, which in expressions as a ratio of integers or as a decimal can only be approximated. The most common algorithm for this, one used as a basis in many computers and calculators, is the Babylonian method^[7] of computing square roots, which is one of many methods of computing square roots. It goes as follows:
First, pick a guess, a₀ > 0; the value of the guess affects only how many iterations are required to reach an approximation of a certain accuracy. Then, using that guess, iterate through the following recursive computation:

${\displaystyle a_{n+1}={\frac {a_{n}+{\frac {2}{a_{n}}}}{2}}={\frac {a_{n}}{2}}+{\frac {1}{a_{n}}}.}$

The more iterations through the algorithm (that is, the more computations performed and the greater "n"), the better approximation of the square root of 2 is achieved. Each iteration approximately doubles the number of correct digits. Starting with a₀ = 1 the next approximations are

• 3/2 = 1.5
• 17/12 = 1.416...
• 577/408 = 1.414215...
• 665857/470832 = 1.4142135623746...

The value of √2 was calculated to 137,438,953,444 decimal places by Yasumasa Kanada's team in 1997. In February 2006 the record for the calculation of √2 was eclipsed with the use of a home computer. Shigeru Kondo calculated 1 trillion decimal places in 2010.^[8] For a development of this record, see the table below. Among mathematical constants with computationally challenging decimal expansions, only π has been calculated more precisely.^[9] Such computations aim to check empirically whether such numbers are normal.

Record progression

This is a table of recent records in calculating digits of √2 ( 1 trillion = 10¹² = 1,000,000,000,000 ).

Reference:^[10]

Date	Name	Number of digits
June 28, 2016	Ron Watkins	10 trillion
April 3, 2016	Ron Watkins	5 trillion
February 9, 2012	Alexander Yee	2 trillion
March 22, 2010	Shigeru Kondo	1 trillion= 1012

Proofs of irrationality

A short proof of the irrationality of √2 can be obtained from the rational root theorem, that is, if p(x) is a monic polynomial with integer coefficients, then any rational root of p(x) is necessarily an integer. Applying this to the polynomial p(x) = x² − 2, it follows that √2 is either an integer or irrational. Because √2 is not an integer (2 is not a perfect square), √2 must therefore be irrational. This proof can be generalized to show that any root of any natural number which is not the square of a natural number is irrational.

See quadratic irrational or infinite descent for a proof that the square root of any non-square natural number is irrational.

Proof by infinite descent

One proof of the number's irrationality is the following proof by infinite descent. It is also a proof by contradiction, also known as an indirect proof, in that the proposition is proved by assuming that the opposite of the proposition is true and showing that this assumption is false, thereby implying that the proposition must be true.

1. Assume that √2 is a rational number, meaning that there exists a pair of integers whose ratio is √2.
2. If the two integers have a common factor, it can be eliminated using the Euclidean algorithm.
3. Then √2 can be written as an irreducible fraction a/b such that a and b are coprime integers (having no common factor).
4. It follows that a²/b² = 2 and a² = 2b².   ( (a/b)ⁿ = aⁿ/bⁿ  )
5. Therefore, a² is even because it is equal to 2b². (2b² is necessarily even because it is 2 times another whole number and multiples of 2 are even.)
6. It follows that a must be even (as squares of odd integers are never even).
7. Because a is even, there exists an integer k that fulfills: a = 2k.
8. Substituting 2k from step 7 for a in the second equation of step 4: 2b² = (2k)² is equivalent to 2b² = 4k², which is equivalent to b² = 2k².
9. Because 2k² is divisible by two and therefore even, and because 2k² = b², it follows that b² is also even which means that b is even.
10. By steps 5 and 8 a and b are both even, which contradicts that a/b is irreducible as stated in step 3.

Q.E.D.

Because there is a contradiction, the assumption (1) that √2 is a rational number must be false. This means that √2 is not a rational number; i.e., √2 is irrational.

This proof was hinted at by Aristotle, in his Analytica Priora, §I.23.^[11] It appeared first as a full proof in Euclid's Elements, as proposition 117 of Book X. However, since the early 19th century historians have agreed that this proof is an interpolation and not attributable to Euclid.^[12]

Proof by unique factorization

An alternative proof uses the same approach with the fundamental theorem of arithmetic which says every integer greater than 1 has a unique factorization into powers of primes.

1. Assume that √2 is a rational number. Then there are integers a and b such that a is coprime to b and √2 = a/b. In other words, √2 can be written as an irreducible fraction.
2. The value of b cannot be 1 as there is no integer a the square of which is 2.
3. There must be a prime p which divides b and which does not divide a, otherwise the fraction would not be irreducible.
4. The square of a can be factored as the product of the primes into which a is factored but with each power doubled.
5. Therefore, by unique factorization the prime p which divides b, and also its square, cannot divide the square of a.
6. Therefore, the square of an irreducible fraction cannot be reduced to an integer.
7. Therefore, √2 cannot be a rational number.

This proof can be generalized to show that if an integer is not an exact kth power of another integer then its kth root is irrational. For a proof of the same result which does not rely on the fundamental theorem of arithmetic.

Proof by infinite descent, not involving factoring

The following reductio ad absurdum argument showing the irrationality of √2 is less well-known. It uses the additional information 2 > √2 > 1 so that 1 > √2 − 1 > 0.^[13]

1. Assume that √2 is a rational number. This would mean that there exist positive integers m and n with n ≠ 0 such that m/n = √2. Then m = n√2 and m√2 = 2n.
2. We may assume that n is the smallest integer so that n√2 is an integer. That is, that the fraction m/n is in lowest terms.
3. Because 1 > √2 − 1 > 0, it follows from (1) that n > n(√2 − 1) = m − n > 0. So n > m − n > 0.
4. Also from (1), we have √2 = m/n = m(√2 − 1)/n(√2 − 1) = 2n − m/m − n.
5. Thus the fraction m/n for √2, which according to (2) is already in lowest terms, is represented by (4) in yet lower terms (which follows from the result (3)). This is a contradiction, so the assumption that √2 is rational must be false.

This argument may be tightened as follows.

Let b be the least positive integer for which √2 is a rational a/b. Then b has the property that twice its square is a square, that is, 2b² = a². For a contradiction, we show that a − b is a smaller positive integer with the same property. Multiply the inequalities 1 > √2 − 1 > 0 by b to show b > a − b > 0. Now twice the square of a − b is 2a² − 4ab + 2b². Rewrite the first and last terms using b's property to yield a² − 4ab + 4b², which is just the expansion of (2b − a)², the promised square. Thus, √2 can also be written as (a − b)/(2 b − a). This procedure can be iterated and understood geometrically, as shown below.

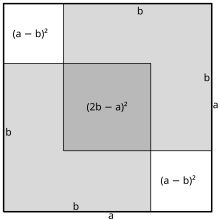
Geometric proof

Figure 1. Stanley Tennenbaum's geometric proof of the irrationality of √2.

The immediately preceding argument has a simple geometric formulation attributed by John Horton Conway to Stanley Tennenbaum when the latter was a student in the early 1950s^[14] and whose most recent appearance is in an article by Noson Yanofsky in the May–June 2016 issue of American Scientist.^[15] Given two squares with integer sides respectively a and b, one of which has twice the area of the other, place two copies of the smaller square in the larger as shown in Figure 1. The square overlap region in the middle ((2b − a)²) must equal the sum of the two uncovered squares (2(a − b)²). But these squares on the diagonal have positive integer sides that are smaller than the original squares. Repeating this process we can find arbitrarily small squares one twice the area of the other, yet both having positive integer sides, which is impossible since positive integers cannot be less than 1.

Figure 2. Tom Apostol's geometric proof of the irrationality of sqrt(2).

Another geometric reductio ad absurdum argument showing that √2 is irrational appeared in 2000 in the American Mathematical Monthly.^[16] It is also an example of proof by infinite descent. It makes use of classic compass and straightedge construction, proving the theorem by a method similar to that employed by ancient Greek geometers. It is essentially the algebraic proof of the previous section viewed geometrically in yet another way.

Let △ABC be a right isosceles triangle with hypotenuse length m and legs n as shown in Figure 2. By the Pythagorean theorem, m/n = √2. Suppose m and n are integers. Let m:n be a ratio given in its lowest terms.

Draw the arcs BD and CE with centre A. Join DE. It follows that AB = AD, AC = AE and the ∠BAC and ∠DAE coincide. Therefore, the triangles ABC and ADE are congruent by SAS.

Because ∠EBF is a right angle and ∠BEF is half a right angle, △BEF is also a right isosceles triangle. Hence BE = m − n implies BF = m − n. By symmetry, DF = m − n, and △FDC is also a right isosceles triangle. It also follows that FC = n − (m − n) = 2n − m.

Hence we have an even smaller right isosceles triangle, with hypotenuse length 2n − m and legs m − n. These values are integers even smaller than m and n and in the same ratio, contradicting the hypothesis that m:n is in lowest terms. Therefore, m and n cannot be both integers, hence √2 is irrational.

Pythagorean theorem proof

The square root of 2 is the diagonal of a square with side lengths 1.

This is another proof by contradiction, supposing that √2 is rational.

1. That means that we can make a right isosceles triangle where the side lengths are natural numbers and the legs and the hypotenuse do not share any common factors (except 1).
2. Since the legs are equal, so are their squares. So in order for the Pythagorean theorem to work for this special right triangle, the square of the hypotenuse has to be an even number (and if we cut it in half once then we have the area of the square of the leg).
3. Recall that the square of an even number is even and the square of an odd number is odd. So if the square of the hypotenuse is even the hypotenuse is even as well.
4. Remember that a square is a quadrilateral with 2 pairs of parallel sides which are equal in length and has 4 right angles. So both sides of the square of the hypotenuse are even.
5. So the square of the hypotenuse of this right triangle can be cut in half twice and still have integer area. Since we only want to cut it in half once, then we'll get an even number.
6. So the square of the leg is even. Now according to (2) the leg must be even.
7. This contradicts our assumption at (1) that the leg and hypotenuse have no common factors (except 1). Because if they're both even they share a common factor of 2. So the assumption that √2 was rational has to be false. Or in other words √2 is an irrational number. Q. E. D.

Analytic proof

• Lemma: let α ∈ ℝ⁺ and p₁, p₂,… q₁, q₂,… ∈ ℕ such that |αq_n − p_n| ≠ 0 for all n ∈ ℕ and

${\displaystyle \lim _{n\rightarrow \infty }p_{n}=\lim _{n\rightarrow \infty }q_{n}=\infty }$

${\displaystyle \lim _{n\rightarrow \infty }\left|\alpha q_{n}-p_{n}\right|=0.}$

Then α is irrational.

Proof: suppose α = a/b with a,b ∈ ℕ⁺.

For sufficiently big n

${\displaystyle 0<\left|\alpha q_{n}-p_{n}\right|<{\frac {1}{b}}}$

then

${\displaystyle 0<\left|{\frac {aq_{n}}{b}}-p_{n}\right|<{\frac {1}{b}}}$

${\displaystyle 0<\left|aq_{n}-bp_{n}\right|<1 annotation="">$

but aq_n − bp_n is an integer, absurd, then α is irrational.

• √2 is irrational.

Proof: let p₁ = q₁ = 1 and

${\displaystyle p_{n+1}=p_{n}^{2}+2q_{n}^{2}}$

${\displaystyle q_{n+1}=2p_{n}q_{n}}$

for all n ∈ ℕ.

By induction,

${\displaystyle 0<\left|{\sqrt {2}}q_{n}-p_{n}\right|<{\frac {1}{2^{2^{n-1}}}}}$

for all n ∈ ℕ. For n = 1,

${\displaystyle 0<\left|{\sqrt {2}}q_{1}-p_{1}\right|<{\frac {1}{2}}}$

and if this is true for n then it is true for n + 1. In fact

${\displaystyle 0<\left|{\sqrt {2}}q_{n}-p_{n}\right|^{2}<{\frac {1}{2^{2^{n}}}}}$

${\displaystyle 0<\left|{\sqrt {2}}(2p_{n}q_{n})-\left(p_{n}^{2}+2q_{n}^{2}\right)\right|<{\frac {1}{2^{2^{n}}}}}$

${\displaystyle 0<\left|{\sqrt {2}}q_{n+1}-p_{n+1}\right|<{\frac {1}{2^{2^{n}}}}.}$

By application of the lemma, √2 is irrational.

Constructive proof

In a constructive approach, one distinguishes between on the one hand not being rational, and on the other hand being irrational (i.e., being quantifiably apart from every rational), the latter being a stronger property. Given positive integers a and b, because the valuation (i.e., highest power of 2 dividing a number) of 2b² is odd, while the valuation of a² is even, they must be distinct integers; thus |2b² − a²| ≥ 1. Then^[17]

${\displaystyle \left|{\sqrt {2}}-{\frac {a}{b}}\right|={\frac {|2b^{2}-a^{2}|}{b^{2}\left({\sqrt {2}}+{\frac {a}{b}}\right)}}\geq {\frac {1}{b^{2}\left({\sqrt {2}}+{\frac {a}{b}}\right)}}\geq {\frac {1}{3b^{2}}},}$

the latter inequality being true because we assume a/b ≤ 3 − √2 (otherwise the quantitative apartness can be trivially established). This gives a lower bound of 1/3b² for the difference |√2 − a/b|, yielding a direct proof of irrationality not relying on the law of excluded middle; see Errett Bishop (1985, p. 18). This proof constructively exhibits a discrepancy between √2 and any rational.

Properties of the square root of two

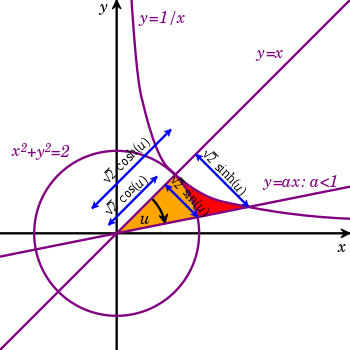
Angle size and sector area are the same when the conic radius is √2. This diagram illustrates the circular and hyperbolic functions based on sector areas u.

One-half of √2, also the reciprocal of √2, approximately 0.707106781186548, is a common quantity in geometry and trigonometry because the unit vector that makes a 45° angle with the axes in a plane has the coordinates

${\displaystyle \left({\frac {\sqrt {2}}{2}},{\frac {\sqrt {2}}{2}}\right).}$

This number satisfies

${\displaystyle {\tfrac {1}{2}}{\sqrt {2}}={\sqrt {\tfrac {1}{2}}}={\frac {1}{\sqrt {2}}}=\cos 45^{\circ }=\sin 45^{\circ }.}$

One interesting property of √2 is as follows:

${\displaystyle \!\ {1 \over {{\sqrt {2}}-1}}={\sqrt {2}}+1}$

since

${\displaystyle \left({\sqrt {2}}+1\right)\left({\sqrt {2}}-1\right)=2-1=1.}$

This is related to the property of silver ratios.

√2 can also be expressed in terms of the copies of the imaginary unit i using only the square root and arithmetic operations:

${\displaystyle {\frac {{\sqrt {i}}+i{\sqrt {i}}}{i}}{\text{ and }}{\frac {{\sqrt {-i}}-i{\sqrt {-i}}}{-i}}}$

if the square root symbol is interpreted suitably for the complex numbers i and −i.

√2 is also the only real number other than 1 whose infinite tetrate (i.e., infinite exponential tower) is equal to its square. In other words: if for c > 1 we define x₁ = c and x_n+1 = c^x_n for n > 1, we will call the limit of x_n as n → ∞ (if this limit exists) f(c). Then √2 is the only number c > 1 for which f(c) = c². Or symbolically:

${\displaystyle {\sqrt {2}}^{({\sqrt {2}}^{({\sqrt {2}}^{(\ \cdot ^{\cdot ^{\cdot })))}}}}=2.}$

√2 appears in Viète's formula for π:

${\displaystyle 2^{m}{\sqrt {2-{\sqrt {2+{\sqrt {2+\cdots +{\sqrt {2}}}}}}}}\to \pi {\text{ as }}m\to \infty }$

for m square roots and only one minus sign.^[18]

Similar in appearance but with a finite number of terms, √2 appears in various trigonometric constants:^[19]

${\displaystyle \sin(\pi /32)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(\pi /16)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2}}}}}};}$

${\displaystyle \sin(3\pi /32)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2+{\sqrt {2-{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(\pi /8)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2}}}};}$

${\displaystyle \sin(5\pi /32)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2-{\sqrt {2-{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(3\pi /16)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2-{\sqrt {2}}}}}};}$

${\displaystyle \sin(7\pi /32)={\tfrac {1}{2}}{\sqrt {2-{\sqrt {2-{\sqrt {2+{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(\pi /4)={\tfrac {1}{2}}{\sqrt {2}};}$

${\displaystyle \sin(9\pi /32)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2-{\sqrt {2+{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(5\pi /16)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2-{\sqrt {2}}}}}};}$

${\displaystyle \sin(11\pi /32)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2-{\sqrt {2-{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(3\pi /8)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2}}}};}$

${\displaystyle \sin(13\pi /32)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2-{\sqrt {2}}}}}}}};}$

${\displaystyle \sin(7\pi /16)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}};}$

${\displaystyle \sin(15\pi /32)={\tfrac {1}{2}}{\sqrt {2+{\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}}}.}$

It is not known whether √2 is a normal number, a stronger property than irrationality, but statistical analyses of its binary expansion are consistent with the hypothesis that it is normal to base two.^[20]

Series and product representations

The identity cos π/4 = sin π/4 = 1/√2, along with the infinite product representations for the sine and cosine, leads to products such as

${\displaystyle {\frac {1}{\sqrt {2}}}=\prod _{k=0}^{\infty }\left(1-{\frac {1}{(4k+2)^{2}}}\right)=\left(1-{\frac {1}{4}}\right)\left(1-{\frac {1}{36}}\right)\left(1-{\frac {1}{100}}\right)\cdots }$

and

${\displaystyle {\sqrt {2}}=\prod _{k=0}^{\infty }{\frac {(4k+2)^{2}}{(4k+1)(4k+3)}}=\left({\frac {2\cdot 2}{1\cdot 3}}\right)\left({\frac {6\cdot 6}{5\cdot 7}}\right)\left({\frac {10\cdot 10}{9\cdot 11}}\right)\left({\frac {14\cdot 14}{13\cdot 15}}\right)\cdots }$

or equivalently,

${\displaystyle {\sqrt {2}}=\prod _{k=0}^{\infty }\left(1+{\frac {1}{4k+1}}\right)\left(1-{\frac {1}{4k+3}}\right)=\left(1+{\frac {1}{1}}\right)\left(1-{\frac {1}{3}}\right)\left(1+{\frac {1}{5}}\right)\left(1-{\frac {1}{7}}\right)\cdots .}$

The number can also be expressed by taking the Taylor series of a trigonometric function. For example, the series for cos π/4 gives

${\displaystyle {\frac {1}{\sqrt {2}}}=\sum _{k=0}^{\infty }{\frac {(-1)^{k}\left({\frac {\pi }{4}}\right)^{2k}}{(2k)!}}.}$

The Taylor series of √1 + x with x = 1 and using the double factorial n!! gives

${\displaystyle {\sqrt {2}}=\sum _{k=0}^{\infty }(-1)^{k+1}{\frac {(2k-3)!!}{(2k)!!}}=1+{\frac {1}{2}}-{\frac {1}{2\cdot 4}}+{\frac {1\cdot 3}{2\cdot 4\cdot 6}}-{\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6\cdot 8}}+\cdots .}$

The convergence of this series can be accelerated with an Euler transform, producing

${\displaystyle {\sqrt {2}}=\sum _{k=0}^{\infty }{\frac {(2k+1)!}{2^{3k+1}(k!)^{2}}}={\frac {1}{2}}+{\frac {3}{8}}+{\frac {15}{64}}+{\frac {35}{256}}+{\frac {315}{4096}}+{\frac {693}{16384}}+\cdots .}$

It is not known whether √2 can be represented with a BBP-type formula. BBP-type formulas are known for π√2 and √2ln(1+√2), however.^[21]

The number can be represented by an infinite series of Egyptian fractions, with denominators defined by 2ⁿth terms of a Fibonacci-like recurrence relation a(n)=34a(n-1)-a(n-2), a(0)=0, a(1)=6.^[22]

${\displaystyle {\sqrt {2}}={\frac {3}{2}}-{\frac {1}{2}}\sum _{n=0}^{\infty }{\frac {1}{a(2^{n})}}={\frac {3}{2}}-{\frac {1}{2}}\left({\frac {1}{6}}+{\frac {1}{204}}+{\frac {1}{235416}}+\dots \right)}$

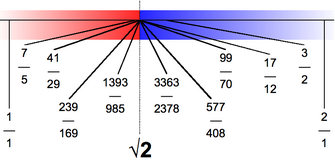
Continued fraction representation

The square root of 2 and approximations by convergents of continued fractions

The square root of two has the following continued fraction representation:

${\displaystyle \!\ {\sqrt {2}}=1+{\cfrac {1}{2+{\cfrac {1}{2+{\cfrac {1}{2+{\cfrac {1}{2+\ddots }}}}}}}}.}$

The convergents formed by truncating this representation form a sequence of fractions that approximate the square root of two to increasing accuracy, and that are described by the Pell numbers (known as side and diameter numbers to the ancient Greeks because of their use in approximating the ratio between the sides and diagonal of a square). The first convergents are: 1/1, 3/2, 7/5, 17/12, 41/29, 99/70, 239/169, 577/408. The convergent p/q differs from √2 by almost exactly 1/2q²√2^{[citation needed]} and then the next convergent is p + 2q/p + q.

Nested square representations

The following nested square expressions converge to ${\displaystyle {\sqrt {2}}}$:

${\displaystyle \!\ {\sqrt {2}}={\frac {3}{2}}-2\left({\frac {1}{4}}-\left({\frac {1}{4}}-\left({\frac {1}{4}}-\left({\frac {1}{4}}-\dots \right)^{2}\right)^{2}\right)^{2}\right)^{2}={\frac {3}{2}}-4\left({\frac {1}{8}}+\left({\frac {1}{8}}+\left({\frac {1}{8}}+\left({\frac {1}{8}}+\dots \right)^{2}\right)^{2}\right)^{2}\right)^{2}.}$

Derived constants

The reciprocal of the square root of two (the square root of 1/2) is a widely used constant.
${\displaystyle {\frac {1}{\sqrt {2}}}={\frac {\sqrt {2}}{2}}=\sin 45^{\circ }=\cos 45^{\circ }=0.70710\,67811\,86547\,52440\,08443\,62104\,84903\,928...}$ (sequence A010503 in the OEIS)

Paper size

The (approximate) aspect ratio of paper sizes under ISO 216 (A4, A0, etc.) is 1:√2. This ratio of lengths of the shorter over the longer side guarantees that cutting a sheet in half along a line parallel to its shorter side results in the smaller sheets having the same (approximate) ratio as the original sheet.

Proof:
Let ${\displaystyle S=}$ shorter length and ${\displaystyle L=}$ longer length of the sides of a sheet of paper, with

${\displaystyle R={\frac {L}{S}}={\sqrt {2}}}$ as required by ISO 216.

Let ${\displaystyle R'={\frac {L'}{S'}}}$ be the analogue ratio of the halved sheet, then

${\displaystyle R'={\frac {S}{L/2}}={\frac {2S}{L}}={\frac {2}{(L/S)}}={\frac {2}{\sqrt {2}}}={\sqrt {2}}=R}$.