Roche limit

From Wikipedia, the free encyclopedia

 

This article is about the distance within which an orbiting object will form rings. For the limits at which an orbiting object will be captured, see Roche lobe. For the gravitational sphere of influence of one astronomical body in the face of perturbations from another heavier body around which it orbits, see Roche sphere.

 

A celestial body (yellow) is orbited by a mass of fluid (blue) held together by gravity, here viewed from above the orbital plane. Far from the Roche limit (white line), the mass is practically spherical.

 

Closer to the Roche limit, the body is deformed by tidal forces.

 

Within the Roche limit, the mass' own gravity can no longer withstand the tidal forces, and the body disintegrates.

 

Particles closer to the primary move more quickly than particles farther away, as represented by the red arrows.

 

The varying orbital speed of the material eventually causes it to form a ring.

In celestial mechanics, the Roche limit, also called Roche radius, is the distance from a celestial body within which a second celestial body, held together only by its own force of gravity, will disintegrate because the first body's tidal forces exceed the second body's gravitational self-attraction. Inside the Roche limit, orbiting material disperses and forms rings, whereas outside the limit material tends to coalesce. The Roche radius depends on the radius of the first body and on the ratio of the bodies' densities.

The term is named after Édouard Roche (French: [ʁɔʃ], English: /rɒʃ/ ROSH), who was the French astronomer who first calculated this theoretical limit in 1848.

Explanation

Comet Shoemaker-Levy 9 was disintegrated by the tidal forces of Jupiter into a string of smaller bodies in 1992, before colliding with the planet in 1994.

The Roche limit typically applies to a satellite's disintegrating due to tidal forces induced by its primary, the body around which it orbits. Parts of the satellite that are closer to the primary are attracted more strongly by gravity from the primary than parts that are farther away; this disparity effectively pulls the near and far parts of the satellite apart from each other, and if the disparity (combined with any centrifugal effects due to the object's spin) is larger than the force of gravity holding the satellite together, it can pull the satellite apart. Some real satellites, both natural and artificial, can orbit within their Roche limits because they are held together by forces other than gravitation. Objects resting on the surface of such a satellite would be lifted away by tidal forces. A weaker satellite, such as a comet, could be broken up when it passes within its Roche limit.

Since, within the Roche limit, tidal forces overwhelm the gravitational forces that might otherwise hold the satellite together, no satellite can gravitationally coalesce out of smaller particles within that limit. Indeed, almost all known planetary rings are located within their Roche limit. (Notable exceptions are Saturn's E-Ring and Phoebe ring. These two rings could possibly be remnants from the planet's proto-planetary accretion disc that failed to coalesce into moonlets, or conversely have formed when a moon passed within its Roche limit and broke apart.)

The Roche limit is not the only factor that causes comets to break apart. Splitting by thermal stress, internal gas pressure and rotational splitting are other ways for a comet to split under stress.

Selected examples

The table below shows the mean density and the equatorial radius for selected objects in the Solar System.

Primary	Density (kg/m3)	Radius (m)
Sun	1,408	696,000,000
Earth	5,513	6,378,137
Moon	3,346	1,737,100
Jupiter	1,326	71,493,000
Saturn	687	60,267,000
Uranus	1,318	25,557,000
Neptune	1,638	24,766,000

The equations for the Roche limits relate the minimum sustainable orbital radius to the ratio of the two objects' densities and the radius of the primary body. Hence, using the data above, the Roche limits for these objects can be calculated. This has been done twice for each, assuming the extremes of the rigid and fluid body cases. The average density of comets is taken to be around 500 kg/m³.

The table below gives the Roche limits expressed in kilometres and in primary radii. The mean radius of the orbit can be compared with the Roche limits. For convenience, the table lists the mean radius of the orbit for each, excluding the comets, whose orbits are extremely variable and eccentric.

Body	Satellite	Roche limit (rigid)		Roche limit (fluid)		Mean orbital radius (km)
		Distance (km)	R	Distance (km)	R
Earth	Moon	9,492	1.49	18,381	2.88	384,399
Earth	average comet	17,887	2.80	34,638	5.43	N/A
Sun	Earth	556,397	0.80	1,077,467	1.55	149,597,890
Sun	Jupiter	894,677	1.29	1,732,549	2.49	778,412,010
Sun	Moon	657,161	0.94	1,272,598	1.83	149,597,890 approximately
Sun	average comet	1,238,390	1.78	2,398,152	3.45	N/A

These bodies are well outside their Roche limits by various factors, from 21 for the Moon (over its fluid-body Roche limit) as part of the Earth–Moon system, upwards to hundreds for Earth and Jupiter.

The table below gives each satellite's closest approach in its orbit divided by its own Roche limit. Again, both rigid and fluid body calculations are given. Note that Pan, Cordelia and Naiad, in particular, may be quite close to their actual break-up points.

In practice, the densities of most of the inner satellites of giant planets are not known. In these cases, shown in italics, likely values have been assumed, but their actual Roche limit can vary from the value shown.

Primary	Satellite	Orbital radius / Roche limit
		(rigid)	(fluid)
Sun	Mercury	104:1	54:1
Earth	Moon	41:1	21:1
Mars	Phobos	172%	89%
	Deimos	451%	234%
Jupiter	Metis	~186%	~94%
	Adrastea	~188%	~95%
	Amalthea	175%	88%
	Thebe	254%	128%
Saturn	Pan	142%	70%
	Atlas	156%	78%
	Prometheus	162%	80%
	Pandora	167%	83%
	Epimetheus	200%	99%
	Janus	195%	97%
Uranus	Cordelia	~154%	~79%
	Ophelia	~166%	~86%
	Bianca	~183%	~94%
	Cressida	~191%	~98%
	Desdemona	~194%	~100%
	Juliet	~199%	~102%
Neptune	Naiad	~139%	~72%
	Thalassa	~145%	~75%
	Despina	~152%	~78%
	Galatea	153%	79%
	Larissa	~218%	~113%
Pluto	Charon	12.5:1	6.5:1

Determination

The limiting distance to which a satellite can approach without breaking up depends on the rigidity of the satellite. At one extreme, a completely rigid satellite will maintain its shape until tidal forces break it apart. At the other extreme, a highly fluid satellite gradually deforms leading to increased tidal forces, causing the satellite to elongate, further compounding the tidal forces and causing it to break apart more readily.

Most real satellites would lie somewhere between these two extremes, with tensile strength rendering the satellite neither perfectly rigid nor perfectly fluid. For example, a rubble-pile asteroid will behave more like a fluid than a solid rocky one; an icy body will behave quite rigidly at first but become more fluid as tidal heating accumulates and its ices begin to melt.

But note that, as defined above, the Roche limit refers to a body held together solely by the gravitational forces which cause otherwise unconnected particles to coalesce, thus forming the body in question. The Roche limit is also usually calculated for the case of a circular orbit, although it is straightforward to modify the calculation to apply to the case (for example) of a body passing the primary on a parabolic or hyperbolic trajectory.

Rigid-satellite calculation

The rigid-body Roche limit is a simplified calculation for a spherical satellite. Irregular shapes such as those of tidal deformation on the body or the primary it orbits are neglected. It is assumed to be in hydrostatic equilibrium. These assumptions, although unrealistic, greatly simplify calculations.

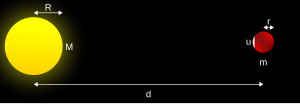
The Roche limit for a rigid spherical satellite is the distance, ${\displaystyle d}$, from the primary at which the gravitational force on a test mass at the surface of the object is exactly equal to the tidal force pulling the mass away from the object:

${\displaystyle d=R_{M}\left(2{\frac {\rho _{M}}{\rho _{m}}}\right)^{\frac {1}{3}}}$

where ${\displaystyle R_{M}}$ is the radius of the primary, ${\displaystyle \rho _{M}}$ is the density of the primary, and ${\displaystyle \rho _{m}}$ is the density of the satellite. This can be equivalently written as

${\displaystyle d=R_{m}\left(2{\frac {M_{M}}{M_{m}}}\right)^{\frac {1}{3}}}$

where ${\displaystyle R_{m}}$ is the radius of the secondary, ${\displaystyle M_{M}}$ is the mass of the primary, and ${\displaystyle M_{m}}$ is the mass of the secondary.

This does not depend on the size of the objects, but on the ratio of densities. This is the orbital distance inside of which loose material (e.g. regolith) on the surface of the satellite closest to the primary would be pulled away, and likewise material on the side opposite the primary will also go away from, rather than toward, the satellite.

Note that this is an approximate result as inertia force and rigid structure are ignored in its derivation.

The orbital period then depends only on the density of the secondary:

${\displaystyle P=2\pi \left({\frac {d^{3}}{GM_{M}}}\right)^{1/2}=2\pi \left({\frac {d^{3}}{(4/3)\pi GR_{M}^{3}\rho _{M}}}\right)^{1/2}={\sqrt {\frac {6\pi }{G\rho _{m}}}}}$

where G is the gravitational constant. For example, a density of 3.346 g/cc (the density of our moon) corresponds to an orbital period of 2.552 hours.

Derivation of the formula

Derivation of the Roche limit

In order to determine the Roche limit, consider a small mass ${\displaystyle u}$ on the surface of the satellite closest to the primary. There are two forces on this mass ${\displaystyle u}$: the gravitational pull towards the satellite and the gravitational pull towards the primary. Assume that the satellite is in free fall around the primary and that the tidal force is the only relevant term of the gravitational attraction of the primary. This assumption is a simplification as free-fall only truly applies to the planetary center, but will suffice for this derivation.

The gravitational pull ${\displaystyle F_{\text{G}}}$ on the mass ${\displaystyle u}$ towards the satellite with mass ${\displaystyle m}$ and radius ${\displaystyle r}$ can be expressed according to Newton's law of gravitation.

${\displaystyle F_{\text{G}}={\frac {Gmu}{r^{2}}}}$

the tidal force ${\displaystyle F_{\text{T}}}$ on the mass ${\displaystyle u}$ towards the primary with radius ${\displaystyle R}$ and mass ${\displaystyle M}$, at a distance ${\displaystyle d}$ between the centers of the two bodies, can be expressed approximately as

${\displaystyle F_{\text{T}}={\frac {2GMur}{d^{3}}}}$.

To obtain this approximation, find the difference in the primary's gravitational pull on the center of the satellite and on the edge of the satellite closest to the primary:

${\displaystyle F_{\text{T}}={\frac {GMu}{(d-r)^{2}}}-{\frac {GMu}{d^{2}}}}$

${\displaystyle F_{\text{T}}=GMu{\frac {d^{2}-(d-r)^{2}}{d^{2}(d-r)^{2}}}}$

${\displaystyle F_{\text{T}}=GMu{\frac {2dr-r^{2}}{d^{4}-2d^{3}r+r^{2}d^{2}}}}$

In the approximation where ${\displaystyle r\ll R}$ and ${\displaystyle R<d}$, it can be said that the ${\displaystyle r^{2}}$ in the numerator and every term with ${\displaystyle r}$ in the denominator goes to zero, which gives us:

${\displaystyle F_{\text{T}}=GMu{\frac {2dr}{d^{4}}}}$

${\displaystyle F_{\text{T}}={\frac {2GMur}{d^{3}}}}$

The Roche limit is reached when the gravitational force and the tidal force balance each other out.

${\displaystyle F_{\text{G}}=F_{\text{T}}\;}$

or

${\displaystyle {\frac {Gmu}{r^{2}}}={\frac {2GMur}{d^{3}}}}$,

which gives the Roche limit, ${\displaystyle d}$, as

${\displaystyle d=r\left(2\,{\frac {M}{m}}\right)^{\frac {1}{3}}}$

The radius of the satellite should not appear in the expression for the limit, so it is re-written in terms of densities.

For a sphere the mass ${\displaystyle M}$ can be written as

${\displaystyle M={\frac {4\pi \rho _{M}R^{3}}{3}}}$ where ${\displaystyle R}$ is the radius of the primary.

And likewise

${\displaystyle m={\frac {4\pi \rho _{m}r^{3}}{3}}}$ where ${\displaystyle r}$ is the radius of the satellite.

Substituting for the masses in the equation for the Roche limit, and cancelling out ${\displaystyle 4\pi /3}$ gives

${\displaystyle d=r\left({\frac {2\rho _{M}R^{3}}{\rho _{m}r^{3}}}\right)^{1/3}}$,

which can be simplified to the following Roche limit:

${\displaystyle d=R\left(2\,{\frac {\rho _{M}}{\rho _{m}}}\right)^{\frac {1}{3}}\approx 1.26R\left({\frac {\rho _{M}}{\rho _{m}}}\right)^{\frac {1}{3}}}$.

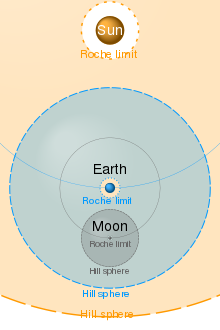
Roche limit, Hill sphere and radius of the planet

Comparison of the Hill spheres and Roche limits of the Sun-Earth-Moon system (not to scale) with shaded regions denoting stable orbits of satellites of each body

Consider a planet with a density of ${\displaystyle \rho _{m}}$ and a radius of ${\displaystyle r}$, orbiting a star withis is the physical meaning of Roche limit, Roche lobe and Hill sphere.

Formula(2) can be described as: ${\displaystyle R_{\text{Roche}}=R_{\text{Hill}}{\sqrt[{3}]{\frac {3M}{m}}}=R_{\text{secondary}}{\sqrt[{3}]{\frac {3M}{m}}}}$, a perfect mathematical symmetry.
This is the astronomical significance of Roche limit and Hill sphere.

Note : Roche limit and Hill sphere are completely different from each other but are both work of Édouard Roche.

Hill sphere of an astronomical body is the region in which it dominates the attraction of satellites whereas Roche limit is the minimum distance to which a satellite can approach its primary body without tidal force overcoming the internal gravity holding the satellite together.

Fluid satellites

A more accurate approach for calculating the Roche limit takes the deformation of the satellite into account. An extreme example would be a tidally locked liquid satellite orbiting a planet, where any force acting upon the satellite would deform it into a prolate spheroid.

The calculation is complex and its result cannot be represented in an exact algebraic formula. Roche himself derived the following approximate solution for the Roche limit:

${\displaystyle d\approx 2.44R\left({\frac {\rho _{M}}{\rho _{m}}}\right)^{1/3}}$

However, a better approximation that takes into account the primary's oblateness and the satellite's mass is:

${\displaystyle d\approx 2.423R\left({\frac {\rho _{M}}{\rho _{m}}}\right)^{1/3}\left({\frac {(1+{\frac {m}{3M}})+{\frac {c}{3R}}(1+{\frac {m}{M}})}{1-c/R}}\right)^{1/3}}$

where ${\displaystyle c/R}$ is the oblateness of the primary. The numerical factor is calculated with the aid of a computer.

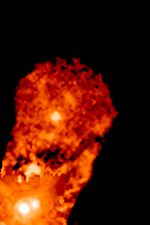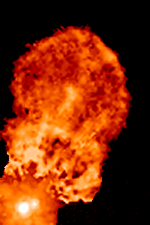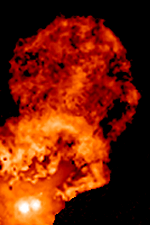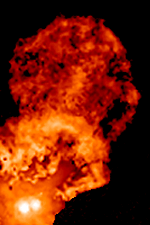
The fluid solution is appropriate for bodies that are only loosely held together, such as a comet. For instance, comet Shoemaker–Levy 9's decaying orbit around Jupiter passed within its Roche limit in July 1992, causing it to fragment into a number of smaller pieces. On its next approach in 1994 the fragments crashed into the planet. Shoemaker–Levy 9 was first observed in 1993, but its orbit indicated that it had been captured by Jupiter a few decades prior.

Derivation of the formula

As the fluid satellite case is more delicate than the rigid one, the satellite is described with some simplifying assumptions. First, assume the object consists of incompressible fluid that has constant density ${\displaystyle \rho _{m}}$ and volume ${\displaystyle V}$ that do not depend on external or internal forces.

Second, assume the satellite moves in a circular orbit and it remains in synchronous rotation. This means that the angular speed ${\displaystyle \omega }$ at which it rotates around its center of mass is the same as the angular speed at which it moves around the overall system barycenter.

The angular speed ${\displaystyle \omega }$ is given by Kepler's third law:

${\displaystyle \omega ^{2}=G\,{\frac {M+m}{d^{3}}}.}$

When M is very much bigger than m, this will be close to

${\displaystyle \omega ^{2}=G\,{\frac {M}{d^{3}}}.}$

The synchronous rotation implies that the liquid does not move and the problem can be regarded as a static one. Therefore, the viscosity and friction of the liquid in this model do not play a role, since these quantities would play a role only for a moving fluid.

Given these assumptions, the following forces should be taken into account:

• The force of gravitation due to the main body;
• the centrifugal force in the rotary reference system; and
• the self-gravitation field of the satellite.

Since all of these forces are conservative, they can be expressed by means of a potential. Moreover, the surface of the satellite is an equipotential one. Otherwise, the differences of potential would give rise to forces and movement of some parts of the liquid at the surface, which contradicts the static model assumption. Given the distance from the main body, the form of the surface that satisfies the equipotential condition must be determined.

Radial distance of one point on the surface of the ellipsoid to the center of mass

As the orbit has been assumed circular, the total gravitational force and orbital centrifugal force acting on the main body cancel. That leaves two forces: the tidal force and the rotational centrifugal force. The tidal force depends on the position with respect to the center of mass, already considered in the rigid model. For small bodies, the distance of the liquid particles from the center of the body is small in relation to the distance d to the main body. Thus the tidal force can be linearized, resulting in the same formula for F_T as given above.

While this force in the rigid model depends only on the radius r of the satellite, in the fluid case, all the points on the surface must be considered, and the tidal force depends on the distance Δd from the center of mass to a given particle projected on the line joining the satellite and the main body. We call Δd the radial distance. Since the tidal force is linear in Δd, the related potential is proportional to the square of the variable and for ${\displaystyle m\ll M}$ we have

${\displaystyle V_{T}=-{\frac {3GM}{2d^{3}}}\Delta d^{2}\,}$

Likewise, the centrifugal force has a potential

${\displaystyle V_{C}=-{\frac {1}{2}}\omega ^{2}\Delta d^{2}=-{\frac {GM}{2d^{3}}}\Delta d^{2}\,}$

for rotational angular velocity ${\displaystyle \omega }$.

We want to determine the shape of the satellite for which the sum of the self-gravitation potential and V_T + V_C is constant on the surface of the body. In general, such a problem is very difficult to solve, but in this particular case, it can be solved by a skillful guess due to the square dependence of the tidal potential on the radial distance Δd To a first approximation, we can ignore the centrifugal potential V_C and consider only the tidal potential V_T.

Since the potential V_T changes only in one direction, i.e. the direction toward the main body, the satellite can be expected to take an axially symmetric form. More precisely, we may assume that it takes a form of a solid of revolution. The self-potential on the surface of such a solid of revolution can only depend on the radial distance to the center of mass. Indeed, the intersection of the satellite and a plane perpendicular to the line joining the bodies is a disc whose boundary by our assumptions is a circle of constant potential. Should the difference between the self-gravitation potential and V_T be constant, both potentials must depend in the same way on Δd. In other words, the self-potential has to be proportional to the square of Δd. Then it can be shown that the equipotential solution is an ellipsoid of revolution. Given a constant density and volume the self-potential of such body depends only on the eccentricity ε of the ellipsoid:

${\displaystyle V_{s}=V_{s_{0}}+G\pi \rho _{m}\cdot f(\varepsilon )\cdot \Delta d^{2},}$

where ${\displaystyle V_{s_{0}}}$ is the constant self-potential on the intersection of the circular edge of the body and the central symmetry plane given by the equation Δd=0.

The dimensionless function f is to be determined from the accurate solution for the potential of the ellipsoid

${\displaystyle f(\varepsilon )={\frac {1-\varepsilon ^{2}}{\varepsilon ^{3}}}\cdot \left[\left(3-\varepsilon ^{2}\right)\cdot \operatorname {artanh} \varepsilon -3\varepsilon \right]}$

and, surprisingly enough, does not depend on the volume of the satellite.

The graph of the dimensionless function f which indicates how the strength of the tidal potential depends on the eccentricity ε of the ellipsoid.

Although the explicit form of the function f looks complicated, it is clear that we may and do choose the value of ε so that the potential V_T is equal to V_S plus a constant independent of the variable Δd. By inspection, this occurs when

${\displaystyle {\frac {2G\pi \rho _{M}R^{3}}{d^{3}}}=G\pi \rho _{m}f(\varepsilon )}$

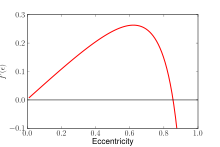
This equation can be solved numerically. The graph indicates that there are two solutions and thus the smaller one represents the stable equilibrium form (the ellipsoid with the smaller eccentricity). This solution determines the eccentricity of the tidal ellipsoid as a function of the distance to the main body. The derivative of the function f has a zero where the maximal eccentricity is attained. This corresponds to the Roche limit.

The derivative of f determines the maximal eccentricity. This gives the Roche limit.

More precisely, the Roche limit is determined by the fact that the function f, which can be regarded as a nonlinear measure of the force squeezing the ellipsoid towards a spherical shape, is bounded so that there is an eccentricity at which this contracting force becomes maximal. Since the tidal force increases when the satellite approaches the main body, it is clear that there is a critical distance at which the ellipsoid is torn up.

The maximal eccentricity can be calculated numerically as the zero of the derivative of f'. One obtains

${\displaystyle \varepsilon _{\text{max}}\approx 0{.}86}$

which corresponds to the ratio of the ellipsoid axes 1:1.95. Inserting this into the formula for the function f one can determine the minimal distance at which the ellipsoid exists. This is the Roche limit,

${\displaystyle d\approx 2{.}423\cdot R\cdot {\sqrt[{3}]{\frac {\rho _{M}}{\rho _{m}}}}\,.}$

Surprisingly, including the centrifugal potential makes remarkably little difference, though the object becomes a Roche ellipsoid, a general triaxial ellipsoid with all axes having different lengths. The potential becomes a much more complicated function of the axis lengths, requiring elliptic functions. However, the solution proceeds much as in the tidal-only case, and we find

${\displaystyle d\approx 2{.}455\cdot R\cdot {\sqrt[{3}]{\frac {\rho _{M}}{\rho _{m}}}}\,.}$

The ratios of polar to orbit-direction to primary-direction axes are 1:1.06:2.07.

 

T Tauri star

From Wikipedia, the free encyclopedia

 

Artist's impression of a T Tauri star with a circumstellar accretion disc

 

Star formation
Object classes
Interstellar medium Molecular cloud Bok globule Dark nebula Young stellar object Protostar Pre-main-sequence star T Tauri star Herbig Ae/Be star Herbig–Haro object
Theoretical concepts
Accretion Initial mass function Jeans instability Kelvin–Helmholtz mechanism Nebular hypothesis Planetary migration

T Tauri stars (TTS) are a class of variable stars that are less than about ten million years old. This class is named after the prototype, T Tauri, a young star in the Taurus star-forming region. They are found near molecular clouds and identified by their optical variability and strong chromospheric lines. T Tauri stars are pre-main-sequence stars in the process of contracting to the main sequence along the Hayashi track, a luminosity–temperature relationship obeyed by infant stars of less than 3 solar masses (M_☉) in the pre-main-sequence phase of stellar evolution. It ends when a star of 0.5 M_☉ or larger develops a radiative zone, or when a smaller star commences nuclear fusion on the main sequence.

History

While T Tauri itself was discovered in 1852, the T Tauri class of stars were initially defined by Alfred Harrison Joy in 1945.

Characteristics

T Tauri stars comprise the youngest visible F, G, K and M spectral type stars (<2 M_☉). Their surface temperatures are similar to those of main-sequence stars of the same mass, but they are significantly more luminous because their radii are larger. Their central temperatures are too low for hydrogen fusion. Instead, they are powered by gravitational energy released as the stars contract, while moving towards the main sequence, which they reach after about 100 million years. They typically rotate with a period between one and twelve days, compared to a month for the Sun, and are very active and variable.

There is evidence of large areas of starspot coverage, and they have intense and variable X-ray and radio emissions (approximately 1000 times that of the Sun). Many have extremely powerful stellar winds; some eject gas in high-velocity bipolar jets. Another source of brightness variability are clumps (protoplanets and planetesimals) in the disk surrounding T Tauri stars.

The ejection of a bubble of hot gas from XZ Tauri, a binary system of T Tauri stars. The scale is much larger than that of the Solar System.

Their spectra show a higher lithium abundance than the Sun and other main-sequence stars because lithium is destroyed at temperatures above 2,500,000 K. From a study of lithium abundances in 53 T Tauri stars, it has been found that lithium depletion varies strongly with size, suggesting that "lithium burning" by the p-p chain during the last highly convective and unstable stages during the later pre–main sequence phase of the Hayashi contraction may be one of the main sources of energy for T Tauri stars. Rapid rotation tends to improve mixing and increase the transport of lithium into deeper layers where it is destroyed. T Tauri stars generally increase their rotation rates as they age, through contraction and spin-up, as they conserve angular momentum. This causes an increased rate of lithium loss with age. Lithium burning will also increase with higher temperatures and mass, and will last for at most a little over 100 million years.

The p-p chain for lithium burning is as follows

p	+	6 3Li	→	7 4Be
7 4Be	+	e−	→	7 3Li	+  ν
p	+	7 3Li	→	8 4Be		(unstable)
		8 4Be	→	2 4 2He	+ energy

It will not occur in stars with less than sixty times the mass of Jupiter (M_J). In this way, the rate of lithium depletion can be used to calculate the age of the star.

Types

Several types of TTSs exist:

• Classical T Tauri star (CTTS)
• Weak-line T Tauri star (WTTS)
  • Naked T Tauri star (NTTS), which is a subset of WTTS.

Protoplanetary discs in the Orion Nebula

Roughly half of T Tauri stars have circumstellar disks, which in this case are called protoplanetary discs because they are probably the progenitors of planetary systems like the Solar System. Circumstellar discs are estimated to dissipate on timescales of up to 10 million years. Most T Tauri stars are in binary star systems. In various stages of their life, they are called young stellar object (YSOs). It is thought that the active magnetic fields and strong solar wind of Alfvén waves of T Tauri stars are one means by which angular momentum gets transferred from the star to the protoplanetary disc. A T Tauri stage for the Solar System would be one means by which the angular momentum of the contracting Sun was transferred to the protoplanetary disc and hence, eventually to the planets.

Analogs of T Tauri stars in the higher mass range (2–8 solar masses)—A and B spectral type pre–main-sequence stars, are called Herbig Ae/Be-type stars. More massive (>8 solar masses) stars in pre–main sequence stage are not observed, because they evolve very quickly: when they become visible (i.e. disperses surrounding circumstellar gas and dust cloud), the hydrogen in the center is already burning and they are main sequence objects.

Planets

Planets around T Tauri stars include:

• HD 106906 b around an F-type star
• 1RXS J160929.1−210524 around a K-type star
• Gliese 674 b around an M-type star
• V830 Tau b around an M-type star
• PDS 70b around a K-type star

 

Hot Jupiter

From Wikipedia, the free encyclopedia

 

Artist's impression of HD 188753 b, a hot Jupiter

Hot Jupiters are a class of gas giant exoplanets that are inferred to be physically similar to Jupiter but that have very short orbital periods (P < 10 days). The close proximity to their stars and high surface-atmosphere temperatures resulted in the moniker "hot Jupiters".

Hot Jupiters are the easiest extrasolar planets to detect via the radial-velocity method, because the oscillations they induce in their parent stars' motion are relatively large and rapid compared to those of other known types of planets. One of the best-known hot Jupiters is 51 Pegasi b. Discovered in 1995, it was the first extrasolar planet found orbiting a Sun-like star. 51 Pegasi b has an orbital period of about 4 days.

General characteristics

Hot Jupiters (along left edge, including most of planets detected using the transit method, indicated with black dots) discovered up to 2 January 2014

 

Hot Jupiter with hidden water

Though there is diversity among hot Jupiters, they do share some common properties.

• Their defining characteristics are their large masses and short orbital periods, spanning 0.36–11.8 Jupiter masses and 1.3–111 Earth days. The mass cannot be greater than approximately 13.6 Jupiter masses because then the pressure and temperature inside the planet would be high enough to cause deuterium fusion, and the planet would be a brown dwarf.
• Most have nearly circular orbits (low eccentricities). It is thought that their orbits are circularized by perturbations from nearby stars or tidal forces. Whether they remain in these circular orbits for long periods of time or collide with their host stars depends on the coupling of their orbital and physical evolution, which are related through the dissipation of energy and tidal deformation.
• Many have unusually low densities. The lowest one measured thus far is that of TrES-4 at 0.222 g/cm³. The large radii of hot Jupiters are not yet fully understood but it is thought that the expanded envelopes can be attributed to high stellar irradiation, high atmospheric opacities, possible internal energy sources, and orbits close enough to their stars for the outer layers of the planets to exceed their Roche limit and be pulled further outward.
• Usually they are tidally locked, with one side always facing its host star.
• They are likely to have extreme and exotic atmospheres due to their short periods, relatively long days, and tidal locking.
• Atmospheric dynamics models predict strong vertical stratification with intense winds and super-rotating equatorial jets driven by radiative forcing and the transfer of heat and momentum.Recent models also predict a variety of storms (vortices) that can mix their atmospheres and transport hot and cold regions of gas.
• The day-night temperature difference at the photosphere is predicted to be substantial, approximately 500 K for a model based on HD 209458b.
• They appear to be more common around F- and G-type stars and less so around K-type stars. Hot Jupiters around red dwarfs are very rare. Generalizations about the distribution of these planets must take into account the various observational biases, but in general their prevalence decreases exponentially as a function of the absolute stellar magnitude.

Formation and evolution

There are two general schools of thought regarding the origin of hot Jupiters: formation at a distance followed by inward migration and in-situ formation at the distances at which they're currently observed. The prevalent view is formation via orbital migration.

Migration

In the migration hypothesis, a hot Jupiter forms beyond the frost line, from rock, ice, and gases via the core accretion method of planetary formation. The planet then migrates inwards to the star where it eventually forms a stable orbit. The planet may have migrated inward smoothly via type II orbital migration. Or it may have migrated more suddenly due to gravitational scattering onto eccentric orbits during an encounter with another massive planet, followed by the circularization and shrinking of the orbits due to tidal interactions with the star. A hot Jupiter's orbit could also have been altered via the Kozai mechanism, causing an exchange of inclination for eccentricity resulting in a high eccentricity low perihelion orbit, in combination with tidal friction. This requires a massive body—another planet or a stellar companion—on a more distant and inclined orbit; approximately 50% of hot Jupiters have distant Jupiter-mass or larger companions, which can leave the hot Jupiter with an orbit inclined relative to the star's rotation.

The type II migration happens during the solar nebula phase, i.e. when gas is still present. Energetic stellar photons and strong stellar winds at this time remove most of the remaining nebula. Migration via the other mechanism can happen after the loss of the gas disk.

In situ

Instead of being gas giants that migrated inward, in an alternate hypothesis the cores of the hot Jupiters began as more common super-Earths which accreted their gas envelopes at their current locations, becoming gas giants in situ. The super-Earths providing the cores in this hypothesis could have formed either in situ or at greater distances and have undergone migration before acquiring their gas envelopes. Since super-Earths are often found with companions, the hot Jupiters formed in situ could also be expected to have companions. The increase of the mass of the locally growing hot Jupiter has a number of possible effects on neighboring planets. If the hot Jupiter maintains an eccentricity greater than 0.01, sweeping secular resonances can increase the eccentricity of a companion planet, causing it to collide with the hot Jupiter. The core of the hot Jupiter in this case would be unusually large. If the hot Jupiter's eccentricity remains small the sweeping secular resonances could also tilt the orbit of the companion. Traditionally, the in situ mode of conglomeration has been disfavored because the assembly of massive cores, which is necessary for the formation of hot Jupiters, requires surface densities of solids ≈ 10⁴ g/cm², or larger. Recent surveys, however, have found that the inner regions of planetary systems are frequently occupied by super-Earth type planets. If these super-Earths formed at greater distances and migrated closer, the formation of in situ hot Jupiters is not entirely in situ.

Atmospheric loss

If the atmosphere of a hot Jupiter is stripped away via hydrodynamic escape, its core may become a chthonian planet. The amount of gas removed from the outermost layers depends on the planet's size, the gases forming the envelope, the orbital distance from the star, and the star's luminosity. In a typical system, a gas giant orbiting at 0.02 AU around its parent star loses 5–7% of its mass during its lifetime, but orbiting closer than 0.015 AU can mean evaporation of a substantially larger fraction of the planet's mass. No such objects have been found yet and they are still hypothetical.

Comparison of "hot Jupiter" exoplanets (artist concept).

From top left to lower right: WASP-12b, WASP-6b, WASP-31b, WASP-39b, HD 189733b, HAT-P-12b, WASP-17b, WASP-19b, HAT-P-1b and HD 209458b.

Terrestrial planets in systems with hot Jupiters

Simulations have shown that the migration of a Jupiter-sized planet through the inner protoplanetary disk (the region between 5 and 0.1 AU from the star) is not as destructive as expected. More than 60% of the solid disk materials in that region are scattered outward, including planetesimals and protoplanets, allowing the planet-forming disk to reform in the gas giant's wake. In the simulation, planets up to two Earth masses were able to form in the habitable zone after the hot Jupiter passed through and its orbit stabilized at 0.1 AU. Due to the mixing of inner-planetary-system material with outer-planetary-system material from beyond the frost line, simulations indicated that the terrestrial planets that formed after a hot Jupiter's passage would be particularly water-rich. According to a 2011 study, hot Jupiters may become disrupted planets while migrating inwards; this could explain an abundance of "hot" Earth-sized to Neptune-sized planets within 0.2 AU of their host star.

One example of these sorts of systems is that of WASP-47. There are three inner planets and an outer gas giant in the habitable zone. The innermost planet, WASP-47e, is a large terrestrial planet of 6.83 Earth masses and 1.8 Earth radii; the hot Jupiter, b, is little heavier than Jupiter, but about 12.63 Earth radii; a final hot Neptune, c, is 15.2 Earth masses and 3.6 Earth radii. A similar orbital architecture is also exhibited by the Kepler-30 system.

Retrograde orbit

It has been found that several hot Jupiters have retrograde orbits, in stark contrast to what would be expected from most theories on planetary formation, though it is possible that the star itself flipped over early in their system's formation due to interactions between the star's magnetic field and the planet-forming disc, rather than the planet's orbit being disturbed. By combining new observations with the old data it was found that more than half of all the hot Jupiters studied have orbits that are misaligned with the rotation axis of their parent stars, and six exoplanets in this study have retrograde motion.

Recent research has found that several hot Jupiters are in misaligned systems. This misalignment may be related to the heat of the photosphere the hot Jupiter is orbiting. There are many proposed theories as to why this might occur. One such theory involves tidal dissipation and suggests there is a single mechanism for producing hot Jupiters and this mechanism yields a range of obliquities. Cooler stars with higher tidal dissipation damps the obliquity (explaining why hot Jupiters orbiting cooler stars are well aligned) while hotter stars do not damp the obliquity (explaining the observed misalignment).

Ultra-hot Jupiters

Ultra-hot Jupiters are hot Jupiters with a dayside temperature greater than 2,200 K. In such dayside atmospheres, most molecules dissociate into their constituent atoms and circulate to the nightside where they recombine into molecules again.

One example is TOI-1431b, announced by the University of Southern Queensland in April 2021, which has an orbital period of just two and a half days. Its dayside temperature is 2,700 K (2,427 °C), making it hotter than 40% of stars in our galaxy. The nightside temperature is 2,600 K (2,300 °C).

Ultra-short period planets

Main article: Ultra-short period planet

Ultra-short period planets (USP) are a class of planets with orbital periods below one day and occur only around stars of less than about 1.25 solar masses.

Confirmed transiting hot Jupiters that have orbital periods of less than one day include WASP-18b, WASP-19b, WASP-43b, and WASP-103b.

Puffy planets

Gas giants with a large radius and very low density are sometimes called "puffy planets" or "hot Saturns", due to their density being similar to Saturn's. Puffy planets orbit close to their stars so that the intense heat from the star combined with internal heating within the planet will help inflate the atmosphere. Six large-radius low-density planets have been detected by the transit method. In order of discovery they are: HAT-P-1b, COROT-1b, TrES-4, WASP-12b, WASP-17b, and Kepler-7b. Some hot Jupiters detected by the radial-velocity method may be puffy planets. Most of these planets are around or below Jupiter mass as more massive planets have stronger gravity keeping them at roughly Jupiter's size. Indeed, hot Jupiters with masses below Jupiter, and temperatures above 1800 Kelvin, are so inflated and puffed out that they are all on unstable evolutionary paths which eventually lead to Roche-Lobe overflow and the evaporation and loss of the planet's atmosphere.

Even when taking surface heating from the star into account, many transiting hot Jupiters have a larger radius than expected. This could be caused by the interaction between atmospheric winds and the planet's magnetosphere creating an electric current through the planet that heats it up, causing it to expand. The hotter the planet, the greater the atmospheric ionization, and thus the greater the magnitude of the interaction and the larger the electric current, leading to more heating and expansion of the planet. This theory matches the observation that planetary temperature is correlated with inflated planetary radii.

Moons

Theoretical research suggests that hot Jupiters are unlikely to have moons, due to both a small Hill sphere and the tidal forces of the stars they orbit, which would destabilize any satellite's orbit, the latter process being stronger for larger moons. This means that for most hot Jupiters, stable satellites would be small asteroid-sized bodies. Furthermore, the physical evolution of hot Jupiters can determine the final fate of their moons: stall them in semi-asymptotic semimajor axes, or eject them from the system where they may undergo other unknown processes. In spite of this, observations of WASP-12b suggest that it is orbited by at least 1 large exomoon.

Hot Jupiters around red giants

It has been proposed that gas giants orbiting red giants at distances similar to that of Jupiter could be hot Jupiters due to the intense irradiation they would receive from their stars. It is very likely that in the Solar System Jupiter will become a hot Jupiter after the transformation of the Sun into a red giant. The recent discovery of particularly low density gas giants orbiting red giant stars supports this theory.

Hot Jupiters orbiting red giants would differ from those orbiting main-sequence stars in a number of ways, most notably the possibility of accreting material from the stellar winds of their stars and, assuming a fast rotation (not tidally locked to their stars), a much more evenly distributed heat with many narrow-banded jets. Their detection using the transit method would be much more difficult due to their tiny size compared to the stars they orbit, as well as the long time needed (months or even years) for one to transit their star as well as to be occulted by it.

Star-planet interactions

Theoretical research since 2000 suggested that "hot Jupiters" may cause increased flaring due to the interaction of the magnetic fields of the star and its orbiting exoplanet, or because of tidal forces between them. These effects are called "star-planet interactions" or SPIs. The HD 189733 system is the best-studied exoplanet system where this effect was thought to occur.

In 2008, a team of astronomers first described how as the exoplanet orbiting HD 189733 A reaches a certain place in its orbit, it causes increased stellar flaring. In 2010, a different team found that every time they observe the exoplanet at a certain position in its orbit, they also detected X-ray flares. In 2019, astronomers analyzed data from Arecibo Observatory, MOST, and the Automated Photoelectric Telescope, in addition to historical observations of the star at radio, optical, ultraviolet, and X-ray wavelengths to examine these claims. They found that the previous claims were exaggerated and the host star failed to display many of the brightness and spectral characteristics associated with stellar flaring and solar active regions, including sunspots. Their statistical analysis also found that many stellar flares are seen regardless of the position of the exoplanet, therefore debunking the earlier claims. The magnetic fields of the host star and exoplanet do not interact, and this system is no longer believed to have a "star-planet interaction." Some researchers had also suggested that HD 189733 accretes, or pulls, material from its orbiting exoplanet at a rate similar to those found around young protostars in T Tauri star systems. Later analysis demonstrated that very little, if any, gas was accreted from the "hot Jupiter" companion.

Hadrian's Wall

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Hadrian%27s_Wall 

Hadrian (/ˈheɪdriən/; Latin: Caesar Traianus Hadrianus [ˈkae̯s̠ar t̪rajˈjaːnʊs̠ (h)a.d̪riˈjaːnʊs̠]; 24 January 76 – 10 July 138) was Roman emperor from 117 to 138. He was born into a Roman Italo-Hispanic family that settled in Spain from the Italian city of Atri in Picenum. His father was of senatorial rank and was a first cousin of Emperor Trajan. Hadrian married Trajan's grand-niece Vibia Sabina early in his career, before Trajan became emperor and possibly at the behest of Trajan's wife Pompeia Plotina. Plotina and Trajan's close friend and adviser Lucius Licinius Sura were well disposed towards Hadrian. When Trajan died, his widow claimed that he had nominated Hadrian as emperor immediately before his death.

Rome's military and Senate approved Hadrian's succession, but four leading senators were unlawfully put to death soon after. They had opposed Hadrian or seemed to threaten his succession, and the Senate held him responsible for it and never forgave him. He earned further disapproval among the elite by abandoning Trajan's expansionist policies and territorial gains in Mesopotamia, Assyria, Armenia, and parts of Dacia. Hadrian preferred to invest in the development of stable, defensible borders and the unification of the empire's disparate peoples. He is known for building Hadrian's Wall, which marked the northern limit of Britannia.

Hadrian energetically pursued his own Imperial ideals and personal interests. He visited almost every province of the Empire, accompanied by an Imperial retinue of specialists and administrators. He encouraged military preparedness and discipline, and he fostered, designed, or personally subsidised various civil and religious institutions and building projects. In Rome itself, he rebuilt the Pantheon and constructed the vast Temple of Venus and Roma. In Egypt, he may have rebuilt the Serapeum of Alexandria. He was an ardent admirer of Greece and sought to make Athens the cultural capital of the Empire, so he ordered the construction of many opulent temples there. His intense relationship with Greek youth Antinous and the latter's untimely death led Hadrian to establish a widespread cult late in his reign. He suppressed the Bar Kokhba revolt in Judaea, but his reign was otherwise peaceful.

Hadrian's last years were marred by chronic illness. He saw the Bar Kokhba revolt as the failure of his panhellenic ideal. He executed two more senators for their alleged plots against him, and this provoked further resentment. His marriage to Vibia Sabina had been unhappy and childless; he adopted Antoninus Pius in 138 and nominated him as a successor, on the condition that Antoninus adopt Marcus Aurelius and Lucius Verus as his own heirs. Hadrian died the same year at Baiae, and Antoninus had him deified, despite opposition from the Senate. Edward Gibbon includes him among the Empire's "Five Good Emperors", a "benevolent dictator"; Hadrian's own Senate found him remote and authoritarian. He has been described as enigmatic and contradictory, with a capacity for both great personal generosity and extreme cruelty and driven by insatiable curiosity, self-conceit, and ambition.