From Wikipedia, the free encyclopedia
A
scientific theory is an explanation of an aspect of the
natural world that can be
repeatedly tested and verified in accordance with the
scientific method, using accepted
protocols of
observation, measurement, and evaluation of results. Where possible, theories are tested under controlled conditions in an
experiment. In circumstances not amenable to experimental testing, theories are evaluated through principles of
abductive reasoning. Established scientific theories have withstood rigorous scrutiny and embody scientific
knowledge.
The meaning of the term
scientific theory (often contracted to
theory for brevity) as used in the
disciplines of science is significantly different from the common
vernacular usage of
theory. In everyday speech,
theory can imply an explanation that represents an unsubstantiated and speculative
guess,
whereas in science it describes an explanation that has been tested and
widely accepted as valid. These different usages are comparable to the
opposing usages of
prediction in science versus common speech, where it denotes a mere hope.
The strength of a scientific theory is related to the diversity of phenomena it can explain and its simplicity. As additional
scientific evidence
is gathered, a scientific theory may be modified and ultimately
rejected if it cannot be made to fit the new findings; in such
circumstances, a more accurate theory is then required. That doesn’t
mean that all theories can be fundamentally changed (for example, well
established foundational scientific theories such as evolution,
heliocentric theory, cell theory, theory of plate tectonics etc). In
certain cases, the less-accurate unmodified scientific theory can still
be treated as a theory if it is useful (due to its sheer simplicity) as
an approximation under specific conditions. A case in point is
Newton's laws of motion, which can serve as an approximation to
special relativity at velocities that are small relative to the speed of light.
Scientific theories are
testable and make
falsifiable predictions. They describe the causes of a particular natural phenomenon and are used to explain and predict aspects of the physical
universe
or specific areas of inquiry (for example, electricity, chemistry, and
astronomy). Scientists use theories to further scientific knowledge, as
well as to facilitate advances in
technology or
medicine.
As with other forms of scientific knowledge, scientific theories are both
deductive and
inductive, aiming for
predictive and
explanatory power.
The
paleontologist Stephen Jay Gould
wrote that "...facts and theories are different things, not rungs in a
hierarchy of increasing certainty. Facts are the world's data. Theories
are structures of ideas that explain and interpret facts."
Types
Albert Einstein
described two types of scientific theories: "Constructive theories" and
"principle theories". Constructive theories are constructive models for
phenomena: for example,
kinetic energy. Principle theories are empirical generalisations such as Newton's laws of motion.
Characteristics
Essential criteria
Typically for any theory to be accepted within most academia there is
one simple criterion. The essential criterion is that the theory must
be observable and repeatable. The aforementioned criterion is essential
to prevent fraud and perpetuate science itself.
The
tectonic plates of the world were mapped in the second half of the 20th
century. Plate tectonic theory successfully explains numerous
observations about the Earth, including the distribution of earthquakes,
mountains, continents, and oceans.
The defining characteristic of all scientific knowledge, including theories, is the ability to make
falsifiable or testable
predictions.
The relevance and specificity of those predictions determine how
potentially useful the theory is. A would-be theory that makes no
observable predictions is not a scientific theory at all. Predictions
not sufficiently specific to be tested are similarly not useful. In both
cases, the term "theory" is not applicable.
A body of descriptions of
knowledge can be called a theory if it fulfills the following criteria:
- It makes falsifiable predictions with consistent accuracy across a broad area of scientific inquiry (such as mechanics).
- It is well-supported by many independent strands of evidence, rather than a single foundation.
- It is consistent with preexisting experimental results and at least
as accurate in its predictions as are any preexisting theories.
These qualities are certainly true of such established theories as
special and
general relativity,
quantum mechanics,
plate tectonics, the
modern evolutionary synthesis, etc.
Other criteria
In addition, scientists prefer to work with a theory that meets the following qualities:
- It can be subjected to minor adaptations to account for new data
that do not fit it perfectly, as they are discovered, thus increasing
its predictive capability over time.
- It is among the most parsimonious explanations, economical in the use of proposed entities or explanatory steps as per Occam's razor.
This is because for each accepted explanation of a phenomenon, there
may be an extremely large, perhaps even incomprehensible, number of
possible and more complex alternatives, because one can always burden
failing explanations with ad hoc hypotheses to prevent them from being falsified; therefore, simpler theories are preferable to more complex ones because they are more testable.
Definitions from scientific organizations
The
United States National Academy of Sciences defines scientific theories as follows:
The formal scientific definition of theory is quite
different from the everyday meaning of the word. It refers to a
comprehensive explanation of some aspect of nature that is supported by a
vast body of evidence. Many scientific theories are so well established
that no new evidence is likely to alter them substantially. For
example, no new evidence will demonstrate that the Earth does not orbit
around the sun (heliocentric theory), or that living things are not made
of cells (cell theory), that matter is not composed of atoms, or that
the surface of the Earth is not divided into solid plates that have
moved over geological timescales (the theory of plate tectonics)...One
of the most useful properties of scientific theories is that they can be
used to make predictions about natural events or phenomena that have
not yet been observed.
From the
American Association for the Advancement of Science:
A scientific theory is a well-substantiated explanation
of some aspect of the natural world, based on a body of facts that have
been repeatedly confirmed through observation and experiment. Such
fact-supported theories are not "guesses" but reliable accounts of the
real world. The theory of biological evolution is more than "just a
theory". It is as factual an explanation of the universe as the atomic
theory of matter or the germ theory of disease. Our understanding of
gravity is still a work in progress. But the phenomenon of gravity, like
evolution, is an accepted fact.
Note that the term
theory would not be appropriate for describing untested but intricate hypotheses or even
scientific models.
Formation
The
scientific method involves the proposal and testing of
hypotheses, by deriving
predictions
from the hypotheses about the results of future experiments, then
performing those experiments to see whether the predictions are valid.
This provides evidence either for or against the hypothesis. When enough
experimental results have been gathered in a particular area of
inquiry, scientists may propose an explanatory framework that accounts
for as many of these as possible. This explanation is also tested, and
if it fulfills the necessary criteria (see above), then the explanation
becomes a theory. This can take many years, as it can be difficult or
complicated to gather sufficient evidence.
Once all of the criteria have been met, it will be widely accepted by scientists (see
scientific consensus)
as the best available explanation of at least some phenomena. It will
have made predictions of phenomena that previous theories could not
explain or could not predict accurately, and it will have resisted
attempts at falsification. The strength of the evidence is evaluated by
the scientific community, and the most important experiments will have
been replicated by multiple independent groups.
Theories do not have to be perfectly accurate to be scientifically useful. For example, the predictions made by
classical mechanics
are known to be inaccurate in the relatistivic realm, but they are
almost exactly correct at the comparatively low velocities of common
human experience. In
chemistry, there are many
acid-base theories
providing highly divergent explanations of the underlying nature of
acidic and basic compounds, but they are very useful for predicting
their chemical behavior. Like all knowledge in science, no theory can ever be completely
certain, since it is possible that future experiments might conflict with the theory's predictions.
However, theories supported by the scientific consensus have the
highest level of certainty of any scientific knowledge; for example,
that all objects are subject to
gravity or that life on Earth
evolved from a
common ancestor.
Acceptance of a theory does not require that all of its major
predictions be tested, if it is already supported by sufficiently strong
evidence. For example, certain tests may be unfeasible or technically
difficult. As a result, theories may make predictions that have not yet
been confirmed or proven incorrect; in this case, the predicted results
may be described informally with the term "theoretical". These
predictions can be tested at a later time, and if they are incorrect,
this may lead to the revision or rejection of the theory.
Modification and improvement
If
experimental results contrary to a theory's predictions are observed,
scientists first evaluate whether the experimental design was sound, and
if so they confirm the results by independent
replication.
A search for potential improvements to the theory then begins.
Solutions may require minor or major changes to the theory, or none at
all if a satisfactory explanation is found within the theory's existing
framework.
Over time, as successive modifications build on top of each other,
theories consistently improve and greater predictive accuracy is
achieved. Since each new version of a theory (or a completely new
theory) must have more predictive and explanatory power than the last,
scientific knowledge consistently becomes more accurate over time.
If modifications to the theory or other explanations seem to be
insufficient to account for the new results, then a new theory may be
required. Since scientific knowledge is usually durable, this occurs
much less commonly than modification.
Furthermore, until such a theory is proposed and accepted, the previous
theory will be retained. This is because it is still the best available
explanation for many other phenomena, as verified by its predictive
power in other contexts. For example, it has been known since 1859 that
the observed
perihelion precession of Mercury violates Newtonian mechanics, but the theory remained the best explanation available until
relativity
was supported by sufficient evidence. Also, while new theories may be
proposed by a single person or by many, the cycle of modifications
eventually incorporates contributions from many different scientists.
After the changes, the accepted theory will explain more
phenomena and have greater predictive power (if it did not, the changes
would not be adopted); this new explanation will then be open to further
replacement or modification. If a theory does not require modification
despite repeated tests, this implies that the theory is very accurate.
This also means that accepted theories continue to accumulate evidence
over time, and the length of time that a theory (or any of its
principles) remains accepted often indicates the strength of its
supporting evidence.
Unification
In some cases, two or more theories may be replaced by a single
theory that explains the previous theories as approximations or special
cases, analogous to the way a theory is a unifying explanation for many
confirmed hypotheses; this is referred to as
unification of theories. For example,
electricity and
magnetism are now known to be two aspects of the same phenomenon, referred to as
electromagnetism.
When the predictions of different theories appear to contradict
each other, this is also resolved by either further evidence or
unification. For example, physical theories in the 19th century implied
that the
Sun could not have been burning long enough to allow certain geological changes as well as the
evolution of life. This was resolved by the discovery of
nuclear fusion, the main energy source of the Sun.
Contradictions can also be explained as the result of theories
approximating more fundamental (non-contradictory) phenomena. For
example,
atomic theory is an approximation of
quantum mechanics. Current theories describe three separate
fundamental phenomena of which all other theories are approximations; the potential unification of these is sometimes called the
Theory of Everything.
Example: Relativity
In 1905,
Albert Einstein published the principle of
special relativity, which soon became a theory. Special relativity predicted the alignment of the Newtonian principle of
Galilean invariance, also termed
Galilean relativity, with the electromagnetic field. By omitting from special relativity the
luminiferous aether, Einstein stated that
time dilation and
length contraction measured in an object in relative motion is
inertial—that is, the object exhibits constant
velocity, which is
speed with
direction, when measured by its observer. He thereby duplicated the
Lorentz transformation and the
Lorentz contraction
that had been hypothesized to resolve experimental riddles and inserted
into electrodynamic theory as dynamical consequences of the aether's
properties. An elegant theory, special relativity yielded its own
consequences, such as the
equivalence of mass and energy transforming into one another
and the resolution of the paradox that an excitation of the
electromagnetic field could be viewed in one reference frame as
electricity, but in another as magnetism.
Einstein sought to generalize the invariance principle to all reference frames, whether inertial or accelerating. Rejecting Newtonian gravitation—a
central force acting instantly at a distance—Einstein presumed a gravitational field. In 1907, Einstein's
equivalence principle implied that a free fall within a uniform gravitational field is equivalent to
inertial motion. By extending special relativity's effects into three dimensions,
general relativity extended length contraction into
space contraction,
conceiving of 4D space-time as the gravitational field that alters
geometrically and sets all local objects' pathways. Even massless
energy exerts gravitational motion on local objects by "curving" the
geometrical "surface" of 4D space-time. Yet unless the energy is vast,
its relativistic effects of contracting space and slowing time are
negligible when merely predicting motion. Although general relativity is
embraced as the more explanatory theory via
scientific realism, Newton's theory remains successful as merely a predictive theory via
instrumentalism. To calculate trajectories, engineers and NASA still uses Newton's equations, which are simpler to operate.
Theories and laws
Both scientific laws and scientific theories are produced from the
scientific method through the formation and testing of hypotheses, and
can predict the behavior of the natural world. Both are typically
well-supported by observations and/or experimental evidence. However, scientific laws are descriptive accounts of how nature will behave under certain conditions.
Scientific theories are broader in scope, and give overarching
explanations of how nature works and why it exhibits certain
characteristics. Theories are supported by evidence from many different
sources, and may contain one or several laws.
A common misconception is that scientific theories are
rudimentary ideas that will eventually graduate into scientific laws
when enough data and evidence have been accumulated. A theory does not
change into a scientific law with the accumulation of new or better
evidence. A theory will always remain a theory; a law will always remain
a law. Both theories and laws could potentially be falsified by countervailing evidence.
Theories and laws are also distinct from
hypotheses. Unlike hypotheses, theories and laws may be simply referred to as
scientific fact.
However, in science, theories are different from facts even when they are well supported. For example,
evolution is both a
theory and a fact.
About theories
Theories as axioms
The
logical positivists thought of scientific theories as statements in a
formal language.
First-order logic is an example of a formal language. The logical
positivists envisaged a similar scientific language. In addition to
scientific theories, the language also included observation sentences
("the sun rises in the east"), definitions, and mathematical statements.
The phenomena explained by the theories, if they could not be directly
observed by the senses (for example,
atoms and
radio waves), were treated as theoretical concepts. In this view, theories function as
axioms: predicted observations are derived from the theories much like
theorems are derived in
Euclidean geometry.
However, the predictions are then tested against reality to verify the
theories, and the "axioms" can be revised as a direct result.
The phrase "
the received view of theories" is used to describe this approach. Terms commonly associated with it are "
linguistic" (because theories are components of a language) and "
syntactic"
(because a language has rules about how symbols can be strung
together). Problems in defining this kind of language precisely, e.g.,
are objects seen in microscopes observed or are they theoretical
objects, led to the effective demise of logical positivism in the 1970s.
Theories as models
The
semantic view of theories, which identifies scientific theories with
models rather than
propositions, has replaced the received view as the dominant position in theory formulation in the philosophy of science.
A model is a logical framework intended to represent reality (a "model
of reality"), similar to the way that a map is a graphical model that
represents the territory of a city or country.
In this approach, theories are a specific category of models that fulfill the necessary criteria (see
above).
One can use language to describe a model; however, the theory is the
model (or a collection of similar models), and not the description of
the model. A model of the solar system, for example, might consist of
abstract objects that represent the sun and the planets. These objects
have associated properties, e.g., positions, velocities, and masses. The
model parameters, e.g., Newton's Law of Gravitation, determine how the
positions and velocities change with time. This model can then be tested
to see whether it accurately predicts future observations; astronomers
can verify that the positions of the model's objects over time match the
actual positions of the planets. For most planets, the Newtonian
model's predictions are accurate; for
Mercury, it is slightly inaccurate and the model of
general relativity must be used instead.
The word "
semantic"
refers to the way that a model represents the real world. The
representation (literally, "re-presentation") describes particular
aspects of a phenomenon or the manner of interaction among a set of
phenomena. For instance, a scale model of a house or of a solar system
is clearly not an actual house or an actual solar system; the aspects of
an actual house or an actual solar system represented in a scale model
are, only in certain limited ways, representative of the actual entity. A
scale model of a house is not a house; but to someone who wants to
learn about houses, analogous to a scientist who wants to understand reality, a sufficiently detailed scale model may suffice.
Differences between theory and model
Several commentators
have stated that the distinguishing characteristic of theories is that
they are explanatory as well as descriptive, while models are only
descriptive (although still predictive in a more limited sense).
Philosopher
Stephen Pepper
also distinguished between theories and models, and said in 1948 that
general models and theories are predicated on a "root" metaphor that
constrains how scientists theorize and model a phenomenon and thus
arrive at testable hypotheses.
Engineering practice makes a distinction between "mathematical
models" and "physical models"; the cost of fabricating a physical model
can be minimized by first creating a mathematical model using a computer
software package, such as a
computer aided design tool. The component parts are each themselves modelled, and the fabrication tolerances are specified. An
exploded view drawing
is used to lay out the fabrication sequence. Simulation packages for
displaying each of the subassemblies allow the parts to be rotated,
magnified, in realistic detail. Software packages for creating the bill
of materials for construction allows subcontractors to specialize in
assembly processes, which spreads the cost of manufacturing machinery
among multiple customers. See:
Computer-aided engineering,
Computer-aided manufacturing, and
3D printing
Assumptions in formulating theories
An assumption (or
axiom) is a statement that is accepted without evidence. For example, assumptions can be used as premises in a logical argument.
Isaac Asimov described assumptions as follows:
...it is incorrect to speak of an assumption as either
true or false, since there is no way of proving it to be either (If
there were, it would no longer be an assumption). It is better to
consider assumptions as either useful or useless, depending on whether
deductions made from them corresponded to reality...Since we must start
somewhere, we must have assumptions, but at least let us have as few
assumptions as possible.
Certain assumptions are necessary for all empirical claims (e.g. the assumption that
reality
exists). However, theories do not generally make assumptions in the
conventional sense (statements accepted without evidence). While
assumptions are often incorporated during the formation of new theories,
these are either supported by evidence (such as from previously
existing theories) or the evidence is produced in the course of
validating the theory. This may be as simple as observing that the
theory makes accurate predictions, which is evidence that any
assumptions made at the outset are correct or approximately correct
under the conditions tested.
Conventional assumptions, without evidence, may be used if the
theory is only intended to apply when the assumption is valid (or
approximately valid). For example, the
special theory of relativity assumes an
inertial frame of reference.
The theory makes accurate predictions when the assumption is valid, and
does not make accurate predictions when the assumption is not valid.
Such assumptions are often the point with which older theories are
succeeded by new ones (the
general theory of relativity works in non-inertial reference frames as well).
The term "assumption" is actually broader than its standard use,
etymologically speaking. The Oxford English Dictionary (OED) and online
Wiktionary indicate its Latin source as
assumere ("accept, to take to oneself, adopt, usurp"), which is a conjunction of
ad- ("to, towards, at") and
sumere (to take). The root survives, with shifted meanings, in the Italian
assumere and Spanish
sumir.
The first sense of "assume" in the OED is "to take unto (oneself),
receive, accept, adopt". The term was originally employed in religious
contexts as in "to receive up into heaven", especially "the reception of
the Virgin Mary into heaven, with body preserved from corruption",
(1297 CE) but it was also simply used to refer to "receive into
association" or "adopt into partnership". Moreover, other senses of
assumere included (i) "investing oneself with (an attribute)", (ii) "to
undertake" (especially in Law), (iii) "to take to oneself in appearance
only, to pretend to possess", and (iv) "to suppose a thing to be" (all
senses from OED entry on "assume"; the OED entry for "assumption" is
almost perfectly symmetrical in senses). Thus, "assumption" connotes
other associations than the contemporary standard sense of "that which
is assumed or taken for granted; a supposition, postulate" (only the
11th of 12 senses of "assumption", and the 10th of 11 senses of
"assume").
Descriptions
From philosophers of science
Karl Popper described the characteristics of a scientific theory as follows:
- It is easy to obtain confirmations, or verifications, for nearly every theory—if we look for confirmations.
- Confirmations should count only if they are the result of risky
predictions; that is to say, if, unenlightened by the theory in
question, we should have expected an event which was incompatible with
the theory—an event which would have refuted the theory.
- Every "good" scientific theory is a prohibition: it forbids certain
things to happen. The more a theory forbids, the better it is.
- A theory which is not refutable by any conceivable event is
non-scientific. Irrefutability is not a virtue of a theory (as people
often think) but a vice.
- Every genuine test of a theory is an attempt to falsify it, or to
refute it. Testability is falsifiability; but there are degrees of
testability: some theories are more testable, more exposed to
refutation, than others; they take, as it were, greater risks.
- Confirming evidence should not count except when it is the result of
a genuine test of the theory; and this means that it can be presented
as a serious but unsuccessful attempt to falsify the theory. (I now
speak in such cases of "corroborating evidence".)
- Some genuinely testable theories, when found to be false, might
still be upheld by their admirers—for example by introducing post hoc
(after the fact) some auxiliary hypothesis
or assumption, or by reinterpreting the theory post hoc in such a way
that it escapes refutation. Such a procedure is always possible, but it
rescues the theory from refutation only at the price of destroying, or
at least lowering, its scientific status, by tampering with evidence.
The temptation to tamper can be minimized by first taking the time to
write down the testing protocol before embarking on the scientific work.
Popper summarized these statements by saying that the central
criterion of the scientific status of a theory is its "falsifiability,
or refutability, or testability". Echoing this,
Stephen Hawking
states, "A theory is a good theory if it satisfies two requirements: It
must accurately describe a large class of observations on the basis of a
model that contains only a few arbitrary elements, and it must make
definite predictions about the results of future observations." He also
discusses the "unprovable but falsifiable" nature of theories, which is a
necessary consequence of inductive logic, and that "you can disprove a
theory by finding even a single observation that disagrees with the
predictions of the theory".
Several philosophers and historians of science have, however,
argued that Popper's definition of theory as a set of falsifiable
statements is wrong because, as
Philip Kitcher
has pointed out, if one took a strictly Popperian view of "theory",
observations of Uranus when first discovered in 1781 would have
"falsified" Newton's celestial mechanics. Rather, people suggested that
another planet influenced Uranus' orbit—and this prediction was indeed
eventually confirmed.
Kitcher agrees with Popper that "There is surely something right in the idea that a science can succeed only if it can fail."
He also says that scientific theories include statements that cannot be
falsified, and that good theories must also be creative. He insists we
view scientific theories as an "elaborate collection of statements",
some of which are not falsifiable, while others—those he calls
"auxiliary hypotheses", are.
According to Kitcher, good scientific theories must have three features:
- Unity: "A science should be unified…. Good theories consist of
just one problem-solving strategy, or a small family of problem-solving
strategies, that can be applied to a wide range of problems."
- Fecundity:
"A great scientific theory, like Newton's, opens up new areas of
research…. Because a theory presents a new way of looking at the world,
it can lead us to ask new questions, and so to embark on new and
fruitful lines of inquiry…. Typically, a flourishing science is
incomplete. At any time, it raises more questions than it can currently
answer. But incompleteness is not vice. On the contrary, incompleteness
is the mother of fecundity…. A good theory should be productive; it
should raise new questions and presume those questions can be answered
without giving up its problem-solving strategies."
- Auxiliary hypotheses
that are independently testable: "An auxiliary hypothesis ought to be
testable independently of the particular problem it is introduced to
solve, independently of the theory it is designed to save." (For
example, the evidence for the existence of Neptune is independent of the
anomalies in Uranus's orbit.)
Like other definitions of theories, including Popper's, Kitcher makes
it clear that a theory must include statements that have observational
consequences. But, like the observation of irregularities in the orbit
of Uranus, falsification is only one possible consequence of
observation. The production of new hypotheses is another possible and
equally important result.
Analogies and metaphors
The concept of a scientific theory has also been described using analogies and metaphors. For instance, the logical empiricist
Carl Gustav Hempel likened the structure of a scientific theory to a "complex spatial network:"
Its terms are represented by the knots, while the
threads connecting the latter correspond, in part, to the definitions
and, in part, to the fundamental and derivative hypotheses included in
the theory. The whole system floats, as it were, above the plane of
observation and is anchored to it by the rules of interpretation. These
might be viewed as strings which are not part of the network but link
certain points of the latter with specific places in the plane of
observation. By virtue of these interpretive connections, the network
can function as a scientific theory: From certain observational data, we
may ascend, via an interpretive string, to some point in the
theoretical network, thence proceed, via definitions and hypotheses, to
other points, from which another interpretive string permits a descent
to the plane of observation.
Michael Polanyi made an analogy between a theory and a map:
A theory is something other than myself. It may be set
out on paper as a system of rules, and it is the more truly a theory the
more completely it can be put down in such terms. Mathematical theory
reaches the highest perfection in this respect. But even a geographical
map fully embodies in itself a set of strict rules for finding one's way
through a region of otherwise uncharted experience. Indeed, all theory
may be regarded as a kind of map extended over space and time.
A scientific theory can also be thought of as a book that captures
the fundamental information about the world, a book that must be
researched, written, and shared. In 1623,
Galileo Galilei wrote:
Philosophy [i.e. physics] is written in this grand book—I
mean the universe—which stands continually open to our gaze, but it
cannot be understood unless one first learns to comprehend the language
and interpret the characters in which it is written. It is written in
the language of mathematics, and its characters are triangles, circles,
and other geometrical figures, without which it is humanly impossible to
understand a single word of it; without these, one is wandering around
in a dark labyrinth.
The book metaphor could also be applied in the following passage, by the contemporary philosopher of science
Ian Hacking:
I myself prefer an Argentine fantasy. God did not write a
Book of Nature of the sort that the old Europeans imagined. He wrote a
Borgesian library, each book of which is as brief as possible, yet each
book of which is inconsistent with every other. No book is redundant.
For every book there is some humanly accessible bit of Nature such that
that book, and no other, makes possible the comprehension, prediction
and influencing of what is going on…Leibniz said that God chose a world
which maximized the variety of phenomena while choosing the simplest
laws. Exactly so: but the best way to maximize phenomena and have
simplest laws is to have the laws inconsistent with each other, each
applying to this or that but none applying to all.
In physics
In
physics, the term
theory is generally used for a mathematical framework—derived from a small set of basic
postulates
(usually symmetries—like equality of locations in space or in time, or
identity of electrons, etc.)—that is capable of producing experimental
predictions for a given category of physical systems. A good example is
classical electromagnetism, which encompasses results derived from
gauge symmetry (sometimes called
gauge invariance) in a form of a few equations called
Maxwell's equations.
The specific mathematical aspects of classical electromagnetic theory
are termed "laws of electromagnetism," reflecting the level of
consistent and reproducible evidence that supports them. Within
electromagnetic theory generally, there are numerous hypotheses about
how electromagnetism applies to specific situations. Many of these
hypotheses are already considered to be adequately tested, with new ones
always in the making and perhaps untested. An example of the latter
might be the
radiation reaction force. As of 2009, its effects on the periodic motion of charges are detectable in
synchrotrons, but only as
averaged
effects over time. Some researchers are now considering experiments
that could observe these effects at the instantaneous level (i.e. not
averaged over time).
Examples
Note that many fields of inquiry do not have specific named theories, e.g.
developmental biology.
Scientific knowledge outside a named theory can still have a high level
of certainty, depending on the amount of evidence supporting it. Also
note that since theories draw evidence from many different fields, the
categorization is not absolute.
- Biology: cell theory, theory of evolution (modern evolutionary synthesis), germ theory, particulate inheritance theory, dual inheritance theory
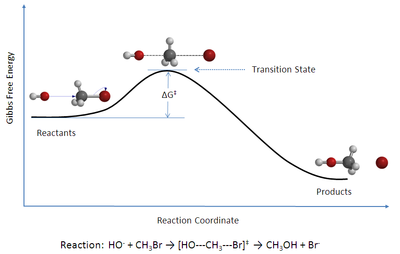
- Chemistry: collision theory, kinetic theory of gases, Lewis theory, molecular theory, molecular orbital theory, transition state theory, valence bond theory
- Physics: atomic theory, Big Bang theory, Dynamo theory, perturbation theory, theory of relativity (successor to classical mechanics), quantum field theory
- Other: Climate change theory (from climatology),[56] plate tectonics theory (from geology), theories of the origin of the Moon, theories for the Moon illusion






![{\displaystyle {\ce {AB <=>[k_1][k_{-1}] {A}+ {B}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8df558c371c7f125f5833608e30f847abe2601de)


![{\displaystyle {\ce {{A}+{B}<=>{[AB]^{\ddagger }}->{P}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0b873373ba74f1671f87574af29e3a0a9ba9c63d)

![{\displaystyle [{\ce {AB}}_{r}]^{\ddagger }=[{\ce {AB}}_{l}]^{\ddagger }={\frac {1}{2}}[{\ce {AB}}]^{\ddagger }}](https://wikimedia.org/api/rest_v1/media/math/render/svg/90abaaa14ee8a77731a50367fab640c94185a043)
![{\displaystyle K^{\ddagger \ominus }={\frac {\ce {[AB]^{\ddagger }}}{\ce {[A][B]}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/87608a9f0dbd11223a1722caf645739b4400b8b4)
![{\displaystyle [{\ce {AB}}]^{\ddagger }=K^{\ddagger \ominus }[{\ce {A}}][{\ce {B}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6116d9ee717d297370d39b5b918d1e5d790ff3d4)
![{\displaystyle {\frac {d[{\ce {P}}]}{dt}}=k^{\ddagger \ominus }[{\ce {AB}}]^{\ddagger }=k^{\ddagger }K^{\ddagger }[{\ce {A}}][{\ce {B}}]=k[{\ce {A}}][{\ce {B}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3e57c85cb84cdb2c75e3d0cfb8599f7f0d0230c0)














