Möbius strip

From Wikipedia, the free encyclopedia

 

A Möbius strip made with a piece of paper and tape. If its full length were crawled by an ant, the ant would return to its starting point having traversed both sides of the paper without ever crossing an edge.

 

A ray-traced parametric plot of a Möbius strip.

 

A Möbius strip does not self-intersect, but its projection in 2 dimensions does.

In mathematics, a Möbius strip, band, or loop (US: /ˈmoʊbiəs, ˈmeɪ-/ MOH-bee-əs, MAY-, UK: /ˈmɜːbiəs/; German: [ˈmøːbi̯ʊs]), also spelled Mobius or Moebius, is a surface with only one side (when embedded in three-dimensional Euclidean space) and only one boundary curve. The Möbius strip is the simplest non-orientable surface. It can be realized as a ruled surface. Its discovery is attributed independently to the German mathematicians Johann Benedict Listing and August Ferdinand Möbius in 1858, though similar structures can be seen in Roman mosaics c. 200–250 AD. Möbius published his results in his articles "Theorie der elementaren Verwandtschaft" (1863) and "Ueber die Bestimmung des Inhaltes eines Polyëders" (1865).

An example of a Möbius strip can be created by taking a strip of paper and giving one end a half-twist, then joining the ends to form a loop; its boundary is a simple closed curve which can be traced by a single unknotted string. Any topological space homeomorphic to this example is also called a Möbius strip, allowing for a very wide variety of geometric realizations as surfaces with a definite size and shape. For example, any rectangle can be glued left-edge to right-edge with a reversal of orientation. Some, but not all, of these can be smoothly modeled as surfaces in Euclidean space. A closely related, but not homeomorphic, surface is the complete open Möbius band, a surface with no boundaries in which the width of the strip is extended infinitely to become a Euclidean line.

A half-twist clockwise gives an embedding of the Möbius strip which cannot be moved or stretched to give the half-twist counterclockwise; thus, a Möbius strip embedded in Euclidean space is a chiral object with right- or left-handedness. The Möbius strip can also be embedded by twisting the strip any odd number of times, or by knotting and twisting the strip before joining its ends.

Finding algebraic equations cutting out a Möbius strip is straightforward, but these equations do not describe the same geometric shape as the twisted paper model above. Such paper models are developable surfaces having zero Gaussian curvature, and can be described by differential-algebraic equations.

The Euler characteristic of the Möbius strip is zero.

Properties

Once cut Möbius strip: one non Möbius strip

twice cut Möbius strip: one Möbius strip (purple), one non Möbius strip

The Möbius strip has several curious properties. A line drawn along the edge travels in a full circle to a point opposite the starting point. If continued, the line returns to the starting point, and is double the length of the original strip: this single continuous curve traverses the entire boundary.

Cutting a Möbius strip along the center line with a pair of scissors yields one long strip with two full twists in it, rather than two separate strips; the result is not a Möbius strip, but homeomorphic to a cylinder. This happens because the original strip only has one edge, twice as long as the original strip. Cutting creates a second independent edge of the same length, half on each side of the scissors. Cutting this new, longer, strip down the middle creates two strips wound around each other, each with two full twists.

If the strip is cut along about a third in from the edge, it creates two linked strips. The center third is a thinner Möbius strip, the same length as the original strip. The other is a thin strip with two full twists, a neighborhood of the edge of the original strip, with twice the length of the original strip.

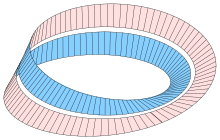
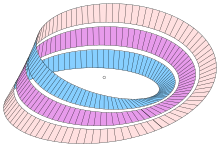
Other analogous strips can be obtained by similarly joining strips with two or more half-twists in them instead of one. For example, a strip with three half-twists, when divided lengthwise, becomes a twisted strip tied in a trefoil knot; if this knot is unraveled, it is found to contain eight half-twists. A strip with N half-twists, when bisected, becomes a strip with N + 1 full twists. Giving it extra twists and reconnecting the ends produces figures called Paradromic Rings.

Geometry and topology

An object that existed in a mobius-strip-shaped universe would be indistinguishable from its own mirror image – this fiddler crab's larger claw switches between left to right with every circulation. It is not impossible that the universe may have this property; see non-orientable wormhole

One way to represent the Möbius strip embedded in three-dimensional Euclidean space is by the parameterization:

$${\displaystyle {\begin{aligned}x(u,v)&=\left(1+{\frac {v}{2}}\cos {\frac {u}{2}}\right)\cos u\\y(u,v)&=\left(1+{\frac {v}{2}}\cos {\frac {u}{2}}\right)\sin u\\z(u,v)&={\frac {v}{2}}\sin {\frac {u}{2}}\\\end{aligned}}}$$

for ${\displaystyle 0\leq u<2\pi }$ and ${\displaystyle -1\leq v\leq 1}$. This creates a Möbius strip of width 1, whose center circle has radius 1, lies in the ${\displaystyle xy}$-plane and is centered at ${\displaystyle (0,0,0)}$. The parameter u runs around the strip while v moves from one edge to the other.

In cylindrical polar coordinates ${\displaystyle (r,\theta ,z)}$, an unbounded version of the Möbius strip can be represented by the equation:

$${\displaystyle \log(r)\sin \left({\tfrac {1}{2}}\theta \right)=z\cos \left({\tfrac {1}{2}}\theta \right).}$$

Widest isometric embedding in 3-space

If a smooth Möbius strip in three-space is a rectangular one – that is, created from identifying two opposite sides of a geometrical rectangle with bending but not stretching the surface – then such an embedding is known to be possible if the aspect ratio of the rectangle is greater than ${\displaystyle {\sqrt {3}}}$, with the shorter sides identified. (For a smaller aspect ratio, it is not known whether a smooth embedding is possible.) As the aspect ratio decreases toward ${\displaystyle {\sqrt {3}}}$, any such embedding seems to approach a shape that can be thought of as a strip of three equilateral triangles, folded on top of one another to occupy an equilateral triangle.

If the Möbius strip in three-space is only once continuously differentiable (class ${\displaystyle C_{1}}$), however, then the theorem of Nash-Kuiper shows that no lower bound exists.

A method of making a Möbius strip from a rectangular strip too wide to simply twist and join (e.g., a rectangle only one unit long and one unit wide) is to first fold the wide direction back and forth using an even number of folds—an "accordion fold"—so that the folded strip becomes narrow enough that it can be twisted and joined, much as a single long-enough strip can be joined. With two folds, for example, a ${\displaystyle 1\times 1}$ strip would become a ${\displaystyle 1\times {\tfrac {1}{3}}}$ folded strip whose cross section is in the shape of an 'N' and would remain an 'N' after a half-twist. This folded strip, three times as long as it is wide, would be long enough to then join at the ends. This method works in principle, but becomes impractical after sufficiently many folds, if paper is used. Using normal paper, this construction can be folded flat, with all the layers of the paper in a single plane, but mathematically, whether this is possible without stretching the surface of the rectangle is not clear.

Topology

To turn a rectangle into a Möbius strip, join the edges labelled A so that the directions of the arrows match.

Topologically, the Möbius strip can be defined as the square ${\displaystyle [0,1]\times [0,1]}$ with its top and bottom sides identified by the relation ${\displaystyle (x,0)\sim (1-x,1)}$ for ${\displaystyle 0\leq x\leq 1}$, as in the diagram.

A less used presentation of the Möbius strip is as the topological quotient of a torus. A torus can be constructed as the square ${\displaystyle [0,1]\times [0,1]}$ with the edges identified as ${\displaystyle (0,y)\sim (1,y)}$ (glue left to right) and ${\displaystyle (x,0)\sim (x,1)}$ (glue bottom to top). If one then also identified ${\displaystyle (x,y)\sim (y,x)}$, then one obtains the Möbius strip. The diagonal of the square (the points ${\displaystyle (x,x)}$ where both coordinates agree) becomes the boundary of the Möbius strip, and carries an orbifold structure, which geometrically corresponds to "reflection" – geodesics (straight lines) in the Möbius strip reflect off the edge back into the strip. Notationally, this is written as ${\displaystyle T^{2}/S^{2}}$ – the 2-torus quotiented by the group action of the symmetric group on two letters (switching coordinates), and it can be thought of as the configuration space of two unordered points on the circle, possibly the same (the edge corresponds to the points being the same), with the torus corresponding to two ordered points on the circle.

The Möbius strip is a two-dimensional compact manifold (i.e. a surface) with boundary. It is a standard example of a surface that is not orientable. In fact, the Möbius strip is the epitome of the topological phenomenon of nonorientability. This is because two-dimensional shapes (surfaces) are the lowest-dimensional shapes for which nonorientability is possible and the Möbius strip is the only surface that is topologically a subspace of every nonorientable surface. As a result, any surface is nonorientable if and only if it contains a Möbius band as a subspace.

The Möbius strip is also a standard example used to illustrate the mathematical concept of a fiber bundle. Specifically, it is a nontrivial bundle over the circle ${\displaystyle S^{1}}$ with its fiber equal to the unit interval, ${\displaystyle I=[0,1]}$. Looking only at the edge of the Möbius strip gives a nontrivial two point (or ${\displaystyle \mathbb {Z} _{2}}$) bundle over ${\displaystyle S^{1}}$.

Computer graphics

A simple construction of the Möbius strip that can be used to portray it in computer graphics or modeling packages is:

• Take a rectangular strip. Rotate it around a fixed point not in its plane. At every step, also rotate the strip along a line in its plane (the line that divides the strip in two) and perpendicular to the main orbital radius. The surface generated on one complete revolution is the Möbius strip.
• Take a Möbius strip and cut it along the middle of the strip. This forms a new strip, which is a rectangle joined by rotating one end a whole turn. By cutting it down the middle again, this forms two interlocking whole-turn strips.

Geometry of the open Möbius band

The open Möbius band is formed by deleting the boundary of the standard Möbius band. It is constructed from the set ${\displaystyle S=\{(x,y)\in \mathbb {R} ^{2}\mid 0\leq x\leq 1,0<y<1\}}$ by identifying (glueing) the points ${\displaystyle (0,y)}$ and ${\displaystyle (1,1-y)}$ for all ${\displaystyle 0<y<1}$.

It may be constructed as a surface of constant positive, negative, or zero (Gaussian) curvature. In the cases of negative and zero curvature, the Möbius band can be constructed as a (geodesically) complete surface, which means that all geodesics ("straight lines" on the surface) may be extended indefinitely in either direction.

Constant negative curvature:

Like the plane and the open cylinder, the open Möbius band admits not only a complete metric of constant curvature 0, but also a complete metric of constant negative curvature. One way to see this is to begin with the upper half plane (Poincaré) model of the hyperbolic plane, a geometry of constant curvature whose lines are represented in the model by semicircles that meet the ${\displaystyle x}$-axis at right angles. Take the subset of the upper half-plane between two nested semicircles, and identify the outer semicircle with the left-right reversal of the inner semicircle. The result is topologically a complete and non-compact Möbius band with constant negative curvature.

(Constant) zero curvature:

This may also be constructed as a complete surface, by starting with portion of the plane ${\displaystyle \mathbb {R} ^{2}}$ defined by ${\displaystyle 0\leq y\leq 1}$ and identifying ${\displaystyle (x,0)}$ with ${\displaystyle (-x,1)}$ for all ${\displaystyle x\in \mathbb {R} }$. The resulting metric makes the open Möbius band into a (geodesically) complete flat surface (i.e., having Gaussian curvature equal to 0 everywhere). This is the only metric on the Möbius band, up to uniform scaling, that is both flat and complete.

Constant positive curvature:

A Möbius band of constant positive curvature cannot be complete, since it is known that the only complete surfaces of constant positive curvature are the sphere and the projective plane. The projective plane ${\displaystyle \mathbb {P} ^{2}}$ of constant curvature +1 may be constructed as the quotient of the unit sphere ${\displaystyle S^{2}}$ in ${\displaystyle \mathbb {R} ^{3}}$ by the antipodal map ${\displaystyle A:S^{2}\to S^{2}}$ defined by ${\displaystyle A(x,y,z)=(-x,-y,-z)}$. The open Möbius band is homeomorphic to the once-punctured projective plane, that is, ${\displaystyle \mathbb {P} ^{2}}$ with any one point removed. This may be thought of as the closest that a Möbius band of constant positive curvature can get to being a complete surface: just one point away.

The space of unoriented lines in the plane is diffeomorphic to the open Möbius band. To see why, let ${\displaystyle L(\theta )}$ denote the line through the origin at an angle ${\displaystyle \theta }$ to the positive ${\displaystyle x}$-axis. For each ${\displaystyle L(\theta )}$ there is the family ${\displaystyle P(\theta )}$ of all lines in the plane that are perpendicular to ${\displaystyle L(\theta )}$. Topologically, the family ${\displaystyle P(\theta )}$ is just a line (because each line in ${\displaystyle P(\theta )}$ intersects the line ${\displaystyle L(\theta )}$ in just one point). In this way, as ${\displaystyle \theta }$ increases in the range ${\displaystyle 0\leq \theta <\pi }$, the line ${\displaystyle L(\theta )}$ represents a line's worth of distinct lines in the plane. But when ${\displaystyle \theta }$ reaches ${\displaystyle \pi }$, ${\displaystyle L(\pi )}$ is identical to ${\displaystyle L(0)}$, and so the families ${\displaystyle P(0)}$ and ${\displaystyle P(\pi )}$ of perpendicular lines are also identical families. The line ${\displaystyle L(0)}$, however, has returned to itself as ${\displaystyle L(\pi )}$ pointed in the opposite direction. Every line in the plane corresponds to exactly one line in some family ${\displaystyle P(\theta )}$, for exactly one ${\displaystyle \theta }$, for ${\displaystyle 0\leq \theta <\pi }$, and ${\displaystyle P(\pi )}$ is identical to ${\displaystyle P(0)}$ but returns pointed in the opposite direction. This ensures that the space of all lines in the plane – the union of all the ${\displaystyle P(\theta )}$ for ${\displaystyle 0\leq \theta <\pi }$ – is an open Möbius band.

The group of bijective linear transformations ${\displaystyle GL(2,\mathbb {R} )}$ of the plane to itself (real ${\displaystyle 2\times 2}$ matrices with non-zero determinant) naturally induces bijections of the space of lines in the plane to itself, which form a group of self-homeomorphisms of the space of lines. Hence the same group forms a group of self-homeomorphisms of the Möbius band described in the previous paragraph. But there is no metric on the space of lines in the plane that is invariant under the action of this group of homeomorphisms. In this sense, the space of lines in the plane has no natural metric on it. This means that the Möbius band possesses a natural 4-dimensional Lie group of self-homeomorphisms, given by ${\displaystyle GL(2,\mathbb {R} )}$, but this high degree of symmetry cannot be exhibited as the group of isometries of any metric.

Möbius band with round boundary

The edge, or boundary, of a Möbius strip is homeomorphic (topologically equivalent) to a circle. Under the usual embeddings of the strip in Euclidean space, as above, the boundary is not a true circle. However, it is possible to embed a Möbius strip in three dimensions so that the boundary is a perfect circle lying in some plane. For example, see Figures 307, 308, and 309 of "Geometry and the imagination".

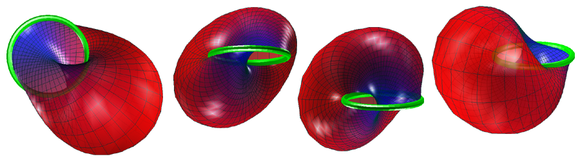
A much more geometric embedding begins with a minimal Klein bottle immersed in the 3-sphere, as discovered by Blaine Lawson. We then take half of this Klein bottle to get a Möbius band embedded in the 3-sphere (the unit sphere in 4-space). The result is sometimes called the "Sudanese Möbius Band", where "sudanese" refers not to the country Sudan but to the names of two topologists, Sue Goodman and Daniel Asimov. Applying stereographic projection to the Sudanese band places it in three-dimensional space, as can be seen below – a version due to George Francis can be found here.

From Lawson's minimal Klein bottle we derive an embedding of the band into the 3-sphere S³, regarded as a subset of C², which is geometrically the same as R⁴. We map angles η, φ to complex numbers z₁, z₂ via

${\displaystyle z_{1}=\sin \eta \,e^{i\varphi }}$

${\displaystyle z_{2}=\cos \eta \,e^{i\varphi /2}.}$

Here the parameter η runs from 0 to π and φ runs from 0 to 2π. Since |z₁|² + |z₂|² = 1, the embedded surface lies entirely in S³. The boundary of the strip is given by |z₂| = 1 (corresponding to η = 0, π), which is clearly a circle on the 3-sphere.

To obtain an embedding of the Möbius strip in R³ one maps S³ to R³ via a stereographic projection. The projection point can be any point on S³ that does not lie on the embedded Möbius strip (this rules out all the usual projection points). One possible choice is ${\displaystyle \left\{1/{\sqrt {2}},i/{\sqrt {2}}\right\}}$. Stereographic projections map circles to circles and preserves the circular boundary of the strip. The result is a smooth embedding of the Möbius strip into R³ with a circular edge and no self-intersections.

The Sudanese Möbius band in the three-sphere S³ is geometrically a fibre bundle over a great circle, whose fibres are great semicircles. The most symmetrical image of a stereographic projection of this band into R³ is obtained by using a projection point that lies on that great circle that runs through the midpoint of each of the semicircles. Each choice of such a projection point results in an image that is congruent to any other. But because such a projection point lies on the Möbius band itself, two aspects of the image are significantly different from the case (illustrated above) where the point is not on the band: 1) the image in R³ is not the full Möbius band, but rather the band with one point removed (from its centerline); and 2) the image is unbounded – and as it gets increasingly far from the origin of R³, it increasingly approximates a plane. Yet this version of the stereographic image has a group of 4 symmetries in R³ (it is isomorphic to the Klein 4-group), as compared with the bounded version illustrated above having its group of symmetries the unique group of order 2. (If all symmetries and not just orientation-preserving isometries of R³ are allowed, the numbers of symmetries in each case doubles.)

But the most geometrically symmetrical version of all is the original Sudanese Möbius band in the three-sphere S³, where its full group of symmetries is isomorphic to the Lie group O(2). Having an infinite cardinality (that of the continuum), this is far larger than the symmetry group of any possible embedding of the Möbius band in R³.

Projective geometry

Using projective geometry, an open Möbius band can be described as the set of solutions to a polynomial equation. Adding a polynomial inequality results in a closed Möbius band. These relate Möbius bands to the geometry of line bundles and the operation of blowing up in algebraic geometry.

The real projective line ${\displaystyle \mathbf {RP} ^{1}}$ is the set ${\displaystyle \mathbf {R} ^{2}\setminus \{(0,0)\}}$ modulo scaling. That is, a point in ${\displaystyle \mathbf {RP} ^{1}}$ is an equivalence class of the form

${\displaystyle [A:B]=\{(\lambda A,\lambda B):\lambda \in \mathbf {R} \setminus \{0\}\}.}$

Every equivalence class ${\displaystyle [A:B]}$ with ${\displaystyle B\neq 0}$ has a unique representative whose second coordinate is 1, namely ${\displaystyle (A/B,1)}$. These points form a copy of the Euclidean line ${\displaystyle \mathbf {R} ^{1}}$. However, the equivalence class of ${\displaystyle [1:0]}$ has no such representative. This extra point behaves like an unsigned infinity, making ${\displaystyle \mathbf {RP} ^{1}}$ topologically the same as the circle ${\displaystyle S^{1}}$. The advantage of ${\displaystyle \mathbf {RP} ^{1}}$ over the circle is that some geometric objects have simpler equations in terms of A and B. This is the case for the Möbius band.

A realization of an open Möbius band is given by the set

${\displaystyle M=\{((x,y),[A:B])\in \mathbf {R} ^{2}\times \mathbf {RP} ^{1}:Ax=By\}.}$

If we delete the line ${\displaystyle x=0}$ from M (or in fact any line), then the resulting subset can be embedded in Euclidean space ${\displaystyle \mathbf {R} ^{3}}$. Deleting this line gives the set

${\displaystyle {\begin{aligned}M'&=\{((x,y),[A:B])\in \mathbf {R} ^{2}\times \mathbf {RP} ^{1}:Ax=By,\ B\neq 0\}\\&=\{(x,y,m)\in \mathbf {R} ^{3}:mx=y\},\end{aligned}}}$

where m corresponds to ${\displaystyle A/B}$.

There is a realization of the closed Möbius band as a similar set, but with an additional inequality to create a boundary:

${\displaystyle N=\{((x,y),[A:B])\in \mathbf {R} ^{2}\times \mathbf {RP} ^{1}:Ax=By,\ x^{2}+y^{2}\leq 1\}.}$

The boundary of N is the set of all points with ${\displaystyle x^{2}+y^{2}=1}$. The geometry of N is very similar to that of M, so we will focus on M in what follows.

The geometry of M can be described in terms of lines through the origin. Every line through the origin in ${\displaystyle \mathbf {R} ^{2}}$ is the solution set of an equation ${\displaystyle ax+by=0}$. The solution set does not change when ${\displaystyle (a,b)}$ is rescaled, so the line only depends on the equivalence class ${\displaystyle [A:B]}$. That is, the lines through the origin are parametrized by ${\displaystyle \mathbf {RP} ^{1}}$. Furthermore, every point ${\displaystyle (x,y)}$ in ${\displaystyle \mathbf {R} ^{2}}$, except for ${\displaystyle (0,0)}$, lies on a unique line through the origin, specifically, the line defined by ${\displaystyle [-y:x]}$. The point ${\displaystyle (x,y)=(0,0)}$, however, lies on every line through the origin. For this point, the equation ${\displaystyle Ax+By=0}$ degenerates to ${\displaystyle 0=0}$. This is always true, so every ${\displaystyle [A:B]}$ is a solution. Consequently, the set M may be described as the disjoint union of the set of lines through the origin. This is the same as the union of the lines through the origin, except that it contains one copy of the origin for each line. These additional copies of the origin are a copy of ${\displaystyle \mathbf {RP} ^{1}}$ and constitute the center circle of the Möbius band. The lines themselves describe the ruling of the Möbius band. This point of view on M exhibits it both as the total space of the tautological line bundle ${\displaystyle {\mathcal {O}}(-1)}$ on ${\displaystyle \mathbf {RP} ^{1}}$ as well as the blow-up of the origin in ${\displaystyle \mathbf {R} ^{2}}$.

To see the half-twist in M, begin with the point ${\displaystyle (1,0)}$ in ${\displaystyle \mathbf {R} ^{2}}$. This corresponds to a unique point of M, namely ${\displaystyle P=((1,0),[0:1])}$. Draw the counterclockwise half circle to produce a path on M given by ${\displaystyle \gamma (t)=((\cos(2\pi t),\sin(2\pi t)),[-\sin(2\pi t),\cos(2\pi t)])}$. The path stops at ${\displaystyle t=1/2}$, where it gives the point ${\displaystyle Q=((-1,0),[0:1])}$. Except for P and Q, every point in the path lies on a different line through the origin. Therefore ${\displaystyle \gamma (t)}$ travels once around the center circle of M. However, while P and Q lie in the same line of the ruling, they are on opposite sides of the origin. This change in sign is the algebraic manifestation of the half-twist.

Related objects

A closely related 'strange' geometrical object is the Klein bottle. A Klein bottle could in theory be produced by gluing two Möbius strips together along their edges; however this cannot be done in ordinary three-dimensional Euclidean space without creating self-intersections.

Another closely related manifold is the real projective plane. If a circular disk is cut out of the real projective plane, what is left is a Möbius strip. Going in the other direction, if one glues a disk to a Möbius strip by identifying their boundaries, the result is the projective plane. To visualize this, it is helpful to deform the Möbius strip so that its boundary is an ordinary circle (see above). The real projective plane, like the Klein bottle, cannot be embedded in three-dimensions without self-intersections.

In graph theory, the Möbius ladder is a cubic graph closely related to the Möbius strip.

In 1968, Gonzalo Vélez Jahn (UCV, Caracas, Venezuela) discovered three dimensional bodies with Möbian characteristics; these were later described by Martin Gardner as prismatic rings that became toroidal polyhedrons in his August 1978 Mathematical Games column in Scientific American.

Applications

Mathematical art: a scarf designed as a Möbius strip

There have been several technical applications for the Möbius strip. Giant Möbius strips have been used as conveyor belts that last longer because the entire surface area of the belt gets the same amount of wear, and as continuous-loop recording tapes (to double the playing time). Möbius strips are common in the manufacture of fabric computer printer and typewriter ribbons, as they let the ribbon be twice as wide as the print head while using both halves evenly.

A Möbius resistor is an electronic circuit element that cancels its own inductive reactance. Nikola Tesla patented similar technology in 1894: "Coil for Electro Magnets" was intended for use with his system of global transmission of electricity without wires.

The Möbius strip is the configuration space of two unordered points on a circle. Consequently, in music theory, the space of all two-note chords, known as dyads, takes the shape of a Möbius strip; this and generalizations to more points is a significant application of orbifolds to music theory.

In physics/electro-technology as:

• A compact resonator with a resonance frequency that is half that of identically constructed linear coils
• An inductionless resistor
• Superconductors with high transition temperature
• Möbius resonator

In chemistry/nano-technology as:

• Molecular knots with special characteristics (Knotane, Chirality)
• Molecular engines
• Graphene volume (nano-graphite) with new electronic characteristics, like helical magnetism
• A special type of aromaticity: Möbius aromaticity
• Charged particles caught in the magnetic field of the Earth that can move on a Möbius band
• The cyclotide (cyclic protein) kalata B1, active substance of the plant Oldenlandia affinis, contains Möbius topology for the peptide backbone.

Arts and entertainment

Ancient Roman mosaic depicting a Möbius strip

The Möbius strip principle has been used as a method of creating the illusion of magic. The trick, known as the Afghan bands, was very popular in the first half of the twentieth century. Many versions of this trick exist and have been performed by famous illusionists such as Harry Blackstone Sr. and Thomas Nelson Downs.

In creative works

The universal recycling symbol (♲) design has its three arrows forming a Möbius loop. According to its designer Gary Anderson, "the figure was designed as a Mobius strip to symbolize continuity within a finite entity".

 

Automated mining

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Automated_mining 

Automated mining involves the removal of human labor from the mining process. The mining industry is in the transition towards automation. It can still require a large amount of human capital, particularly in the developing world where labor costs are low so there is less incentive for increasing efficiency. There are two types of automated mining- process and software automation, and the application of robotic technology to mining vehicles and equipment.

Mine automation software

In order to gain more control over their operations, mining companies may implement mining automation software or processes. Reports generated by mine automation software allow administrators to identify productivity bottlenecks, increase accountability, and better understand return on investment.

Mining equipment automation

Addressing concerns about how to improve productivity and safety in the mine site, some mine companies are turning to equipment automation consisting of robotic hardware and software technologies that convert vehicles or equipment into autonomous mining units.

Mine equipment automation comes in four different forms: remote control, teleoperation, driver assist, and full automation.

Remote control

Remote control mining equipment usually refers to mining vehicles such as excavators or bulldozers that are controlled with a handheld remote control. An operator stands in line-of-sight and uses the remote control to perform the normal vehicle functions. Because visibility and feel of the machine are heavily reduced, vehicle productivity is generally reduced as well using remote control. Remote control technology is generally used to enable mining equipment to operate in dangerous conditions such as unstable terrain, blast areas or in high risk areas of falling debris, or underground mining. Remote control technology is generally the least expensive way to automate mining equipment making it an ideal entry point for companies looking to test the viability of robotic technology in their mine.

Teleoperated mining equipment

Teleoperated mining equipment refers to mining vehicles that are controlled by an operator at a remote location with the use of cameras, sensors, and possibly additional positioning software. Teleoperation allows an operator to further remove themselves from the mining location and control a vehicle from a more protected environment. Joysticks or other handheld controls are still used to control the vehicle's functions, and operators have greater access to vehicle telemetry and positioning data through the teleoperation software. With the operator removed from the cab, teleoperated mining vehicles may also experience reduced productivity; however, the operator has a better vantage point than remote control from on-vehicle cameras and sensors and is further removed from potentially dangerous conditions.

Driver assist

"Driver assist" refers to partly automated control of mining machines. Only some of the functions are automated and operator intervention is needed. Common functions include both spotting assist and collision avoidance systems.

Full automation

"Full automation" can refer to the autonomous control of one or more mining vehicles. Robotic components manage all critical vehicle functions including ignition, steering, transmission, acceleration, braking, and implement control (i.e. blade control, dump bed control, excavator bucket and boom, etc.) without the need for operator intervention. Fully autonomous mining systems experience the most productivity gains as software controls one or more mining vehicles allowing operators to take on the role of mining facilitators, troubleshooting errors and monitoring efficiency.

Benefits

The benefits of mining equipment automation technologies are varied but may include: improved safety, better fuel efficiency, increased productivity, reduced unscheduled maintenance, improved working conditions, better vehicle utilization, and reduced driver fatigue and attrition. Automation technologies are an efficient way to mitigate the effects of widespread labor shortages for positions such as haul truck driver. In the face of falling commodity prices, many mining companies are looking for ways to dramatically reduce overhead costs while still maintaining site safety and integrity; automation may be the answer.

Drawbacks

Critics of vehicle automation often focus on the potential for robotic technology to eliminate jobs while proponents counter that while some jobs will become obsolete (normally the dirty, dangerous, or monotonous jobs), others will be created. Communities supporting underprivileged workers that rely on entry level mining positions are worried about and are calling for social responsibility as mining companies transition to automation technologies that promise to increase productivity in the face of falling commodity prices. Risk averse mining companies are also reluctant to commit large amounts of capital to an unproven technology, preferring more often to enter the automation scene at lower, more inexpensive levels such as remote control.

Examples of autonomous mining equipment

Mine of the future

Rio Tinto Group embarked on their Mine of the Future initiative in 2008. From a control center in Perth, Rio Tinto employees operate autonomous mining equipment in Australia's remote but mineral rich Pilbara region. The autonomous mining vehicles reduce the footprint of the mining giant while improving productivity and vehicle utilization. As of June 2014, Rio Tinto's autonomous mining fleet reached the milestone of 200 million tonnes hauled. Rio Tinto also operate a number of autonomous blast hole drill rigs.

Bingham Canyon Mine

Located near Salt Lake City, Utah, the Bingham Canyon Mine (Kennecott Utah Copper/Rio Tinto) is one of the largest open pit mine in the world and one of the world's largest copper producers. In April 2013, the mine experienced a catastrophic landslide that halted much of the mine's operations. As part of the cleanup efforts and to improve safety, mine administrators turned to remote control excavator, dozers and teleremote blast hole drills to perform work on the highly unstable terrain areas. Robotic technology helped Kennecott to reduce the steeper, more dangerous areas of the slide to allow manned vehicles access for cleanup efforts.

Automation of underground works in China

German company «EEP Elektro-Elektronik Pranjic» delivered and put into operation more than 60 sets of advanced automatic control for underground coal mining for the period ~ 2006-2016. For the first time completely deserted coal mining technology has been used by the Chinese concern «China National Coal Group Corp. (CME)» at the mine «Tang Shan Gou» (longwall mining, shearers, three lava, depth 200 m), and at the mine «Nan Liang» (one plow, depth 100 m). Both coal mines have coal layer thickness 1-1.7 m. Monitoring the harvesting is carried out by means of video cameras (in real time with signal transmission over optical fiber). Typically, an underground staff is required to monitor the production process and for carrying out repairs. Automation has improved the safety and economic performance.

Next Generation Mining

BHP have deployed a number of autonomous mining components as part of their Next Generation Mining program. This includes autonomous drills  and autonomous trucks  in the Pilbara region.

Autonomy in Europe

In March 2021, Ferrexpo plc announced that it had successfully deployed the first large scale autonomous mining trucks in Europe with the conversion of its CAT 793D haul trucks. The Company has used semi-autonomous drill rigs at its operations since 2017.

 

Android (robot)

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Android_(robot)

An android is a robot or other artificial being designed to resemble a human, and often made from a flesh-like material. Historically, androids were completely within the domain of science fiction and frequently seen in film and television, but recent advances in robot technology now allow the design of functional and realistic humanoid robots.

Etymology

Early example of the term androides used to describe human-like mechanical devices, London Times, 22 December 1795

The word was coined from the Greek root ἀνδρ- andr- "man, male" (as opposed to ἀνθρωπ- anthrōp- "human being") and the suffix -oid "having the form or likeness of". In Greek, however, ανδροειδής is an adjective. While the term "android" is used in reference to human-looking robots in general, a robot with a female appearance can also be referred to as a gynoid.

The Oxford English Dictionary traces the earliest use (as "Androides") to Ephraim Chambers' 1728 Cyclopaedia, in reference to an automaton that St. Albertus Magnus allegedly created. By the late 1700s "androides", elaborate mechanical devices resembling humans performing human activities, were displayed in exhibit halls. The term "android" appears in US patents as early as 1863 in reference to miniature human-like toy automatons. The term android was used in a more modern sense by the French author Auguste Villiers de l'Isle-Adam in his work Tomorrow's Eve (1886). This story features an artificial humanlike robot named Hadaly. As said by the officer in the story, "In this age of Realien advancement, who knows what goes on in the mind of those responsible for these mechanical dolls." The term made an impact into English pulp science fiction starting from Jack Williamson's The Cometeers (1936) and the distinction between mechanical robots and fleshy androids was popularized by Edmond Hamilton's Captain Future (1940–1944).

Although Karel Čapek's robots in R.U.R. (Rossum's Universal Robots) (1921)—the play that introduced the word robot to the world—were organic artificial humans, the word "robot" has come to primarily refer to mechanical humans, animals, and other beings. The term "android" can mean either one of these, while a cyborg ("cybernetic organism" or "bionic man") would be a creature that is a combination of organic and mechanical parts.

The term "droid", popularized by George Lucas in the original Star Wars film and now used widely within science fiction, originated as an abridgment of "android", but has been used by Lucas and others to mean any robot, including distinctly non-human form machines like R2-D2. The word "android" was used in Star Trek: The Original Series episode "What Are Little Girls Made Of?" The abbreviation "andy", coined as a pejorative by writer Philip K. Dick in his novel Do Androids Dream of Electric Sheep?, has seen some further usage, such as within the TV series Total Recall 2070.

Authors have used the term android in more diverse ways than robot or cyborg. In some fictional works, the difference between a robot and android is only superficial, with androids being made to look like humans on the outside but with robot-like internal mechanics. In other stories, authors have used the word "android" to mean a wholly organic, yet artificial, creation. Other fictional depictions of androids fall somewhere in between.

Eric G. Wilson, who defines an android as a "synthetic human being", distinguishes between three types of android, based on their body's composition:

• the mummy type – made of "dead things" or "stiff, inanimate, natural material", such as mummies, puppets, dolls and statues
• the golem type – made from flexible, possibly organic material, including golems and homunculi
• the automaton type – made from a mix of dead and living parts, including automatons and robots

Although human morphology is not necessarily the ideal form for working robots, the fascination in developing robots that can mimic it can be found historically in the assimilation of two concepts: simulacra (devices that exhibit likeness) and automata (devices that have independence).

Projects

Several projects aiming to create androids that look, and, to a certain degree, speak or act like a human being have been launched or are underway.

Japan

DER 01, a Japanese actroid

Japanese robotics have been leading the field since the 1970s. Waseda University initiated the WABOT project in 1967, and in 1972 completed the WABOT-1, the first android, a full-scale humanoid intelligent robot. Its limb control system allowed it to walk with the lower limbs, and to grip and transport objects with hands, using tactile sensors. Its vision system allowed it to measure distances and directions to objects using external receptors, artificial eyes and ears. And its conversation system allowed it to communicate with a person in Japanese, with an artificial mouth.

In 1984, WABOT-2 was revealed, and made a number of improvements. It was capable of playing the organ. Wabot-2 had ten fingers and two feet, and was able to read a score of music. It was also able to accompany a person. In 1986, Honda began its humanoid research and development program, to create humanoid robots capable of interacting successfully with humans.

The Intelligent Robotics Lab, directed by Hiroshi Ishiguro at Osaka University, and the Kokoro company demonstrated the Actroid at Expo 2005 in Aichi Prefecture, Japan and released the Telenoid R1 in 2010. In 2006, Kokoro developed a new DER 2 android. The height of the human body part of DER2 is 165 cm. There are 47 mobile points. DER2 can not only change its expression but also move its hands and feet and twist its body. The "air servosystem" which Kokoro developed originally is used for the actuator. As a result of having an actuator controlled precisely with air pressure via a servosystem, the movement is very fluid and there is very little noise. DER2 realized a slimmer body than that of the former version by using a smaller cylinder. Outwardly DER2 has a more beautiful proportion. Compared to the previous model, DER2 has thinner arms and a wider repertoire of expressions. Once programmed, it is able to choreograph its motions and gestures with its voice.

The Intelligent Mechatronics Lab, directed by Hiroshi Kobayashi at the Tokyo University of Science, has developed an android head called Saya, which was exhibited at Robodex 2002 in Yokohama, Japan. There are several other initiatives around the world involving humanoid research and development at this time, which will hopefully introduce a broader spectrum of realized technology in the near future. Now Saya is working at the Science University of Tokyo as a guide.

The Waseda University (Japan) and NTT Docomo's manufacturers have succeeded in creating a shape-shifting robot WD-2. It is capable of changing its face. At first, the creators decided the positions of the necessary points to express the outline, eyes, nose, and so on of a certain person. The robot expresses its face by moving all points to the decided positions, they say. The first version of the robot was first developed back in 2003. After that, a year later, they made a couple of major improvements to the design. The robot features an elastic mask made from the average head dummy. It uses a driving system with a 3DOF unit. The WD-2 robot can change its facial features by activating specific facial points on a mask, with each point possessing three degrees of freedom. This one has 17 facial points, for a total of 56 degrees of freedom. As for the materials they used, the WD-2's mask is fabricated with a highly elastic material called Septom, with bits of steel wool mixed in for added strength. Other technical features reveal a shaft driven behind the mask at the desired facial point, driven by a DC motor with a simple pulley and a slide screw. Apparently, the researchers can also modify the shape of the mask based on actual human faces. To "copy" a face, they need only a 3D scanner to determine the locations of an individual's 17 facial points. After that, they are then driven into position using a laptop and 56 motor control boards. In addition, the researchers also mention that the shifting robot can even display an individual's hair style and skin color if a photo of their face is projected onto the 3D Mask.

Singapore

Prof Nadia Thalmann, a Nanyang Technological University scientist, directed efforts of the Institute for Media Innovation along with the School of Computer Engineering in the development of a social robot, Nadine. Nadine is powered by software similar to Apple's Siri or Microsoft's Cortana. Nadine may become a personal assistant in offices and homes in future, or she may become a companion for the young and the elderly.

Assoc Prof Gerald Seet from the School of Mechanical & Aerospace Engineering and the BeingThere Centre led a three-year R&D development in tele-presence robotics, creating EDGAR. A remote user can control EDGAR with the user's face and expressions displayed on the robot's face in real time. The robot also mimics their upper body movements. 

South Korea

EveR-2, the first android that has the ability to sing

KITECH researched and developed EveR-1, an android interpersonal communications model capable of emulating human emotional expression via facial "musculature" and capable of rudimentary conversation, having a vocabulary of around 400 words. She is 160 cm tall and weighs 50 kg, matching the average figure of a Korean woman in her twenties. EveR-1's name derives from the Biblical Eve, plus the letter r for robot. EveR-1's advanced computing processing power enables speech recognition and vocal synthesis, at the same time processing lip synchronization and visual recognition by 90-degree micro-CCD cameras with face recognition technology. An independent microchip inside her artificial brain handles gesture expression, body coordination, and emotion expression. Her whole body is made of highly advanced synthetic jelly silicon and with 60 artificial joints in her face, neck, and lower body; she is able to demonstrate realistic facial expressions and sing while simultaneously dancing. In South Korea, the Ministry of Information and Communication has an ambitious plan to put a robot in every household by 2020. Several robot cities have been planned for the country: the first will be built in 2016 at a cost of 500 billion won (US$440 million), of which 50 billion is direct government investment. The new robot city will feature research and development centers for manufacturers and part suppliers, as well as exhibition halls and a stadium for robot competitions. The country's new Robotics Ethics Charter will establish ground rules and laws for human interaction with robots in the future, setting standards for robotics users and manufacturers, as well as guidelines on ethical standards to be programmed into robots to prevent human abuse of robots and vice versa.

United States

Walt Disney and a staff of Imagineers created Great Moments with Mr. Lincoln that debuted at the 1964 New York World's Fair.

Dr. William Barry, an Education Futurist and former visiting West Point Professor of Philosophy and Ethical Reasoning at the United States Military Academy, created an AI android character named "Maria Bot". This Interface AI android was named after the infamous fictional robot Maria in the 1927 film Metropolis, as a well-behaved distant relative. Maria Bot is the first AI Android Teaching Assistant at the university level. Maria Bot has appeared as a keynote speaker as a duo with Barry for a TEDx talk in Everett, Washington in February 2020.

Dr. William Barry (left) with Maria Bot (right)

Resembling a human from the shoulders up, Maria Bot is a virtual being android that has complex facial expressions and head movement and engages in conversation about a variety of subjects. She uses AI to process and synthesize information to make her own decisions on how to talk and engage. She collects data through conversations, direct data inputs such as books or articles, and through internet sources.

Maria Bot was built by an international high-tech company for Barry to help improve education quality and eliminate education poverty. Maria Bot is designed to create new ways for students to engage and discuss ethical issues raised by the increasing presence of robots and artificial intelligence. Barry also uses Maria Bot to demonstrate that programming a robot with life-affirming, ethical framework makes them more likely to help humans to do the same. 

Dr. William Barry (right) and a fan pose with Maria Bot (center)

Maria Bot is an ambassador robot for good and ethical AI technology.

Hanson Robotics, Inc., of Texas and KAIST produced an android portrait of Albert Einstein, using Hanson's facial android technology mounted on KAIST's life-size walking bipedal robot body. This Einstein android, also called "Albert Hubo", thus represents the first full-body walking android in history. Hanson Robotics, the FedEx Institute of Technology, and the University of Texas at Arlington also developed the android portrait of sci-fi author Philip K. Dick (creator of Do Androids Dream of Electric Sheep?, the basis for the film Blade Runner), with full conversational capabilities that incorporated thousands of pages of the author's works. In 2005, the PKD android won a first-place artificial intelligence award from AAAI.

Use in fiction

Androids are a staple of science fiction. Isaac Asimov pioneered the fictionalization of the science of robotics and artificial intelligence, notably in his 1950s series I, Robot. One thing common to most fictional androids is that the real-life technological challenges associated with creating thoroughly human-like robots—such as the creation of strong artificial intelligence—are assumed to have been solved. Fictional androids are often depicted as mentally and physically equal or superior to humans—moving, thinking and speaking as fluidly as them.

The tension between the nonhuman substance and the human appearance—or even human ambitions—of androids is the dramatic impetus behind most of their fictional depictions. Some android heroes seek, like Pinocchio, to become human, as in the film Bicentennial Man, or Data in Star Trek: The Next Generation. Others, as in the film Westworld, rebel against abuse by careless humans. Android hunter Deckard in Do Androids Dream of Electric Sheep? and its film adaptation Blade Runner discovers that his targets appear to be, in some ways, more "human" than he is. Android stories, therefore, are not essentially stories "about" androids; they are stories about the human condition and what it means to be human.

One aspect of writing about the meaning of humanity is to use discrimination against androids as a mechanism for exploring racism in society, as in Blade Runner. Perhaps the clearest example of this is John Brunner's 1968 novel Into the Slave Nebula, where the blue-skinned android slaves are explicitly shown to be fully human. More recently, the androids Bishop and Annalee Call in the films Aliens and Alien Resurrection are used as vehicles for exploring how humans deal with the presence of an "Other". The 2018 video game Detroit: Become Human also explores how androids are treated as second class citizens in a near future society.

Female androids, or "gynoids", are often seen in science fiction, and can be viewed as a continuation of the long tradition of men attempting to create the stereotypical "perfect woman". Examples include the Greek myth of Pygmalion and the female robot Maria in Fritz Lang's Metropolis. Some gynoids, like Pris in Blade Runner, are designed as sex-objects, with the intent of "pleasing men's violent sexual desires", or as submissive, servile companions, such as in The Stepford Wives. Fiction about gynoids has therefore been described as reinforcing "essentialist ideas of femininity", although others have suggested that the treatment of androids is a way of exploring racism and misogyny in society.

The 2015 Japanese film Sayonara, starring Geminoid F, was promoted as "the first movie to feature an android performing opposite a human actor".