Feed forward (control)

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Feed_forward_(control)

The three types of control system

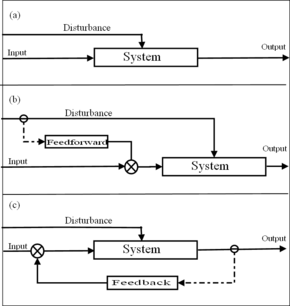
1. Open-loop control
2. Feed-forward
3. Feedback (closed loop) control

A feed forward (sometimes written feedforward) is an element or pathway within a control system that passes a controlling signal from a source in its external environment to a load elsewhere in its external environment. This is often a command signal from an external operator.

In mechanical engineering, a feedforward control system is a control system that uses sensors to detect disturbances affecting the machine and then applies an additional input to minimize the effect of the disturbance. This requires a mathematical model of the machine so that the effect of disturbances can be properly predicted.

A control system which has only feed-forward behavior responds to its control signal in a pre-defined way without responding to the way the load reacts; it is in contrast with a system that also has feedback, which adjusts the input to take account of how it affects the load, and how the load itself may vary unpredictably; the load is considered to belong to the external environment of the system.

In a feed-forward system, the control variable adjustment is not error-based. Instead it is based on knowledge about the process in the form of a mathematical model of the process and knowledge about, or measurements of, the process disturbances.

Some prerequisites are needed for control scheme to be reliable by pure feed-forward without feedback: the external command or controlling signal must be available, and the effect of the output of the system on the load should be known (that usually means that the load must be predictably unchanging with time). Sometimes pure feed-forward control without feedback is called 'ballistic', because once a control signal has been sent, it cannot be further adjusted; any corrective adjustment must be by way of a new control signal. In contrast, 'cruise control' adjusts the output in response to the load that it encounters, by a feedback mechanism.

These systems could relate to control theory, physiology, or computing.

Overview

With feed-forward or feedforward control, the disturbances are measured and accounted for before they have time to affect the system. In the house example, a feed-forward system may measure the fact that the door is opened and automatically turn on the heater before the house can get too cold. The difficulty with feed-forward control is that the effects of the disturbances on the system must be accurately predicted, and there must not be any unmeasured disturbances. For instance, if a window was opened that was not being measured, the feed-forward-controlled thermostat might let the house cool down.

The term has specific meaning within the field of CPU-based automatic control. The discipline of "feedforward control" as it relates to modern, CPU based automatic controls is widely discussed, but is seldom practiced due to the difficulty and expense of developing or providing for the mathematical model required to facilitate this type of control. Open-loop control and feedback control, often based on canned PID control algorithms, are much more widely used.

There are three types of control systems: open loop, feed-forward, and feedback. An example of a pure open loop control system is manual non-power-assisted steering of a motor car; the steering system does not have access to an auxiliary power source and does not respond to varying resistance to turning of the direction wheels; the driver must make that response without help from the steering system. In comparison, power steering has access to a controlled auxiliary power source, which depends on the engine speed. When the steering wheel is turned, a valve is opened which allows fluid under pressure to turn the driving wheels. A sensor monitors that pressure so that the valve only opens enough to cause the correct pressure to reach the wheel turning mechanism. This is feed-forward control where the output of the system, the change in direction of travel of the vehicle, plays no part in the system. See Model predictive control.

If the driver is included in the system, then they do provide a feedback path by observing the direction of travel and compensating for errors by turning the steering wheel. In that case you have a feedback system, and the block labeled "System" in Figure(c) is a feed-forward system.

In other words, systems of different types can be nested, and the overall system regarded as a black-box.

Feedforward control is distinctly different from open loop control and teleoperator systems. Feedforward control requires a mathematical model of the plant (process and/or machine being controlled) and the plant's relationship to any inputs or feedback the system might receive. Neither open loop control nor teleoperator systems require the sophistication of a mathematical model of the physical system or plant being controlled. Control based on operator input without integral processing and interpretation through a mathematical model of the system is a teleoperator system and is not considered feedforward control.

History

Historically, the use of the term "feedforward" is found in works by Harold S. Black in US patent 1686792 (invented 17 March 1923) and D. M. MacKay as early as 1956. While MacKay's work is in the field of biological control theory, he speaks only of feedforward systems. MacKay does not mention "Feedforward Control" or allude to the discipline of "Feedforward Controls." MacKay and other early writers who use the term "feedforward" are generally writing about theories of how human or animal brains work. Black also has US patent 2102671 invented 2 August 1927 on the technique of feedback applied to electronic systems.

The discipline of "feedforward controls" was largely developed by professors and graduate students at Georgia Tech, MIT, Stanford and Carnegie Mellon. Feedforward is not typically hyphenated in scholarly publications. Meckl and Seering of MIT and Book and Dickerson of Georgia Tech began the development of the concepts of Feedforward Control in the mid-1970s. The discipline of Feedforward Controls was well defined in many scholarly papers, articles and books by the late 1980s.

Benefits

The benefits of feedforward control are significant and can often justify the extra cost, time and effort required to implement the technology. Control accuracy can often be improved by as much as an order of magnitude if the mathematical model is of sufficient quality and implementation of the feedforward control law is well thought out. Energy consumption by the feedforward control system and its driver is typically substantially lower than with other controls. Stability is enhanced such that the controlled device can be built of lower cost, lighter weight, springier materials while still being highly accurate and able to operate at high speeds. Other benefits of feedforward control include reduced wear and tear on equipment, lower maintenance costs, higher reliability and a substantial reduction in hysteresis. Feedforward control is often combined with feedback control to optimize performance.

Model

The mathematical model of the plant (machine, process or organism) used by the feedforward control system may be created and input by a control engineer or it may be learned by the control system.^[16] Control systems capable of learning and/or adapting their mathematical model have become more practical as microprocessor speeds have increased. The discipline of modern feedforward control was itself made possible by the invention of microprocessors.

Feedforward control requires integration of the mathematical model into the control algorithm such that it is used to determine the control actions based on what is known about the state of the system being controlled. In the case of control for a lightweight, flexible robotic arm, this could be as simple as compensating between when the robot arm is carrying a payload and when it is not. The target joint angles are adjusted to place the payload in the desired position based on knowing the deflections in the arm from the mathematical model's interpretation of the disturbance caused by the payload. Systems that plan actions and then pass the plan to a different system for execution do not satisfy the above definition of feedforward control. Unless the system includes a means to detect a disturbance or receive an input and process that input through the mathematical model to determine the required modification to the control action, it is not true feedforward control.

Open system

In systems theory, an open system is a feed forward system that does not have any feedback loop to control its output. In contrast, a closed system uses on a feedback loop to control the operation of the system. In an open system, the output of the system is not fed back into the input to the system for control or operation.

Applications

Physiological feed-forward system

Main article: Neural top–down control of physiology

In physiology, feed-forward control is exemplified by the normal anticipatory regulation of heartbeat in advance of actual physical exertion by the central autonomic network. Feed-forward control can be likened to learned anticipatory responses to known cues (predictive coding). Feedback regulation of the heartbeat provides further adaptiveness to the running eventualities of physical exertion. Feedforward systems are also found in biological control of other variables by many regions of animals brains.

Even in the case of biological feedforward systems, such as in the human brain, knowledge or a mental model of the plant (body) can be considered to be mathematical as the model is characterized by limits, rhythms, mechanics and patterns.

A pure feed-forward system is different from a homeostatic control system, which has the function of keeping the body's internal environment 'steady' or in a 'prolonged steady state of readiness.' A homeostatic control system relies mainly on feedback (especially negative), in addition to the feedforward elements of the system.

Gene regulation and feed-forward

Feed-forward loops (FFLs), a three-node graph of the form A affects B and C and B affects C, are frequently observed in transcription networks in several organisms including E. coli and S. cerevisiae, suggesting that they perform functions that are important for the functioning of these organisms. In E. coli and S. cerevisiae transcription networks have been extensively studied, FFLs occur approximately three times more frequently than expected based on random (Erdös-Rényi) networks.

Edges in transcription networks are directed and signed, as they represent activation (+) or repression (-). The sign of a path in a transcription network can be obtained by multiplying the signs of the edges in the path, so a path with an odd number of negative signs is negative. There are eight possible three-node FFLs as each of the three arrows can be either repression or activation, which can be classified into coherent or incoherent FFLs. Coherent FFLs have the same sign for both the paths from A to C, and incoherent FFLs have different signs for the two paths.

The temporal dynamics of FFLs show that coherent FFLs can be sign-sensitive delays that filter input into the circuit. We consider the differential equations for a Type-I coherent FFL, where all the arrows are positive:

${\displaystyle \frac{\delta B}{\delta t}={\beta }_B(A)-{\gamma }_{B}B}$

${\displaystyle \frac{\delta C}{\delta t}={\beta }_C(A,B)-{\gamma }_{C}C}$

Where ${\displaystyle {\beta }_y}$ and ${\displaystyle {\beta }_z}$ are increasing functions in ${\displaystyle A}$ and ${\displaystyle B}$ representing production, and ${\displaystyle {\gamma }_Y}$ and ${\displaystyle {\gamma }_z}$ are rate constants representing degradation or dilution of ${\displaystyle B}$ and ${\displaystyle C}$ respectively. ${\displaystyle {\beta }_C(A,B)}$ can represent an AND gate where ${\displaystyle {\beta }_C(A,B)=0}$ if either ${\displaystyle A=0}$ or ${\displaystyle B=0}$, for instance if ${\displaystyle {\beta }_C(A,B)={\beta }_C{\theta }_A(A>k_{AC}){\theta }_A(B>k_{ABC})}$ where ${\displaystyle {\theta }_A}$ and ${\displaystyle {\theta }_B}$ are step functions. In this case the FFL creates a time-delay between a sustained on-signal, i.e. increase in ${\displaystyle A}$ and the output increase in ${\displaystyle C}$. This is because production of ${\displaystyle A}$ must first induce production of ${\displaystyle B}$, which is then needed to induce production of ${\displaystyle C}$. However, there is no time-delay in for an off-signal because a reduction of ${\displaystyle A}$ immediately results in a decrease in the production term ${\displaystyle {\beta }_C(A,B)}$. This system therefore filters out fluctuations in the on-signal and detects persistent signals. This is particularly relevant in settings with stochastically fluctuating signals. In bacteria these circuits create time delays ranging from a few minutes to a few hours.

Similarly, an inclusive-OR gate in which ${\displaystyle C}$ is activated by either ${\displaystyle A}$ or ${\displaystyle B}$ is a sign-sensitive delay with no delay after the ON step but with a delay after the OFF step. This is because an ON pulse immediately activates B and C, but an OFF step does not immediately result in deactivation of C because B can still be active. This can protect the system from fluctuations that result in the transient loss of the ON signal and can also provide a form of memory. Kalir, Mangan, and Alon, 2005 show that the regulatory system for flagella in E. coli is regulated with a Type 1 coherent feedforward loop.

For instance, the regulation of the shift from one carbon source to another in diauxic growth in E. coli can be controlled via a type-1 coherent FFL. In diauxic growth cells growth using two carbon sources by first rapidly consuming the preferred carbon source, and then slowing growth in a lag phase before consuming the second less preferred carbon source. In E. coli, glucose is preferred over both arabinose and lactose. The absence of glucose is represented via a small molecule cAMP. Diauxic growth in glucose and lactose is regulated by a simple regulatory system involving cAMP and the lac operon. However, growth in arabinose is regulated by a feedforward loop with an AND gate which confers an approximately 20 minute time delay between the ON-step in which cAMP concentration increases when glucose is consumed and when arabinose transporters are expressed. There is no time delay with the OFF signal which occurs when glucose is present. This prevents the cell from shifting to growth on arabinose based on short term fluctuations in glucose availability.

Additionally, feedforward loops can facilitate cellular memory. Doncic and Skotheim (2003) show this in the regulation in the mating of yeast, where extracellular mating pheromone which indices mating behavior including preventing cells from entering the cell cycle. The mating pheromone activates the MAPK pathway which then activates the cell-cycle inhibitor Far1 and activates the Ste12 transcription factor that increases the synthesis of inactive Far1. In this system the concentration of active Far1 depends on the time integral of a function of the external mating pheromone concentration. This dependence on past levels of mating pheromone is a form of cellular memory. This system simultaneously allows for the stability and reversibility.

Incoherent feedforward loops, in which the two paths from the input to the output node have different signs result in short pulses in response to an ON signal. In this system input A simultaneous directly increases and indirectly decreases synthesis of output node C. If the indirect path to C (via B) is slower than the direct path a pulse of output is produced in the time period before levels of B are high enough to inhibit synthesis of C. Response to epidermal growth factor (EGF) in dividing mammalian cells is an example of a Type-1 incoherent FFL.

The frequent observation of feed-forward loops in several biological contexts across scales suggests that they have structural properties that are highly adaptive in several contexts. Several theoretical and experimental studies including those discussed here show that FFLs create a mechanism for biological systems to process and store information, which is important for predictive behavior and survival in complex dynamically changing environments.

Feed-forward systems in computing

Main article: Perceptron

In computing, feed-forward normally refers to a perceptron network in which the outputs from all neurons go to following but not preceding layers, so there are no feedback loops. The connections are set up during a training phase, which in effect is when the system is a feedback system.

Long distance telephony

In the early 1970s, intercity coaxial transmission systems, including L-carrier, used feed-forward amplifiers to diminish linear distortion. This more complex method allowed wider bandwidth than earlier feedback systems. Optical fiber, however, made such systems obsolete before many were built.

Automation and machine control

Feedforward control is a discipline within the field of automatic controls used in automation.

Parallel feed-forward compensation with derivative (PFCD)

The method is rather a new technique that changes the phase of an open-loop transfer function of a non-minimum phase system into minimum phase.

Feedforward neural network

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Feedforward_neural_network 

In a feedforward network, information always moves one direction; it never goes backwards.

Simplified example of training a neural network in object detection: The network is trained by multiple images that are known to depict starfish and sea urchins, which are correlated with "nodes" that represent visual features. The starfish match with a ringed texture and a star outline, whereas most sea urchins match with a striped texture and oval shape. However, the instance of a ring textured sea urchin creates a weakly weighted association between them.

 

Subsequent run of the network on an input image (left): The network correctly detects the starfish. However, the weakly weighted association between ringed texture and sea urchin also confers a weak signal to the latter from one of two intermediate nodes. In addition, a shell that was not included in the training gives a weak signal for the oval shape, also resulting in a weak signal for the sea urchin output. These weak signals may result in a false positive result for sea urchin.
In reality, textures and outlines would not be represented by single nodes, but rather by associated weight patterns of multiple nodes.

A feedforward neural network (FNN) is one of the two broad types of artificial neural network, characterized by direction of the flow of information between its layers. Its flow is uni-directional, meaning that the information in the model flows in only one direction—forward—from the input nodes, through the hidden nodes (if any) and to the output nodes, without any cycles or loops, in contrast to recurrent neural networks, which have a bi-directional flow. Modern feedforward networks are trained using the backpropagation method and are colloquially referred to as the "vanilla" neural networks.

Timeline

• In 1958, a layered network of perceptrons, consisting of an input layer, a hidden layer with randomized weights that did not learn, and an output layer with learning connections, was introduced already by Frank Rosenblatt in his book Perceptron. This extreme learning machine was not yet a deep learning network.

• In 1965, the first deep-learning feedforward network, not yet using stochastic gradient descent, was published by Alexey Grigorevich Ivakhnenko and Valentin Lapa, at the time called the Group Method of Data Handling.

• In 1967, a deep-learning network, using stochastic gradient descent for the first time, was able to classify non-linearily separable pattern classes, as reported Shun'ichi Amari. Amari's student Saito conducted the computer experiments, using a five-layered feedforward network with two learning layers.

• In 1970, modern backpropagation method, an efficient application of a chain-rule-based supervised learning, was for the first time published by the Finnish researcher Seppo Linnainmaa. The term (i.e. "back-propagating errors") itself has been used by Rosenblatt himself, but he did not know how to implement it, although a continuous precursor of backpropagation was already used in the context of control theory in 1960 by Henry J. Kelley. It is known also as a reverse mode of automatic differentiation.

• In 1982, backpropagation was applied in the way that has become standard, for the first time by Paul Werbos.

• In 1985, an experimental analysis of the technique was conducted by David E. Rumelhart et al. Many improvements to the approach have been made in subsequent decades.

• In 1987, using a stochastic gradient descent within a (wide 12-layer nonlinear) feed-forward network, Matthew Brand has trained it to reproduce logic functions of nontrivial circuit depth, using small batches of random input/output samples. He, however, concluded that on hardware (sub-megaflop computers) available at the time it was impractical, and proposed using fixed random early layers as an input hash for a single modifiable layer.

• In 1990s, an (much simpler) alternative to using neural networks, although still related support vector machine approach was developed by Vladimir Vapnik and his colleagues. In addition to performing linear classification, they were able to efficiently perform a non-linear classification using what is called the kernel trick, using high-dimensional feature spaces.

• In 2003, interest in backpropagation networks returned due to the successes of deep learning being applied to language modelling by Yoshua Bengio with co-authors.

• In 2017, modern transformer architectures has been introduced.

• In 2021, a very simple NN architecture combining two deep MLPs with skip connections and layer normalizations was designed and called MLP-Mixer; its realizations featuring 19 to 431 millions of parameters were shown to be comparable to vision transformers of similar size on ImageNet and similar image classification tasks.

Mathematical foundations

Activation function

The two historically common activation functions are both sigmoids, and are described by

${\displaystyle y(v_i)=\tanh (v_i)\text{and}y(v_i)=(1+e^{-v_i}{)}^{-1}}$.

The first is a hyperbolic tangent that ranges from -1 to 1, while the other is the logistic function, which is similar in shape but ranges from 0 to 1. Here ${\displaystyle y_i}$ is the output of the ${\displaystyle i}$th node (neuron) and ${\displaystyle v_i}$ is the weighted sum of the input connections. Alternative activation functions have been proposed, including the rectifier and softplus functions. More specialized activation functions include radial basis functions (used in radial basis networks, another class of supervised neural network models).

In recent developments of deep learning the rectified linear unit (ReLU) is more frequently used as one of the possible ways to overcome the numerical problems related to the sigmoids.

Learning

Learning occurs by changing connection weights after each piece of data is processed, based on the amount of error in the output compared to the expected result. This is an example of supervised learning, and is carried out through backpropagation.

We can represent the degree of error in an output node ${\displaystyle j}$ in the ${\displaystyle n}$th data point (training example) by ${\displaystyle e_j(n)=d_j(n)-y_j(n)}$, where ${\displaystyle d_j(n)}$ is the desired target value for ${\displaystyle n}$th data point at node ${\displaystyle j}$, and ${\displaystyle y_j(n)}$ is the value produced at node ${\displaystyle j}$ when the ${\displaystyle n}$th data point is given as an input.

The node weights can then be adjusted based on corrections that minimize the error in the entire output for the ${\displaystyle n}$th data point, given by

${\displaystyle \mathcal{E}(n)=\frac{1}{2}\sum _{\text{output node }j}{e}_j^2(n)}$.

Using gradient descent, the change in each weight ${\displaystyle w_{ij}}$ is

${\displaystyle \mathrm{\Delta }{w}_{ji}(n)=-\eta \frac{\mathrm{\partial }\mathcal{E}(n)}{\mathrm{\partial }{v}_j(n)}{y}_i(n)}$

where ${\displaystyle y_i(n)}$ is the output of the previous neuron ${\displaystyle i}$, and ${\displaystyle \eta }$ is the learning rate, which is selected to ensure that the weights quickly converge to a response, without oscillations. In the previous expression, ${\displaystyle \frac{\mathrm{\partial }\mathcal{E}(n)}{\mathrm{\partial }{v}_j(n)}}$ denotes the partial derivate of the error ${\displaystyle \mathcal{E}(n)}$ according to the weighted sum ${\displaystyle v_j(n)}$ of the input connections of neuron ${\displaystyle i}$.

The derivative to be calculated depends on the induced local field ${\displaystyle v_j}$, which itself varies. It is easy to prove that for an output node this derivative can be simplified to

${\displaystyle -\frac{\mathrm{\partial }\mathcal{E}(n)}{\mathrm{\partial }{v}_j(n)}=e_j(n){\varphi }^{\mathrm{\prime }}(v_j(n))}$

where ${\displaystyle {\varphi }^{\mathrm{\prime }}}$ is the derivative of the activation function described above, which itself does not vary. The analysis is more difficult for the change in weights to a hidden node, but it can be shown that the relevant derivative is

${\displaystyle -\frac{\mathrm{\partial }\mathcal{E}(n)}{\mathrm{\partial }{v}_j(n)}={\varphi }^{\mathrm{\prime }}(v_j(n))\sum _k-\frac{\mathrm{\partial }\mathcal{E}(n)}{\mathrm{\partial }{v}_k(n)}{w}_{kj}(n)}$.

This depends on the change in weights of the ${\displaystyle k}$th nodes, which represent the output layer. So to change the hidden layer weights, the output layer weights change according to the derivative of the activation function, and so this algorithm represents a backpropagation of the activation function.

History

Linear neural network

The simplest kind of feedforward neural network is a linear network, which consists of a single layer of output nodes; the inputs are fed directly to the outputs via a series of weights. The sum of the products of the weights and the inputs is calculated in each node. The mean squared errors between these calculated outputs and a given target values are minimized by creating an adjustment to the weights. This technique has been known for over two centuries as the method of least squares or linear regression. It was used as a means of finding a good rough linear fit to a set of points by Legendre (1805) and Gauss (1795) for the prediction of planetary movement.

Perceptron

Main article: Perceptron

If using a threshold, i.e. a linear activation function, the resulting linear threshold unit is called a perceptron. (Often the term is used to denote just one of these units.) Multiple parallel linear units are able to approximate any continuous function from a compact interval of the real numbers into the interval [−1,1] despite the limited computational power of single unit with a linear threshold function. This result can be found in Peter Auer, Harald Burgsteiner and Wolfgang Maass "A learning rule for very simple universal approximators consisting of a single layer of perceptrons".

Perceptrons can be trained by a simple learning algorithm that is usually called the delta rule. It calculates the errors between calculated output and sample output data, and uses this to create an adjustment to the weights, thus implementing a form of gradient descent.

Multilayer perceptron

A two-layer neural network capable of calculating XOR. The numbers within the neurons represent each neuron's explicit threshold. The numbers that annotate arrows represent the weight of the inputs. Note that If the threshold of 2 is met then a value of 1 is used for the weight multiplication to the next layer. Not meeting the threshold results in 0 being used. The bottom layer of inputs is not always considered a real neural network layer

A multilayer perceptron (MLP) is a misnomer for a modern feedforward artificial neural network, consisting of fully connected neurons with a nonlinear kind of activation function, organized in at least three layers, notable for being able to distinguish data that is not linearly separable. It is a misnomer because the original perceptron used a Heaviside step function, instead of a nonlinear kind of activation function (used by modern networks).

Other feedforward networks

Examples of other feedforward networks include convolutional neural networks and radial basis function networks, which use a different activation function.