Curvature

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Curvature

 

In mathematics, curvature is any of several strongly related concepts in geometry. Intuitively, the curvature is the amount by which a curve deviates from being a straight line, or a surface deviates from being a plane.

For curves, the canonical example is that of a circle, which has a curvature equal to the reciprocal of its radius. Smaller circles bend more sharply, and hence have higher curvature. The curvature at a point of a differentiable curve, is the curvature of its osculating circle, that is the circle that best approximates the curve near this point. The curvature of a straight line is zero. The curvature of a curve at a point is normally a scalar quantity, that is, it is expressed by a single real number.

For surfaces (and, more generally for higher-dimensional manifolds), that are embedded in a Euclidean space, the concept of curvature is more complex, as depending on the choice of a direction on the surface or manifold. This leads to the concepts of maximal curvature, minimal curvature, and mean curvature.

For Riemannian manifolds (of dimension at least two) that are not necessarily embedded in a Euclidean space, one can define the curvature intrinsically, that is without referring to an external space. See Curvature of Riemannian manifolds for the definition, which is done in terms of lengths of curves traced on the manifold, and expressed, using linear algebra, by the Riemann curvature tensor. 

History

The curvature of a differentiable curve was originally defined through osculating circles. In this setting, Augustin-Louis Cauchy showed that the center of curvature is the intersection point of two infinitely close normal lines to the curve.

Plane curves

Intuitively, the curvature is a measure of the instantaneous rate of change of direction of a point that moves on the curve: the larger the curvature, the larger this rate of change. In other words, the curvature measures how fast the unit tangent vector to the curve rotates. In fact, it can be proved that this instantaneous rate of change is exactly the curvature. More precisely, suppose that the point is moving on the curve at a constant speed of one unit, that is, the position of the point P(s) is a function of the parameter s, which may be thought as the time or as the arc length from a given origin. Let T(s) be a unit tangent vector of the curve at P(s), which is also the derivative of P(s) with respect to s. Then, the derivative of T(s) with respect to s is a vector that is normal to the curve and whose length is the curvature.

For being meaningful, the definition of the curvature and its different characterizations require that the curve is continuously differentiable near P, for having a tangent that varies continuously; it requires also that the curve is twice differentiable at P, for insuring the existence of the involved limits, and of the derivative of T(s).

The characterization of the curvature in terms of the derivative of the unit tangent vector is probably less intuitive than the definition in terms of the osculating circle, but formulas for computing the curvature are easier to deduce. Therefore, and also because of its use in kinematics, this characterization is often given as a definition of the curvature.

Osculating circle

Historically, the curvature of a differentiable curve was defined through the osculating circle, which is the circle that best approximates the curve at a point. More precisely, given a point P on a curve, every other point Q of the curve defines a circle (or sometimes a line) passing through Q and tangent to the curve at P. The osculating circle is the limit, if it exists, of this circle when Q tends to P. Then the center and the radius of curvature of the curve at P are the center and the radius of the osculating circle. The curvature is the reciprocal of radius of curvature. That is, the curvature is

${\displaystyle \kappa ={\frac {1}{R}},}$

where R is the radius of curvature.

This definition is difficult to manipulate and to express in formulas. Therefore, other equivalent definitions have been introduced. 

In terms of arc-length parametrization

Every differentiable curve can be parametrized with respect to arc length. In the case of a plane curve, this means the existence of a parametrization γ(s) = (x(s), y(s)), where x and y are real-valued differentiable functions whose derivatives verify

${\displaystyle \|{\boldsymbol {\gamma }}'\|={\sqrt {x'(s)^{2}+y'(s)^{2}}}=1.}$

This means that the tangent vector

${\displaystyle \mathbf {T} (s)=(x'(s),y'(s))}$

has a norm equal to one and is thus a unit tangent vector.

If the curve is twice differentiable, that is, if the second derivatives of x and y exist, then the derivative of T(s) exists. This vector is normal to the curve, its norm is the curvature κ(s), and it is oriented toward the center of curvature. That is,

${\displaystyle {\begin{aligned}&\mathbf {T} (s)={\boldsymbol {\gamma }}'(s),\\&\mathbf {T} '(s)\cdot \mathbf {T} (s)=0\\&\kappa (s)=\|\mathbf {T} '(s)\|=\|{\boldsymbol {\gamma }}''(s)\|={\sqrt {x''(s)^{2}+y''(s)^{2}}}\\\end{aligned}}}$

Moreover, as the radius of curvature is ${\displaystyle R(s)={\frac {1}{\kappa (s)}},}$ and the center of curvature is on the normal to the curve, the center of curvature is the point

${\displaystyle C(s)={\frac {1}{\kappa (s)^{2}}}\mathbf {T} '(s).}$

If N(s) is the unit normal vector obtained from T(s) by a counterclockwise rotation of π / 2, then

${\displaystyle \mathbf {T} '(s)=k(s)\mathbf {N} (s),}$

with k(s) = ± κ(s). The real number k(s) is called the oriented or signed curvature. It depends on both the orientation of the plane (definition of counterclockwise), and the orientation of the curve provided by the parametrization. In fact, the change of variable s → –s provides another arc-length parametrization, and changes the sign of k(s).

In terms of a general parametrization

Let γ(t) = (x(t), y(t)) be a proper parametric representation of a twice differentiable plane curve. Here proper means that on the domain of definition of the parametrization, the derivative ${\displaystyle \textstyle {\frac {d{\boldsymbol {\gamma }}}{dt}}}$ is defined, differentiable and nowhere equal to the zero vector.

With such a parametrization, the signed curvature is

${\displaystyle k={\frac {x'y''-y'x''}{\left({x'}^{2}+{y'}^{2}\right)^{\frac {3}{2}}}},}$

where primes refer to derivatives with respect to t. The curvature κ is thus

${\displaystyle \kappa ={\frac {|x'y''-y'x''|}{\left({x'}^{2}+{y'}^{2}\right)^{\frac {3}{2}}}}.}$

These can be expressed in a coordinate-free way as

${\displaystyle k={\frac {\det({\boldsymbol {\gamma }}',{\boldsymbol {\gamma }}'')}{\|{\boldsymbol {\gamma }}'\|^{3}}},\qquad \kappa ={\frac {|\det({\boldsymbol {\gamma }}',{\boldsymbol {\gamma }}'')|}{\|{\boldsymbol {\gamma }}'\|^{3}}}.}$

These formulas can be derived from the special case of arc-length parametrization in the following way. The above condition on the parametrisation imply that the arc length s is a differentiable monotonic function of the parameter t, and conversely that t is a monotonic function of s. Moreover, by changing, if needed, s to –s, one may suppose that these functions are increasing and have a positive derivative. Using notation of the preceding section and the chain rule, one has

${\displaystyle {\frac {d{\boldsymbol {\gamma }}}{dt}}={\frac {ds}{dt}}\mathbf {T} ,}$

and thus, by taking the norm of both sides

${\displaystyle {\frac {dt}{ds}}={\frac {1}{\|{\boldsymbol {\gamma }}'\|}},}$

where the prime denotes the derivation with respect to t. 

The curvature is the norm of the derivative of T with respect to s. By using the above formula and the chain rule this derivative and its norm can be expressed in terms of ${\displaystyle {\boldsymbol {\gamma }}'}$ and ${\displaystyle {\boldsymbol {\gamma }}''}$ only, with the arc-length parameter s completely eliminated, giving the above formulas for the curvature.

Graph of a function

The graph of a function y = f(x), is a special case of a parametrized curve, of the form

${\displaystyle {\begin{aligned}x&=t\\y&=f(t).\end{aligned}}}$

As the first and second derivatives of x are 1 and 0, previous formulas simplify to

${\displaystyle \kappa ={\frac {|y''|}{\left(1+{y'}^{2}\right)^{\frac {3}{2}}}},}$

for the curvature, and to

${\displaystyle k={\frac {y''}{\left(1+{y'}^{2}\right)^{\frac {3}{2}}}},}$

for the signed curvature. 

In the general case of a curve, the sign of the signed curvature is somehow arbitrary, as depending on an orientation of the curve. In the case of the graph of a function, there is a natural orientation by increasing values of x. This makes significant the sign of the signed curvature.

The sign of the signed curvature is the same as the sign of the second derivative of f. If it is positive then the graph has an upward concavity, and, if it is negative the graph has a downward concavity. It is zero, then one has an inflection point or an undulation point.

When the slope of the graph (that is the derivative of the function) is small, the signed curvature is well approximated by the second derivative. More precisely, using big O notation, one has

${\displaystyle k(x)=y''+O(y'^{2}).}$

It is common in physics and engineering to approximate the curvature with the second derivative, for example, in beam theory or for deriving wave equation of a tense string, and other applications where small slopes are involved. This allows often considering as linear systems that are nonlinear otherwise. 

Polar coordinates

If a curve is defined in polar coordinates by the radius expressed as a function of the polar angle, that is r is a function of θ, then its curvature is

${\displaystyle \kappa (\theta )={\frac {\left|r^{2}+2{r'}^{2}-r\,r''\right|}{\left(r^{2}+{r'}^{2}\right)^{\frac {3}{2}}}}}$

where the prime refers to differentiation with respect to θ. 

This results from the formula for general parametrizations, by considering the parametrization

${\displaystyle {\begin{aligned}x&=r(\theta )\cos \theta \\y&=r(\theta )\sin \theta \end{aligned}}}$

Implicit curve

For a curve defined by an implicit equation F(x, y) = 0 with partial derivatives denoted F_x, F_y, F_xx, F_xy, F_yy, the curvature is given by

${\displaystyle \kappa ={\frac {\left|F_{y}^{2}F_{xx}-2F_{x}F_{y}F_{xy}+F_{x}^{2}F_{yy}\right|}{\left(F_{x}^{2}+F_{y}^{2}\right)^{\frac {3}{2}}}}.}$

The signed curvature is not defined, as it depends on an orientation of the curve that is not provided by the implicit equation. Also, changing F into –F does not change the curve, but changes the sign of the numerator if the absolute value is omitted in the preceding formula.

A point of the curve where F_x = F_y = 0 is a singular point, which means that the curve in not differentiable at this point, and thus that the curvature is not defined (most often, the point is either a crossing point or a cusp). 

Above formula for the curvature can be derived from the expression of the curvature of the graph of a function by using the implicit function theorem and the fact that, on such a curve, one has

${\displaystyle {\frac {dy}{dx}}=-{\frac {F_{x}}{F_{y}}}.}$

Examples

It can be useful to verify on simple examples that the different formulas given in the preceding sections give the same result.

Circle

A common parametrization of a circle of radius r is γ(t) = (r cos t, r sin t). The formula for the curvature gives

${\displaystyle k(t)={\frac {r^{2}\sin ^{2}t+r^{2}\cos ^{2}t}{{\sqrt {r^{2}\cos ^{2}t+r^{2}\sin ^{2}t}}^{3/2}}}={\frac {1}{r}}.}$

It follows, as expected, that the radius of curvature is the radius of the circle, and that the center of curvature is the center of the circle.

The circle is a rare case where the arc-length parametrization is easy to compute, as it is ${\displaystyle {\boldsymbol {\gamma }}(s)=\left(r\cos {\frac {s}{r}},r\sin {\frac {s}{r}}\right).}$ It is an arc-length parametrization, since the norm of ${\displaystyle {\boldsymbol {\gamma }}(s)=\left(\cos {\frac {s}{r}},\sin {\frac {s}{r}}\right)}$ is equal to one. This parametrization gives the same value for the curvature, as it amounts to divides by ${\displaystyle r^{3}}$ both the numerator and the denominator in the preceding formula.

The same circle can also be defined by the implicit equation F(x, y) = 0 with F(x, y) = x² + y² – r². Then, the formula for the curvature in this case gives

${\displaystyle {\begin{aligned}\kappa &={\frac {\left|F_{y}^{2}F_{xx}-2F_{x}F_{y}F_{xy}+F_{x}^{2}F_{yy}\right|}{\left(F_{x}^{2}+F_{y}^{2}\right)^{\frac {3}{2}}}}\\&={\frac {8y^{2}+8x^{2}}{\left(4x^{2}+4y^{2}\right)^{\frac {3}{2}}}}\\&={\frac {8r^{2}}{(4r^{2})^{\frac {3}{2}}}}={\frac {1}{r}}.\end{aligned}}}$

Parabola

Consider the parabola y = ax² + bx + c. 

It is the graph of a function, with derivative 2ax + b, and second derivative 2a. So, the signed curvature is

${\displaystyle k(x)={\frac {2a}{\left(1+(2ax+b)^{2}\right)^{\frac {3}{2}}}}.}$

It has the sign of a for all values of x. This means that, if a > 0, the concavity is upward directed everywhere; if a < 0, the concavity is downward directed; for a = 0, the curvature is zero everywhere, confirming that the parabola degenerates into a line in this case. 

The (unsigned) curvature is maximal for x = –b / 2a, that is at the stationary point (zero derivative) of the function, which is the vertex of the parabola.

Consider the parametrization γ(t) = (t, at² + bt + c) = (x, y). The first derivative of x is 1, and the second derivative is zero. Substituting into the formula for general parametrizations gives exactly the same result as above, with x replaced by tIf we use primes for derivatives with respect to the parameter t.

The same parabola can also be defined by the implicit equation F(x, y) = 0 with F(x, y) = ax² + bx + c – y. As F_y = –1, and F_yy = F_xy = 0, one obtains exactly the same value for the (unsigned) curvature. However, the signed curvature is meaningless here, as –F(x, y) = 0 is a valid implicit equation for the same parabola, which gives the opposite sign for the curvature. 

Frenet–Serret formulas for plane curves

The vectors T and N at two points on a plane curve, a translated version of the second frame (dotted), and the change in T: δT. δs is the distance between the points. In the limit dTds will be in the direction N and the curvature describes the speed of rotation of the frame.

 

The expression of the curvature In terms of arc-length parametrization is essentially the first Frenet–Serret formula

${\displaystyle \mathbf {T} '(s)=\kappa (s)\mathbf {N} (s),}$

where the primes refer to the derivatives with respect to the arc length s, and N(s) is the normal unit vector in the direction of T'(s).

As planar curves have zero torsion, the second Frenet–Serret formula provides the relation

${\displaystyle {\begin{aligned}{\frac {d\mathbf {N} }{ds}}&=-\kappa \mathbf {T} ,\\&=-\kappa {\frac {d{\boldsymbol {\gamma }}}{ds}}.\end{aligned}}}$

For a general parametrization by a parameter t, one needs expressions involving derivatives with respect to t. As these are obtained by multiplying by dsdt the derivatives with respect to s, one has, for any proper parametrization

${\displaystyle \mathbf {N} '(t)=-\kappa (t){\boldsymbol {\gamma }}'(t).}$

Space curves

Animation of the curvature and the acceleration vector T′(s)

As in the case of curves in two dimensions, the curvature of a regular space curve C in three dimensions (and higher) is the magnitude of the acceleration of a particle moving with unit speed along a curve. Thus if γ(s) is the arc-length parametrization of C then the unit tangent vector T(s) is given by

${\displaystyle \mathbf {T} (s)={\boldsymbol {\gamma }}'(s)}$

and the curvature is the magnitude of the acceleration:

${\displaystyle \kappa (s)=\|\mathbf {T} '(s)\|=\|{\boldsymbol {\gamma }}''(s)\|.}$

The direction of the acceleration is the unit normal vector N(s), which is defined by

${\displaystyle \mathbf {N} (s)={\frac {\mathbf {T} '(s)}{\|\mathbf {T} '(s)\|}}.}$

The plane containing the two vectors T(s) and N(s) is the osculating plane to the curve at γ(s). The curvature has the following geometrical interpretation. There exists a circle in the osculating plane tangent to γ(s) whose Taylor series to second order at the point of contact agrees with that of γ(s). This is the osculating circle to the curve. The radius of the circle R(s) is called the radius of curvature, and the curvature is the reciprocal of the radius of curvature:

${\displaystyle \kappa (s)={\frac {1}{R(s)}}.}$

The tangent, curvature, and normal vector together describe the second-order behavior of a curve near a point. In three-dimensions, the third order behavior of a curve is described by a related notion of torsion, which measures the extent to which a curve tends to move in a helical path in space. The torsion and curvature are related by the Frenet–Serret formulas (in three dimensions) and their generalization (in higher dimensions). 

General expressions

For a parametrically-defined space curve in three dimensions given in Cartesian coordinates by γ(t) = (x(t), y(t), z(t)), the curvature is

${\displaystyle \kappa ={\frac {\sqrt {\left(z''y'-y''z'\right)^{2}+\left(x''z'-z''x'\right)^{2}+\left(y''x'-x''y'\right)^{2}}}{\left({x'}^{2}+{y'}^{2}+{z'}^{2}\right)^{\frac {3}{2}}}},}$

where the prime denotes differentiation with respect to the parameter t. This can be expressed independently of the coordinate system by means of the formula

${\displaystyle \kappa ={\frac {\|{\boldsymbol {\gamma }}'\times {\boldsymbol {\gamma }}''\|}{\|{\boldsymbol {\gamma }}'\|^{3}}}}$

where × denotes the vector cross product. Equivalently,

${\displaystyle \kappa ={\frac {\sqrt {\det \left(\left({\boldsymbol {\gamma }}'\times {\boldsymbol {\gamma }}''\right)^{\mathsf {T}}(\gamma '\times \gamma '')\right)}}{\|{\boldsymbol {\gamma }}'\|^{3}}}={\frac {\sqrt {\|{\boldsymbol {\gamma }}'\|^{2}\|{\boldsymbol {\gamma }}''\|^{2}-\left({\boldsymbol {\gamma }}'\cdot {\boldsymbol {\gamma }}''\right)^{2}}}{\|{\boldsymbol {\gamma }}'\|^{3}}}.}$

Here the T denotes the matrix transpose. This last formula (without cross product) is also valid for the curvature of curves in a Euclidean space of any dimension. 

Curvature from arc and chord length

Given two points P and Q on C, let s(P,Q) be the arc length of the portion of the curve between P and Q and let d(P,Q) denote the length of the line segment from P to Q. The curvature of C at P is given by the limit

${\displaystyle \kappa (P)=\lim _{Q\to P}{\sqrt {\frac {24{\big (}s(P,Q)-d(P,Q){\big )}}{s(P,Q)^{3}}}}}$

where the limit is taken as the point Q approaches P on C. The denominator can equally well be taken to be d(P,Q)³. The formula is valid in any dimension. Furthermore, by considering the limit independently on either side of P, this definition of the curvature can sometimes accommodate a singularity at P. The formula follows by verifying it for the osculating circle.

Surfaces

The curvature of curves drawn on a surface is the main tool for the defining and studying the curvature of the surface.

Curves on surfaces

For a curve drawn on a surface (embedded in three-dimensional Euclidean space), several curvatures are defined, which relates the direction of curvature to the surface's unit normal vector. These are the normal curvature, geodesic curvature and geodesic torsion. Any non-singular curve on a smooth surface has its tangent vector T contained in the tangent plane of the surface. The normal curvature, k_n, is the curvature of the curve projected onto the plane containing the curve's tangent T and the surface normal u; the geodesic curvature, k_g, is the curvature of the curve projected onto the surface's tangent plane; and the geodesic torsion (or relative torsion), τ_r, measures the rate of change of the surface normal around the curve's tangent.

Let the curve be arc-length parametrized, and let t = u × T so that T, t, u form an orthonormal basis, called the Darboux frame. The above quantities are related by:

${\displaystyle {\begin{pmatrix}\mathbf {T} '\\\mathbf {t} '\\\mathbf {u} '\end{pmatrix}}={\begin{pmatrix}0&\kappa _{\mathrm {g} }&\kappa _{\mathrm {n} }\\-\kappa _{\mathrm {g} }&0&\tau _{\mathrm {r} }\\-\kappa _{\mathrm {n} }&-\tau _{\mathrm {r} }&0\end{pmatrix}}{\begin{pmatrix}\mathbf {T} \\\mathbf {t} \\\mathbf {u} \end{pmatrix}}}$

Principal curvature

Saddle surface with normal planes in directions of principal curvatures

All curves with the same tangent vector will have the same normal curvature, which is the same as the curvature of the curve obtained by intersecting the surface with the plane containing T and u. Taking all possible tangent vectors, the maximum and minimum values of the normal curvature at a point are called the principal curvatures, k₁ and k₂, and the directions of the corresponding tangent vectors are called principal normal directions. 

Normal sections

Curvature can be evaluated along surface normal sections, similar to § Curves on surfaces above (see for example the Earth radius of curvature). 

Gaussian curvature

In contrast to curves, which do not have intrinsic curvature, but do have extrinsic curvature (they only have a curvature given an embedding), surfaces can have intrinsic curvature, independent of an embedding. The Gaussian curvature, named after Carl Friedrich Gauss, is equal to the product of the principal curvatures, k₁k₂. It has a dimension of length⁻² and is positive for spheres, negative for one-sheet hyperboloids and zero for planes. It determines whether a surface is locally convex (when it is positive) or locally saddle-shaped (when it is negative).

Gaussian curvature is an intrinsic property of the surface, meaning it does not depend on the particular embedding of the surface; intuitively, this means that ants living on the surface could determine the Gaussian curvature. For example, an ant living on a sphere could measure the sum of the interior angles of a triangle and determine that it was greater than 180 degrees, implying that the space it inhabited had positive curvature. On the other hand, an ant living on a cylinder would not detect any such departure from Euclidean geometry; in particular the ant could not detect that the two surfaces have different mean curvatures (see below), which is a purely extrinsic type of curvature.

Formally, Gaussian curvature only depends on the Riemannian metric of the surface. This is Gauss's celebrated Theorema Egregium, which he found while concerned with geographic surveys and mapmaking.

An intrinsic definition of the Gaussian curvature at a point P is the following: imagine an ant which is tied to P with a short thread of length r. It runs around P while the thread is completely stretched and measures the length C(r) of one complete trip around P. If the surface were flat, the ant would find C(r) = 2πr. On curved surfaces, the formula for C(r) will be different, and the Gaussian curvature K at the point P can be computed by the Bertrand–Diguet–Puiseux theorem as

${\displaystyle K=\lim _{r\to 0^{+}}3\left({\frac {2\pi r-C(r)}{\pi r^{3}}}\right).}$

The integral of the Gaussian curvature over the whole surface is closely related to the surface's Euler characteristic; see the Gauss–Bonnet theorem. 

The discrete analog of curvature, corresponding to curvature being concentrated at a point and particularly useful for polyhedra, is the (angular) defect; the analog for the Gauss–Bonnet theorem is Descartes' theorem on total angular defect.

Because (Gaussian) curvature can be defined without reference to an embedding space, it is not necessary that a surface be embedded in a higher-dimensional space in order to be curved. Such an intrinsically curved two-dimensional surface is a simple example of a Riemannian manifold.

Mean curvature

The mean curvature is an extrinsic measure of curvature equal to half the sum of the principal curvatures, k₁ + k₂2. It has a dimension of length⁻¹. Mean curvature is closely related to the first variation of surface area. In particular, a minimal surface such as a soap film has mean curvature zero and a soap bubble has constant mean curvature. Unlike Gauss curvature, the mean curvature is extrinsic and depends on the embedding, for instance, a cylinder and a plane are locally isometric but the mean curvature of a plane is zero while that of a cylinder is nonzero.

Second fundamental form

The intrinsic and extrinsic curvature of a surface can be combined in the second fundamental form. This is a quadratic form in the tangent plane to the surface at a point whose value at a particular tangent vector X to the surface is the normal component of the acceleration of a curve along the surface tangent to X; that is, it is the normal curvature to a curve tangent to X (see above). Symbolically,

${\displaystyle \operatorname {I\!I} (\mathbf {X} ,\mathbf {X} )=\mathbf {N} \cdot (\nabla _{\mathbf {X} }\mathbf {X} )}$

where N is the unit normal to the surface. For unit tangent vectors X, the second fundamental form assumes the maximum value k₁ and minimum value k₂, which occur in the principal directions u₁ and u₂, respectively. Thus, by the principal axis theorem, the second fundamental form is

${\displaystyle \operatorname {I\!I} (\mathbf {X} ,\mathbf {X} )=k_{1}\left(\mathbf {X} \cdot \mathbf {u} _{1}\right)^{2}+k_{2}\left(\mathbf {X} \cdot \mathbf {u} _{2}\right)^{2}.}$

Thus the second fundamental form encodes both the intrinsic and extrinsic curvatures.

Shape operator

An encapsulation of surface curvature can be found in the shape operator, S, which is a self-adjoint linear operator from the tangent plane to itself (specifically, the differential of the Gauss map). 

For a surface with tangent vectors X and normal N, the shape operator can be expressed compactly in index summation notation as

${\displaystyle \partial _{a}\mathbf {N} =-S_{ba}\mathbf {X} _{b}.}$

(Compare the alternative expression of curvature for a plane curve.)

The Weingarten equations give the value of S in terms of the coefficients of the first and second fundamental forms as

${\displaystyle S=\left(EG-F^{2}\right)^{-1}{\begin{pmatrix}eG-fF&fG-gF\\fE-eF&gE-fF\end{pmatrix}}.}$

The principal curvatures are the eigenvalues of the shape operator, the principal curvature directions are its eigenvectors, the Gauss curvature is its determinant, and the mean curvature is half its trace. 

Curvature of space

By extension of the former argument, a space of three or more dimensions can be intrinsically curved. The curvature is intrinsic in the sense that it is a property defined at every point in the space, rather than a property defined with respect to a larger space that contains it. In general, a curved space may or may not be conceived as being embedded in a higher-dimensional ambient space; if not then its curvature can only be defined intrinsically. 

After the discovery of the intrinsic definition of curvature, which is closely connected with non-Euclidean geometry, many mathematicians and scientists questioned whether ordinary physical space might be curved, although the success of Euclidean geometry up to that time meant that the radius of curvature must be astronomically large. In the theory of general relativity, which describes gravity and cosmology, the idea is slightly generalised to the "curvature of spacetime"; in relativity theory spacetime is a pseudo-Riemannian manifold. Once a time coordinate is defined, the three-dimensional space corresponding to a particular time is generally a curved Riemannian manifold; but since the time coordinate choice is largely arbitrary, it is the underlying spacetime curvature that is physically significant. 

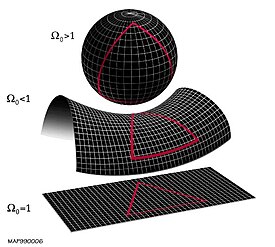
Although an arbitrarily curved space is very complex to describe, the curvature of a space which is locally isotropic and homogeneous is described by a single Gaussian curvature, as for a surface; mathematically these are strong conditions, but they correspond to reasonable physical assumptions (all points and all directions are indistinguishable). A positive curvature corresponds to the inverse square radius of curvature; an example is a sphere or hypersphere. An example of negatively curved space is hyperbolic geometry. A space or space-time with zero curvature is called flat. For example, Euclidean space is an example of a flat space, and Minkowski space is an example of a flat spacetime. There are other examples of flat geometries in both settings, though. A torus or a cylinder can both be given flat metrics, but differ in their topology. Other topologies are also possible for curved space. See also shape of the universe. 

Generalizations

Parallel transporting a vector from A → N → B → A yields a different vector. This failure to return to the initial vector is measured by the holonomy of the surface.

 

The mathematical notion of curvature is also defined in much more general contexts. Many of these generalizations emphasize different aspects of the curvature as it is understood in lower dimensions.

One such generalization is kinematic. The curvature of a curve can naturally be considered as a kinematic quantity, representing the force felt by a certain observer moving along the curve; analogously, curvature in higher dimensions can be regarded as a kind of tidal force (this is one way of thinking of the sectional curvature). This generalization of curvature depends on how nearby test particles diverge or converge when they are allowed to move freely in the space; see Jacobi field.

Another broad generalization of curvature comes from the study of parallel transport on a surface. For instance, if a vector is moved around a loop on the surface of a sphere keeping parallel throughout the motion, then the final position of the vector may not be the same as the initial position of the vector. This phenomenon is known as holonomy.^[7] Various generalizations capture in an abstract form this idea of curvature as a measure of holonomy; see curvature form. A closely related notion of curvature comes from gauge theory in physics, where the curvature represents a field and a vector potential for the field is a quantity that is in general path-dependent: it may change if an observer moves around a loop.

Two more generalizations of curvature are the scalar curvature and Ricci curvature. In a curved surface such as the sphere, the area of a disc on the surface differs from the area of a disc of the same radius in flat space. This difference (in a suitable limit) is measured by the scalar curvature. The difference in area of a sector of the disc is measured by the Ricci curvature. Each of the scalar curvature and Ricci curvature are defined in analogous ways in three and higher dimensions. They are particularly important in relativity theory, where they both appear on the side of Einstein's field equations that represents the geometry of spacetime (the other side of which represents the presence of matter and energy). These generalizations of curvature underlie, for instance, the notion that curvature can be a property of a measure; see curvature of a measure.

Another generalization of curvature relies on the ability to compare a curved space with another space that has constant curvature. Often this is done with triangles in the spaces. The notion of a triangle makes senses in metric spaces, and this gives rise to CAT(k) spaces.

Shape of the universe (updated)

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Shape_of_the_universe

The shape of the universe is the local and global geometry of the universe. The local features of the geometry of the universe are primarily described by its curvature, whereas the topology of the universe describes general global properties of its shape as of a continuous object. The shape of the universe is related to general relativity, which describes how spacetime is curved and bent by mass and energy. 

Cosmologists distinguish between the observable universe and the global universe. The observable universe consists of the part of the universe that can, in principle, be observed by light reaching Earth within the age of the universe. It encompasses a region of space that currently forms a ball centered at Earth of estimated radius 46.5 billion light-years (4.40×10²⁶ m). This does not mean the universe is 46.5 billion years old; instead the universe is measured to be 13.8 billion years old, but space itself has also expanded, causing the size of the observable universe to be larger than the distance traversible by light over the duration of its current age. Assuming an isotropic nature, the observable universe is similar for all contemporary vantage points.

The global shape of the universe can be described with three attributes:

1. Finite or infinite
2. Flat (no curvature), open (negative curvature), or closed (positive curvature)
3. Connectivity, how the universe is put together, i.e., simply connected space or multiply connected.

There are certain logical connections among these properties. For example, a universe with positive curvature is necessarily finite. Although it is usually assumed in the literature that a flat or negatively curved universe is infinite, this need not be the case if the topology is not the trivial one: for example, a three-torus is flat but finite.

The exact shape is still a matter of debate in physical cosmology, but experimental data from various independent sources (WMAP, BOOMERanG, and Planck for example) confirm that the universe is flat with only a 0.4% margin of error. Theorists have been trying to construct a formal mathematical model of the shape of the universe. In formal terms, this is a 3-manifold model corresponding to the spatial section (in comoving coordinates) of the 4-dimensional spacetime of the universe. The model most theorists currently use is the Friedmann–Lemaître–Robertson–Walker (FLRW) model. Arguments have been put forward that the observational data best fit with the conclusion that the shape of the global universe is infinite and flat, but the data are also consistent with other possible shapes, such as the so-called Poincaré dodecahedral space and the Sokolov–Starobinskii space (quotient of the upper half-space model of hyperbolic space by 2-dimensional lattice).

 

 

Shape of the observable universe

As stated in the introduction, there are two aspects to consider:

1. its local geometry, which predominantly concerns the curvature of the universe, particularly the observable universe, and
2. its global geometry, which concerns the topology of the universe as a whole.

The observable universe can be thought of as a sphere that extends outwards from any observation point for 46.5 billion light years, going farther back in time and more redshifted the more distant away one looks. Ideally, one can continue to look back all the way to the Big Bang; in practice, however, the farthest away one can look using light and other electromagnetic radiation is the cosmic microwave background (CMB), as anything past that was opaque. Experimental investigations show that the observable universe is very close to isotropic and homogeneous. 

If the observable universe encompasses the entire universe, we may be able to determine the structure of the entire universe by observation. However, if the observable universe is smaller than the entire universe, our observations will be limited to only a part of the whole, and we may not be able to determine its global geometry through measurement. From experiments, it is possible to construct different mathematical models of the global geometry of the entire universe, all of which are consistent with current observational data; thus it is currently unknown whether the observable universe is identical to the global universe, or is instead many orders of magnitude smaller. The universe may be small in some dimensions and not in others (analogous to the way a cuboid is longer in the dimension of length than it is in the dimensions of width and depth). To test whether a given mathematical model describes the universe accurately, scientists look for the model's novel implications—what are some phenomena in the universe that we have not yet observed, but that must exist if the model is correct—and they devise experiments to test whether those phenomena occur or not. For example, if the universe is a small closed loop, one would expect to see multiple images of an object in the sky, although not necessarily images of the same age. 

Cosmologists normally work with a given space-like slice of spacetime called the comoving coordinates, the existence of a preferred set of which is possible and widely accepted in present-day physical cosmology. The section of spacetime that can be observed is the backward light cone (all points within the cosmic light horizon, given time to reach a given observer), while the related term Hubble volume can be used to describe either the past light cone or comoving space up to the surface of last scattering. To speak of "the shape of the universe (at a point in time)" is ontologically naive from the point of view of special relativity alone: due to the relativity of simultaneity we cannot speak of different points in space as being "at the same point in time" nor, therefore, of "the shape of the universe at a point in time". However, the comoving coordinates (if well-defined) provide a strict sense to those by using the time since the Big Bang (measured in the reference of CMB) as a distinguished universal time. 

Curvature of the universe

The curvature is a quantity describing how the geometry of a space differs locally from the one of the flat space. The curvature of any locally isotropic space (and hence of a locally isotropic universe) falls into one of the three following cases:

1. Zero curvature (flat); a drawn triangle's angles add up to 180° and the Pythagorean theorem holds; such 3-dimensional space is locally modeled by Euclidean space E³.
2. Positive curvature; a drawn triangle's angles add up to more than 180°; such 3-dimensional space is locally modeled by a region of a 3-sphere S³.
3. Negative curvature; a drawn triangle's angles add up to less than 180°; such 3-dimensional space is locally modeled by a region of a hyperbolic space H³.

Curved geometries are in the domain of Non-Euclidean geometry. An example of a positively curved space would be the surface of a sphere such as the Earth. A triangle drawn from the equator to a pole will have at least two angles equal 90°, which makes the sum of the 3 angles greater than 180°. An example of a negatively curved surface would be the shape of a saddle or mountain pass. A triangle drawn on a saddle surface will have the sum of the angles adding up to less than 180°.

The local geometry of the universe is determined by whether the density parameter Ω is greater than, less than, or equal to 1.
From top to bottom: a spherical universe with Ω > 1, a hyperbolic universe with Ω < 1, and a flat universe with Ω = 1. These depictions of two-dimensional surfaces are merely easily visualizable analogs to the 3-dimensional structure of (local) space.

 

General relativity explains that mass and energy bend the curvature of spacetime and is used to determine what curvature the universe has by using a value called the density parameter, represented with Omega (Ω). The density parameter is the average density of the universe divided by the critical energy density, that is, the mass energy needed for a universe to be flat. Put another way,

• If Ω = 1, the universe is flat
• If Ω > 1, there is positive curvature
• if Ω < 1 there is negative curvature

One can experimentally calculate this Ω to determine the curvature two ways. One is to count up all the mass-energy in the universe and take its average density then divide that average by the critical energy density. Data from Wilkinson Microwave Anisotropy Probe (WMAP) as well as the Planck spacecraft give values for the three constituents of all the mass-energy in the universe – normal mass (baryonic matter and dark matter), relativistic particles (photons and neutrinos), and dark energy or the cosmological constant:

Ω_mass ≈ 0.315±0.018

Ω_relativistic ≈ 9.24×10⁻⁵

Ω_Λ ≈ 0.6817±0.0018

Ω_total= Ω_mass + Ω_relativistic + Ω_Λ= 1.00±0.02

The actual value for critical density value is measured as ρ_critical= 9.47×10⁻²⁷ kg m⁻³. From these values, within experimental error, the universe seems to be flat.

Another way to measure Ω is to do so geometrically by measuring an angle across the observable universe. We can do this by using the CMB and measuring the power spectrum and temperature anisotropy. For an intuition, one can imagine finding a gas cloud that is not in thermal equilibrium due to being so large that light speed cannot propagate the thermal information. Knowing this propagation speed, we then know the size of the gas cloud as well as the distance to the gas cloud, we then have two sides of a triangle and can then determine the angles. Using a method similar to this, the BOOMERanG experiment has determined that the sum of the angles to 180° within experimental error, corresponding to an Ω_total ≈ 1.00±0.12.

These and other astronomical measurements constrain the spatial curvature to be very close to zero, although they do not constrain its sign. This means that although the local geometries of spacetime are generated by the theory of relativity based on spacetime intervals, we can approximate 3-space by the familiar Euclidean geometry. 

The Friedmann–Lemaître–Robertson–Walker (FLRW) model using Friedmann equations is commonly used to model the universe. The FLRW model provides a curvature of the universe based on the mathematics of fluid dynamics, that is, modeling the matter within the universe as a perfect fluid. Although stars and structures of mass can be introduced into an "almost FLRW" model, a strictly FLRW model is used to approximate the local geometry of the observable universe. Another way of saying this is that if all forms of dark energy are ignored, then the curvature of the universe can be determined by measuring the average density of matter within it, assuming that all matter is evenly distributed (rather than the distortions caused by 'dense' objects such as galaxies). This assumption is justified by the observations that, while the universe is "weakly" inhomogeneous and anisotropic (see the large-scale structure of the cosmos), it is on average homogeneous and isotropic. 

Global universe structure

Global structure covers the geometry and the topology of the whole universe—both the observable universe and beyond. While the local geometry does not determine the global geometry completely, it does limit the possibilities, particularly a geometry of a constant curvature. The universe is often taken to be a geodesic manifold, free of topological defects; relaxing either of these complicates the analysis considerably. A global geometry is a local geometry plus a topology. It follows that a topology alone does not give a global geometry: for instance, Euclidean 3-space and hyperbolic 3-space have the same topology but different global geometries.

As stated in the introduction, investigations within the study of the global structure of the universe include:

• Whether the universe is infinite or finite in extent
• Whether the geometry of the global universe is flat, positively curved, or negatively curved
• Whether the topology is simply connected like a sphere or multiply connected, like a torus

Infinite or finite

One of the presently unanswered questions about the universe is whether it is infinite or finite in extent. For intuition, it can be understood that a finite universe has a finite volume that, for example, could be in theory filled up with a finite amount of material, while an infinite universe is unbounded and no numerical volume could possibly fill it. Mathematically, the question of whether the universe is infinite or finite is referred to as boundedness. An infinite universe (unbounded metric space) means that there are points arbitrarily far apart: for any distance d, there are points that are of a distance at least d apart. A finite universe is a bounded metric space, where there is some distance d such that all points are within distance d of each other. The smallest such d is called the diameter of the universe, in which case the universe has a well-defined "volume" or "scale." 

With or without boundary

Assuming a finite universe, the universe can either have an edge or no edge. Many finite mathematical spaces, e.g., a disc, have an edge or boundary. Spaces that have an edge are difficult to treat, both conceptually and mathematically. Namely, it is very difficult to state what would happen at the edge of such a universe. For this reason, spaces that have an edge are typically excluded from consideration. 

However, there exist many finite spaces, such as the 3-sphere and 3-torus, which have no edges. Mathematically, these spaces are referred to as being compact without boundary. The term compact basically means that it is finite in extent ("bounded") and complete. The term "without boundary" means that the space has no edges. Moreover, so that calculus can be applied, the universe is typically assumed to be a differentiable manifold. A mathematical object that possesses all these properties, compact without boundary and differentiable, is termed a closed manifold. The 3-sphere and 3-torus are both closed manifolds.

Curvature

The curvature of the universe places constraints on the topology. If the spatial geometry is spherical, i.e., possess positive curvature, the topology is compact. For a flat (zero curvature) or a hyperbolic (negative curvature) spatial geometry, the topology can be either compact or infinite. Many textbooks erroneously state that a flat universe implies an infinite universe; however, the correct statement is that a flat universe that is also simply connected implies an infinite universe. For example, Euclidean space is flat, simply connected, and infinite, but the torus is flat, multiply connected, finite, and compact. 

In general, local to global theorems in Riemannian geometry relate the local geometry to the global geometry. If the local geometry has constant curvature, the global geometry is very constrained, as described in Thurston geometries. 

The latest research shows that even the most powerful future experiments (like the SKA) will not be able to distinguish between flat, open and closed universe if the true value of cosmological curvature parameter is smaller than 10⁻⁴. If the true value of the cosmological curvature parameter is larger than 10⁻³ we will be able to distinguish between these three models even now.

Results of the Planck mission released in 2015 show the cosmological curvature parameter, Ω_K, to be 0.000±0.005, consistent with a flat universe.

Universe with zero curvature

In a universe with zero curvature, the local geometry is flat. The most obvious global structure is that of Euclidean space, which is infinite in extent. Flat universes that are finite in extent include the torus and Klein bottle. Moreover, in three dimensions, there are 10 finite closed flat 3-manifolds, of which 6 are orientable and 4 are non-orientable. These are the Bieberbach manifolds. The most familiar is the aforementioned 3-torus universe.

In the absence of dark energy, a flat universe expands forever but at a continually decelerating rate, with expansion asymptotically approaching zero. With dark energy, the expansion rate of the universe initially slows down, due to the effect of gravity, but eventually increases. The ultimate fate of the universe is the same as that of an open universe.

A flat universe can have zero total energy. 

Universe with positive curvature

Universe in an expanding sphere. The galaxies farthest away are moving fastest and hence experience length contraction and so become smaller to an observer in the centre.

 

A positively curved universe is described by elliptic geometry, and can be thought of as a three-dimensional hypersphere, or some other spherical 3-manifold (such as the Poincaré dodecahedral space), all of which are quotients of the 3-sphere. 

Poincaré dodecahedral space is a positively curved space, colloquially described as "soccerball-shaped", as it is the quotient of the 3-sphere by the binary icosahedral group, which is very close to icosahedral symmetry, the symmetry of a soccer ball. This was proposed by Jean-Pierre Luminet and colleagues in 2003 and an optimal orientation on the sky for the model was estimated in 2008.

Universe with negative curvature

A hyperbolic universe, one of a negative spatial curvature, is described by hyperbolic geometry, and can be thought of locally as a three-dimensional analog of an infinitely extended saddle shape. There are a great variety of hyperbolic 3-manifolds, and their classification is not completely understood. Those of finite volume can be understood via the Mostow rigidity theorem. For hyperbolic local geometry, many of the possible three-dimensional spaces are informally called "horn topologies", so called because of the shape of the pseudosphere, a canonical model of hyperbolic geometry. An example is the Picard horn, a negatively curved space, colloquially described as "funnel-shaped".

Curvature: open or closed

When cosmologists speak of the universe as being "open" or "closed", they most commonly are referring to whether the curvature is negative or positive. These meanings of open and closed are different from the mathematical meaning of open and closed used for sets in topological spaces and for the mathematical meaning of open and closed manifolds, which gives rise to ambiguity and confusion. In mathematics, there are definitions for a closed manifold (i.e., compact without boundary) and open manifold (i.e., one that is not compact and without boundary). A "closed universe" is necessarily a closed manifold. An "open universe" can be either a closed or open manifold. For example, in the Friedmann–Lemaître–Robertson–Walker (FLRW) model the universe is considered to be without boundaries, in which case "compact universe" could describe a universe that is a closed manifold. 

Milne model ("spherical" expanding)

If one applies Minkowski space-based special relativity to expansion of the universe, without resorting to the concept of a curved spacetime, then one obtains the Milne model. Any spatial section of the universe of a constant age (the proper time elapsed from the Big Bang) will have a negative curvature; this is merely a pseudo-Euclidean geometric fact analogous to one that concentric spheres in the flat Euclidean space are nevertheless curved. Spatial geometry of this model is an unbounded hyperbolic space. The entire universe is contained within a light cone, namely the future cone of the Big Bang. For any given moment t > 0 of coordinate time (assuming the Big Bang has t = 0), the entire universe is bounded by a sphere of radius exactly c t. The apparent paradox of an infinite universe contained within a sphere is explained with length contraction: the galaxies farther away, which are travelling away from the observer the fastest, will appear thinner.

This model is essentially a degenerate FLRW for Ω = 0. It is incompatible with observations that definitely rule out such a large negative spatial curvature. However, as a background in which gravitational fields (or gravitons) can operate, due to diffeomorphism invariance, the space on the macroscopic scale, is equivalent to any other (open) solution of Einstein's field equations.

XMLHttpRequest

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/XMLHttpRequest

XMLHttpRequest (XHR) is an API in the form of an object whose methods transfer data between a web browser and a web server. The object is provided by the browser's JavaScript environment. Particularly, retrieval of data from XHR for the purpose of continually modifying a loaded web page is the underlying concept of Ajax design. Despite the name, XHR can be used with protocols other than HTTP and data can be in the form of not only XML, but also JSON, HTML or plain text.

WHATWG maintains an XHR standard as a living document. Ongoing work at the W3C to create a stable specification is based on snapshots of the WHATWG standard.

History

The concept behind the XMLHttpRequest object was originally created by the developers of Outlook Web Access (by Microsoft) for Microsoft Exchange Server 2000. An interface called IXMLHTTPRequest was developed and implemented into the second version of the MSXML library using this concept. The second version of the MSXML library was shipped with Internet Explorer 5.0 in March 1999, allowing access, via ActiveX, to the IXMLHTTPRequest interface using the XMLHTTP wrapper of the MSXML library.

Internet Explorer versions 5 and 6 did not define the XMLHttpRequest object identifier in their scripting languages as the XMLHttpRequest identifier itself was not standard at the time of their releases. Backward compatibility can be achieved through object detection if the XMLHttpRequest identifier does not exist. Microsoft added the XMLHttpRequest object identifier to its scripting languages in Internet Explorer 7.0 released in October 2006.

The Mozilla project developed and implemented an interface called nsIXMLHttpRequest into the Gecko layout engine. This interface was modeled to work as closely to Microsoft's IXMLHTTPRequest interface as possible. Mozilla created a wrapper to use this interface through a JavaScript object which they called XMLHttpRequest. The XMLHttpRequest object was accessible as early as Gecko version 0.6 released on December 6 of 2000, but it was not completely functional until as late as version 1.0 of Gecko released on June 5, 2002. The XMLHttpRequest object became a de facto standard in other major web clients, implemented in Safari 1.2 released in February 2004, Konqueror, Opera 8.0 released in April 2005, and iCab 3.0b352 released in September 2005.

With the advent of cross-browser JavaScript libraries such as jQuery, developers can invoke XMLHttpRequest functionality indirectly. 

Standards

The World Wide Web Consortium published a Working Draft specification for the XMLHttpRequest object on April 5, 2006, edited by Anne van Kesteren of Opera Software and Dean Jackson of W3C. Its goal is "to document a minimum set of interoperable features based on existing implementations, allowing Web developers to use these features without platform-specific code."

The W3C also published another Working Draft specification for the XMLHttpRequest object, "XMLHttpRequest Level 2", on February 25 of 2008. Level 2 consists of extended functionality to the XMLHttpRequest object, including, but not limited to, progress events, support for cross-site requests, and the handling of byte streams. At the end of 2011, the Level 2 specification was abandoned and absorbed into the original specification.

At the end of 2012, the WHATWG took over development and maintains a living standard using Web IDL. W3C's current drafts are based on snapshots of the WHATWG standard. 

HTTP request

The following sections demonstrate how a request using the XMLHttpRequest object functions within a conforming user agent based on the W3C Working Draft. As the W3C standard for the XMLHttpRequest object is still a draft, user agents may not abide by all the functionings of the W3C definition and any of the following is subject to change. Extreme care should be taken into consideration when scripting with the XMLHttpRequest object across multiple user agents. This article will try to list the inconsistencies between the major user agents.

The open method

The HTTP and HTTPS requests of the XMLHttpRequest object must be initialized through the open method. This method must be invoked prior to the actual sending of a request to validate and resolve the request method, URL, and URI user information to be used for the request. This method does not assure that the URL exists or the user information is correct. This method can accept up to five parameters, but requires only two, to initialize a request.

open( Method, URL, Asynchronous, UserName, Password )

The first parameter of the method is a text string indicating the HTTP request method to use. The request methods that must be supported by a conforming user agent, defined by the W3C draft for the XMLHttpRequest object, are currently listed as the following.

• GET (supported by Internet Explorer 7 (and later), Mozilla 1+)
• POST (supported by IE7 (and later), Mozilla 1 (and later))
• HEAD (supported by IE7 (and later))
• PUT
• DELETE
• OPTIONS (supported by IE7 (and later))

However, request methods are not limited to the ones listed above. The W3C draft states that a browser may support additional request methods at their own discretion.

The second parameter of the method is another text string, this one indicating the URL of the HTTP request. The W3C recommends that browsers should raise an error and not allow the request of a URL with either a different port or ihost URI component from the current document.

The third parameter, a boolean value indicating whether or not the request will be asynchronous, is not a required parameter by the W3C draft. The default value of this parameter should be assumed to be true by a W3C conforming user agent if it is not provided. An asynchronous request ("true") will not wait on a server response before continuing on with the execution of the current script. It will instead invoke the onreadystatechange event listener of the XMLHttpRequest object throughout the various stages of the request. A synchronous request ("false") however will block execution of the current script until the request has been completed, thus not invoking the onreadystatechange event listener. Note that starting with Gecko 30.0 (Firefox 30.0 / Thunderbird 30.0 / SeaMonkey 2.27), Blink 39.0 (Chrome), and Edge 13, synchronous requests on the main thread have been deprecated due to their negative impact on the user experience as they will cause freezing of the UI while the thread performs the request.

The fourth and fifth parameters are the username and password, respectively. These parameters, or just the username, may be provided for authentication and authorization if required by the server for this request. 

var xmlhttp;

if (window.XMLHttpRequest) {
    xmlhttp = new XMLHttpRequest();
    xmlhttp.open("GET", filepath, false);
    xmlhttp.send(null);
}

The setRequestHeader method

Upon successful initialization of a request, the setRequestHeader method of the XMLHttpRequest object can be invoked to send HTTP headers with the request.

setRequestHeader( Name, Value )

The first parameter of this method is the text string name of the header. The second parameter is the text string value. This method must be invoked for each header that needs to be sent with the request. Any headers attached here will be removed the next time the open method is invoked in a W3C conforming user agent.

The send method

To send an HTTP request, the send method of the XMLHttpRequest must be invoked. This method accepts a single parameter containing the content to be sent with the request. 

send( Data )

This parameter may be omitted if no content needs to be sent. The W3C draft states that this parameter may be any type available to the scripting language as long as it can be turned into a text string, with the exception of the DOM document object. If a user agent cannot serialise the parameter, then the parameter should be ignored. Firefox 3.0.x and previous versions will however throw an exception if send is called without an argument.

If the parameter is a DOM document object, a user agent should assure the document is turned into well-formed XML using the encoding indicated by the inputEncoding property of the document object. If the Content-Type request header was not added through setRequestHeader yet, it should automatically be added by a conforming user agent as "application/xml;charset=charset," where charset is the encoding used to encode the document.

If the user agent is configured to use a proxy server, then the XMLHttpRequest object will modify the request appropriately so as to connect to the proxy instead of the origin server, and send Proxy-Authorization headers as configured.

The onreadystatechange event listener

If the open method of the XMLHttpRequest object was invoked with the third parameter set to true for an asynchronous request, the onreadystatechange event listener will be automatically invoked for each of the following actions that change the readyState property of the XMLHttpRequest object.

State changes work like this:

• State Description

   0  The request is not initialized.
   1  The request has been set up.
   2  The request has been sent.
   3  The request is in process.
   4  The request is completed.

• After the open method has been invoked successfully, the readyState property of the XMLHttpRequest object should be assigned a value of 1 (OPENED).
• After the send method has been invoked and the HTTP response headers have been received, the readyState property of the XMLHttpRequest object should be assigned a value of 2 (HEADERS_RECEIVED).
• Once the HTTP response content begins to load, the readyState property of the XMLHttpRequest object should be assigned a value of 3 (LOADING).
• Once the HTTP response content has finished loading, the readyState property of the XMLHttpRequest object should be assigned a value of 4 (DONE).

The listener will only respond to state changes which occur after the listener is defined. To detect states 1 and 2, the listener must be defined before the open method is invoked. The open method must be invoked before the send method is invoked.

var request = new XMLHttpRequest();
request.onreadystatechange = function () {
    var DONE = this.DONE || 4;
    if (this.readyState === DONE){
        alert(this.readyState);
    }
};
request.open('GET', 'somepage.xml', true);
request.setRequestHeader('X-Requested-With', 'XMLHttpRequest'); 

// Tells server that this call is made for ajax purposes. 

// Most libraries like jQuery/Prototype/Dojo do this
request.send(null);  // No data needs to be sent along with the request.

The HTTP response

After a successful and completed call to the send method of the XMLHttpRequest, if the server response was well-formed XML and the Content-Type header sent by the server is understood by the user agent as an Internet media type for XML, the responseXML property of the XMLHttpRequest object will contain a DOM document object. Another property, responseText will contain the response of the server in plain text by a conforming user agent, regardless of whether or not it was understood as XML.

Cross-domain requests

In the early development of the World Wide Web, it was found possible to breach users' security by the use of JavaScript to exchange information from one web site with that from another less reputable one. All modern browsers therefore implement a same origin policy that prevents many such attacks, such as cross-site scripting. XMLHttpRequest data is subject to this security policy, but sometimes web developers want to intentionally circumvent its restrictions. This is sometimes due to the legitimate use of subdomains as, for example, making an XMLHttpRequest from a page created by foo.example.com for information from bar.example.com will normally fail.

Various alternatives exist to circumvent this security feature, including using JSONP, Cross-Origin Resource Sharing (CORS) or alternatives with plugins such as Flash or Silverlight. Cross-origin XMLHttpRequest is specified in W3C's XMLHttpRequest Level 2 specification. Internet Explorer did not implement CORS until version 10. The two previous versions (8 and 9) offered similar functionality through the XDomainRequest (XDR) API. CORS is now supported by all modern browsers (desktop and mobile).

The CORS protocol has several restrictions, with two models of support. The simple model does not allow setting custom request headers and omits cookies. Further, only the HEAD, GET and POST request methods are supported, and POST only allows the following MIME types: "text/plain", "application/x-www-urlencoded" and "multipart/form-data". Only "text/plain" was initially supported. The other model detects when one of the non-simple features are requested and sends a pre-flight request to the server to negotiate the feature.

Fetch alternative

Program flow using asynchronous XHR callbacks can present difficulty with readability and maintenance. ECMAScript 2015 (ES6) added the promise construct to simplify asynchronous logic. Browsers have since implemented the alternative fetch() interface to achieve the same functionality as XHR using promises instead of callbacks. 

Fetch is also standardized by WHATWG.