Supergiant

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Supergiant 

Hertzsprung–Russell diagram

Spectral type

O

B

A

F

G

K

M

L

T

Brown dwarfs

White dwarfs

Red dwarfs

Subdwarfs

Main sequence
("dwarfs")

Subgiants

Giants

Red giants

Blue giants

Bright giants

Supergiants

Red supergiant

Hypergiants

absolute
magni-
tude
(M_V)

Supergiants are among the most massive and most luminous stars. Supergiant stars occupy the top region of the Hertzsprung–Russell diagram with absolute visual magnitudes between about −3 and −8. The temperature range of supergiant stars spans from about 3,400 K to over 20,000 K.

Definition

The title supergiant, as applied to a star, does not have a single concrete definition. The term giant star was first coined by Hertzsprung when it became apparent that the majority of stars fell into two distinct regions of the Hertzsprung–Russell diagram. One region contained larger and more luminous stars of spectral types A to M and received the name giant. Subsequently, as they lacked any measurable parallax, it became apparent that some of these stars were significantly larger and more luminous than the bulk, and the term super-giant arose, quickly adopted as supergiant.

Spectral luminosity class

The four brightest stars in NGC 4755 are blue supergiant stars, with a red supergiant star at the centre. (ESO VLT)

Supergiant stars can be identified on the basis of their spectra, with distinctive lines sensitive to high luminosity and low surface gravity. In 1897, Antonia C. Maury had divided stars based on the widths of their spectral lines, with her class "c" identifying stars with the narrowest lines. Although it was not known at the time, these were the most luminous stars. In 1943, Morgan and Keenan formalised the definition of spectral luminosity classes, with class I referring to supergiant stars. The same system of MK luminosity classes is still used today, with refinements based on the increased resolution of modern spectra. Supergiants occur in every spectral class from young blue class O supergiants to highly evolved red class M supergiants. Because they are enlarged compared to main-sequence and giant stars of the same spectral type, they have lower surface gravities, and changes can be observed in their line profiles. Supergiants are also evolved stars with higher levels of heavy elements than main-sequence stars. This is the basis of the MK luminosity system which assigns stars to luminosity classes purely from observing their spectra.

In addition to the line changes due to low surface gravity and fusion products, the most luminous stars have high mass-loss rates and resulting clouds of expelled circumstellar materials which can produce emission lines, P Cygni profiles, or forbidden lines. The MK system assigns stars to luminosity classes: Ib for supergiants; Ia for luminous supergiants; and 0 (zero) or Ia⁺ for hypergiants. In reality there is much more of a continuum than well defined bands for these classifications, and classifications such as Iab are used for intermediate luminosity supergiants. Supergiant spectra are frequently annotated to indicate spectral peculiarities, for example B2 Iae or F5 Ipec.

Evolutionary supergiants

Supergiants can also be defined as a specific phase in the evolutionary history of certain stars. Stars with initial masses above 8-10 M_☉ quickly and smoothly initiate helium core fusion after they have exhausted their hydrogen, and continue fusing heavier elements after helium exhaustion until they develop an iron core, at which point the core collapses to produce a Type II supernova. Once these massive stars leave the main sequence, their atmospheres inflate, and they are described as supergiants. Stars initially under 10 M_☉ will never form an iron core and in evolutionary terms do not become supergiants, although they can reach luminosities thousands of times the sun's. They cannot fuse carbon and heavier elements after the helium is exhausted, so they eventually just lose their outer layers, leaving the core of a white dwarf. The phase where these stars have both hydrogen and helium burning shells is referred to as the asymptotic giant branch (AGB), as stars gradually become more and more luminous class M stars. Stars of 8-10 M_☉ may fuse sufficient carbon on the AGB to produce an oxygen-neon core and an electron-capture supernova, but astrophysicists categorise these as super-AGB stars rather than supergiants.

Categorisation of evolved stars

There are several categories of evolved stars that are not supergiants in evolutionary terms but may show supergiant spectral features or have luminosities comparable to supergiants.

Asymptotic-giant-branch (AGB) and post-AGB stars are highly evolved lower-mass red giants with luminosities that can be comparable to more massive red supergiants, but because of their low mass, being in a different stage of development (helium shell burning), and their lives ending in a different way (planetary nebula and white dwarf rather than supernova), astrophysicists prefer to keep them separate. The dividing line becomes blurred at around 7–10 M_☉ (or as high as 12 M_☉ in some models) where stars start to undergo limited fusion of elements heavier than helium. Specialists studying these stars often refer to them as super AGB stars, since they have many properties in common with AGB such as thermal pulsing. Others describe them as low-mass supergiants since they start to burn elements heavier than helium and can explode as supernovae. Many post-AGB stars receive spectral types with supergiant luminosity classes. For example, RV Tauri has an Ia (bright supergiant) luminosity class despite being less massive than the sun. Some AGB stars also receive a supergiant luminosity class, most notably W Virginis variables such as W Virginis itself, stars that are executing a blue loop triggered by thermal pulsing. A very small number of Mira variables and other late AGB stars have supergiant luminosity classes, for example α Herculis.

Classical Cepheid variables typically have supergiant luminosity classes, although only the most luminous and massive will actually go on to develop an iron core. The majority of them are intermediate mass stars fusing helium in their cores and will eventually transition to the asymptotic giant branch. δ Cephei itself is an example with a luminosity of 2,000 L_☉ and a mass of 4.5 M_☉.

Wolf–Rayet stars are also high-mass luminous evolved stars, hotter than most supergiants and smaller, visually less bright but often more luminous because of their high temperatures. They have spectra dominated by helium and other heavier elements, usually showing little or no hydrogen, which is a clue to their nature as stars even more evolved than supergiants. Just as the AGB stars occur in almost the same region of the HR diagram as red supergiants, Wolf–Rayet stars can occur in the same region of the HR diagram as the hottest blue supergiants and main-sequence stars.

The most massive and luminous main-sequence stars are almost indistinguishable from the supergiants they quickly evolve into. They have almost identical temperatures and very similar luminosities, and only the most detailed analyses can distinguish the spectral features that show they have evolved away from the narrow early O-type main-sequence to the nearby area of early O-type supergiants. Such early O-type supergiants share many features with WNLh Wolf–Rayet stars and are sometimes designated as slash stars, intermediates between the two types.

Luminous blue variables (LBVs) stars occur in the same region of the HR diagram as blue supergiants but are generally classified separately. They are evolved, expanded, massive, and luminous stars, often hypergiants, but they have very specific spectral variability, which defies the assignment of a standard spectral type. LBVs observed only at a particular time or over a period of time when they are stable, may simply be designated as hot supergiants or as candidate LBVs due to their luminosity.

Hypergiants are frequently treated as a different category of star from supergiants, although in all important respects they are just a more luminous category of supergiant. They are evolved, expanded, massive and luminous stars like supergiants, but at the most massive and luminous extreme, and with particular additional properties of undergoing high mass-loss due to their extreme luminosities and instability. Generally only the more evolved supergiants show hypergiant properties, since their instability increases after high mass-loss and some increase in luminosity.

Some B[e] stars are supergiants although other B[e] stars are clearly not. Some researchers distinguish the B[e] objects as separate from supergiants, while researchers prefer to define massive evolved B[e] stars as a subgroup of supergiants. The latter has become more common with the understanding that the B[e] phenomenon arises separately in a number of distinct types of stars, including some that are clearly just a phase in the life of supergiants.

Properties

The disc and atmosphere of Betelgeuse (ESO)

Supergiants have masses from 8 to 12 times the Sun (M_☉) upwards, and luminosities from about 1,000 to over a million times the Sun (L_☉). They vary greatly in radius, usually from 30 to 500, or even in excess of 1,000 solar radii (R_☉). They are massive enough to begin helium-core burning gently before the core becomes degenerate, without a flash and without the strong dredge-ups that lower-mass stars experience. They go on to successively ignite heavier elements, usually all the way to iron. Also because of their high masses, they are destined to explode as supernovae.

The Stefan–Boltzmann law dictates that the relatively cool surfaces of red supergiants radiate much less energy per unit area than those of blue supergiants; thus, for a given luminosity, red supergiants are larger than their blue counterparts. Radiation pressure limits the largest cool supergiants to around 1,500 R_☉ and the most massive hot supergiants to around a million L_☉ (M_bol around −10). Stars near and occasionally beyond these limits become unstable, pulsate, and experience rapid mass loss.

Surface gravity

The supergiant luminosity class is assigned on the basis of spectral features that are largely a measure of surface gravity, although such stars are also affected by other properties such as microturbulence. Supergiants typically have surface gravities of around log(g) 2.0 cgs and lower, although bright giants (luminosity class II) have statistically very similar surface gravities to normal Ib supergiants. Cool luminous supergiants have lower surface gravities, with the most luminous (and unstable) stars having log(g) around zero. Hotter supergiants, even the most luminous, have surface gravities around one, due to their higher masses and smaller radii.

Temperature

There are supergiant stars at all of the main spectral classes and across the whole range of temperatures from mid-M class stars at around 3,400 K to the hottest O class stars over 40,000 K. Supergiants are generally not found cooler than mid-M class. This is expected theoretically since they would be catastrophically unstable; however, there are potential exceptions among extreme stars such as VX Sagittarii.

Although supergiants exist in every class from O to M, the majority are spectral type B, more than at all other spectral classes combined. A much smaller grouping consists of very low-luminosity G-type supergiants, intermediate mass stars burning helium in their cores before reaching the asymptotic giant branch. A distinct grouping is made up of high-luminosity supergiants at early B (B0-2) and very late O (O9.5), more common even than main sequence stars of those spectral types.

The relative numbers of blue, yellow, and red supergiants is an indicator of the speed of stellar evolution and is used as a powerful test of models of the evolution of massive stars.

Luminosity

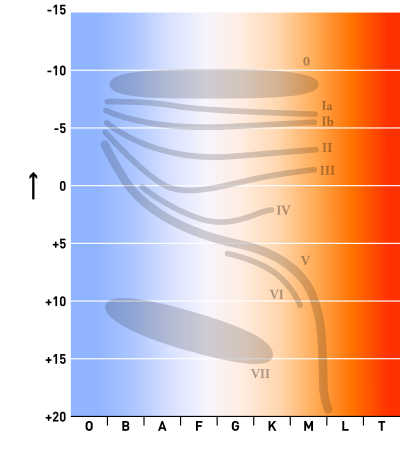
The supergiants lie more or less on a horizontal band occupying the entire upper portion of the HR diagram, but there are some variations at different spectral types. These variations are due partly to different methods for assigning luminosity classes at different spectral types, and partly to actual physical differences in the stars.

The bolometric luminosity of a star reflects its total output of electromagnetic radiation at all wavelengths. For very hot and very cool stars, the bolometric luminosity is dramatically higher than the visual luminosity, sometimes several magnitudes or a factor of five or more. This bolometric correction is approximately one magnitude for mid B, late K, and early M stars, increasing to three magnitudes (a factor of 15) for O and mid M stars.

All supergiants are larger and more luminous than main sequence stars of the same temperature. This means that hot supergiants lie on a relatively narrow band above bright main sequence stars. A B0 main sequence star has an absolute magnitude of about −5, meaning that all B0 supergiants are significantly brighter than absolute magnitude −5. Bolometric luminosities for even the faintest blue supergiants are tens of thousands of times the sun (L_☉). The brightest can be over a million L_☉ and are often unstable such as α Cygni variables and luminous blue variables.

The very hottest supergiants with early O spectral types occur in an extremely narrow range of luminosities above the highly luminous early O main sequence and giant stars. They are not classified separately into normal (Ib) and luminous (Ia) supergiants, although they commonly have other spectral type modifiers such as "f" for nitrogen and helium emission (e.g. O2 If for HD 93129A).^[18]

Yellow supergiants can be considerably fainter than absolute magnitude −5, with some examples around −2 (e.g. 14 Persei). With bolometric corrections around zero, they may only be a few hundred times the luminosity of the sun. These are not massive stars, though; instead, they are stars of intermediate mass that have particularly low surface gravities, often due to instability such as Cepheid pulsations. These intermediate mass stars' being classified as supergiants during a relatively long-lasting phase of their evolution account for the large number of low luminosity yellow supergiants. The most luminous yellow stars, the yellow hypergiants, are amongst the visually brightest stars, with absolute magnitudes around −9, although still less than a million L_☉.

There is a strong upper limit to the luminosity of red supergiants at around half a million L_☉. Stars that would be brighter than this shed their outer layers so rapidly that they remain hot supergiants after they leave the main sequence. The majority of red supergiants were 10-15 M_☉ main sequence stars and now have luminosities below 100,000 L_☉, and there are very few bright supergiant (Ia) M class stars. The least luminous stars classified as red supergiants are some of the brightest AGB and post-AGB stars, highly expanded and unstable low mass stars such as the RV Tauri variables. The majority of AGB stars are given giant or bright giant luminosity classes, but particularly unstable stars such as W Virginis variables may be given a supergiant classification (e.g. W Virginis itself). The faintest red supergiants are around absolute magnitude −3.

Variability

RS Puppis is a supergiant and Classical Cepheid variable.

While most supergiants such as Alpha Cygni variables, semiregular variables, and irregular variables show some degree of photometric variability, certain types of variables amongst the supergiants are well defined. The instability strip crosses the region of supergiants, and specifically many yellow supergiants are Classical Cepheid variables. The same region of instability extends to include the even more luminous yellow hypergiants, an extremely rare and short-lived class of luminous supergiant. Many R Coronae Borealis variables, although not all, are yellow supergiants, but this variability is due to their unusual chemical composition rather than a physical instability.

Further types of variable stars such as RV Tauri variables and PV Telescopii variables are often described as supergiants. RV Tau stars are frequently assigned spectral types with a supergiant luminosity class on account of their low surface gravity, and they are amongst the most luminous of the AGB and post-AGB stars, having masses similar to the sun; likewise, the even rarer PV Tel variables are often classified as supergiants, but have lower luminosities than supergiants and peculiar B[e] spectra extremely deficient in hydrogen. Possibly they are also post-AGB objects or "born-again" AGB stars.

The LBVs are variable with multiple semi-regular periods and less predictable eruptions and giant outbursts. They are usually supergiants or hypergiants, occasionally with Wolf-Rayet spectra—extremely luminous, massive, evolved stars with expanded outer layers, but they are so distinctive and unusual that they are often treated as a separate category without being referred to as supergiants or given a supergiant spectral type. Often their spectral type will be given just as "LBV" because they have peculiar and highly variable spectral features, with temperatures varying from about 8,000 K in outburst up to 20,000 K or more when "quiescent."

Chemical abundances

The abundance of various elements at the surface of supergiants is different from less luminous stars. Supergiants are evolved stars and may have undergone convection of fusion products to the surface.

Cool supergiants show enhanced helium and nitrogen at the surface due to convection of these fusion products to the surface during the main sequence of very massive stars, to dredge-ups during shell burning, and to the loss of the outer layers of the star. Helium is formed in the core and shell by fusion of hydrogen and nitrogen which accumulates relative to carbon and oxygen during CNO cycle fusion. At the same time, carbon and oxygen abundances are reduced. Red supergiants can be distinguished from luminous but less massive AGB stars by unusual chemicals at the surface, enhancement of carbon from deep third dredge-ups, as well as carbon-13, lithium and s-process elements. Late-phase AGB stars can become highly oxygen-enriched, producing OH masers.

Hotter supergiants show differing levels of nitrogen enrichment. This may be due to different levels of mixing on the main sequence due to rotation or because some blue supergiants are newly evolved from the main sequence while others have previously been through a red supergiant phase. Post-red supergiant stars have a generally higher level of nitrogen relative to carbon due to convection of CNO-processed material to the surface and the complete loss of the outer layers. Surface enhancement of helium is also stronger in post-red supergiants, representing more than a third of the atmosphere.

Evolution

Main article: Stellar evolution

O type main-sequence stars and the most massive of the B type blue-white stars become supergiants. Due to their extreme masses, they have short lifespans, between 30 million years and a few hundred thousand years. They are mainly observed in young galactic structures such as open clusters, the arms of spiral galaxies, and in irregular galaxies. They are less abundant in spiral galaxy bulges and are rarely observed in elliptical galaxies, or globular clusters, which are composed mainly of old stars.

Supergiants develop when massive main-sequence stars run out of hydrogen in their cores, at which point they start to expand, just like lower-mass stars. Unlike lower-mass stars, however, they begin to fuse helium in the core smoothly and not long after exhausting their hydrogen. This means that they do not increase their luminosity as dramatically as lower-mass stars, and they progress nearly horizontally across the HR diagram to become red supergiants. Also unlike lower-mass stars, red supergiants are massive enough to fuse elements heavier than helium, so they do not puff off their atmospheres as planetary nebulae after a period of hydrogen and helium shell burning; instead, they continue to burn heavier elements in their cores until they collapse. They cannot lose enough mass to form a white dwarf, so they will leave behind a neutron star or black hole remnant, usually after a core collapse supernova explosion.

Stars more massive than about 40 M_☉ cannot expand into a red supergiant. Because they burn too quickly and lose their outer layers too quickly, they reach the blue supergiant stage, or perhaps yellow hypergiant, before returning to become hotter stars. The most massive stars, above about 100 M_☉, hardly move at all from their position as O main-sequence stars. These convect so efficiently that they mix hydrogen from the surface right down to the core. They continue to fuse hydrogen until it is almost entirely depleted throughout the star, then rapidly evolve through a series of stages of similarly hot and luminous stars: supergiants, slash stars, WNh-, WN-, and possibly WC- or WO-type stars. They are expected to explode as supernovae, but it is not clear how far they evolve before this happens. The existence of these supergiants still burning hydrogen in their cores may necessitate a slightly more complex definition of supergiant: a massive star with increased size and luminosity due to fusion products building up, but still with some hydrogen remaining.

The first stars in the universe are thought to have been considerably brighter and more massive than the stars in the modern universe. Part of the theorized population III of stars, their existence is necessary to explain observations of elements other than hydrogen and helium in quasars. Possibly larger and more luminous than any supergiant known today, their structure was quite different, with reduced convection and less mass loss. Their very short lives are likely to have ended in violent photodisintegration or pair instability supernovae.

Supernova progenitors

Main article: Supernova

Most type II supernova progenitors are thought to be red supergiants, while the less common type Ib/c supernovae are produced by hotter Wolf–Rayet stars that have completely lost more of their hydrogen atmosphere. Almost by definition, supergiants are destined to end their lives violently. Stars large enough to start fusing elements heavier than helium do not seem to have any way to lose enough mass to avoid catastrophic core collapse, although some may collapse, almost without trace, into their own central black holes.

The simple "onion" models showing red supergiants inevitably developing to an iron core and then exploding have been shown, however, to be too simplistic. The progenitor for the unusual type II Supernova 1987A was a blue supergiant, thought to have already passed through the red supergiant phase of its life, and this is now known to be far from an exceptional situation. Much research is now focused on how blue supergiants can explode as a supernova and when red supergiants can survive to become hotter supergiants again.

Well known examples

Supergiants are rare and short-lived stars, but their high luminosity means that there are many naked-eye examples, including some of the brightest stars in the sky. Rigel, the brightest star in the constellation Orion, is a typical blue-white supergiant; Deneb is the brightest star in Cygnus, a white supergiant; Delta Cephei is the famous prototype Cepheid variable, a yellow supergiant; and Antares and UY Scuti are red supergiants. μ Cephei is one of the reddest stars visible to the naked eye and one of the largest in the galaxy. Rho Cassiopeiae, a variable, yellow hypergiant, is one of the most luminous naked-eye stars. Betelgeuse is a red supergiant and the second brightest star in the constellation Orion.

Giant star

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Giant_star 

 

Hertzsprung–Russell diagram

Spectral type

O

B

A

F

G

K

M

L

T

Brown dwarfs

White dwarfs

Red dwarfs

Subdwarfs

Main sequence
("dwarfs")

Subgiants

Giants

Red giants

Blue giants

Bright giants

Supergiants

Red supergiant

Hypergiants

absolute
magni-
tude
(M_V)

A giant star is a star with substantially larger radius and luminosity than a main-sequence (or dwarf) star of the same surface temperature. They lie above the main sequence (luminosity class V in the Yerkes spectral classification) on the Hertzsprung–Russell diagram and correspond to luminosity classes II and III. The terms giant and dwarf were coined for stars of quite different luminosity despite similar temperature or spectral type by Ejnar Hertzsprung about 1905.

Giant stars have radii up to a few hundred times the Sun and luminosities between 10 and a few thousand times that of the Sun. Stars still more luminous than giants are referred to as supergiants and hypergiants.

A hot, luminous main-sequence star may also be referred to as a giant, but any main-sequence star is properly called a dwarf, regardless of how large and luminous it is.

Formation

Internal structure of a Sun-like star and a red giant. ESO image.

A star becomes a giant after all the hydrogen available for fusion at its core has been depleted and, as a result, leaves the main sequence. The behaviour of a post-main-sequence star depends largely on its mass.

Intermediate-mass stars

For a star with a mass above about 0.25 solar masses (M_☉), once the core is depleted of hydrogen it contracts and heats up so that hydrogen starts to fuse in a shell around the core. The portion of the star outside the shell expands and cools, but with only a small increase in luminosity, and the star becomes a subgiant. The inert helium core continues to grow and increase in temperature as it accretes helium from the shell, but in stars up to about 10-12 M_☉ it does not become hot enough to start helium burning (higher-mass stars are supergiants and evolve differently). Instead, after just a few million years the core reaches the Schönberg–Chandrasekhar limit, rapidly collapses, and may become degenerate. This causes the outer layers to expand even further and generates a strong convective zone that brings heavy elements to the surface in a process called the first dredge-up. This strong convection also increases the transport of energy to the surface, the luminosity increases dramatically, and the star moves onto the red-giant branch where it will stably burn hydrogen in a shell for a substantial fraction of its entire life (roughly 10% for a Sun-like star). The core continues to gain mass, contract, and increase in temperature, whereas there is some mass loss in the outer layers.

If the star's mass, when on the main sequence, was below approximately 0.4 M_☉, it will never reach the central temperatures necessary to fuse helium. It will therefore remain a hydrogen-fusing red giant until it runs out of hydrogen, at which point it will become a helium white dwarf. According to stellar evolution theory, no star of such low mass can have evolved to that stage within the age of the Universe.

In stars above about 0.4 M_☉ the core temperature eventually reaches 10⁸ K and helium will begin to fuse to carbon and oxygen in the core by the triple-alpha process. When the core is degenerate helium fusion begins explosively, but most of the energy goes into lifting the degeneracy and the core becomes convective. The energy generated by helium fusion reduces the pressure in the surrounding hydrogen-burning shell, which reduces its energy-generation rate. The overall luminosity of the star decreases, its outer envelope contracts again, and the star moves from the red-giant branch to the horizontal branch.

When the core helium is exhausted, a star with up to about 8 M_☉ has a carbon–oxygen core that becomes degenerate and starts helium burning in a shell. As with the earlier collapse of the helium core, this starts convection in the outer layers, triggers a second dredge-up, and causes a dramatic increase in size and luminosity. This is the asymptotic giant branch (AGB) analogous to the red-giant branch but more luminous, with a hydrogen-burning shell contributing most of the energy. Stars only remain on the AGB for around a million years, becoming increasingly unstable until they exhaust their fuel, go through a planetary nebula phase, and then become a carbon–oxygen white dwarf.

High-mass stars

Main-sequence stars with masses above about 12 M_☉ are already very luminous and they move horizontally across the HR diagram when they leave the main sequence, briefly becoming blue giants before they expand further into blue supergiants. They start core-helium burning before the core becomes degenerate and develop smoothly into red supergiants without a strong increase in luminosity. At this stage they have comparable luminosities to bright AGB stars although they have much higher masses, but will further increase in luminosity as they burn heavier elements and eventually become a supernova.

Stars in the 8~12 M_☉ range have somewhat intermediate properties and have been called super-AGB stars. They largely follow the tracks of lighter stars through RGB, HB, and AGB phases, but are massive enough to initiate core carbon burning and even some neon burning. They form oxygen–magnesium–neon cores, which may collapse in an electron-capture supernova, or they may leave behind an oxygen–neon white dwarf.

O class main sequence stars are already highly luminous. The giant phase for such stars is a brief phase of slightly increased size and luminosity before developing a supergiant spectral luminosity class. Type O giants may be more than a hundred thousand times as luminous as the sun, brighter than many supergiants. Classification is complex and difficult with small differences between luminosity classes and a continuous range of intermediate forms. The most massive stars develop giant or supergiant spectral features while still burning hydrogen in their cores, due to mixing of heavy elements to the surface and high luminosity which produces a powerful stellar wind and causes the star's atmosphere to expand.

Low-mass stars

A star whose initial mass is less than approximately 0.25 M_☉ will not become a giant star at all. For most of their lifetimes, such stars have their interior thoroughly mixed by convection and so they can continue fusing hydrogen for a time in excess of 10¹² years, much longer than the current age of the Universe. They steadily become hotter and more luminous throughout this time. Eventually they do develop a radiative core, subsequently exhausting hydrogen in the core and burning hydrogen in a shell surrounding the core. (Stars with a mass in excess of 0.16 M_☉ may expand at this point, but will never become very large.) Shortly thereafter, the star's supply of hydrogen will be completely exhausted and it is expected to become a helium white dwarf, although the universe is too young for any such star to exist yet, so no star with that history has ever been observed.

Subclasses

There are a wide range of giant-class stars and several subdivisions are commonly used to identify smaller groups of stars.

Subgiants

Main article: Subgiant

Subgiants are an entirely separate spectroscopic luminosity class (IV) from giants, but share many features with them. Although some subgiants are simply over-luminous main-sequence stars due to chemical variation or age, others are a distinct evolutionary track towards true giants.

Examples:

• Gamma Geminorum (γ Gem), an A-type subgiant;
• Eta Bootis (η Boo), a G-type subgiant.

Bright giants

Bright giants are stars of luminosity class II in the Yerkes spectral classification. These are stars which straddle the boundary between ordinary giants and supergiants, based on the appearance of their spectra. The bright giant luminosity class was first defined in 1943.

Well known stars which are classified as bright giants include:

• Canopus
• Epsilon Canis Majoris
• Omicron Scorpii
• Theta Scorpii
• Beta Draconis
• Beta Capricorni
• Alpha Herculis
• Gamma Canis Majoris

Red giants

Main article: Red giant

Within any giant luminosity class, the cooler stars of spectral class K, M, S, and C, (and sometimes some G-type stars) are called red giants. Red giants include stars in a number of distinct evolutionary phases of their lives: a main red-giant branch (RGB); a red horizontal branch or red clump; the asymptotic giant branch (AGB), although AGB stars are often large enough and luminous enough to get classified as supergiants; and sometimes other large cool stars such as immediate post-AGB stars. The RGB stars are by far the most common type of giant star due to their moderate mass, relatively long stable lives, and luminosity. They are the most obvious grouping of stars after the main sequence on most HR diagrams, although white dwarfs are more numerous but far less luminous.

Examples:

• Pollux, a K-type giant.
• Epsilon Ophiuchi, a G-type red giant.
• Arcturus (α Bootes), a K-type giant.
• Gamma Comae Berenices (γ Comae Berenices), a K-type giant.
• Mira (ο Ceti), an M-type giant and prototype Mira variable.
• Aldebaran, a K-type giant

Yellow giants

Giant stars with intermediate temperatures (spectral class G, F, and at least some A) are called yellow giants. They are far less numerous than red giants, partly because they only form from stars with somewhat higher masses, and partly because they spend less time in that phase of their lives. However, they include a number of important classes of variable stars. High-luminosity yellow stars are generally unstable, leading to the instability strip on the HR diagram where the majority of stars are pulsating variables. The instability strip reaches from the main sequence up to hypergiant luminosities, but at the luminosities of giants there are several classes of pulsating variable stars:

• RR Lyrae variables, pulsating horizontal-branch class A (sometimes F) stars with periods less than a day and amplitudes of a magnitude of less;
• W Virginis variables, more-luminous pulsating variables also known as type II Cepheids, with periods of 10–20 days;
• Type I Cepheid variables, more luminous still and mostly supergiants, with even longer periods;
• Delta Scuti variables, includes subgiant and main-sequence stars.

Yellow giants may be moderate-mass stars evolving for the first time towards the red-giant branch, or they may be more evolved stars on the horizontal branch. Evolution towards the red-giant branch for the first time is very rapid, whereas stars can spend much longer on the horizontal branch. Horizontal-branch stars, with more heavy elements and lower mass, are more unstable.

Examples:

• Sigma Octantis (σ Octantis), an F-type giant and a Delta Scuti variable;
• Capella Aa (α Aurigae Aa), a G-type giant.

Blue (and sometimes white) giants

Main article: Blue giant

The hottest giants, of spectral classes O, B, and sometimes early A, are called blue giants. Sometimes A- and late-B-type stars may be referred to as white giants.

The blue giants are a very heterogeneous grouping, ranging from high-mass, high-luminosity stars just leaving the main sequence to low-mass, horizontal-branch stars. Higher-mass stars leave the main sequence to become blue giants, then bright blue giants, and then blue supergiants, before expanding into red supergiants, although at the very highest masses the giant stage is so brief and narrow that it can hardly be distinguished from a blue supergiant.

Lower-mass, core-helium-burning stars evolve from red giants along the horizontal branch and then back again to the asymptotic giant branch, and depending on mass and metallicity they can become blue giants. It is thought that some post-AGB stars experiencing a late thermal pulse can become peculiar blue giants.

Mass–luminosity relation

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Mass%E2%80%93luminosity_relation 

In astrophysics, the mass–luminosity relation is an equation giving the relationship between a star's mass and its luminosity, first noted by Jakob Karl Ernst Halm. The relationship is represented by the equation:

${\displaystyle \frac{L}{L_{\odot }}={\left(\frac{M}{M_{\odot }}\right)}^a}$

where L_⊙ and M_⊙ are the luminosity and mass of the Sun and 1 < a < 6. The value a = 3.5 is commonly used for main-sequence stars. This equation and the usual value of a = 3.5 only applies to main-sequence stars with masses 2M_⊙ < M < 55M_⊙ and does not apply to red giants or white dwarfs. As a star approaches the Eddington luminosity then a = 1.

In summary, the relations for stars with different ranges of mass are, to a good approximation, as the following:

$${\displaystyle \frac{L}{L_{\odot }}\approx 0.23{\left(\frac{M}{M_{\odot }}\right)}^{2.3}(M<0.43M_{\odot })}$$

$${\displaystyle \frac{L}{L_{\odot }}={\left(\frac{M}{M_{\odot }}\right)}^4(0.43M_{\odot }<M<2M_{\odot })}$$

$${\displaystyle \frac{L}{L_{\odot }}\approx 1.4{\left(\frac{M}{M_{\odot }}\right)}^{3.5}(2M_{\odot }<M<55M_{\odot })}$$

$${\displaystyle \frac{L}{L_{\odot }}\approx 32000\frac{M}{M_{\odot }}(M>55M_{\odot })}$$

For stars with masses less than 0.43M_⊙, convection is the sole energy transport process, so the relation changes significantly. For stars with masses M > 55M_⊙ the relationship flattens out and becomes L ∝ M but in fact those stars don't last because they are unstable and quickly lose matter by intense solar winds. It can be shown this change is due to an increase in radiation pressure in massive stars. These equations are determined empirically by determining the mass of stars in binary systems to which the distance is known via standard parallax measurements or other techniques. After enough stars are plotted, stars will form a line on a logarithmic plot and slope of the line gives the proper value of a.

Another form, valid for K-type main-sequence stars, that avoids the discontinuity in the exponent has been given by Cuntz & Wang; it reads:

$${\displaystyle \frac{L}{L_{\odot }}\approx {\left(\frac{M}{M_{\odot }}\right)}^{a(M)}(0.20M_{\odot }<M<0.85M_{\odot })}$$

with

$${\displaystyle a(M)=-141.7\cdot M^4+232.4\cdot M^3-129.1\cdot M^2+33.29\cdot M+0.215}$$

(M in M_⊙). This relation is based on data by Mann and collaborators, who used moderate-resolution spectra of nearby late-K and M dwarfs with known parallaxes and interferometrically determined radii to refine their effective temperatures and luminosities. Those stars have also been used as a calibration sample for Kepler candidate objects. Besides avoiding the discontinuity in the exponent at M = 0.43M_⊙, the relation also recovers a = 4.0 for M ≃ 0.85M_⊙.

The mass/luminosity relation is important because it can be used to find the distance to binary systems which are too far for normal parallax measurements, using a technique called "dynamical parallax". In this technique, the masses of the two stars in a binary system are estimated, usually as being the mass of the Sun. Then, using Kepler's laws of celestial mechanics, the distance between the stars is calculated. Once this distance is found, the distance away can be found via the arc subtended in the sky, giving a preliminary distance measurement. From this measurement and the apparent magnitudes of both stars, the luminosities can be found, and by using the mass–luminosity relationship, the masses of each star. These masses are used to re-calculate the separation distance, and the process is repeated. The process is iterated many times, and accuracies as high as 5% can be achieved. The mass/luminosity relationship can also be used to determine the lifetime of stars by noting that lifetime is approximately proportional to M/L although one finds that more massive stars have shorter lifetimes than that which the M/L relationship predicts. A more sophisticated calculation factors in a star's loss of mass over time.

Derivation

Deriving a theoretically exact mass/luminosity relation requires finding the energy generation equation and building a thermodynamic model of the inside of a star. However, the basic relation L ∝ M³ can be derived using some basic physics and simplifying assumptions. The first such derivation was performed by astrophysicist Arthur Eddington in 1924. The derivation showed that stars can be approximately modelled as ideal gases, which was a new, somewhat radical idea at the time. What follows is a somewhat more modern approach based on the same principles.

An important factor controlling the luminosity of a star (energy emitted per unit time) is the rate of energy dissipation through its bulk. Where there is no heat convection, this dissipation happens mainly by photons diffusing. By integrating Fick's first law over the surface of some radius r in the radiation zone (where there is negligible convection), we get the total outgoing energy flux which is equal to the luminosity by conservation of energy:

$${\displaystyle L=-4\pi r^{2}D\frac{\mathrm{\partial }u}{\mathrm{\partial }r}}$$

where D is the photons diffusion coefficient, and u is the energy density.

Note that this assumes that the star is not fully convective, and that all heat creating processes (nucleosynthesis) happen in the core, below the radiation zone. These two assumptions are not correct in red giants, which do not obey the usual mass-luminosity relation. Stars of low mass are also fully convective, hence do not obey the law.

Approximating the star by a black body, the energy density is related to the temperature by the Stefan–Boltzmann law:

$${\displaystyle u=\frac{4}{c}{\sigma }_B{T}^4}$$

where

$${\displaystyle {\sigma }_B=\frac{2{\pi }^5{k}_B^4}{15c^2{h}^3}=\frac{{\pi }^2{k}_B^4}{60{\hslash }^3{c}^2}.}$$

is the Stefan–Boltzmann constant, c is the speed of light, k_B is Boltzmann constant and ${\displaystyle \hslash }$ is the reduced Planck constant.

As in the theory of diffusion coefficient in gases, the diffusion coefficient D approximately satisfies:

$${\displaystyle D=\frac{1}{3}c\lambda }$$

where λ is the photon mean free path.

Since matter is fully ionized in the star core (as well as where the temperature is of the same order of magnitude as inside the core), photons collide mainly with electrons, and so λ satisfies

$${\displaystyle \lambda =\frac{1}{n_e{\sigma }_{e\cdot \gamma }}}$$

Here ${\displaystyle n_e}$ is the electron density and:

$${\displaystyle {\sigma }_{e\cdot \gamma }=\frac{8\pi }{3}{\left(\frac{\alpha \hslash c}{m_e{c}^2}\right)}^2}$$

is the cross section for electron-photon scattering, equal to Thomson cross-section. α is the fine-structure constant and m_e the electron mass.

The average stellar electron density is related to the star mass M and radius R

$${\displaystyle ⟨n_e⟩=\frac{M}{m_{n}4\pi R^3/3}}$$

Finally, by the virial theorem, the total kinetic energy is equal to half the gravitational potential energy E_G, so if the average nuclei mass is m_n, then the average kinetic energy per nucleus satisfies:

$${\displaystyle \frac{3}{2}{k}_{B}T=\frac{1}{2}{E}_G\frac{m_n}{M}=C\frac{3}{10}\frac{GM{m}_n}{R}}$$

where the temperature T is averaged over the star and C is a factor of order one related to the stellar structure and can be estimated from the star approximate polytropic index. Note that this does not hold for large enough stars, where the radiation pressure is larger than the gas pressure in the radiation zone, hence the relation between temperature, mass and radius is different, as elaborated below.

Wrapping up everything, we also take r to be equal to R up to a factor, and n_e at r is replaced by its stellar average up to a factor. The combined factor is approximately 1/15 for the sun, and we get:

$${\displaystyle \begin{array}{rl}L& =-4\pi r^{2}D\frac{\mathrm{\partial }u}{\mathrm{\partial }r}\approx 4\pi R^{2}D\frac{u}{R}\\ L& \approx \frac{1}{15}\frac{64{\pi }^2}{9}\frac{{\sigma }_B}{{\sigma }_{e\cdot \gamma }}\frac{R^4{T}^4{m}_n}{M}\\ & \approx \frac{1}{15}\frac{2{\pi }^3}{9\cdot 5^5}\frac{G^4{m}_e^2{m}_n^5}{{\alpha }^2{\hslash }^5}{M}^3=4\cdot {{10}^{26}}_W{\left(\frac{M}{M_{\odot }}\right)}^3\end{array}}$$

The added factor is actually dependent on M, therefore the law has an approximate ${\displaystyle M^{3.5}}$ dependence.

Distinguishing between small and large stellar masses

Main article: Eddington luminosity

One may distinguish between the cases of small and large stellar masses by deriving the above results using radiation pressure. In this case, it is easier to use the optical opacity ${\displaystyle \kappa }$ and to consider the internal temperature T_I directly; more precisely, one can consider the average temperature in the radiation zone.

The consideration begins by noting the relation between the radiation pressure P_rad and luminosity. The gradient of radiation pressure is equal to the momentum transfer absorbed from the radiation, giving:

$${\displaystyle \frac{d{P}_{rad}}{dr}=-\frac{\kappa \rho }{c}\frac{L}{4\pi r^2},}$$

where c is the velocity of light. Here, ${\displaystyle 1/\kappa \rho =l}$; the photon mean free path.

The radiation pressure is related to the temperature by ${\displaystyle P_{rad}=\frac{4\sigma }{3c}{T_I}^4}$, therefore

$${\displaystyle {T_I}^3\frac{d{T}_I}{dr}=-\frac{3\kappa \rho }{16\sigma }\frac{L}{4\pi r^2},}$$

from which it follows directly that

$${\displaystyle L\propto {T_I}^4\frac{R}{\rho }\propto {T_I}^4\frac{R^4}{M}.}$$

In the radiation zone gravity is balanced by the pressure on the gas coming from both itself (approximated by ideal gas pressure) and from the radiation. For a small enough stellar mass the latter is negligible and one arrives at

$${\displaystyle T_I\propto \frac{M}{R}}$$

as before. More precisely, since integration was done from 0 to R so ${\displaystyle T_I-T_E}$ on the left side, but the surface temperature T_E can be neglected with respect to the internal temperature T_I.

From this it follows directly that

$${\displaystyle L\propto M^3.}$$

For a large enough stellar mass, the radiation pressure is larger than the gas pressure in the radiation zone. Plugging in the radiation pressure, instead of the ideal gas pressure used above, yields

$${\displaystyle {T_I}^4\propto \frac{M^2}{R^4},}$$

hence

$${\displaystyle L\propto M.}$$

Core and surface temperatures

To the first approximation, stars are black body radiators with a surface area of 4πR². Thus, from the Stefan–Boltzmann law, the luminosity is related to the surface temperature T_S, and through it to the color of the star, by

$${\displaystyle L=4\pi R^2{\sigma }_B{T}_S^4}$$

where σ_B is Stefan–Boltzmann constant, 5.67×10⁻⁸ W m⁻² K⁻⁴

The luminosity is equal to the total energy produced by the star per unit time. Since this energy is produced by nucleosynthesis, usually in the star core (this is not true for red giants), the core temperature is related to the luminosity by the nucleosynthesis rate per unit volume:

$${\displaystyle L=\frac{dE}{dt}\approx ϵ\frac{4\pi }{3}{R}^3{n}_A{n}_B\frac{4\sqrt{2}}{\sqrt{3m_R}}\sqrt{E_0(T)}\frac{S(E_0(T))}{kT}{e}^{-\frac{3E_0(T)}{kT}}}$$

Here, ε is the total energy emitted in the chain reaction or reaction cycle. $E_0={\left(\sqrt{E_G}kT/2\right)}^{2/3}$ is the Gamow peak energy, dependent on E_G, the Gamow factor. Additionally, S(E)/E is the reaction cross section, n is number density, ${\displaystyle m_R=m_A\cdot m_B/(m_A+m_B)}$ is the reduced mass for the particle collision, and A,B are the two species participating in the limiting reaction (e.g. both stand for a proton in the proton-proton chain reaction, or A a proton and B an ¹⁴
₇N
nucleus for the CNO cycle).

Since the radius R is itself a function of the temperature and the mass, one may solve this equation to get the core temperature.