In mathematics, the octonions are a normed division algebra over the real numbers, usually represented by the capital letter O, using boldface O or blackboard bold  .
Octonions have eight dimensions; twice the number of dimensions of the
quaternions, of which they are an extension. Along with the real
numbers R, complex numbers C, and quaternions H,
octonions complete the set of numbers capable of being added,
subtracted, multiplied or divided; as such, they are believed by some
researchers to have fundamental importance in physical theory. They are noncommutative and nonassociative, but satisfy a weaker form of associativity; namely, they are alternative.
.
Octonions have eight dimensions; twice the number of dimensions of the
quaternions, of which they are an extension. Along with the real
numbers R, complex numbers C, and quaternions H,
octonions complete the set of numbers capable of being added,
subtracted, multiplied or divided; as such, they are believed by some
researchers to have fundamental importance in physical theory. They are noncommutative and nonassociative, but satisfy a weaker form of associativity; namely, they are alternative.
Octonions are not as well known as the quaternions and complex numbers, which are much more widely studied and used. Despite this, they have some interesting properties and are related to a number of exceptional structures in mathematics, among them the exceptional Lie groups. Additionally, octonions have applications in fields such as string theory, special relativity, and quantum logic. Applying the Cayley-Dickson construction to the octonions produces the sedenions.
The octonions were discovered in 1843 by John T. Graves, inspired by his friend W. R. Hamilton's discovery of quaternions. Graves called his discovery octaves, and mentioned them in a letter to Hamilton dated 16 December 1843, but his first publication of his result in (Graves 1845) was slightly later than Arthur Cayley's article on them. The octonions were discovered independently by Cayley[2] and are sometimes referred to as Cayley numbers or the Cayley algebra. Hamilton described the early history of Graves' discovery.[3]
 .
Octonions have eight dimensions; twice the number of dimensions of the
quaternions, of which they are an extension. Along with the real
numbers R, complex numbers C, and quaternions H,
octonions complete the set of numbers capable of being added,
subtracted, multiplied or divided; as such, they are believed by some
researchers to have fundamental importance in physical theory. They are noncommutative and nonassociative, but satisfy a weaker form of associativity; namely, they are alternative.
.
Octonions have eight dimensions; twice the number of dimensions of the
quaternions, of which they are an extension. Along with the real
numbers R, complex numbers C, and quaternions H,
octonions complete the set of numbers capable of being added,
subtracted, multiplied or divided; as such, they are believed by some
researchers to have fundamental importance in physical theory. They are noncommutative and nonassociative, but satisfy a weaker form of associativity; namely, they are alternative.
Octonions are not as well known as the quaternions and complex numbers, which are much more widely studied and used. Despite this, they have some interesting properties and are related to a number of exceptional structures in mathematics, among them the exceptional Lie groups. Additionally, octonions have applications in fields such as string theory, special relativity, and quantum logic. Applying the Cayley-Dickson construction to the octonions produces the sedenions.
The octonions were discovered in 1843 by John T. Graves, inspired by his friend W. R. Hamilton's discovery of quaternions. Graves called his discovery octaves, and mentioned them in a letter to Hamilton dated 16 December 1843, but his first publication of his result in (Graves 1845) was slightly later than Arthur Cayley's article on them. The octonions were discovered independently by Cayley[2] and are sometimes referred to as Cayley numbers or the Cayley algebra. Hamilton described the early history of Graves' discovery.[3]
Definition
The octonions can be thought of as octets (or 8-tuples) of real numbers. Every octonion is a real linear combination of the unit octonions:Addition and subtraction of octonions is done by adding and subtracting corresponding terms and hence their coefficients, like quaternions. Multiplication is more complex. Multiplication is distributive over addition, so the product of two octonions can be calculated by summing the products of all the terms, again like quaternions. The product of each pair of terms can be given by multiplication of the coefficients and a multiplication table of the unit octonions.
Most off-diagonal elements of the table are antisymmetric, making it almost a skew-symmetric matrix except for the elements on the main diagonal, as well as the row and column for which e0 is an operand.
The table can be summarized as follows:[5]

where
 is the Kronecker delta and
is the Kronecker delta and  is a completely antisymmetric tensor with value +1 when ijk = 123, 145, 176, 246, 257, 347, 365.
is a completely antisymmetric tensor with value +1 when ijk = 123, 145, 176, 246, 257, 347, 365.
The above definition though is not unique, but is only one of 480 possible definitions for octonion multiplication with e0 = 1. The others can be obtained by permuting and changing the signs of the non-scalar basis elements. The 480 different algebras are isomorphic, and there is rarely a need to consider which particular multiplication rule is used. Each of these 480 definitions is invariant up to signs under some 7-cycle of the points (1234567), and for each 7-cycle there are four definitions, differing by signs and reversal of order. A common choice is to use the definition invariant under the 7-cycle (1234567) with e1e2 = e4 as it is particularly easy to remember the multiplication.
A variation of this sometimes used is to label the elements of the basis by the elements ∞, 0, 1, 2, ..., 6, of the projective line over the finite field of order 7. The multiplication is then given by e∞ = 1 and e1e2 = e4, and all expressions obtained from this by adding a constant (mod 7) to all subscripts: in other words using the 7 triples (124) (235) (346) (450) (561) (602) (013). These are the nonzero codewords of the quadratic residue code of length 7 over the Galois field of two elements, GF(2). There is a symmetry of order 7 given by adding a constant mod 7 to all subscripts, and also a symmetry of order 3 given by multiplying all subscripts by one of the quadratic residues 1, 2, 4 mod 7.[6][7]
The multiplication table for a geometric algebra of signature (−−−−) can be given in terms of the following 7 quaternionic triples (omitting the identity element): (I,j,k), (i,J,k), (i,j,K), (I,J,K), (∗I,i,m), (∗J,j,m), (∗K,k,m) in which the lowercase items are vectors (mathematics and physics) and the uppercase ones are bivectors and ∗ = mijk (which is in fact the Hodge star operator). If the ∗ is forced to be equal to the identity then the multiplication ceases to be associative, but the ∗ may be removed from the multiplication table resulting in an octonion multiplication table.
Note that in keeping ∗ = mijk associative and thus not reducing the 4-dimensional geometric algebra to an octonion one, the whole multiplication table can be derived from the equation for ∗. Consider the gamma matrices. The formula defining the fifth gamma matrix shows that it is the ∗ of a four-dimensional geometric algebra of the gamma matrices.
Cayley–Dickson construction
A more systematic way of defining the octonions is via the Cayley–Dickson construction. Just as quaternions can be defined as pairs of complex numbers, the octonions can be defined as pairs of quaternions. Addition is defined pairwise. The product of two pairs of quaternions (a, b) and (c, d) is defined by denotes the conjugate of the quaternion z. This definition is equivalent to the one given above when the eight unit octonions are identified with the pairs
denotes the conjugate of the quaternion z. This definition is equivalent to the one given above when the eight unit octonions are identified with the pairs
- (1,0), (i,0), (j,0), (k,0), (0,1), (0,i), (0,j), (0,k)
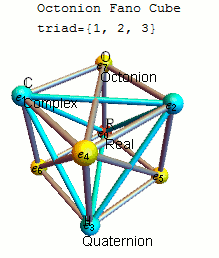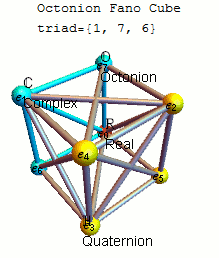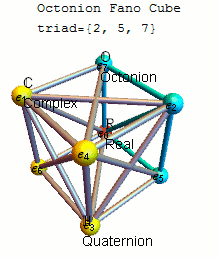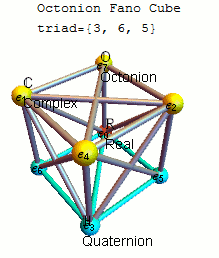
Fano plane mnemonic
A mnemonic for the products of the unit octonions.[8]
A 3D mnemonic visualization showing the 7 triads as hyperplanes through the Real ( ) vertex of the octonion example given above.[8]
) vertex of the octonion example given above.[8]
 ) vertex of the octonion example given above.[8]
) vertex of the octonion example given above.[8]A convenient mnemonic for remembering the products of unit octonions is given by the diagram at the right, which represents the multiplication table of Cayley and Graves.[4][9] This diagram with seven points and seven lines (the circle through 1, 2, and 3 is considered a line) is called the Fano plane. The lines are oriented. The seven points correspond to the seven standard basis elements of Im(O) (see definition below). Each pair of distinct points lies on a unique line and each line runs through exactly three points.
Let (a, b, c) be an ordered triple of points lying on a given line with the order specified by the direction of the arrow. Then multiplication is given by
- ab = c and ba = −c
- 1 is the multiplicative identity,
- ei2 = −1 for each point in the diagram
Conjugate, norm, and inverse
The conjugate of an octonionThe real part of x is given by
Conjugation of octonions satisfies the equation
The existence of a norm on O implies the existence of inverses for every nonzero element of O. The inverse of x ≠ 0 is given by
Properties
Octonionic multiplication is neither commutative:if
are distinct and non-zero,
if
are distinct, non-zero or if
.
The octonions do retain one important property shared by R, C, and H: the norm on O satisfies
Wider number systems exist which have a multiplicative modulus (e.g. 16-dimensional conic sedenions). Their modulus is defined differently from their norm, and they also contain zero divisors.
As shown by Hurwitz, the only normed division algebras over the reals are R, C, H, and O. These four algebras also form the only alternative, finite-dimensional division algebras over the reals (up to isomorphism).
Not being associative, the nonzero elements of O do not form a group. They do, however, form a loop, indeed a Moufang loop.
Commutator and cross product
The commutator of two octonions x and y is given byAutomorphisms
An automorphism, A, of the octonions is an invertible linear transformation of O which satisfiesSee also: PSL(2,7) – the automorphism group of the Fano plane.
Isotopies
An isotopy of an algebra is a triple of bijective linear maps a, b, c such that if xy = z then a(x)b(y) = c(z). For a = b = c this is the same as an automorphism. The isotopy group of an algebra is the group of all isotopies, which contains the group of automorphisms as a subgroup.The isotopy group of the octonions is the group Spin8(R), with a, b, and c acting as the three 8-dimensional representations.[12] The subgroup of elements where c fixes the identity is the subgroup Spin7(R), and the subgroup where a, b, and c all fix the identity is the automorphism group G2.
Integral octonions
There are several natural ways to choose an integral form of the octonions. The simplest is just to take the octonions whose coordinates are integers. This gives a nonassociative algebra over the integers called the Gravesian octonions. However it is not a maximal order (in the sense of ring theory); there are exactly 7 maximal orders containing it. These 7 maximal orders are all equivalent under automorphisms. The phrase "integral octonions" usually refers to a fixed choice of one of these seven orders.These maximal orders were constructed by Kirmse (1925), Dickson and Bruck as follows. Label the 8 basis vectors by the points of the projective plane over the field with 7 elements. First form the "Kirmse integers" : these consist of octonions whose coordinates are integers or half integers, and that are half integers (that is, halves of odd integers) on one of the 16 sets
- ∅ (∞124) (∞235) (∞346) (∞450) (∞561) (∞602) (∞013) (∞0123456) (0356) (1460) (2501) (3612) (4023) (5134) (6245)
The Kirmse integers and the 7 maximal orders are all isometric to the E8 lattice rescaled by a factor of 1/√2. In particular there are 240 elements of minimum nonzero norm 1 in each of these orders, forming a Moufang loop of order 240.
The integral octonions have a "division with remainder" property: given integral octonions a and b ≠ 0, we can find q and r with a = qb + r, where the remainder r has norm less than that of b.
In the integral octonions, all left ideals and right ideals are 2-sided ideals, and the only 2-sided ideals are the principal ideals nO where n is a non-negative integer.
The integral octonions have a version of factorization into primes, though it is not straightforward to state because the octonions are not associative so the product of octonions depends on the order in which one does the products. The irreducible integral octonions are exactly those of prime norm, and every integral octonion can be written as a product of irreducible octonions. More precisely an integral octonion of norm mn can be written as a product of integral octonions of norms m and n.
The automorphism group of the integral octonions is the group G2(F2) of order 12096, which has a simple subgroup of index 2 isomorphic to the unitary group 2A2(32). The isotopy group of the integral octonions is the perfect double cover of the group of rotations of the E8 lattice.











![{\displaystyle [x,y]=xy-yx.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/adaa95a59e9814f5965d79550c54297626db87a9)













































