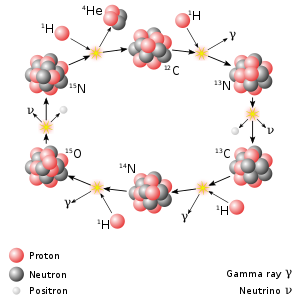Energy transformation

From Wikipedia, the free encyclopedia
https://en.wikipedia.org/wiki/Energy_transformation

Fire is an example of energy transformation

Energy transformation using Energy Systems Language

Energy transformation, also known as energy conversion, is the process of changing energy from one form to another. In physics, energy is a quantity that provides the capacity to perform work or moving (e.g. lifting an object) or provides heat. In addition to being converted, according to the law of conservation of energy, energy is transferable to a different location or object, but it cannot be created or destroyed.

The energy in many of its forms may be used in natural processes, or to provide some service to society such as heating, refrigeration, lighting or performing mechanical work to operate machines. For example, to heat a home, the furnace burns fuel, whose chemical potential energy is converted into thermal energy, which is then transferred to the home's air to raise its temperature.

Limitations in the conversion of thermal energy

Conversions to thermal energy from other forms of energy may occur with 100% efficiency. Conversion among non-thermal forms of energy may occur with fairly high efficiency, though there is always some energy dissipated thermally due to friction and similar processes. Sometimes the efficiency is close to 100%, such as when potential energy is converted to kinetic energy as an object falls in a vacuum. This also applies to the opposite case; for example, an object in an elliptical orbit around another body converts its kinetic energy (speed) into gravitational potential energy (distance from the other object) as it moves away from its parent body. When it reaches the furthest point, it will reverse the process, accelerating and converting potential energy into kinetic. Since space is a near-vacuum, this process has close to 100% efficiency.

Thermal energy is unique because it in most cases (willow) cannot be converted to other forms of energy. Only a difference in the density of thermal/heat energy (temperature) can be used to perform work, and the efficiency of this conversion will be (much) less than 100%. This is because thermal energy represents a particularly disordered form of energy; it is spread out randomly among many available states of a collection of microscopic particles constituting the system (these combinations of position and momentum for each of the particles are said to form a phase space). The measure of this disorder or randomness is entropy, and its defining feature is that the entropy of an isolated system never decreases. One cannot take a high-entropy system (like a hot substance, with a certain amount of thermal energy) and convert it into a low entropy state (like a low-temperature substance, with correspondingly lower energy), without that entropy going somewhere else (like the surrounding air). In other words, there is no way to concentrate energy without spreading out energy somewhere else.

Thermal energy in equilibrium at a given temperature already represents the maximal evening-out of energy between all possible states because it is not entirely convertible to a "useful" form, i.e. one that can do more than just affect temperature. The second law of thermodynamics states that the entropy of a closed system can never decrease. For this reason, thermal energy in a system may be converted to other kinds of energy with efficiencies approaching 100% only if the entropy of the universe is increased by other means, to compensate for the decrease in entropy associated with the disappearance of the thermal energy and its entropy content. Otherwise, only a part of that thermal energy may be converted to other kinds of energy (and thus useful work). This is because the remainder of the heat must be reserved to be transferred to a thermal reservoir at a lower temperature. The increase in entropy for this process is greater than the decrease in entropy associated with the transformation of the rest of the heat into other types of energy.

In order to make energy transformation more efficient, it is desirable to avoid thermal conversion. For example, the efficiency of nuclear reactors, where the kinetic energy of the nuclei is first converted to thermal energy and then to electrical energy, lies at around 35%. By direct conversion of kinetic energy to electric energy, effected by eliminating the intermediate thermal energy transformation, the efficiency of the energy transformation process can be dramatically improved.

History of energy transformation

Energy transformations in the universe over time are usually characterized by various kinds of energy, which have been available since the Big Bang, later being "released" (that is, transformed to more active types of energy such as kinetic or radiant energy) by a triggering mechanism.

Release of energy from gravitational potential

A direct transformation of energy occurs when hydrogen produced in the Big Bang collects into structures such as planets, in a process during which part of the gravitational potential is to be converted directly into heat. In Jupiter, Saturn, and Neptune, for example, such heat from the continued collapse of the planets' large gas atmospheres continue to drive most of the planets' weather systems. These systems, consisting of atmospheric bands, winds, and powerful storms, are only partly powered by sunlight. However, on Uranus, little of this process occurs.

On Earth, a significant portion of the heat output from the interior of the planet, estimated at a third to half of the total, is caused by the slow collapse of planetary materials to a smaller size, generating heat.

Release of energy from radioactive potential

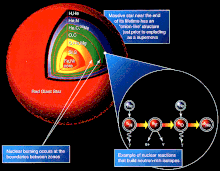
Familiar examples of other such processes transforming energy from the Big Bang include nuclear decay, which releases energy that was originally "stored" in heavy isotopes, such as uranium and thorium. This energy was stored at the time of the nucleosynthesis of these elements. This process uses the gravitational potential energy released from the collapse of Type II supernovae to create these heavy elements before they are incorporated into star systems such as the Solar System and the Earth. The energy locked into uranium is released spontaneously during most types of radioactive decay, and can be suddenly released in nuclear fission bombs. In both cases, a portion of the energy binding the atomic nuclei together is released as heat.

Release of energy from hydrogen fusion potential

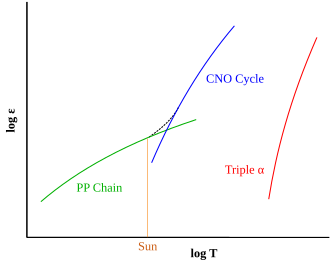
In a similar chain of transformations beginning at the dawn of the universe, nuclear fusion of hydrogen in the Sun releases another store of potential energy which was created at the time of the Big Bang. At that time, according to one theory, space expanded and the universe cooled too rapidly for hydrogen to completely fuse into heavier elements. This resulted in hydrogen representing a store of potential energy which can be released by nuclear fusion. Such a fusion process is triggered by heat and pressure generated from the gravitational collapse of hydrogen clouds when they produce stars, and some of the fusion energy is then transformed into starlight. Considering the solar system, starlight, overwhelmingly from the Sun, may again be stored as gravitational potential energy after it strikes the Earth. This occurs in the case of avalanches, or when water evaporates from oceans and is deposited as precipitation high above sea level (where, after being released at a hydroelectric dam, it can be used to drive turbine/generators to produce electricity).

Sunlight also drives many weather phenomena on Earth. One example is a hurricane, which occurs when large unstable areas of warm ocean, heated over months, give up some of their thermal energy suddenly to power a few days of violent air movement. Sunlight is also captured by plants as a chemical potential energy via photosynthesis, when carbon dioxide and water are converted into a combustible combination of carbohydrates, lipids, and oxygen. The release of this energy as heat and light may be triggered suddenly by a spark, in a forest fire; or it may be available more slowly for animal or human metabolism when these molecules are ingested, and catabolism is triggered by enzyme action.

Through all of these transformation chains, the potential energy stored at the time of the Big Bang is later released by intermediate events, sometimes being stored in several different ways for long periods between releases, as more active energy. All of these events involve the conversion of one kind of energy into others, including heat.

Examples

Examples of sets of energy conversions in machines

A coal-fired power plant involves these energy transformations:

1. Chemical energy in the coal is converted into thermal energy in the exhaust gases of combustion
2. Thermal energy of the exhaust gases converted into thermal energy of steam through heat exchange
3. Kinetic energy of steam converted to mechanical energy in the turbine
4. Mechanical energy of the turbine is converted to electrical energy by the generator, which is the ultimate output

In such a system, the first and fourth steps are highly efficient, but the second and third steps are less efficient. The most efficient gas-fired electrical power stations can achieve 50% conversion efficiency.^{[citation needed]} Oil- and coal-fired stations are less efficient.

In a conventional automobile, the following energy transformations occur:

1. Chemical energy in the fuel is converted into kinetic energy of expanding gas via combustion
2. Kinetic energy of expanding gas converted to the linear piston movement
3. Linear piston movement converted to rotary crankshaft movement
4. Rotary crankshaft movement passed into transmission assembly
5. Rotary movement passed out of transmission assembly
6. Rotary movement passed through a differential
7. Rotary movement passed out of differential to drive wheels
8. Rotary movement of drive wheels converted to linear motion of the vehicle

Other energy conversions

Lamatalaventosa Wind Farm

There are many different machines and transducers that convert one energy form into another. A short list of examples follows:

• ATP hydrolysis (chemical energy in adenosine triphosphate → mechanical energy)
• Battery (electricity) (chemical energy → electrical energy)
• Electric generator (kinetic energy or mechanical work → electrical energy)
• Electric heater (electric energy → heat)
• Fire (chemical energy → heat and light)
• Friction (kinetic energy → heat)
• Fuel cell (chemical energy → electrical energy)
• Geothermal power (heat→ electrical energy)
• Heat engines, such as the internal combustion engine used in cars, or the steam engine (heat → mechanical energy)
• Hydroelectric dam (gravitational potential energy → electrical energy)
• Electric lamp (electrical energy → heat and light)
• Microphone (sound → electrical energy)
• Ocean thermal power (heat → electrical energy)
• Photosynthesis (electromagnetic radiation → chemical energy)
• Piezoelectrics (strain → electrical energy)
• Thermoelectric (heat → electrical energy)
• Wave power (mechanical energy → electrical energy)
• Windmill (wind energy → electrical energy or mechanical energy)

Natural-gas processing

From Wikipedia, the free encyclopedia

A natural-gas processing plant in Aderklaa, Austria

Natural-gas processing is a range of industrial processes designed to purify raw natural gas by removing contaminants such as solids, water, carbon dioxide (CO₂), hydrogen sulfide (H₂S), mercury and higher molecular mass hydrocarbons (condensate) to produce pipeline quality dry natural gas for pipeline distribution and final use. Some of the substances which contaminate natural gas have economic value and are further processed or sold. Hydrocarbons that are liquid at ambient conditions: temperature and pressure (i.e., pentane and heavier) are called natural-gas condensate (sometimes also called natural gasoline or simply condensate).

Raw natural gas comes primarily from three types of wells: crude oil wells, gas wells, and condensate wells. Crude oil and natural gas are often found together in the same reservoir. Natural gas produced in wells with crude oil is generally classified as associated-dissolved gas as the gas had been associated with or dissolved in crude oil. Natural gas production not associated with crude oil is classified as “non-associated.” In 2009, 89 percent of U.S. wellhead production of natural gas was non-associated. Non-associated gas wells producing a dry gas in terms of condensate and water can send the dry gas directly to a pipeline or gas plant without undergoing any separation processIng allowing immediate use.

Natural-gas processing begins underground or at the well-head. In a crude oil well, natural gas processing begins as the fluid loses pressure and flows through the reservoir rocks until it reaches the well tubing. In other wells, processing begins at the wellhead which extracts the composition of natural gas according to the type, depth, and location of the underground deposit and the geology of the area.

Natural gas when relatively free of hydrogen sulfide is called sweet gas; natural gas that contains elevated hydrogen sulfide levels is called sour gas; natural gas, or any other gas mixture, containing significant quantities of hydrogen sulfide or carbon dioxide or similar acidic gases, is called acid gas.

Types of raw-natural-gas wells

• Crude oil wells: Natural gas that comes from crude oil wells is typically called associated gas. This gas could exist as a separate gas cap above the crude oil in the underground reservoir or could be dissolved in the crude oil, ultimately coming out of solution as the pressure is reduced during production. Condensate produced from oil wells is often referred to as lease condensate.
• Dry gas wells: These wells typically produce only raw natural gas that contains no condensate with little to no crude oil and are called non-associated gas. Condensate from dry gas is extracted at gas processing plants and is often called plant condensate.
• Condensate wells: These wells typically produce raw natural gas along with natural gas liquid with little to no crude oil and are called non-associated gas. Such raw natural gas is often referred to as wet gas.
• Coal seam wells: These wells typically produce raw natural gas from methane deposits in the pores of coal seams, often existing underground in a more concentrated state of adsorption onto the surface of the coal itself. Such gas is referred to as coalbed gas or coalbed methane (coal seam gas in Australia). Coalbed gas has become an important source of energy in recent decades.

Contaminants in raw natural gas

See also: Natural-gas condensate

Raw natural gas typically consists primarily of methane (CH₄) and ethane (C₂H₆), the shortest and lightest hydrocarbon molecules. It often also contains varying amounts of:

• Heavier gaseous hydrocarbons: propane (C₃H₈), normal butane (n-C₄H₁₀), isobutane (i-C₄H₁₀) and pentanes. All of these are collectively referred to as Natural Gas Liquids or NGL and can be processed into finished by-products.
• Liquid hydrocarbons (also referred to as casinghead gasoline or natural gasoline) and/or crude oil.
• Acid gases: carbon dioxide (CO₂), hydrogen sulfide (H₂S) and mercaptans such as methanethiol (CH₃SH) and ethanethiol (C₂H₅SH).
• Other gases: nitrogen (N₂) and helium (He).
• Water: water vapor and liquid water. Also dissolved salts and dissolved gases (acids).
• Mercury: minute amounts of mercury primarily in elemental form, but chlorides and other species are possibly present.
• Naturally occurring radioactive material (NORM): natural gas may contain radon, and the produced water may contain dissolved traces of radium, which can accumulate within piping and processing equipment; rendering piping and equipment radioactive over time.

Natural gas quality standards

Raw natural gas must be purified to meet the quality standards specified by the major pipeline transmission and distribution companies. Those quality standards vary from pipeline to pipeline and are usually a function of a pipeline system's design and the markets that it serves. In general, the standards specify that the natural gas:

• Be within a specific range of heating value (caloric value). For example, in the United States, it should be about 1035 ± 5% BTU per cubic foot of gas at 1 atmosphere and 60 °F (41 MJ ± 5% per cubic metre of gas at 1 atmosphere and 15.6 °C). In the United Kingdom the gross calorific value must be in the range 37.0 – 44.5 MJ/m³ for entry into the National Transmission System (NTS).
• Be delivered at or above a specified hydrocarbon dew point temperature (below which some of the hydrocarbons in the gas might condense at pipeline pressure forming liquid slugs that could damage the pipeline.) Hydrocarbon dew-point adjustment reduces the concentration of heavy hydrocarbons so no condensation occurs during the ensuing transport in the pipelines. In the UK the hydrocarbon dew point is defined as <-2 °C for entry into the NTS. The hydrocarbon dewpoint changes with the prevailing ambient temperature, the seasonal variation is:

Seasonal variation of hydrocarbon dewpoint

Hydrocarbon dewpoint	30 °F (–1.1 °C)	35 °F (1.7 °C)	40 °F (4.4 °C)	45 °F (7.2 °C)	50 °F (10 °C)
Months	December January February March	April November	May October	June September	July August

The natural gas should:

• Be free of particulate solids and liquid water to prevent erosion, corrosion or other damage to the pipeline.
• Be dehydrated of water vapor sufficiently to prevent the formation of methane hydrates within the gas processing plant or subsequently within the sales gas transmission pipeline. A typical water content specification in the U.S. is that gas must contain no more than seven pounds of water per million standard cubic feet of gas. In the UK this is defined as <-10 °C @ 85barg for entry into the NTS.
• Contain no more than trace amounts of components such as hydrogen sulfide, carbon dioxide, mercaptans, and nitrogen. The most common specification for hydrogen sulfide content is 0.25 grain H₂S per 100 cubic feet of gas, or approximately 4 ppm. Specifications for CO₂ typically limit the content to no more than two or three percent. In the UK hydrogen sulfide is specified ≤5 mg/m³ and total sulfur as ≤50 mg/m³, carbon dioxide as ≤2.0% (molar), and nitrogen as ≤5.0% (molar) for entry into the NTS.
• Maintain mercury at less than detectable limits (approximately 0.001 ppb by volume) primarily to avoid damaging equipment in the gas processing plant or the pipeline transmission system from mercury amalgamation and embrittlement of aluminum and other metals.

Description of a natural-gas processing plant

There are a variety of ways in which to configure the various unit processes used in the treatment of raw natural gas. The block flow diagram below is a generalized, typical configuration for the processing of raw natural gas from non-associated gas wells showing how raw natural gas is processed into sales gas piped to the end user markets. and various byproducts:

• Natural-gas condensate
• Sulfur
• Ethane
• Natural gas liquids (NGL): propane, butanes and C₅+ (which is the commonly used term for pentanes plus higher molecular weight hydrocarbons)

Raw natural gas is commonly collected from a group of adjacent wells and is first processed in a separator vessels at that collection point for removal of free liquid water and natural gas condensate. The condensate is usually then transported to an oil refinery and the water is treated and disposed of as wastewater.

The raw gas is then piped to a gas processing plant where the initial purification is usually the removal of acid gases (hydrogen sulfide and carbon dioxide). There are several processes available for that purpose as shown in the flow diagram, but amine treating is the process that was historically used. However, due to a range of performance and environmental constraints of the amine process, a newer technology based on the use of polymeric membranes to separate the carbon dioxide and hydrogen sulfide from the natural gas stream has gained increasing acceptance. Membranes are attractive since no reagents are consumed.

The acid gases, if present, are removed by membrane or amine treating and can then be routed into a sulfur recovery unit which converts the hydrogen sulfide in the acid gas into either elemental sulfur or sulfuric acid. Of the processes available for these conversions, the Claus process is by far the most well known for recovering elemental sulfur, whereas the conventional Contact process and the WSA (Wet sulfuric acid process) are the most used technologies for recovering sulfuric acid. Smaller quantities of acid gas may be disposed of by flaring.

The residual gas from the Claus process is commonly called tail gas and that gas is then processed in a tail gas treating unit (TGTU) to recover and recycle residual sulfur-containing compounds back into the Claus unit. Again, as shown in the flow diagram, there are a number of processes available for treating the Claus unit tail gas and for that purpose a WSA process is also very suitable since it can work autothermally on tail gases.

The next step in the gas processing plant is to remove water vapor from the gas using either the regenerable absorption in liquid triethylene glycol (TEG), commonly referred to as glycol dehydration, deliquescent chloride desiccants, and or a Pressure Swing Adsorption (PSA) unit which is regenerable adsorption using a solid adsorbent. Other newer processes like membranes may also be considered.

Mercury is then removed by using adsorption processes (as shown in the flow diagram) such as activated carbon or regenerable molecular sieves.

Although not common, nitrogen is sometimes removed and rejected using one of the three processes indicated on the flow diagram:

• Cryogenic process (Nitrogen Rejection Unit), using low temperature distillation. This process can be modified to also recover helium, if desired (see also industrial gas).
• Absorption process, using lean oil or a special solvent as the absorbent.
• Adsorption process, using activated carbon or molecular sieves as the adsorbent. This process may have limited applicability because it is said to incur the loss of butanes and heavier hydrocarbons.

NGL fractionation train

The NGL fractionation process treats offgas from the separators at an oil terminal or the overhead fraction from a crude distillation column in a refinery. Fractionation aims to produce useful products including natural gas suitable for piping to industrial and domestic consumers; liquefied petroleum gases (Propane and Butane) for sale; and gasoline feedstock for liquid fuel blending. The recovered NGL stream is processed through a fractionation train consisting of up to five distillation towers in series: a demethanizer, a deethanizer, a depropanizer, a debutanizer and a butane splitter. The fractionation train typically uses a cryogenic low temperature distillation process involving expansion of the recovered NGL through a turbo-expander followed by distillation in a demethanizing fractionating column. Some gas processing plants use lean oil absorption process rather than the cryogenic turbo-expander process.

The gaseous feed to the NGL fractionation plant is typically compressed to about 60 barg and 37 °C. The feed is cooled to -22 °C, by exchange with the demethanizer overhead product and by a refrigeration system and is split into three streams:

• condensed liquid passes through a Joule-Thomson valve reducing the pressure to 20 bar and enters the demethanizzer as the lower feed at -44.7 °C.
• some of the vapour is routed through a turbo-expander and enters the demethanizer as the upper feed at -64 °C.
• the remaining vapor is chilled by the demethanizer overhead product and Joule-Thomson cooling (through a valve) and enters the column as reflux at -96 °C.

The overhead product is mainly methane at 20 bar and -98 °C. This is heated and compressed to yield a sales gas at 20 bar and 40 °C. The bottom product is NGL at 20 barg which is fed to the deethanizer.  

The overhead product from the deethanizer is ethane and the bottoms are fed to the depropanizer. The overhead product from the depropanizer is propane and the bottoms are fed to the debutanizer. The overhead product from the debutanizer is a mixture of normal and iso-butane, and the bottoms product is a C₅+ gasoline mixture.

The operating conditions of the vessels in the NGL fractionation train are typically as follows.

NGL column operating conditions

	Demethanizer	Deethanizer	Depropanizer	Debutanizer	Butane Splitter
Feed pressure	60 barg	30 barg
Feed temperature	37 °C	25 °C	37 °C	125 °C	59 °C
Column operating pressure	20 barg	26-30 barg	10-16.2 barg	3.8-17 barg	4.9-7 barg
Overhead product temperature	-98°C		50 °C	59 °C	49 °C
Bottom product temperature	12 °C	37 °C	125 °C	118 °C	67 °C
Overhead product	Methane (natural gas)	Ethane	Propane	Butane	Isobutane
Bottom product	Natural gas liquids	(Depropanizer feed)	(Debutanizer feed)	Gasoline	Normal Butane

A typical composition of the feed and product is as follows.

Stream composition, % volume

Component	Feed	NGL	Ethane	Propane	Isobutane	n-Butane	Gasoline
Methane	89.4	0.5	1.36
Ethane	4.9	37.0	95.14	7.32
Propane	2.2	26.0	3.5	90.18	2.0
Isobutane	1.3	7.2		2.5	96.0	4.5
n-Butane	2.2	14.8			2.0	95.0	3.0
Isopentane		5.0					33.13
n-Pentane		3.5				0.5	23.52
n-Hexane		4.0					26.9
n-Heptane		2.0					13.45
Total	100	100	100	100	100	100	100

Sweetening Units

The recovered streams of propane, butanes and C₅+ may be "sweetened" in a Merox process unit to convert undesirable mercaptans into disulfides and, along with the recovered ethane, are the final NGL by-products from the gas processing plant. Currently, most cryogenic plants do not include fractionation for economic reasons, and the NGL stream is instead transported as a mixed product to standalone fractionation complexes located near refineries or chemical plants that use the components for feedstock. In case laying pipeline is not possible for geographical reason, or the distance between source and consumer exceed 3000 km, natural gas is then transported by ship as LNG (liquefied natural gas) and again converted into its gaseous state in the vicinity of the consumer.

Products

The residue gas from the NGL recovery section is the final, purified sales gas which is pipelined to the end-user markets. Rules and agreements are made between buyer and seller regarding the quality of the gas. These usually specify the maximum allowable concentration of CO₂, H₂S and H₂O as well as requiring the gas to be commercially free from objectionable odours and materials, and dust or other solid or liquid matter, waxes, gums and gum forming constituents, which might damage or adversely affect operation of the buyers equipment. When an upset occurs on the treatment plant buyers can usually refuse to accept the gas, lower the flow rate or re-negotiate the price.

Helium recovery

If the gas has significant helium content, the helium may be recovered by fractional distillation. Natural gas may contain as much as 7% helium, and is the commercial source of the noble gas. For instance, the Hugoton Gas Field in Kansas and Oklahoma in the United States contains concentrations of helium from 0.3% to 1.9%, which is separated out as a valuable byproduct.

Stellar nucleosynthesis

From Wikipedia, the free encyclopedia
https://en.wikipedia.org/wiki/Stellar_nucleosynthesis

Logarithm of the relative energy output (ε) of proton–proton (PP), CNO and Triple-α fusion processes at different temperatures (T). The dashed line shows the combined energy generation of the PP and CNO processes within a star. At the Sun's core temperature, the PP process is more efficient.

Stellar nucleosynthesis is the creation (nucleosynthesis) of chemical elements by nuclear fusion reactions within stars. Stellar nucleosynthesis has occurred since the original creation of hydrogen, helium and lithium during the Big Bang. As a predictive theory, it yields accurate estimates of the observed abundances of the elements. It explains why the observed abundances of elements change over time and why some elements and their isotopes are much more abundant than others. The theory was initially proposed by Fred Hoyle in 1946, who later refined it in 1954. Further advances were made, especially to nucleosynthesis by neutron capture of the elements heavier than iron, by Margaret and Geoffrey Burbidge, William Alfred Fowler and Fred Hoyle in their famous 1957 B²FH paper, which became one of the most heavily cited papers in astrophysics history.

Stars evolve because of changes in their composition (the abundance of their constituent elements) over their lifespans, first by burning hydrogen (main sequence star), then helium (horizontal branch star), and progressively burning higher elements. However, this does not by itself significantly alter the abundances of elements in the universe as the elements are contained within the star. Later in its life, a low-mass star will slowly eject its atmosphere via stellar wind, forming a planetary nebula, while a higher–mass star will eject mass via a sudden catastrophic event called a supernova. The term supernova nucleosynthesis is used to describe the creation of elements during the explosion of a massive star or white dwarf.

The advanced sequence of burning fuels is driven by gravitational collapse and its associated heating, resulting in the subsequent burning of carbon, oxygen and silicon. However, most of the nucleosynthesis in the mass range A = 28–56 (from silicon to nickel) is actually caused by the upper layers of the star collapsing onto the core, creating a compressional shock wave rebounding outward. The shock front briefly raises temperatures by roughly 50%, thereby causing furious burning for about a second. This final burning in massive stars, called explosive nucleosynthesis or supernova nucleosynthesis, is the final epoch of stellar nucleosynthesis.

A stimulus to the development of the theory of nucleosynthesis was the discovery of variations in the abundances of elements found in the universe. The need for a physical description was already inspired by the relative abundances of the chemical elements in the solar system. Those abundances, when plotted on a graph as a function of the atomic number of the element, have a jagged sawtooth shape that varies by factors of tens of millions (see history of nucleosynthesis theory). This suggested a natural process that is not random. A second stimulus to understanding the processes of stellar nucleosynthesis occurred during the 20th century, when it was realized that the energy released from nuclear fusion reactions accounted for the longevity of the Sun as a source of heat and light.

History

In 1920, Arthur Eddington proposed that stars obtained their energy from nuclear fusion of hydrogen to form helium and also raised the possibility that the heavier elements are produced in stars.

In 1920, Arthur Eddington, on the basis of the precise measurements of atomic masses by F.W. Aston and a preliminary suggestion by Jean Perrin, proposed that stars obtained their energy from nuclear fusion of hydrogen to form helium and raised the possibility that the heavier elements are produced in stars. This was a preliminary step toward the idea of stellar nucleosynthesis. In 1928 George Gamow derived what is now called the Gamow factor, a quantum-mechanical formula yielding the probability for two contiguous nuclei to overcome the electrostatic Coulomb barrier between them and approach each other closely enough to undergo nuclear reaction due to the strong nuclear force which is effective only at very short distances. In the following decade the Gamow factor was used by Atkinson and Houtermans and later by Edward Teller and Gamow himself to derive the rate at which nuclear reactions would occur at the high temperatures believed to exist in stellar interiors.

In 1939, in a Nobel lecture entitled "Energy Production in Stars", Hans Bethe analyzed the different possibilities for reactions by which hydrogen is fused into helium. He defined two processes that he believed to be the sources of energy in stars. The first one, the proton–proton chain reaction, is the dominant energy source in stars with masses up to about the mass of the Sun. The second process, the carbon–nitrogen–oxygen cycle, which was also considered by Carl Friedrich von Weizsäcker in 1938, is more important in more massive main-sequence stars. These works concerned the energy generation capable of keeping stars hot. A clear physical description of the proton–proton chain and of the CNO cycle appears in a 1968 textbook. Bethe's two papers did not address the creation of heavier nuclei, however. That theory was begun by Fred Hoyle in 1946 with his argument that a collection of very hot nuclei would assemble thermodynamically into iron. Hoyle followed that in 1954 with a paper describing how advanced fusion stages within massive stars would synthesize the elements from carbon to iron in mass.

Hoyle's theory was extended to other processes, beginning with the publication of the 1957 review paper "Synthesis of the Elements in Stars" by Burbidge, Burbidge, Fowler and Hoyle, more commonly referred to as the B²FH paper. This review paper collected and refined earlier research into a heavily cited picture that gave promise of accounting for the observed relative abundances of the elements; but it did not itself enlarge Hoyle's 1954 picture for the origin of primary nuclei as much as many assumed, except in the understanding of nucleosynthesis of those elements heavier than iron by neutron capture. Significant improvements were made by Alastair G. W. Cameron and by Donald D. Clayton. In 1957 Cameron presented his own independent approach to nucleosynthesis, informed by Hoyle's example, and introduced computers into time-dependent calculations of evolution of nuclear systems. Clayton calculated the first time-dependent models of the s-process in 1961 and of the r-process in 1965, as well as of the burning of silicon into the abundant alpha-particle nuclei and iron-group elements in 1968, and discovered radiogenic chronologies for determining the age of the elements.

Cross section of a supergiant showing nucleosynthesis and elements formed.

Key reactions

A version of the periodic table indicating the origins – including stellar nucleosynthesis – of the elements.

The most important reactions in stellar nucleosynthesis:

• Hydrogen fusion:
  • Deuterium fusion
  • The proton–proton chain
  • The carbon–nitrogen–oxygen cycle
• Helium fusion:
  • The triple-alpha process
  • The alpha process
• Fusion of heavier elements:
  • Lithium burning: a process found most commonly in brown dwarfs
  • Carbon-burning process
  • Neon-burning process
  • Oxygen-burning process
  • Silicon-burning process
• Production of elements heavier than iron:
  • Neutron capture:
    • The r-process
    • The s-process
  • Proton capture:
    • The rp-process
    • The p-process
  • Photodisintegration

Hydrogen fusion

Main articles: Proton–proton chain reaction, CNO cycle, and Deuterium fusion

 

Proton–proton chain reaction

CNO-I cycle
 

The helium nucleus is released at the top-left step.

Hydrogen fusion (nuclear fusion of four protons to form a helium-4 nucleus) is the dominant process that generates energy in the cores of main-sequence stars. It is also called "hydrogen burning", which should not be confused with the chemical combustion of hydrogen in an oxidizing atmosphere. There are two predominant processes by which stellar hydrogen fusion occurs: proton–proton chain and the carbon–nitrogen–oxygen (CNO) cycle. Ninety percent of all stars, with the exception of white dwarfs, are fusing hydrogen by these two processes.

In the cores of lower-mass main-sequence stars such as the Sun, the dominant energy production process is the proton–proton chain reaction. This creates a helium-4 nucleus through a sequence of reactions that begin with the fusion of two protons to form a deuterium nucleus (one proton plus one neutron) along with an ejected positron and neutrino. In each complete fusion cycle, the proton–proton chain reaction releases about 26.2 MeV. The proton–proton chain reaction cycle is relatively insensitive to temperature; a 10% rise of temperature would increase energy production by this method by 46%, hence, this hydrogen fusion process can occur in up to a third of the star's radius and occupy half the star's mass. For stars above 35% of the Sun's mass, the energy flux toward the surface is sufficiently low and energy transfer from the core region remains by radiative heat transfer, rather than by convective heat transfer. As a result, there is little mixing of fresh hydrogen into the core or fusion products outward.

In higher-mass stars, the dominant energy production process is the CNO cycle, which is a catalytic cycle that uses nuclei of carbon, nitrogen and oxygen as intermediaries and in the end produces a helium nucleus as with the proton–proton chain. During a complete CNO cycle, 25.0 MeV of energy is released. The difference in energy production of this cycle, compared to the proton–proton chain reaction, is accounted for by the energy lost through neutrino emission. The CNO cycle is very temperature sensitive, a 10% rise of temperature would produce a 350% rise in energy production. About 90% of the CNO cycle energy generation occurs within the inner 15% of the star's mass, hence it is strongly concentrated at the core. This results in such an intense outward energy flux that convective energy transfer becomes more important than does radiative transfer. As a result, the core region becomes a convection zone, which stirs the hydrogen fusion region and keeps it well mixed with the surrounding proton-rich region. This core convection occurs in stars where the CNO cycle contributes more than 20% of the total energy. As the star ages and the core temperature increases, the region occupied by the convection zone slowly shrinks from 20% of the mass down to the inner 8% of the mass. The Sun produces on the order of 1% of its energy from the CNO cycle.

The type of hydrogen fusion process that dominates in a star is determined by the temperature dependency differences between the two reactions. The proton–proton chain reaction starts at temperatures about 4×10⁶ K, making it the dominant fusion mechanism in smaller stars. A self-maintaining CNO chain requires a higher temperature of approximately 16×10⁶ K, but thereafter it increases more rapidly in efficiency as the temperature rises, than does the proton–proton reaction. Above approximately 17×10⁶ K, the CNO cycle becomes the dominant source of energy. This temperature is achieved in the cores of main-sequence stars with at least 1.3 times the mass of the Sun. The Sun itself has a core temperature of about 15.7×10⁶ K. As a main-sequence star ages, the core temperature will rise, resulting in a steadily increasing contribution from its CNO cycle.

Helium fusion

Main articles: Triple-alpha process and Alpha process

Main sequence stars accumulate helium in their cores as a result of hydrogen fusion, but the core does not become hot enough to initiate helium fusion. Helium fusion first begins when a star leaves the red giant branch after accumulating sufficient helium in its core to ignite it. In stars around the mass of the Sun, this begins at the tip of the red giant branch with a helium flash from a degenerate helium core, and the star moves to the horizontal branch where it burns helium in its core. More massive stars ignite helium in their core without a flash and execute a blue loop before reaching the asymptotic giant branch. Such a star initially moves away from the AGB toward bluer colours, then loops back again to what is called the Hayashi track. An important consequence of blue loops is that they give rise to classical Cepheid variables, of central importance in determining distances in the Milky Way and to nearby galaxies Despite the name, stars on a blue loop from the red giant branch are typically not blue in colour but are rather yellow giants, possibly Cepheid variables. They fuse helium until the core is largely carbon and oxygen. The most massive stars become supergiants when they leave the main sequence and quickly start helium fusion as they become red supergiants. After the helium is exhausted in the core of a star, helium fusion will continue in a shell around the carbon–oxygen core.

In all cases, helium is fused to carbon via the triple-alpha process, i.e., three helium nuclei are transformed into carbon via ⁸Be. This can then form oxygen, neon, and heavier elements via the alpha process. In this way, the alpha process preferentially produces elements with even numbers of protons by the capture of helium nuclei. Elements with odd numbers of protons are formed by other fusion pathways.

Reaction rate

The reaction rate density between species A and B, having number densities n_A,B, is given by:

${\displaystyle r=n_A{n}_{B}k}$

where k is the reaction rate constant of each single elementary binary reaction composing the nuclear fusion process:

${\displaystyle k=⟨\sigma (v)v⟩}$

here, σ(v) is the cross-section at relative velocity v, and averaging is performed over all velocities.

Semi-classically, the cross section is proportional to ${\displaystyle \pi {\lambda }^2}$, where ${\displaystyle \lambda =h/p}$ is the de Broglie wavelength. Thus semi-classically the cross section is proportional to $\frac{m}{E}$.

However, since the reaction involves quantum tunneling, there is an exponential damping at low energies that depends on Gamow factor E_G, giving an Arrhenius equation:

${\displaystyle \sigma (E)=\frac{S(E)}{E}{e}^{-\sqrt{\frac{E_{\text{G}}}{E}}}}$

where S(E) depends on the details of the nuclear interaction, and has the dimension of an energy multiplied for a cross section.

One then integrates over all energies to get the total reaction rate, using the Maxwell–Boltzmann distribution and the relation:

${\displaystyle \frac{r}{V}=n_A{n}_B{\int }_0^{\mathrm{\infty }}\frac{S(E)}{E}{e}^{-\sqrt{\frac{E_{\text{G}}}{E}}}2\sqrt{\frac{E}{\pi (kT{)}^3}}{e}^{-\frac{E}{kT}}\sqrt{\frac{2E}{m_{\text{R}}}}dE}$

where ${\displaystyle m_{\text{R}}=\frac{m_1{m}_2}{m_1+m_2}}$ is the reduced mass.

Since this integration has an exponential damping at high energies of the form ${\displaystyle \sim e^{-\frac{E}{kT}}}$ and at low energies from the Gamow factor, the integral almost vanished everywhere except around the peak, called Gamow peak, at E₀, where:

${\displaystyle \frac{\mathrm{\partial }}{\mathrm{\partial }E}\left(-\sqrt{\frac{E_{\text{G}}}{E}}-\frac{E}{kT}\right)=0}$

Thus:

${\displaystyle E_0={\left(\frac{1}{2}kT\sqrt{E_{\text{G}}}\right)}^{\frac{2}{3}}}$

The exponent can then be approximated around E₀ as:

${\displaystyle e^{-\frac{E}{kT}-\sqrt{\frac{E_{\text{G}}}{E}}}\approx e^{-\frac{3E_0}{kT}}\exp \left(-\frac{(E-E_0{)}^2}{\frac{4}{3}{E}_{0}kT}\right)}$

And the reaction rate is approximated as:

${\displaystyle \frac{r}{V}\approx n_A{n}_B\frac{4\sqrt{2}}{\sqrt{3m_{\text{R}}}}\sqrt{E_0}\frac{S(E_0)}{kT}{e}^{-\frac{3E_0}{kT}}}$

Values of S(E₀) are typically 10⁻³ – 10³ keV·b, but are damped by a huge factor when involving a beta decay, due to the relation between the intermediate bound state (e.g. diproton) half-life and the beta decay half-life, as in the proton–proton chain reaction. Note that typical core temperatures in main-sequence stars give kT of the order of keV.

Thus, the limiting reaction in the CNO cycle, proton capture by ¹⁴
₇N
, has S(E₀) ~ S(0) = 3.5 keV·b, while the limiting reaction in the proton–proton chain reaction, the creation of deuterium from two protons, has a much lower S(E₀) ~ S(0) = 4×10⁻²² keV·b. Incidentally, since the former reaction has a much higher Gamow factor, and due to the relative abundance of elements in typical stars, the two reaction rates are equal at a temperature value that is within the core temperature ranges of main-sequence stars.