Molecular symmetry

From Wikipedia, the free encyclopedia

Molecular symmetry in chemistry describes the symmetry present in molecules and the classification of molecules according to their symmetry. Molecular symmetry is a fundamental concept in chemistry, as it can predict or explain many of a molecule's chemical properties, such as its dipole moment and its allowed spectroscopic transitions (based on selection rules such as the Laporte rule). Many university level textbooks on physical chemistry, quantum chemistry, and inorganic chemistry devote a chapter to symmetry.^{[1][2][3][4][5]}

While various frameworks for the study of molecular symmetry exist, group theory is the predominant one. This framework is also useful in studying the symmetry of molecular orbitals, with applications such as the Hückel method, ligand field theory, and the Woodward-Hoffmann rules. Another framework on a larger scale is the use of crystal systems to describe crystallographic symmetry in bulk materials.

Many techniques for the practical assessment of molecular symmetry exist, including X-ray crystallography and various forms of spectroscopy, for example infrared spectroscopy of metal carbonyls. Spectroscopic notation is based on symmetry considerations.

Symmetry concepts

The study of symmetry in molecules is an adaptation of mathematical group theory.

Elements

The symmetry of a molecule can be described by 5 types of symmetry elements.

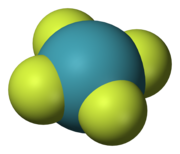
• Symmetry axis: an axis around which a rotation by results in a molecule indistinguishable from the original. This is also called an n-fold rotational axis and abbreviated C_n. Examples are the C₂ in water and the C₃ in ammonia. A molecule can have more than one symmetry axis; the one with the highest n is called the principal axis, and by convention is assigned the z-axis in a Cartesian coordinate system.
• Plane of symmetry: a plane of reflection through which an identical copy of the original molecule is given. This is also called a mirror plane and abbreviated σ. Water has two of them: one in the plane of the molecule itself and one perpendicular to it. A symmetry plane parallel with the principal axis is dubbed vertical (σ_v) and one perpendicular to it horizontal (σ_h). A third type of symmetry plane exists: If a vertical symmetry plane additionally bisects the angle between two 2-fold rotation axes perpendicular to the principal axis, the plane is dubbed dihedral (σ_d). A symmetry plane can also be identified by its Cartesian orientation, e.g., (xz) or (yz).
• Center of symmetry or inversion center, abbreviated i. A molecule has a center of symmetry when, for any atom in the molecule, an identical atom exists diametrically opposite this center an equal distance from it. There may or may not be an atom at the center. Examples are xenon tetrafluoride where the inversion center is at the Xe atom, and benzene (C₆H₆) where the inversion center is at the center of the ring.
• Rotation-reflection axis: an axis around which a rotation by , followed by a reflection in a plane perpendicular to it, leaves the molecule unchanged. Also called an n-fold improper rotation axis, it is abbreviated S_n. Examples are present in tetrahedral silicon tetrafluoride, with three S₄ axes, and the staggered conformation of ethane with one S₆ axis.
• Identity, abbreviated to E, from the German 'Einheit' meaning unity.^[6] This symmetry element simply consists of no change: every molecule has this element. While this element seems physically trivial, it must be included in the list of symmetry elements so that they form a mathematical group, whose definition requires inclusion of the identity element. It is so called because it is analogous to multiplying by one (unity).

Operations

XeF₄, with square planar geometry, has two C₄ rotations in opposite directions and a C₂ rotation.

The 5 symmetry elements have associated with them 5 types of symmetry operations, which leave the molecule in a state indistinguishable from the starting state. They are sometimes distinguished from symmetry elements by a caret or circumflex. Thus, Ĉ_n is the rotation of a molecule around an axis and Ê is the identity operation. A symmetry element can have more than one symmetry operation associated with it. For example, the C₄ axis of the square xenon tetrafluoride (XeF₄) molecule is associated with two Ĉ₄ rotations (90°) in opposite directions and a Ĉ₂ rotation (180°). Since Ĉ₁ is equivalent to Ê, Ŝ₁ to σ and Ŝ₂ to î, all symmetry operations can be classified as either proper or improper rotations.

Molecular symmetry groups

Groups

The symmetry operations of a molecule (or other object) form a group, which is a mathematical structure usually denoted in the form (G,*)) consisting of a set G and a binary combination operation say '*' satisfying certain properties listed below.

In a molecular symmetry group, the group elements are the symmetry operations (not the symmetry elements), and the binary combination consists of applying first one symmetry operation and then the other. An example is the sequence of a C₄ rotation about the z-axis and a reflection in the xy-plane, denoted σ(xy)C₄. By convention the order of operations is from right to left.

A molecular symmetry group obeys the defining properties of any group.

(1) closure property:
          For every pair of elements x and y in G, the product x*y is also in G.
          ( in symbols, for every two elements x, y∈G, x*y is also in G ).
This means that the group is closed so that combining two elements produces no new elements. Symmetry operations have this property because a sequence of two operations will produce a third state indistinguishable from the second and therefore from the first, so that the net effect on the molecule is still a symmetry operation.
(2) associative property:
          For every x and y and z in G, both (x*y)*z and x*(y*z) result with the same element in G.
          ( in symbols, (x*y)*z = x*(y*z ) for every x, y, and z ∈ G)
(3) existence of identity property:
          There must be an element ( say e ) in G such that product any element of G with e make no change to the element.
          ( in symbols, x*e=e*x= x for every x∈ G )
(4) existence of inverse property:
          For each element ( x ) in G, there must be an element y in G such that product of x and y is the identity element e.
          ( in symbols, for each x∈G there is a y ∈ G such that x*y=y*x= e for every x∈G )

The order of a group is the number of elements in the group. For groups of small orders, the group properties can be easily verified by considering its composition table, a table whose rows and columns correspond to elements of the group and whose entries correspond to their products.

Point group

The successive application (or composition) of one or more symmetry operations of a molecule has an effect equivalent to that of some single symmetry operation of the molecule. Moreover the set of all symmetry operations including this composition operation obeys all the properties of a group, given above. So (S,*) is a group where S is the set of all symmetry operations of some molecule, and * denotes the composition (repeated application) of symmetry operations. This group is called the point group of that molecule, because the set of symmetry operations leave at least one point fixed. For some symmetries, an entire axis or an entire plane are fixed.

The symmetry of a crystal, however, is described by a space group of symmetry operations, which includes translations in space.

Examples

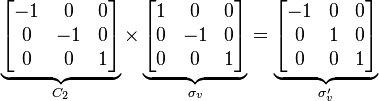
    (1)   The point group for the water molecule is C_2v, consisting of the symmetry operations E, C₂, σ_v and σ_v'. Its order is thus 4. Each operation is its own inverse. As an example of closure, a C₂ rotation followed by a σ_v reflection is seen to be a σ_v' symmetry operation: σ_v*C₂ = σ_v'. (Note that "Operation A followed by B to form C" is written BA = C).

    (2)   Another example is the ammonia molecule, which is pyramidal and contains a three-fold rotation axis as well as three mirror planes at an angle of 120° to each other. Each mirror plane contains an N-H bond and bisects the H-N-H bond angle opposite to that bond. Thus ammonia molecule belongs to the C_3v point group that has order 6: an identity element E, two rotation operations C₃ and C₃², and three mirror reflections σ_v, σ_v' and σ_v".

Common point groups

The following table contains a list of point groups with representative molecules. The description of structure includes common shapes of molecules based on VSEPR theory.

Point group	Symmetry operations	Simple description of typical geometry	Example 1	Example 2	Example 3
C1	E	no symmetry, chiral	bromochlorofluoromethane	lysergic acid
Cs	E σh	mirror plane, no other symmetry	thionyl chloride	hypochlorous acid	chloroiodomethane
Ci	E i	inversion center	(R,R) 1,2-dichloro-1,2-dibromoethane (anti conformer)
C∞v	E 2C∞ ∞σv	linear	Hydrogen fluoride	nitrous oxide (dinitrogen monoxide)
D∞h	E 2C∞ ∞σi i 2S∞ ∞C2	linear with inversion center	oxygen	carbon dioxide
C2	E C2	"open book geometry," chiral	hydrogen peroxide
C3	E C3	propeller, chiral	triphenylphosphine
C2h	E C2 i σh	planar with inversion center	trans-1,2-dichloroethylene
C3h	E C3 C32 σh S3 S35	propeller	boric acid
C2v	E C2 σv(xz) σv'(yz)	angular (H2O) or see-saw (SF4)	water	sulfur tetrafluoride	sulfuryl fluoride
C3v	E 2C3 3σv	trigonal pyramidal	ammonia	phosphorus oxychloride
C4v	E 2C4 C2 2σv 2σd	square pyramidal	xenon oxytetrafluoride
D2	E C2(x) C2(y) C2(z)	twist, chiral	cyclohexane twist conformation
D3	E C3(z) 3C2	triple helix, chiral	Tris(ethylenediamine)cobalt(III) cation
D2h	E C2(z) C2(y) C2(x) i σ(xy) σ(xz) σ(yz)	planar with inversion center	ethylene	dinitrogen tetroxide	diborane
D3h	E 2C3 3C2 σh 2S3 3σv	trigonal planar or trigonal bipyramidal	boron trifluoride	phosphorus pentachloride
D4h	E 2C4 C2 2C2' 2C2 i 2S4 σh 2σv 2σd	square planar	xenon tetrafluoride	octachlorodimolybdate(III) anion
D5h	E 2C5 2C52 5C2 σh 2S5 2S53 5σv	pentagonal	ruthenocene	C70
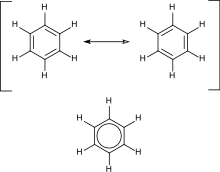
D6h	E 2C6 2C3 C2 3C2' 3C2‘’ i 2S3 2S6 σh 3σd 3σv	hexagonal	benzene	bis(benzene)chromium
D2d	E 2S4 C2 2C2' 2σd	90° twist	allene	tetrasulfur tetranitride
D3d	E C3 3C2 i 2S6 3σd	60° twist	ethane (staggered rotamer)	cyclohexane chair conformation
D4d	E 2S8 2C4 2S83 C2 4C2' 4σd	45° twist	dimanganese decacarbonyl (staggered rotamer)
D5d	E 2C5 2C52 5C2 i 3S103 2S10 5σd	36° twist	ferrocene (staggered rotamer)
Td	E 8C3 3C2 6S4 6σd	tetrahedral	methane	phosphorus pentoxide	adamantane
Oh	E 8C3 6C2 6C4 3C2 i 6S4 8S6 3σh 6σd	octahedral or cubic	cubane	sulfur hexafluoride
Ih	E 12C5 12C52 20C3 15C2 i 12S10 12S103 20S6 15σ	icosahedral or dodecahedral	Buckminsterfullerene	dodecaborate anion	dodecahedrane

Representations

The symmetry operations can be represented in many ways. A convenient representation is by matrices. For any vector representing a point in Cartesian coordinates, left-multiplying it gives the new location of the point transformed by the symmetry operation. Composition of operations corresponds to matrix multiplication. In the C_2v example this is:

Although an infinite number of such representations exist, the irreducible representations (or "irreps") of the group are commonly used, as all other representations of the group can be described as a linear combination of the irreducible representations.

Character tables

For each point group, a character table summarizes information on its symmetry operations and on its irreducible representations. As there are always equal numbers of irreducible representations and classes of symmetry operations, the tables are square.
The table itself consists of characters that represent how a particular irreducible representation transforms when a particular symmetry operation is applied. Any symmetry operation in a molecule's point group acting on the molecule itself will leave it unchanged. But, for acting on a general entity, such as a vector or an orbital, this need not be the case. The vector could change sign or direction, and the orbital could change type. For simple point groups, the values are either 1 or −1: 1 means that the sign or phase (of the vector or orbital) is unchanged by the symmetry operation (symmetric) and −1 denotes a sign change (asymmetric).

The representations are labeled according to a set of conventions:

• A, when rotation around the principal axis is symmetrical
• B, when rotation around the principal axis is asymmetrical
• E and T are doubly and triply degenerate representations, respectively
• when the point group has an inversion center, the subscript g (German: gerade or even) signals no change in sign, and the subscript u (ungerade or uneven) a change in sign, with respect to inversion.
• with point groups C_∞v and D_∞h the symbols are borrowed from angular momentum description: Σ, Π, Δ.

The tables also capture information about how the Cartesian basis vectors, rotations about them, and quadratic functions of them transform by the symmetry operations of the group, by noting which irreducible representation transforms in the same way. These indications are conventionally on the righthand side of the tables. This information is useful because chemically important orbitals (in particular p and d orbitals) have the same symmetries as these entities.

The character table for the C_2v symmetry point group is given below:

C2v	E	C2	σv(xz)	σv'(yz)
A1	1	1	1	1	z	x2, y2, z2
A2	1	1	−1	−1	Rz	xy
B1	1	−1	1	−1	x, Ry	xz
B2	1	−1	−1	1	y, Rx	yz

Consider the example of water (H₂O), which has the C_2v symmetry described above. The 2p_x orbital of oxygen is oriented perpendicular to the plane of the molecule and switches sign with a C₂ and a σ_v'(yz) operation, but remains unchanged with the other two operations (obviously, the character for the identity operation is always +1). This orbital's character set is thus {1, −1, 1, −1}, corresponding to the B₁ irreducible representation. Likewise, the 2p_z orbital is seen to have the symmetry of the A₁ irreducible representation, 2p_y B₂, and the 3d_xy orbital A₂. These assignments and others are noted in the rightmost two columns of the table.

Historical background

Hans Bethe used characters of point group operations in his study of ligand field theory in 1929, and Eugene Wigner used group theory to explain the selection rules of atomic spectroscopy.^[7] The first character tables were compiled by László Tisza (1933), in connection to vibrational spectra. Robert Mulliken was the first to publish character tables in English (1933), and E. Bright Wilson used them in 1934 to predict the symmetry of vibrational normal modes.^[8] The complete set of 32 crystallographic point groups was published in 1936 by Rosenthal and Murphy.^[9]

Nonrigid molecules

The symmetry groups described above are useful for describing rigid molecules which undergo only small oscillations about a single equilibrium geometry, so that the symmetry operations all correspond to simple geometrical operations. However Longuet-Higgins has proposed a more general type of symmetry groups suitable for non-rigid molecules with multiple equivalent geometries.^[10][11]
These groups are known as permutation-inversion groups, because a symmetry operation may be an energetically feasible permutation of equivalent nuclei, or an inversion with respect to the centre of mass, or a combination of the two.

For example, ethane (C₂H₆) has three equivalent staggered conformations. Conversion of one conformation to another occurs at ordinary temperature by internal rotation of one methyl group relative to the other. This is not a rotation of the entire molecule about the C₃ axis, but can be described as a permutation of the three identical hydrogens of one methyl group. Although each conformation has D_3d symmetry as in the table above, description of the internal rotation and associated quantum states and energy levels requires the more complete permutation-inversion group.

Similarly, ammonia (NH₃) has two equivalent pyramidal (C_3v) conformations which are interconverted by the process known as nitrogen inversion. This is not an inversion in the sense used for symmetry operations of rigid molecules, since NH₃ has no inversion center. Rather it is a reflection of all atoms about the centre of mass (close to the nitrogen), which happens to be energetically feasible for this molecule. Again the permutation-inversion group is used to describe the interaction of the two geometries.

A second and similar approach to the symmetry of nonrigid molecules is due to Altmann.^[12][13] In this approach the symmetry groups are known as Schrödinger supergroups and consist of two types of operations (and their combinations): (1) the geometric symmetry operations (rotations, reflections, inversions) of rigid molecules, and (2) isodynamic operations which take a nonrigid molecule into an energetically equivalent form by a physically reasonable process such as rotation about a single bond (as in ethane) or a molecular inversion (as in ammonia).^[13]

Organic chemistry

From Wikipedia, the free encyclopedia

Methane, CH₄, in line-angle representation, showing four carbon-hydrogen single (σ) bonds in black, and the 3D shape of such tetrahedral molecules, with ~109° interior bond angles, in green. Methane is the simplest organic chemical and simplest hydrocarbon, and molecules can be built up conceptually from it by exchanging up to all 4 hydrogens with carbon or other atoms.

Organic chemistry is a chemistry subdiscipline involving the scientific study of the structure, properties, and reactions of organic compounds and organic materials, i.e., matter in its various forms that contain carbon atoms.^[1][2] Study of structure includes using spectroscopy (e.g., NMR), mass spectrometry, and other physical and chemical methods to determine the chemical composition and constitution of organic compounds and materials. Study of properties includes both physical properties and chemical properties, and uses similar methods as well as methods to evaluate chemical reactivity, with the aim to understand the behavior of the organic matter in its pure form (when possible), but also in solutions, mixtures, and fabricated forms. The study of organic reactions includes probing their scope through use in preparation of target compounds (e.g., natural products, drugs, polymers, etc.) by chemical synthesis, as well as the focused study of the reactivities of individual organic molecules, both in the laboratory and via theoretical (in silico) study.

The range of chemicals studied in organic chemistry include hydrocarbons (compounds containing only carbon and hydrogen), as well as myriad compositions based always on carbon, but also containing other elements,^[1][3][4] especially:

• oxygen, nitrogen, sulfur, phosphorus (these, included in many organic chemicals in biology) and the radiostable elements of the halogens.

In the modern era, the range extends further into the periodic table, with main group elements, including:

• Group 1 and 2 organometallic compounds, i.e., involving alkali (e.g., lithium, sodium, and potassium) or alkaline earth metals (e.g., magnesium), or
• metalloids (e.g., boron and silicon) or other metals (e.g., aluminium and tin).

In addition, much modern research focuses on organic chemistry involving further organometallics, including the lanthanides, but especially the:

• transition metals (e.g., zinc, copper, palladium, nickel, cobalt, titanium, chromium, etc.).

Line-angle representation

Ball-and-stick representation

Space-filling representation

Three representations of an organic compound, 5α-Dihydroprogesterone (5α-DHP), a steroid hormone. For molecules showing color, the carbon atoms are in black, hydrogens in gray, and oxygens in red. In the line angle representation, carbon atoms are implied at every terminus of a line and vertex of multiple lines, and hydrogen atoms are implied to fill the remaining needed valences (up to 4).

Finally, organic compounds form the basis of all earthly life and constitute a significant part of human endeavors in chemistry. The bonding patterns open to carbon, with its valence of four—formal single, double, and triple bonds, as well as various structures with delocalized electrons—make the array of organic compounds structurally diverse, and their range of applications enormous. They either form the basis of, or are important constituents of, many commercial products including pharmaceuticals; petrochemicals and products made from them (including lubricants, solvents, etc.); plastics; fuels and explosives; etc. As indicated, the study of organic chemistry overlaps with organometallic chemistry and biochemistry, but also with medicinal chemistry, polymer chemistry, as well as many aspects of materials science.^[1]

Periodic table of elements of interest in organic chemistry. The table illustrates all elements of current interest in modern organic and organometallic chemistry, indicating main group elements in orange, and transition metals and lanthanides (Lan) in grey.

History

Main article: History of chemistry

Friedrich Wöhler

Before the nineteenth century, chemists generally believed that compounds obtained from living organisms were endowed with a vital force that distinguished them from inorganic compounds. According to the concept of vitalism (vital force theory), organic matter was endowed with a "vital force".^[5] During the first half of the nineteenth century, some of the first systematic studies of organic compounds were reported. Around 1816 Michel Chevreul started a study of soaps made from various fats and alkalis. He separated the different acids that, in combination with the alkali, produced the soap. Since these were all individual compounds, he demonstrated that it was possible to make a chemical change in various fats (which traditionally come from organic sources), producing new compounds, without "vital force". In 1828 Friedrich Wöhler produced the organic chemical urea (carbamide), a constituent of urine, from the inorganic ammonium cyanate NH₄CNO, in what is now called the Wöhler synthesis. Although Wöhler was always cautious about claiming that he had disproved the theory of vital force, this event has often been thought of as a turning point.^[5]

In 1856 William Henry Perkin, while trying to manufacture quinine, accidentally manufactured the organic dye now known as Perkin's mauve. Through its great financial success, this discovery greatly increased interest in organic chemistry.^[6]

The crucial breakthrough for organic chemistry was the concept of chemical structure, developed independently and simultaneously by Friedrich August Kekulé and Archibald Scott Couper in 1858.^[7] Both men suggested that tetravalent carbon atoms could link to each other to form a carbon lattice, and that the detailed patterns of atomic bonding could be discerned by skillful interpretations of appropriate chemical reactions.

The pharmaceutical industry began in the last decade of the 19th century when the manufacturing of acetylsalicylic acid (more commonly referred to as aspirin) in Germany was started by Bayer.^[8] The first time a drug was systematically improved was with arsphenamine (Salvarsan). Though numerous derivatives of the dangerous toxic atoxyl were examined by Paul Ehrlich and his group, the compound with best effectiveness and toxicity characteristics was selected for production.^{[citation needed]}

An example of an organometallic molecule, a catalyst called Grubbs' catalyst, as a ball-and-stick model based on an X-ray crystal structure.^[9] The formula of the catalyst is often given as RuCl₂(PCy₃)₂(=CHPh), where the ruthenium metal atom, Ru, is at very center in turquoise, carbons are in black, hydrogens in gray-white, chlorine in green, and phosphorus in orange. The metal ligand at the bottom is a tricyclohexyl phosphine, abbreviated PCy, and another of these appears at the top of the image (where its rings are obscuring one another). The group projecting out to the right has a metal-carbon double bond, as is known as an alkylidene. Robert Grubbs shared the 2005 Nobel prize in chemistry with Richard R. Schrock and Yves Chauvin for their work on the reactions such catalysts mediate, called olefin metathesis.

Early examples of organic reactions and applications were often serendipitous. The latter half of the 19th century however witnessed systematic studies of organic compounds, Illustrative is the development of synthetic indigo. The production of indigo from plant sources dropped from 19,000 tons in 1897 to 1,000 tons by 1914 thanks to the synthetic methods developed by Adolf von Baeyer. In 2002, 17,000 tons of synthetic indigo were produced from petrochemicals.^[10]

In the early part of the 20th Century, polymers and enzymes were shown to be large organic molecules, and petroleum was shown to be of biological origin.

The multistep synthesis of complex organic compounds is called total synthesis. Total synthesis of complex natural compounds increased in complexity to glucose and terpineol. For example, cholesterol-related compounds have opened ways to synthesize complex human hormones and their modified derivatives. Since the start of the 20th century, complexity of total syntheses has been increased to include molecules of high complexity such as lysergic acid and vitamin B12.^[11]

The total synthesis of vitamin B12 marked a major achievement in organic chemistry.

The development of organic chemistry benefited from the discovery of petroleum and the development of the petrochemical industry. The conversion of individual compounds obtained from petroleum into different compound types by various chemical processes led to the birth of the petrochemical industry, which successfully manufactured artificial rubbers, various organic adhesives, property-modifying petroleum additives, and plastics.

The majority of chemical compounds occurring in biological organisms are in fact carbon compounds, so the association between organic chemistry and biochemistry is so close that biochemistry might be regarded as in essence a branch of organic chemistry. Although the history of biochemistry might be taken to span some four centuries, fundamental understanding of the field only began to develop in the late 19th century and the actual term biochemistry was coined around the start of 20th century. Research in the field increased throughout the twentieth century, without any indication of slackening in the rate of increase, as may be verified by inspection of abstraction and indexing services such as BIOSIS Previews and Biological Abstracts, which began in the 1920s as a single annual volume, but has grown so drastically that by the end of the 20th century it was only available to the everyday user as an online electronic database.^[12]

Characterization

Since organic compounds often exist as mixtures, a variety of techniques have also been developed to assess purity, especially important being chromatography techniques such as HPLC and gas chromatography. Traditional methods of separation include distillation, crystallization, and solvent extraction.

Organic compounds were traditionally characterized by a variety of chemical tests, called "wet methods", but such tests have been largely displaced by spectroscopic or other computer-intensive methods of analysis.^[13] Listed in approximate order of utility, the chief analytical methods are:

• Nuclear magnetic resonance (NMR) spectroscopy is the most commonly used technique, often permitting complete assignment of atom connectivity and even stereochemistry using correlation spectroscopy. The principal constituent atoms of organic chemistry - hydrogen and carbon - exist naturally with NMR-responsive isotopes, respectively ¹H and ¹³C.
• Elemental analysis: A destructive method used to determine the elemental composition of a molecule. See also mass spectrometry, below.
• Mass spectrometry indicates the molecular weight of a compound and, from the fragmentation patterns, its structure. High resolution mass spectrometry can usually identify the exact formula of a compound and is used in lieu of elemental analysis. In former times, mass spectrometry was restricted to neutral molecules exhibiting some volatility, but advanced ionization techniques allow one to obtain the "mass spec" of virtually any organic compound.
• Crystallography is an unambiguous method for determining molecular geometry, the proviso being that single crystals of the material must be available and the crystal must be representative of the sample. Highly automated software allows a structure to be determined within hours of obtaining a suitable crystal.

Traditional spectroscopic methods such as infrared spectroscopy, optical rotation, UV/VIS spectroscopy provide relatively nonspecific structural information but remain in use for specific classes of compounds.

Properties

Physical properties of organic compounds typically of interest include both quantitative and
qualitative features. Quantitative information includes melting point, boiling point, and index of refraction. Qualitative properties include odor, consistency, solubility, and color.

Melting and boiling properties

Organic compounds typically melt and many boil. In contrast, while inorganic materials generally can be melted, many do not boil, tending instead to degrade. In earlier times, the melting point (m.p.) and boiling point (b.p.) provided crucial information on the purity and identity of organic compounds. The melting and boiling points correlate with the polarity of the molecules and their molecular weight. Some organic compounds, especially symmetrical ones, sublime, that is they evaporate without melting. A well-known example of a sublimable organic compound is para-dichlorobenzene, the odiferous constituent of modern mothballs. Organic compounds are usually not very stable at temperatures above 300 °C, although some exceptions exist.

Solubility

Neutral organic compounds tend to be hydrophobic; that is, they are less soluble in water than in organic solvents. Exceptions include organic compounds that contain ionizable(which can be converted in ions) groups as well as low molecular weight alcohols, amines, and carboxylic acids where hydrogen bonding occurs. Organic compounds tend to dissolve in organic solvents. Solvents can be either pure substances like ether or ethyl alcohol, or mixtures, such as the paraffinic solvents such as the various petroleum ethers and white spirits, or the range of pure or mixed aromatic solvents obtained from petroleum or tar fractions by physical separation or by chemical conversion.
Solubility in the different solvents depends upon the solvent type and on the functional groups if present.

Solid state properties

Various specialized properties of molecular crystals and organic polymers with conjugated systems are of interest depending on applications, e.g. thermo-mechanical and electro-mechanical such as piezoelectricity, electrical conductivity (see conductive polymers and organic semiconductors), and electro-optical (e.g. non-linear optics) properties. For historical reasons, such properties are mainly the subjects of the areas of polymer science and materials science.

Nomenclature

Various names and depictions for one organic compound.

The names of organic compounds are either systematic, following logically from a set of rules, or nonsystematic, following various traditions. Systematic nomenclature is stipulated by specifications from IUPAC. Systematic nomenclature starts with the name for a parent structure within the molecule of interest. This parent name is then modified by prefixes, suffixes, and numbers to unambiguously convey the structure. Given that millions of organic compounds are known, rigorous use of systematic names can be cumbersome. Thus, IUPAC recommendations are more closely followed for simple compounds, but not complex molecules. To use the systematic naming, one must know the structures and names of the parent structures. Parent structures include unsubstituted hydrocarbons, heterocycles, and monofunctionalized derivatives thereof.

Nonsystematic nomenclature is simpler and unambiguous, at least to organic chemists. Nonsystematic names do not indicate the structure of the compound. They are common for complex molecules, which includes most natural products. Thus, the informally named lysergic acid diethylamide is systematically named (6aR,9R)-N,N-diethyl-7-methyl-4,6,6a,7,8,9-hexahydroindolo-[4,3-fg] quinoline-9-carboxamide.

With the increased use of computing, other naming methods have evolved that are intended to be interpreted by machines. Two popular formats are SMILES and InChI.

Structural drawings

Organic molecules are described more commonly by drawings or structural formulas, combinations of drawings and chemical symbols. The line-angle formula is simple and unambiguous. In this system, the endpoints and intersections of each line represent one carbon, and hydrogen atoms can either be notated explicitly or assumed to be present as implied by tetravalent carbon. The depiction of organic compounds with drawings is greatly simplified by the fact that carbon in almost all organic compounds has four bonds, nitrogen three, oxygen two, and hydrogen one.

Classification of organic compounds

Functional groups

The family of carboxylic acids contains a carboxyl (-COOH) functional group. Acetic acid, shown here, is an example.

The concept of functional groups is central in organic chemistry, both as a means to classify structures and for predicting properties. A functional group is a molecular module, and the reactivity of that functional group is assumed, within limits, to be the same in a variety of molecules. Functional groups can have decisive influence on the chemical and physical properties of organic compounds. Molecules are classified on the basis of their functional groups. Alcohols, for example, all have the subunit C-O-H. All alcohols tend to be somewhat hydrophilic, usually form esters, and usually can be converted to the corresponding halides. Most functional groups feature heteroatoms (atoms other than C and H). Organic compounds are classified according to functional groups, alcohols, carboxylic acids, amines, etc.

Aliphatic compounds

The aliphatic hydrocarbons are subdivided into three groups of homologous series according to their state of saturation:

• paraffins, which are alkanes without any double or triple bonds,
• olefins or alkenes which contain one or more double bonds, i.e. di-olefins (dienes) or poly-olefins.
• alkynes, which have one or more triple bonds.

The rest of the group is classed according to the functional groups present. Such compounds can be "straight-chain", branched-chain or cyclic. The degree of branching affects characteristics, such as the octane number or cetane number in petroleum chemistry.

Both saturated (alicyclic) compounds and unsaturated compounds exist as cyclic derivatives. The most stable rings contain five or six carbon atoms, but large rings (macrocycles) and smaller rings are common. The smallest cycloalkane family is the three-membered cyclopropane ((CH₂)₃). Saturated cyclic compounds contain single bonds only, whereas aromatic rings have an alternating (or conjugated) double bond. Cycloalkanes do not contain multiple bonds, whereas the cycloalkenes and the cycloalkynes do.

Aromatic compounds

Benzene is one of the best-known aromatic compounds as it is one of the simplest and most stable aromatics.

Aromatic hydrocarbons contain conjugated double bonds. This means that every carbon atom in the ring is sp2 hybridized, allowing for added stability. The most important example is benzene, the structure of which was formulated by Kekulé who first proposed the delocalization or resonance principle for explaining its structure. For "conventional" cyclic compounds, aromaticity is conferred by the presence of 4n + 2 delocalized pi electrons, where n is an integer. Particular instability (antiaromaticity) is conferred by the presence of 4n conjugated pi electrons.

Heterocyclic compounds

The characteristics of the cyclic hydrocarbons are again altered if heteroatoms are present, which can exist as either substituents attached externally to the ring (exocyclic) or as a member of the ring itself (endocyclic). In the case of the latter, the ring is termed a heterocycle. Pyridine and furan are examples of aromatic heterocycles while piperidine and tetrahydrofuran are the corresponding alicyclic heterocycles. The heteroatom of heterocyclic molecules is generally oxygen, sulfur, or nitrogen, with the latter being particularly common in biochemical systems.
Examples of groups among the heterocyclics are the aniline dyes, the great majority of the compounds discussed in biochemistry such as alkaloids, many compounds related to vitamins, steroids, nucleic acids (e.g. DNA, RNA) and also numerous medicines. Heterocyclics with relatively simple structures are pyrrole (5-membered) and indole (6-membered carbon ring).

Rings can fuse with other rings on an edge to give polycyclic compounds. The purine nucleoside bases are notable polycyclic aromatic heterocycles. Rings can also fuse on a "corner" such that one atom (almost always carbon) has two bonds going to one ring and two to another. Such compounds are termed spiro and are important in a number of natural products.

Polymers

This swimming board is made of polystyrene, an example of a polymer.

One important property of carbon is that it readily forms chains, or networks, that are linked by carbon-carbon (carbon to carbon) bonds. The linking process is called polymerization, while the chains, or networks, are called polymers. The source compound is called a monomer.

Two main groups of polymers exist: synthetic polymers and biopolymers. Synthetic polymers are artificially manufactured, and are commonly referred to as industrial polymers.^[14] Biopolymers occur within a respectfully natural environment, or without human intervention.

Since the invention of the first synthetic polymer product, bakelite, synthetic polymer products have frequently been invented.^{[citation needed]}

Common synthetic organic polymers are polyethylene (polythene), polypropylene, nylon, teflon (PTFE), polystyrene, polyesters, polymethylmethacrylate (called perspex and plexiglas), and polyvinylchloride (PVC).^{[citation needed]}

Both synthetic and natural rubber are polymers.^{[citation needed]}

Varieties of each synthetic polymer product may exist, for purposes of a specific use. Changing the conditions of polymerization alters the chemical composition of the product and its properties. These alterations include the chain length, or branching, or the tacticity.^{[citation needed]}

With a single monomer as a start, the product is a homopolymer.^{[citation needed]}

Secondary component(s) may be added to create a heteropolymer (co-polymer) and the degree of clustering of the different components can also be controlled.^{[citation needed]}

Physical characteristics, such as hardness, density, mechanical or tensile strength, abrasion resistance, heat resistance, transparency, colour, etc. will depend on the final composition.^{[citation needed]}

Biomolecules

Maitotoxin, a complex organic biological toxin.

Biomolecular chemistry is a major category within organic chemistry which is frequently studied by biochemists. Many complex multi-functional group molecules are important in living organisms. Some are long-chain biopolymers, and these include peptides, DNA, RNA and the polysaccharides such as starches in animals and celluloses in plants. The other main classes are amino acids (monomer building blocks of peptides and proteins), carbohydrates (which includes the polysaccharides), the nucleic acids (which include DNA and RNA as polymers), and the lipids. In addition, animal biochemistry contains many small molecule intermediates which assist in energy production through the Krebs cycle, and produces isoprene, the most common hydrocarbon in animals. Isoprenes in animals form the important steroid structural (cholesterol) and steroid hormone compounds; and in plants form terpenes, terpenoids, some alkaloids, and a class of hydrocarbons called biopolymer polyisoprenoids present in the latex of various species of plants, which is the basis for making rubber.

Small molecules

Molecular models of caffeine.

In pharmacology, an important group of organic compounds is small molecules, also referred to as 'small organic compounds'. In this context, a small molecule is a small organic compound that is biologically active, but is not a polymer. In practice, small molecules have a molar mass less than approximately 1000 g/mol.

Fullerenes

Fullerenes and carbon nanotubes, carbon compounds with spheroidal and tubular structures, have stimulated much research into the related field of materials science. The first fullerene was discovered in 1985 by Sir Harold W. Kroto (one of the authors of this article) of the United Kingdom and by Richard E. Smalley and Robert F. Curl, Jr., of the United States. Using a laser to vaporize graphite rods in an atmosphere of helium gas, these chemists and their assistants obtained cagelike molecules composed of 60 carbon atoms (C60) joined together by single and double bonds to form a hollow sphere with 12 pentagonal and 20 hexagonal faces—a design that resembles a football, or soccer ball. In 1996 the trio was awarded the Nobel Prize for their pioneering efforts. The C60 molecule was named buckminsterfullerene (or, more simply, the buckyball) after the American architect R. Buckminster Fuller, whose geodesic dome is constructed on the same structural principles. The elongated cousins of buckyballs, carbon nanotubes, were identified in 1991 by Iijima Sumio of Japan.

Others

Organic compounds containing bonds of carbon to nitrogen, oxygen and the halogens are not normally grouped separately. Others are sometimes put into major groups within organic chemistry and discussed under titles such as organosulfur chemistry, organometallic chemistry, organophosphorus chemistry and organosilicon chemistry.

Organic synthesis

A synthesis designed by E.J. Corey for oseltamivir (Tamiflu). This synthesis has 11 distinct reactions.

Synthetic organic chemistry is an applied science as it borders engineering, the "design, analysis, and/or construction of works for practical purposes". Organic synthesis of a novel compound is a problem solving task, where a synthesis is designed for a target molecule by selecting optimal reactions from optimal starting materials. Complex compounds can have tens of reaction steps that sequentially build the desired molecule. The synthesis proceeds by utilizing the reactivity of the functional groups in the molecule. For example, a carbonyl compound can be used as a nucleophile by converting it into an enolate, or as an electrophile; the combination of the two is called the aldol reaction. Designing practically useful syntheses always requires conducting the actual synthesis in the laboratory. The scientific practice of creating novel synthetic routes for complex molecules is called total synthesis.

Strategies to design a synthesis include retrosynthesis, popularized by E.J. Corey, starts with the target molecule and splices it to pieces according to known reactions. The pieces, or the proposed precursors, receive the same treatment, until available and ideally inexpensive starting materials are reached. Then, the retrosynthesis is written in the opposite direction to give the synthesis. A "synthetic tree" can be constructed, because each compound and also each precursor has multiple syntheses.

Organic reactions

Organic reactions are chemical reactions involving organic compounds. Many of these reactions are associated with functional groups. The general theory of these reactions involves careful analysis of such properties as the electron affinity of key atoms, bond strengths and steric hindrance. These factors can determine the relative stability of short-lived reactive intermediates, which usually directly determine the path of the reaction.

The basic reaction types are: addition reactions, elimination reactions, substitution reactions, pericyclic reactions, rearrangement reactions and redox reactions. An example of a common reaction is a substitution reaction written as:

Nu⁻ + C-X → C-Nu + X⁻

where X is some functional group and Nu is a nucleophile.

The number of possible organic reactions is basically infinite. However, certain general patterns are observed that can be used to describe many common or useful reactions. Each reaction has a stepwise reaction mechanism that explains how it happens in sequence—although the detailed description of steps is not always clear from a list of reactants alone.

The stepwise course of any given reaction mechanism can be represented using arrow pushing techniques in which curved arrows are used to track the movement of electrons as starting materials transition through intermediates to final products.

AAAS Scientists: Consensus on GMO Safety Firmer Than For Human-Induced Climate Change

Posted: 01/29/2015 3:10 pm EST Updated: 01/30/2015 3:59 pm EST

Original link:  http://www.huffingtonpost.com/jon-entine/post_8915_b_6572130.html

 

In sharp contrast to public views about GMOs, 89% of scientists believe genetically modified foods are safe.

That's the most eye-opening finding in a Pew Research Center study on science literacy, undertaken in cooperation with the American Association for the Advancement of Science (AAAS), and released on January 29.

The overwhelming scientific consensus exceeds the percentage of scientists, 88%, who think humans are mostly responsible for climate change. However, the public appears far more suspicious of scientific claims about GMO safety than they do about the consensus on global warming.

Some 57% of Americans say GM foods are unsafe and a startling 67% do not trust scientists, believing they don't understand the science behind GMOs. AAAS researchers blame poor reporting by mainstream scientists for the trust and literacy gaps.

The survey also contrasts sharply with a statement published earlier this week in a pay-for-play European journal by a group of anti-GMO scientists and activists, including Michael Hansen of the Center for Food Safety, and philosopher Vandana Shiva, claiming, "no scientific consensus on GMO safety."

A huge literacy gap between scientists and the public on biotechnology is one of the many disturbing nuggets that emerged from the Pew Research Center survey, which was conducted in cooperation with the AAAS, the world's largest independent general scientific society. The full study, released on January 29, is available here.

The first of several reports to be released in coming months, this study compares the views of scientists and the general public on the role of science in the United States and globally.

The eye opening take-away: The American population in general borders on scientific illiteracy. The gap between what scientists believe, grounded on empirical evidence, often sharply differs from what the general public thinks is true. The differences are sharpest over biomedical research, including GMOs.

• 88% of AAAS scientists think eating GM food is safe, while only 37% of the public believes that’s true--a 51-percentage point gap
• 68% of scientists say it is safe to eat food grown with pesticides, compared with 28% of citizens--a 40% gap.
• A 42-percentage point gap over the issue of using animals in research--89% of scientists favor it, while only 47% of the public backs the idea.

The scientist/public perception gap is less pronounced over climate, energy and space issues.

• 37-percentage point gap over whether humans are the primary cause of climate change--87% of AAAS scientists say it is, while 50% of the public does.
• 33-percentage point gap on the question about whether humans have evolved over time--98% of scientists say we have, compared with 65% of the public.
• By a 20-percentage point margin, citizens are more likely than scientists to favor offshore oil drilling.
• By a 12-point margin, the public is more likely to say that astronauts are essential for the future of the U.S. space program.

The survey represents a sample of 2,002 adult citizens and 3,748 scientists who are all members of the AAAS.

"As scientists size up the culture and their place in it," Pew said in a statement. "Scientists are notably less upbeat than they were five years ago and express serious concerns about public knowledge of science and the way scientific findings are covered by journalists."

The scientists believe that media hype is one possible reason for large gaps in opinion between their views and that of the public, particularly in the debate over GMOs. Seventy-nine percent of scientists said that the media doesn't distinguish between "well-founded" and "not well-founded" scientific research. Additionally, 52 percent agreed that the media oversimplifies the science.

Three years ago, the AAAS released an unequivocal statement on the safety of GM foods and why a consensus of its members oppose mandatory labelling:

There are several current efforts to require labeling of foods containing products derived from genetically modified crop plants, commonly known as GM crops or GMOs. These efforts are not driven by evidence that GM foods are actually dangerous. Indeed, the science is quite clear: crop improvement by the modern molecular techniques of biotechnology is safe. Rather, these initiatives are driven by a variety of factors, ranging from the persistent perception that such foods are somehow "unnatural" and potentially dangerous to the desire to gain competitive advantage by legislating attachment of a label meant to alarm. Another misconception used as a rationale for labeling is that GM crops are untested.

The AAAS also has addressed claims by anti-GMO advocacy groups, frequently echoed in the media and on activist websites, that GM foods are less tested or nutritionally deficient when compared to organic or other conventional foods.

... contrary to popular misconceptions, GM crops are the most extensively tested crops ever added to our food supply. There are occasional claims that feeding GM foods to animals causes aberrations ranging from digestive disorders, to sterility, tumors and premature death. Although such claims are often sensationalized and receive a great deal of media attention, none have stood up to rigorous scientific scrutiny. Indeed, a recent review of a dozen well-designed long-term animal feeding studies comparing GM and non-GM potatoes, soy, rice, corn and triticale found that the GM and their non-GM counterparts are nutritionally equivalent.

Looking further at the demographics of respondents, the survey finds that those with a college degree are split on GMO safety: 49% say it's generally safe while 47% say it's generally unsafe. Women are more wary than men: only 28% of women think eating GM foods are safe compared to 47% of men.
Race also divides the issue with blacks (24% say its safe) and Hispanics (32%) being more cautious than whites (41%).

The demographics of respondents on pesticide are quite similar to the responses on GMOs. More men say foods with pesticides are safe than women do. Those with more education are more likely to say food grown with pesticides are safe.

When it comes to GM labeling, exactly half of respondents said they "always" or "sometimes" check for a non-GMO label when they are shopping. Of course, those who check labels correlate higher with those who think genetically modified foods are unsafe to eat.

So why are citizens so out of step with scientists on GMO safety?

"One possible reason for the gap: when it comes to GM crops, two-thirds of the public say scientists do not have a clear understanding about the health effects," surmised the researchers.

Yet, oddly enough for a society that doesn't trust scientists on the GMO debate, science itself still holds an esteemed position in the minds of adults. Seventy-nine percent of respondents believe that science has contributed positively to society with 62% saying it has been beneficial for the quality of food. However, the percent of people who believe that science has contributed negatively to food is up 10 points from 2009: 34 percent of respondents say that science has had a negative affect on food.

The public also highly values government investment in science research: 71% support government-funded basic science research and 61% said government funding is essential for scientific progress.

Pew also asked scientists another question: How good is the general state of science is today? Scientists were more negative this year than they were in 2009. Only 52% say that it is a good time for science today while 74% said it was good in 2009.
And due to the public perception of GMOs at least, scientists' more sober assessment might make sense.

Who's to blame? Scientists (75%) say lack of STEM education in grades K-12 is the biggest culprit. The release of the next report is expected in mid-February.

How can scientists and the government bridge the disturbing literacy gap between the mainstream scientific community and a skeptical public? asks Alan Leshner, CEO of the AAAS, in an editorial accompanying the survey release?

Speaking up for the importance of science to society is our only hope, and scientists must not shy away from engaging with the public, even on the most polarizing science-based topics. Scientists need to speak clearly with journalists who provide a great vehicle for translating the nature and implications of their work. Scientists should also meet with members of the public and discuss what makes each side uncomfortable. In these situations, scientists must respond forthrightly to public concerns. In other words, there needs to be a conversation, not a lecture. The public's perceptions of scientists' expertise and trustworthiness are very important but they are not enough. Acceptance of scientific facts is not based solely on comprehension levels. It can be compromised anytime information confronts people's personal, religious, or political views, and whenever scientific facts provoke fear or make people feel that they have no control over a situation. The only recourse is to have genuine, respectful dialogues with people.

Jon Entine, executive director of the Genetic Literacy Project, is a Senior Fellow at the World Food Center Institute for Food and Agricultural Literacy, University of California-Davis. Follow @JonEntine on Twitter.