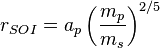From Wikipedia, the free encyclopedia
Orbital mechanics or astrodynamics is the application of ballistics and celestial mechanics to the practical problems concerning the motion of rockets and other spacecraft. The motion of these objects is usually calculated from Newton's laws of motion and Newton's law of universal gravitation. It is a core discipline within space mission design and control. Celestial mechanics treats more broadly the orbital dynamics of systems under the influence of gravity, including both spacecraft and natural astronomical bodies such as star systems, planets, moons, and comets. Orbital mechanics focuses on spacecraft trajectories, including orbital maneuvers, orbit plane changes, and interplanetary transfers, and is used by mission planners to predict the results of propulsive maneuvers. General relativity is a more exact theory than Newton's laws for calculating orbits, and is sometimes necessary for greater accuracy or in high-gravity situations (such as orbits close to the Sun).
History
Until the rise of space travel in the twentieth century, there was little distinction between orbital and celestial mechanics, and at the time of Sputnik, the field was called Space Dynamics (ref. the 1961 book by William Thompson of that name). The fundamental techniques, such as those used to solve the Keplerian problem (determining position as a function of time), are therefore the same in both fields. Furthermore, the history of the fields is almost entirely shared.Johannes Kepler was the first to successfully model planetary orbits to a high degree of accuracy, publishing his laws in 1605. Isaac Newton published more general laws of celestial motion in his 1687 book, Philosophiæ Naturalis Principia Mathematica.
Practical techniques
Rules of thumb
The following rules of thumb are useful for situations approximated by classical mechanics under the standard assumptions of astrodynamics. The specific example discussed is of a satellite orbiting a planet, but the rules of thumb could also apply to other situations, such as orbits of small bodies around a star such as the Sun.- Kepler's laws of planetary motion, which can be mathematically derived from Newton's laws, hold strictly only in describing the motion of two gravitating bodies, in the absence of non-gravitational forces, or approximately when the gravity of a single massive body like the Sun dominates other effects:
- Orbits are elliptical, with the heavier body at one focus of the ellipse. Special cases of this are circular orbits (a circle being simply an ellipse of zero eccentricity) with the planet at the center, and parabolic orbits (which are ellipses with eccentricity of exactly 1, which is simply an infinitely long ellipse) with the planet at the focus.
- A line drawn from the planet to the satellite sweeps out equal areas in equal times no matter which portion of the orbit is measured.
- The square of a satellite's orbital period is proportional to the cube of its average distance from the planet.
- Without applying thrust (such as firing a rocket engine), the height and shape of the satellite's orbit won't change.
- A satellite in a low orbit (or low part of an elliptical orbit) moves more quickly with respect to the surface of the planet than a satellite in a higher orbit (or a high part of an elliptical orbit), due to the stronger gravitational attraction closer to the planet.
- If thrust is applied at only one point in the satellite's orbit, it will return to that same point on each subsequent orbit, though the rest of its path will change. Thus to move from one circular orbit to another, at least two brief applications of thrust are needed.
- From a circular orbit, thrust applied in a direction opposite to the satellite's motion creates an elliptical orbit with a lower periapse (lowest orbital point) at 180 degrees away from the firing point. Thrust applied in the direction of the satellite's motion creates an elliptical orbit with a higher apoapse 180 degrees away from the firing point.
To the degree that the standard assumptions of astrodynamics do not hold, actual trajectories will vary from those calculated. For example, simple atmospheric drag is another complicating factor for objects in Earth orbit. These rules of thumb are decidedly inaccurate when describing two or more bodies of similar mass, such as a binary star system (see n-body problem). (Celestial mechanics uses more general rules applicable to a wider variety of situations.) The differences between classical mechanics and general relativity can also become important near large objects like stars.
Laws of astrodynamics
The fundamental laws of astrodynamics are Newton's law of universal gravitation and Newton's laws of motion, while the fundamental mathematical tool is his differential calculus.Every orbit and trajectory outside atmospheres is in principle reversible, i.e., in the space-time function the time is reversed. The velocities are reversed and the accelerations are the same, including those due to rocket bursts. Thus if a rocket burst is in the direction of the velocity, in the reversed case it is opposite to the velocity. Of course in the case of rocket bursts there is no full reversal of events, both ways the same delta-v is used and the same mass ratio applies.
Standard assumptions in astrodynamics include non-interference from outside bodies, negligible mass for one of the bodies, and negligible other forces (such as from the solar wind, atmospheric drag, etc.). More accurate calculations can be made without these simplifying assumptions, but they are more complicated. The increased accuracy often does not make enough of a difference in the calculation to be worthwhile.
Kepler's laws of planetary motion may be derived from Newton's laws, when it is assumed that the orbiting body is subject only to the gravitational force of the central attractor. When an engine thrust or propulsive force is present, Newton's laws still apply, but Kepler's laws are invalidated. When the thrust stops, the resulting orbit will be different but will once again be described by Kepler's laws. The three laws are:
- The orbit of every planet is an ellipse with the sun at one of the foci.
- A line joining a planet and the sun sweeps out equal areas during equal intervals of time.
- The squares of the orbital periods of planets are directly proportional to the cubes of the semi-major axis of the orbits.
Escape velocity
The formula for escape velocity is easily derived as follows. The specific energy (energy per unit mass) of any space vehicle is composed of two components, the specific potential energy and the specific kinetic energy. The specific potential energy associated with a planet of mass M is given by


 , from the center of the central body to the space vehicle in question. Therefore, the object can reach infinite
, from the center of the central body to the space vehicle in question. Therefore, the object can reach infinite  only if this quantity is nonnegative, which implies
only if this quantity is nonnegative, which implies
Formulae for free orbits
Orbits are conic sections, so, naturally, the formula for the distance of a body for a given angle corresponds to the formula for that curve in polar coordinates, which is:Circular orbits
All bounded orbits where the gravity of a central body dominates are elliptical in nature. A special case of this is the circular orbit, which is an ellipse of zero eccentricity. The formula for the velocity of a body in a circular orbit at distance r from the center of gravity of mass M is is the gravitational constant, equal to
is the gravitational constant, equal to- 6.673 84 × 10−11 m3/(kg·s2)
The quantity GM is often termed the standard gravitational parameter, which has a different value for every planet or moon in the Solar System.
Once the circular orbital velocity is known, the escape velocity is easily found by multiplying by the square root of 2:
Elliptical orbits
If 0 < e > 1, then the denominator of the equation of free orbits varies with the true anomaly θ, but remains positive, never becoming zero. Therefore, the relative position vector remains bounded, having its smallest magnitude at periapsis rp, which is given by:Orbital period
Under standard assumptions the orbital period (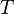 ) of a body traveling along an elliptic orbit can be computed as:
) of a body traveling along an elliptic orbit can be computed as: is standard gravitational parameter,
is standard gravitational parameter, is length of semi-major axis.
is length of semi-major axis.
- The orbital period is equal to that for a circular orbit with the orbit radius equal to the semi-major axis (
 ),
), - For a given semi-major axis the orbital period does not depend on the eccentricity (See also: Kepler's third law).
Velocity
Under standard assumptions the orbital speed ( ) of a body traveling along an elliptic orbit can be computed from the Vis-viva equation as:
) of a body traveling along an elliptic orbit can be computed from the Vis-viva equation as: is the standard gravitational parameter,
is the standard gravitational parameter, is the distance between the orbiting bodies.
is the distance between the orbiting bodies. is the length of the semi-major axis.
is the length of the semi-major axis.
 , or it is the same with the convention that in that case a is negative.
, or it is the same with the convention that in that case a is negative.Energy
Under standard assumptions, specific orbital energy ( ) of elliptic orbit is negative and the orbital energy conservation equation (the Vis-viva equation) for this orbit can take the form:
) of elliptic orbit is negative and the orbital energy conservation equation (the Vis-viva equation) for this orbit can take the form: is the speed of the orbiting body,
is the speed of the orbiting body, is the distance of the orbiting body from the center of mass of the central body,
is the distance of the orbiting body from the center of mass of the central body,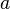 is the semi-major axis,
is the semi-major axis, is the standard gravitational parameter.
is the standard gravitational parameter.
- For a given semi-major axis the specific orbital energy is independent of the eccentricity.
- the time-average of the specific potential energy is equal to 2ε
- the time-average of r−1 is a−1
- the time-average of the specific kinetic energy is equal to -ε
Parabolic orbits
If the eccentricity equals 1, then the orbit equation becomes: is the radial distance of the orbiting body from the mass center of the central body,
is the radial distance of the orbiting body from the mass center of the central body,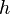 is specific angular momentum of the orbiting body,
is specific angular momentum of the orbiting body,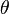 is the true anomaly of the orbiting body,
is the true anomaly of the orbiting body, is the standard gravitational parameter.
is the standard gravitational parameter.
 is the speed of the orbiting body.
is the speed of the orbiting body.
Hyperbolic orbits
If e > 1, the orbit formula,θ∞ = cos−1(-1/e)
sinθ∞ = (e2-1)1/2/e
Energy
Under standard assumptions, specific orbital energy ( ) of a hyperbolic trajectory is greater than zero and the orbital energy conservation equation for this kind of trajectory takes form:
) of a hyperbolic trajectory is greater than zero and the orbital energy conservation equation for this kind of trajectory takes form: is the orbital velocity of orbiting body,
is the orbital velocity of orbiting body, is the radial distance of orbiting body from central body,
is the radial distance of orbiting body from central body,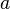 is the negative semi-major axis,
is the negative semi-major axis, is standard gravitational parameter.
is standard gravitational parameter.
Hyperbolic excess velocity
Under standard assumptions the body traveling along hyperbolic trajectory will attain in infinity an orbital velocity called hyperbolic excess velocity (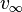 ) that can be computed as:
) that can be computed as:
 is standard gravitational parameter,
is standard gravitational parameter, is the negative semi-major axis of orbit's hyperbola.
is the negative semi-major axis of orbit's hyperbola.
Calculating trajectories
Kepler's equation
One approach to calculating orbits (mainly used historically) is to use Kepler's equation: .
.
 is the eccentricity.
is the eccentricity.With Kepler's formula, finding the time-of-flight to reach an angle (true anomaly) of
 from periapsis is broken into two steps:
from periapsis is broken into two steps:- Compute the eccentric anomaly
 from true anomaly
from true anomaly 
- Compute the time-of-flight
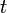 from the eccentric anomaly
from the eccentric anomaly 
 , meaning it cannot be solved for
, meaning it cannot be solved for  algebraically. Kepler's equation can be solved for
algebraically. Kepler's equation can be solved for  analytically by inversion.
analytically by inversion.A solution of Kepler's equation, valid for all real values of
 is:
is:![E =
\begin{cases}
\displaystyle \sum_{n=1}^{\infty}
{\frac{M^{\frac{n}{3}}}{n!}} \lim_{\theta \to 0} \left(
\frac{\mathrm{d}^{\,n-1}}{\mathrm{d}\theta^{\,n-1}} \left(
\frac{\theta}{ \sqrt[3]{\theta - \sin(\theta)} } ^n \right)
\right)
, & \epsilon = 1 \\
\displaystyle \sum_{n=1}^{\infty}
{ \frac{ M^n }{ n! } }
\lim_{\theta \to 0} \left(
\frac{\mathrm{d}^{\,n-1}}{\mathrm{d}\theta^{\,n-1}} \left(
\frac{ \theta }{ \theta - \epsilon \cdot \sin(\theta)} ^n \right)
\right)
, & \epsilon \ne 1
\end{cases}](http://upload.wikimedia.org/math/7/5/0/75038c7606574331ab8f06be8e78f9df.png)
Evaluating this yields:
Alternatively, Kepler's Equation can be solved numerically. First one must guess a value of
 and solve for time-of-flight; then adjust
and solve for time-of-flight; then adjust  as necessary to bring the computed time-of-flight closer to the desired value until the required precision is achieved. Usually, Newton's method is used to achieve relatively fast convergence.
as necessary to bring the computed time-of-flight closer to the desired value until the required precision is achieved. Usually, Newton's method is used to achieve relatively fast convergence.The main difficulty with this approach is that it can take prohibitively long to converge for the extreme elliptical orbits. For near-parabolic orbits, eccentricity
 is nearly 1, and plugging
is nearly 1, and plugging  into the formula for mean anomaly,
into the formula for mean anomaly,  , we find ourselves subtracting two nearly-equal values, and accuracy suffers. For near-circular orbits, it is hard to find the periapsis in the first place (and truly circular orbits have no periapsis at all). Furthermore, the equation was derived on the assumption of an elliptical orbit, and so it does not hold for parabolic or hyperbolic orbits. These difficulties are what led to the development of the universal variable formulation, described below.
, we find ourselves subtracting two nearly-equal values, and accuracy suffers. For near-circular orbits, it is hard to find the periapsis in the first place (and truly circular orbits have no periapsis at all). Furthermore, the equation was derived on the assumption of an elliptical orbit, and so it does not hold for parabolic or hyperbolic orbits. These difficulties are what led to the development of the universal variable formulation, described below.Conic orbits
For simple procedures, such as computing the delta-v for coplanar transfer ellipses, traditional approaches[clarification needed] are fairly effective. Others, such as time-of-flight are far more complicated, especially for near-circular and hyperbolic orbits.The patched conic approximation
The Hohmann transfer orbit alone is a poor approximation for interplanetary trajectories because it neglects the planets' own gravity. Planetary gravity dominates the behaviour of the spacecraft in the vicinity of a planet and in most cases Hohmann severely overestimates delta-v, and produces highly inaccurate prescriptions for burn timings.A relatively simple way to get a first-order approximation of delta-v is based on the 'Patched Conic Approximation' technique. One must choose the one dominant gravitating body in each region of space through which the trajectory will pass, and to model only that body's effects in that region. For instance, on a trajectory from the Earth to Mars, one would begin by considering only the Earth's gravity until the trajectory reaches a distance where the Earth's gravity no longer dominates that of the Sun. The spacecraft would be given escape velocity to send it on its way to interplanetary space. Next, one would consider only the Sun's gravity until the trajectory reaches the neighbourhood of Mars. During this stage, the transfer orbit model is appropriate. Finally, only Mars's gravity is considered during the final portion of the trajectory where Mars's gravity dominates the spacecraft's behaviour. The spacecraft would approach Mars on a hyperbolic orbit, and a final retrograde burn would slow the spacecraft enough to be captured by Mars.
The size of the "neighborhoods" (or spheres of influence) vary with radius
 :
: is the semimajor axis of the planet's orbit relative to the Sun;
is the semimajor axis of the planet's orbit relative to the Sun; 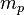 and
and  are the masses of the planet and Sun, respectively.
are the masses of the planet and Sun, respectively.This simplification is sufficient to compute rough estimates of fuel requirements, and rough time-of-flight estimates, but it is not generally accurate enough to guide a spacecraft to its destination. For that, numerical methods are required.
The universal variable formulation
To address computational shortcomings of traditional approaches for solving the 2-body problem, the universal variable formulation was developed. It works equally well for the circular, elliptical, parabolic, and hyperbolic cases, the differential equations converging well when integrated for any orbit. It also generalizes well to problems incorporating perturbation theory.Perturbations
The universal variable formulation works well with the variation of parameters technique, except now, instead of the six Keplerian orbital elements, we use a different set of orbital elements: namely, the satellite's initial position and velocity vectors and
and  at a given epoch
at a given epoch  . In a two-body simulation, these elements are sufficient to compute the satellite's position and velocity at any time in the future, using the universal variable formulation.
. In a two-body simulation, these elements are sufficient to compute the satellite's position and velocity at any time in the future, using the universal variable formulation.Conversely, at any moment in the satellite's orbit, we can measure its position and velocity, and then use the universal variable approach to determine what its initial position and velocity would have been at the epoch. In perfect two-body motion, these orbital elements would be invariant (just like the Keplerian elements would be).
However, perturbations cause the orbital elements to change over time. Hence, we write the position element as
 and the velocity element as
and the velocity element as  , indicating that they vary with time. The technique to compute the effect of perturbations becomes one of finding expressions, either exact or approximate, for the functions
, indicating that they vary with time. The technique to compute the effect of perturbations becomes one of finding expressions, either exact or approximate, for the functions  and
and  .
.The following are some effects which make real orbits differ from the simple models based on a spherical earth. Most of them can be handled on short timescales (perhaps less than a few thousand orbits) by perturbation theory because they are small relative to the corresponding two-body effects.
- Equatorial bulges cause precession of the node and the perigee
- Tesseral harmonics[1] of the gravity field introduce additional perturbations
- Lunar and solar gravity perturbations alter the orbits
- Atmospheric drag reduces the semi-major axis unless make-up thrust is used
Orbital maneuver
In spaceflight, an orbital maneuver is the use of propulsion systems to change the orbit of a spacecraft. For spacecraft far from Earth—for example those in orbits around the Sun—an orbital maneuver is called a deep-space maneuver (DSM).[not verified in body]Orbital transfer
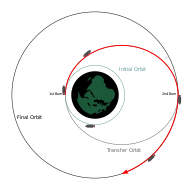
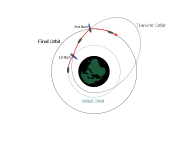
Transfer orbits are usually elliptical orbits that allow spacecraft to move from one (usually substantially circular) orbit to another. Usually they require a burn at the start, a burn at the end, and sometimes one or more burns in the middle.- The Hohmann transfer orbit requires a minimal delta-v.
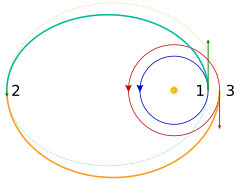
- A bi-elliptic transfer can require less energy than the Hohmann transfer, if the ratio of orbits is 11.94 or greater,[2] but comes at the cost of increased trip time over the Hohmann transfer.
- Faster transfers may use any orbit that intersects both the original and destination orbits, at the cost of higher delta-v.
A Hohmann transfer from a low circular orbit to a higher circular orbit
A bi-elliptic transfer from a low circular starting orbit (dark blue), to a higher circular orbit (red)
Gravity assist and the Oberth effect
In a gravity assist, a spacecraft swings by a planet and leaves in a different direction, at a different speed. This is useful to speed or slow a spacecraft instead of carrying more fuel.This maneuver can be approximated by an elastic collision at large distances, though the flyby does not involve any physical contact. Due to Newton's Third Law (equal and opposite reaction), any momentum gained by a spacecraft must be lost by the planet, or vice versa. However, because the planet is much, much more massive than the spacecraft, the effect on the planet's orbit is negligible.
The Oberth effect can be employed, particularly during a gravity assist operation. This effect is that use of a propulsion system works better at high speeds, and hence course changes are best done when close to a gravitating body; this can multiply the effective delta-v.
Interplanetary Transport Network and fuzzy orbits
It is now possible to use computers to search for routes using the nonlinearities in the gravity of the planets and moons of the Solar System. For example, it is possible to plot an orbit from high earth orbit to Mars, passing close to one of the Earth's Trojan points. Collectively referred to as the Interplanetary Transport Network, these highly perturbative, even chaotic, orbital trajectories in principle need no fuel beyond that needed to reach the Lagrange point (in practice keeping to the trajectory requires some course corrections). The biggest problem with them is they can be exceedingly slow, taking many years to arrive. In addition launch windows can be very far apart.They have, however, been employed on projects such as Genesis. This spacecraft visited the Earth-Sun Lagrange L1 point and returned using very little propellant.






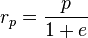

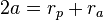
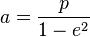


 is
is  is length of
is length of 
 is the distance between the orbiting bodies.
is the distance between the orbiting bodies.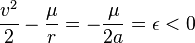
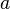 is the
is the 
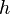 is
is 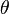 is the
is the 




 is
is 
 .
.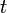 from the eccentric anomaly
from the eccentric anomaly