Probability distribution

From Wikipedia, the free encyclopedia

 

In probability theory and statistics, a probability distribution is a mathematical function that provides the probabilities of occurrence of different possible outcomes in an experiment. In more technical terms, the probability distribution is a description of a random phenomenon in terms of the probabilities of events. For instance, if the random variable X is used to denote the outcome of a coin toss ("the experiment"), then the probability distribution of X would take the value 0.5 for X = heads, and 0.5 for X = tails (assuming the coin is fair). Examples of random phenomena can include the results of an experiment or survey.

A probability distribution is defined in terms of an underlying sample space, which is the set of all possible outcomes of the random phenomenon being observed. The sample space may be the set of real numbers or a higher-dimensional vector space, or it may be a list of non-numerical values; for example, the sample space of a coin flip would be {heads, tails} .

Probability distributions are generally divided into two classes. A discrete probability distribution (applicable to the scenarios where the set of possible outcomes is discrete, such as a coin toss or a roll of dice) can be encoded by a discrete list of the probabilities of the outcomes, known as a probability mass function. On the other hand, a continuous probability distribution (applicable to the scenarios where the set of possible outcomes can take on values in a continuous range (e.g. real numbers), such as the temperature on a given day) is typically described by probability density functions (with the probability of any individual outcome actually being 0). The normal distribution is a commonly encountered continuous probability distribution. More complex experiments, such as those involving stochastic processes defined in continuous time, may demand the use of more general probability measures.

A probability distribution whose sample space is the set of real numbers is called univariate, while a distribution whose sample space is a vector space is called multivariate. A univariate distribution gives the probabilities of a single random variable taking on various alternative values; a multivariate distribution (a joint probability distribution) gives the probabilities of a random vector – a list of two or more random variables – taking on various combinations of values. Important and commonly encountered univariate probability distributions include the binomial distribution, the hypergeometric distribution, and the normal distribution. The multivariate normal distribution is a commonly encountered multivariate distribution.

Introduction

The probability mass function (pmf) p(S) specifies the probability distribution for the sum S of counts from two dice. For example, the figure shows that p(11) = 1/18. The pmf allows the computation of probabilities of events such as P(S > 9) = 1/12 + 1/18 + 1/36 = 1/6, and all other probabilities in the distribution.

To define probability distributions for the simplest cases, one needs to distinguish between discrete and continuous random variables. In the discrete case, it is sufficient to specify a probability mass function ${\displaystyle p}$ assigning a probability to each possible outcome: for example, when throwing a fair dice, each of the six values 1 to 6 has the probability 1/6. The probability of an event is then defined to be the sum of the probabilities of the outcomes that satisfy the event; for example, the probability of the event "the dice rolls an even value" is

${\displaystyle p(2)+p(4)+p(6)=1/6+1/6+1/6=1/2.}$

In contrast, when a random variable takes values from a continuum then typically, any individual outcome has probability zero and only events that include infinitely many outcomes, such as intervals, can have positive probability. For example, the probability that a given object weighs exactly 500 g is zero, because the probability of measuring exactly 500 g tends to zero as the accuracy of our measuring instruments increases. Nevertheless, in quality control one might demand that the probability of a "500 g" package containing between 490 g and 510 g should be no less than 98%, and this demand is less sensitive to the accuracy of measurement instruments.

Continuous probability distributions can be described in several ways. The probability density function describes the infinitesimal probability of any given value, and the probability that the outcome lies in a given interval can be computed by integrating the probability density function over that interval. On the other hand, the cumulative distribution function describes the probability that the random variable is no larger than a given value; the probability that the outcome lies in a given interval can be computed by taking the difference between the values of the cumulative distribution function at the endpoints of the interval. The cumulative distribution function is the antiderivative of the probability density function provided that the latter function exists.

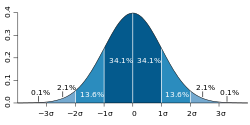
The probability density function (pdf) of the normal distribution, also called Gaussian or "bell curve", the most important continuous random distribution. As notated on the figure, the probabilities of intervals of values correspond to the area under the curve.

Terminology

As probability theory is used in quite diverse applications, terminology is not uniform and sometimes confusing. The following terms are used for non-cumulative probability distribution functions:

• Frequency distribution: A frequency distribution is a table that displays the frequency of various outcomes in a sample.
• Relative frequency distribution: A frequency distribution where each value has been divided (normalized) by a number of outcomes in a sample i.e. sample size.
• Probability distribution: Sometimes used as an alias for Relative frequency distribution but most books use it as a limit to which Relative frequency distribution tends when sample size tends to population size. It's a general term to indicate the way the total probability of 1 is distributed over all various possible outcomes (i.e. over entire population). It may for instance refer to a table that displays the probabilities of various outcomes in a finite population or to the probability density of an uncountably infinite population.
• Cumulative distribution function: is a general functional form to describe a probability distribution.
• Probability distribution function: somewhat ambiguous term sometimes referring to a functional form of probability distribution table. Could be called a "normalized frequency distribution function", where area under the graph equals to 1.
• Probability mass, Probability mass function, p.m.f., Discrete probability distribution function: for discrete random variables.
• Categorical distribution: for discrete random variables with a finite set of values.
• Probability density, Probability density function, p.d.f., Continuous probability distribution function: most often reserved for continuous random variables.

The following terms are somewhat ambiguous as they can refer to non-cumulative or cumulative distributions, depending on authors' preferences:

• Probability distribution function: continuous or discrete, non-cumulative or cumulative.
• Probability function: even more ambiguous, can mean any of the above or other things.

Basic terms

• Mode: for a discrete random variable, the value with highest probability (the location at which the probability mass function has its peak); for a continuous random variable, a location at which the probability density function has a local peak.
• Support: the smallest closed set whose complement has probability zero.
• Head: the range of values where the pmf or pdf is relatively high.
• Tail: the complement of the head within the support; the large set of values where the pmf or pdf is relatively low.
• Expected value or mean: the weighted average of the possible values, using their probabilities as their weights; or the continuous analog thereof.
• Median: the value such that the set of values less than the median, and the set greater than the median, each have probabilities no greater than one-half.
• Variance: the second moment of the pmf or pdf about the mean; an important measure of the dispersion of the distribution.
• Standard deviation: the square root of the variance, and hence another measure of dispersion.
• Symmetry: a property of some distributions in which the portion of the distribution to the left of a specific value is a mirror image of the portion to its right.
• Skewness: a measure of the extent to which a pmf or pdf "leans" to one side of its mean. The third standardized moment of the distribution.
• Kurtosis: a measure of the "fatness" of the tails of a pmf or pdf. The fourth standardized moment of the distribution.

Cumulative distribution function

Because a probability distribution P on the real line is determined by the probability of a scalar random variable X being in a half-open interval (−∞, x], the probability distribution is completely characterized by its cumulative distribution function:

${\displaystyle F(x)=\operatorname {P} [X\leq x]\qquad {\text{ for all }}x\in \mathbb {R} .}$

Discrete probability distribution

The probability mass function of a discrete probability distribution. The probabilities of the singletons {1}, {3}, and {7} are respectively 0.2, 0.5, 0.3. A set not containing any of these points has probability zero.

 

The cdf of a discrete probability distribution, ...

 

... of a continuous probability distribution, ...

 

... of a distribution which has both a continuous part and a discrete part.

A discrete probability distribution is a probability distribution characterized by a probability mass function. Thus, the distribution of a random variable X is discrete, and X is called a discrete random variable, if

${\displaystyle \sum _{u}\operatorname {P} (X=u)=1}$

as u runs through the set of all possible values of X. A discrete random variable can assume only a finite or countably infinite number of values. For the number of potential values to be countably infinite, even though their probabilities sum to 1, the probabilities have to decline to zero fast enough. For example, if ${\displaystyle \operatorname {P} (X=n)={\tfrac {1}{2^{n}}}}$ for n = 1, 2, ..., we have the sum of probabilities 1/2 + 1/4 + 1/8 + ... = 1.

Well-known discrete probability distributions used in statistical modeling include the Poisson distribution, the Bernoulli distribution, the binomial distribution, the geometric distribution, and the negative binomial distribution. Additionally, the discrete uniform distribution is commonly used in computer programs that make equal-probability random selections between a number of choices.

When a sample (a set of observations) is drawn from a larger population, the sample points have an empirical distribution that is discrete and that provides information about the population distribution.

Measure theoretic formulation

A measurable function ${\displaystyle X\colon A\to B}$ between a probability space ${\displaystyle (A,{\mathcal {A}},P)}$ and a measurable space ${\displaystyle (B,{\mathcal {B}})}$ is called a discrete random variable provided its image is a countable set and the pre-image of singleton sets are measurable, i.e., ${\displaystyle X^{-1}(b)\in {\mathcal {A}}}$ for all ${\displaystyle b\in B}$. The latter requirement induces a probability mass function ${\displaystyle f_{X}\colon X(A)\to \mathbb {R} }$ via ${\displaystyle f_{X}(b):=P(X^{-1}(b))}$. Since the pre-images of disjoint sets are disjoint

${\displaystyle \sum _{b\in X(A)}f_{X}(b)=\sum _{b\in X(A)}P(X^{-1}(b))=P\left(\bigcup _{b\in X(A)}X^{-1}(b)\right)=P(A)=1.}$

This recovers the definition given above.

Cumulative distribution function

Equivalently to the above, a discrete random variable can be defined as a random variable whose cumulative distribution function (cdf) increases only by jump discontinuities—that is, its cdf increases only where it "jumps" to a higher value, and is constant between those jumps. The points where jumps occur are precisely the values which the random variable may take.

Delta-function representation

Consequently, a discrete probability distribution is often represented as a generalized probability density function involving Dirac delta functions, which substantially unifies the treatment of continuous and discrete distributions. This is especially useful when dealing with probability distributions involving both a continuous and a discrete part.

Indicator-function representation

For a discrete random variable X, let u₀, u₁, ... be the values it can take with non-zero probability. Denote

${\displaystyle \Omega _{i}=X^{-1}(u_{i})=\{\omega :X(\omega )=u_{i}\},\,i=0,1,2,\dots }$

These are disjoint sets, and for such sets

${\displaystyle P\left(\bigcup _{i}\Omega _{i}\right)=\sum _{i}P(\Omega _{i})=\sum _{i}P(X=u_{i})=1.}$

It follows that the probability that X takes any value except for u₀, u₁, ... is zero, and thus one can write X as

${\displaystyle X(\omega )=\sum _{i}u_{i}1_{\Omega _{i}}(\omega )}$

except on a set of probability zero, where ${\displaystyle 1_{A}}$ is the indicator function of A. This may serve as an alternative definition of discrete random variables.

Continuous probability distribution

A continuous probability distribution is a probability distribution that has a cumulative distribution function that is continuous. Most often they are generated by having a probability density function. Mathematicians call distributions with probability density functions absolutely continuous, since their cumulative distribution function is absolutely continuous with respect to the Lebesgue measure λ. If the distribution of X is continuous, then X is called a continuous random variable. There are many examples of continuous probability distributions: normal, uniform, chi-squared, and others. Intuitively, a continuous random variable is the one which can take a continuous range of values—as opposed to a discrete distribution, where the set of possible values for the random variable is at most countable. While for a discrete distribution an event with probability zero is impossible (e.g., rolling π on a standard die has probability zero and is impossible), this is not so in the case of a continuous random variable. For example, if one measures the width of an oak leaf, the result of 3½ cm is possible; however, it has probability zero because uncountably many other potential values exist even between 3 cm and 4 cm. Each of these individual outcomes has probability zero, yet the probability that the outcome will fall into the interval (3 cm, 4 cm) is nonzero. This apparent paradox is resolved by the fact that the probability that X attains some value within an infinite set, such as an interval, cannot be found by naively adding the probabilities for individual values. Formally, each value has an infinitesimally small probability, which statistically is equivalent to zero.

Formally, if X is a continuous random variable, then it has a probability density function ƒ(x), and therefore its probability of falling into a given interval, say [a, b] is given by the integral

${\displaystyle \operatorname {P} [a\leq X\leq b]=\int _{a}^{b}f(x)\,dx}$

In particular, the probability for X to take any single value a (that is a ≤ X ≤ a) is zero, because an integral with coinciding upper and lower limits is always equal to zero.

The definition states that a continuous probability distribution must possess a density, or equivalently, its cumulative distribution function be absolutely continuous. This requirement is stronger than simple continuity of the cumulative distribution function, and there is a special class of distributions, singular distributions, which are neither continuous nor discrete nor a mixture of those. An example is given by the Cantor distribution. Such singular distributions however are never encountered in practice.

Note on terminology: some authors use the term "continuous distribution" to denote the distribution with continuous cumulative distribution function. Thus, their definition includes both the (absolutely) continuous and singular distributions.

By one convention, a probability distribution ${\displaystyle \,\mu }$ is called continuous if its cumulative distribution function ${\displaystyle F(x)=\mu (-\infty ,x]}$ is continuous and, therefore, the probability measure of singletons ${\displaystyle \mu \{x\}\,=\,0}$ for all ${\displaystyle \,x}$.

Another convention reserves the term continuous probability distribution for absolutely continuous distributions. These distributions can be characterized by a probability density function: a non-negative Lebesgue integrable function ${\displaystyle \,f}$ defined on the real numbers such that

${\displaystyle F(x)=\mu (-\infty ,x]=\int _{-\infty }^{x}f(t)\,dt.}$

Discrete distributions and some continuous distributions (like the Cantor distribution) do not admit such a density.

Some properties

• The probability distribution of the sum of two independent random variables is the convolution of each of their distributions.
• Probability distributions are not a vector space—they are not closed under linear combinations, as these do not preserve non-negativity or total integral 1—but they are closed under convex combination, thus forming a convex subset of the space of functions (or measures).

Kolmogorov definition

In the measure-theoretic formalization of probability theory, a random variable is defined as a measurable function X from a probability space ${\displaystyle \scriptstyle (\Omega ,{\mathcal {F}},\operatorname {P} )}$ to measurable space ${\displaystyle \scriptstyle ({\mathcal {X}},{\mathcal {A}})}$. A probability distribution of X is the pushforward measure X_*P  of X , which is a probability measure on ${\displaystyle \scriptstyle ({\mathcal {X}},{\mathcal {A}})}$ satisfying X_*P = PX ⁻¹.

Random number generation

A frequent problem in statistical simulations (the Monte Carlo method) is the generation of pseudo-random numbers that are distributed in a given way. Most algorithms are based on a pseudorandom number generator that produces numbers X that are uniformly distributed in the half-open interval [0,1). These random variates X are then transformed via some algorithm to create a new random variate having the required probability distribution.

Applications

The concept of the probability distribution and the random variables which they describe underlies the mathematical discipline of probability theory, and the science of statistics. There is spread or variability in almost any value that can be measured in a population (e.g. height of people, durability of a metal, sales growth, traffic flow, etc.); almost all measurements are made with some intrinsic error; in physics many processes are described probabilistically, from the kinetic properties of gases to the quantum mechanical description of fundamental particles. For these and many other reasons, simple numbers are often inadequate for describing a quantity, while probability distributions are often more appropriate.

As a more specific example of an application, the cache language models and other statistical language models used in natural language processing to assign probabilities to the occurrence of particular words and word sequences do so by means of probability distributions.

Common probability distributions

The following is a list of some of the most common probability distributions, grouped by the type of process that they are related to. For a more complete list, see list of probability distributions, which groups by the nature of the outcome being considered (discrete, continuous, multivariate, etc.) Note also that all of the univariate distributions below are singly peaked; that is, it is assumed that the values cluster around a single point. In practice, actually observed quantities may cluster around multiple values. Such quantities can be modeled using a mixture distribution.

Related to real-valued quantities that grow linearly (e.g. errors, offsets)

• Normal distribution (Gaussian distribution), for a single such quantity; the most common continuous distribution

Related to positive real-valued quantities that grow exponentially (e.g. prices, incomes, populations)

• Log-normal distribution, for a single such quantity whose log is normally distributed
• Pareto distribution, for a single such quantity whose log is exponentially distributed; the prototypical power law distribution

Related to real-valued quantities that are assumed to be uniformly distributed over a (possibly unknown) region

• Discrete uniform distribution, for a finite set of values (e.g. the outcome of a fair die)
• Continuous uniform distribution, for continuously distributed values

Related to Bernoulli trials (yes/no events, with a given probability)

• Basic distributions:
  • Bernoulli distribution, for the outcome of a single Bernoulli trial (e.g. success/failure, yes/no)
  • Binomial distribution, for the number of "positive occurrences" (e.g. successes, yes votes, etc.) given a fixed total number of independent occurrences
  • Negative binomial distribution, for binomial-type observations but where the quantity of interest is the number of failures before a given number of successes occurs
  • Geometric distribution, for binomial-type observations but where the quantity of interest is the number of failures before the first success; a special case of the negative binomial distribution
• Related to sampling schemes over a finite population:
  • Hypergeometric distribution, for the number of "positive occurrences" (e.g. successes, yes votes, etc.) given a fixed number of total occurrences, using sampling without replacement
  • Beta-binomial distribution, for the number of "positive occurrences" (e.g. successes, yes votes, etc.) given a fixed number of total occurrences, sampling using a Polya urn scheme (in some sense, the "opposite" of sampling without replacement)

Related to categorical outcomes (events with K possible outcomes, with a given probability for each outcome)

• Categorical distribution, for a single categorical outcome (e.g. yes/no/maybe in a survey); a generalization of the Bernoulli distribution
• Multinomial distribution, for the number of each type of categorical outcome, given a fixed number of total outcomes; a generalization of the binomial distribution
• Multivariate hypergeometric distribution, similar to the multinomial distribution, but using sampling without replacement; a generalization of the hypergeometric distribution

Related to events in a Poisson process (events that occur independently with a given rate)

• Poisson distribution, for the number of occurrences of a Poisson-type event in a given period of time
• Exponential distribution, for the time before the next Poisson-type event occurs
• Gamma distribution, for the time before the next k Poisson-type events occur

Related to the absolute values of vectors with normally distributed components

• Rayleigh distribution, for the distribution of vector magnitudes with Gaussian distributed orthogonal components. Rayleigh distributions are found in RF signals with Gaussian real and imaginary components.
• Rice distribution, a generalization of the Rayleigh distributions for where there is a stationary background signal component. Found in Rician fading of radio signals due to multipath propagation and in MR images with noise corruption on non-zero NMR signals.

Related to normally distributed quantities operated with sum of squares (for hypothesis testing)

• Chi-squared distribution, the distribution of a sum of squared standard normal variables; useful e.g. for inference regarding the sample variance of normally distributed samples (see chi-squared test)
• Student's t distribution, the distribution of the ratio of a standard normal variable and the square root of a scaled chi squared variable; useful for inference regarding the mean of normally distributed samples with unknown variance (see Student's t-test)
• F-distribution, the distribution of the ratio of two scaled chi squared variables; useful e.g. for inferences that involve comparing variances or involving R-squared (the squared correlation coefficient)

Useful as conjugate prior distributions in Bayesian inference

• Beta distribution, for a single probability (real number between 0 and 1); conjugate to the Bernoulli distribution and binomial distribution
• Gamma distribution, for a non-negative scaling parameter; conjugate to the rate parameter of a Poisson distribution or exponential distribution, the precision (inverse variance) of a normal distribution, etc.
• Dirichlet distribution, for a vector of probabilities that must sum to 1; conjugate to the categorical distribution and multinomial distribution; generalization of the beta distribution
• Wishart distribution, for a symmetric non-negative definite matrix; conjugate to the inverse of the covariance matrix of a multivariate normal distribution; generalization of the gamma distribution

Randomness

From Wikipedia, the free encyclopedia

A pseudorandomly generated bitmap.

Randomness is the lack of pattern or predictability in events. A random sequence of events, symbols or steps has no order and does not follow an intelligible pattern or combination. Individual random events are by definition unpredictable, but in many cases the frequency of different outcomes over a large number of events (or "trials") is predictable. For example, when throwing two dice, the outcome of any particular roll is unpredictable, but a sum of 7 will occur twice as often as 4. In this view, randomness is a measure of uncertainty of an outcome, rather than haphazardness, and applies to concepts of chance, probability, and information entropy.

The fields of mathematics, probability, and statistics use formal definitions of randomness. In statistics, a random variable is an assignment of a numerical value to each possible outcome of an event space. This association facilitates the identification and the calculation of probabilities of the events. Random variables can appear in random sequences. A random process is a sequence of random variables whose outcomes do not follow a deterministic pattern, but follow an evolution described by probability distributions. These and other constructs are extremely useful in probability theory and the various applications of randomness.

Randomness is most often used in statistics to signify well-defined statistical properties. Monte Carlo methods, which rely on random input (such as from random number generators or pseudorandom number generators), are important techniques in science, as, for instance, in computational science. By analogy, quasi-Monte Carlo methods use quasirandom number generators.

Random selection, when narrowly associated with a simple random sample, is a method of selecting items (often called units) from a population where the probability of choosing a specific item is the proportion of those items in the population. For example, with a bowl containing just 10 red marbles and 90 blue marbles, a random selection mechanism would choose a red marble with probability 1/10. Note that a random selection mechanism that selected 10 marbles from this bowl would not necessarily result in 1 red and 9 blue. In situations where a population consists of items that are distinguishable, a random selection mechanism requires equal probabilities for any item to be chosen. That is, if the selection process is such that each member of a population, of say research subjects, has the same probability of being chosen then we can say the selection process is random.

History

Ancient fresco of dice players in Pompei.

In ancient history, the concepts of chance and randomness were intertwined with that of fate. Many ancient peoples threw dice to determine fate, and this later evolved into games of chance. Most ancient cultures used various methods of divination to attempt to circumvent randomness and fate.

The Chinese of 3000 years ago were perhaps the earliest people to formalize odds and chance. The Greek philosophers discussed randomness at length, but only in non-quantitative forms. It was only in the 16th century that Italian mathematicians began to formalize the odds associated with various games of chance. The invention of the calculus had a positive impact on the formal study of randomness. In the 1888 edition of his book The Logic of Chance John Venn wrote a chapter on The conception of randomness that included his view of the randomness of the digits of the number pi by using them to construct a random walk in two dimensions.

The early part of the 20th century saw a rapid growth in the formal analysis of randomness, as various approaches to the mathematical foundations of probability were introduced. In the mid- to late-20th century, ideas of algorithmic information theory introduced new dimensions to the field via the concept of algorithmic randomness.

Although randomness had often been viewed as an obstacle and a nuisance for many centuries, in the 20th century computer scientists began to realize that the deliberate introduction of randomness into computations can be an effective tool for designing better algorithms. In some cases such randomized algorithms outperform the best deterministic methods.

In science

Many scientific fields are concerned with randomness:

• Algorithmic probability
• Chaos theory
• Cryptography
• Game theory
• Information theory
• Pattern recognition
• Probability theory
• Quantum mechanics
• Statistical mechanics
• Statistics

In the physical sciences

In the 19th century, scientists used the idea of random motions of molecules in the development of statistical mechanics to explain phenomena in thermodynamics and the properties of gases.

According to several standard interpretations of quantum mechanics, microscopic phenomena are objectively random. That is, in an experiment that controls all causally relevant parameters, some aspects of the outcome still vary randomly. For example, if a single unstable atom is placed in a controlled environment, it cannot be predicted how long it will take for the atom to decay—only the probability of decay in a given time. Thus, quantum mechanics does not specify the outcome of individual experiments but only the probabilities. Hidden variable theories reject the view that nature contains irreducible randomness: such theories posit that in the processes that appear random, properties with a certain statistical distribution are at work behind the scenes, determining the outcome in each case.

In biology

The modern evolutionary synthesis ascribes the observed diversity of life to random genetic mutations followed by natural selection. The latter retains some random mutations in the gene pool due to the systematically improved chance for survival and reproduction that those mutated genes confer on individuals who possess them.

Several authors also claim that evolution and sometimes development require a specific form of randomness, namely the introduction of qualitatively new behaviors. Instead of the choice of one possibility among several pre-given ones, this randomness corresponds to the formation of new possibilities.

The characteristics of an organism arise to some extent deterministically (e.g., under the influence of genes and the environment) and to some extent randomly. For example, the density of freckles that appear on a person's skin is controlled by genes and exposure to light; whereas the exact location of individual freckles seems random.

As far as behavior is concerned, randomness is important if an animal is to behave in a way that is unpredictable to others. For instance, insects in flight tend to move about with random changes in direction, making it difficult for pursuing predators to predict their trajectories.

In mathematics

The mathematical theory of probability arose from attempts to formulate mathematical descriptions of chance events, originally in the context of gambling, but later in connection with physics. Statistics is used to infer the underlying probability distribution of a collection of empirical observations. For the purposes of simulation, it is necessary to have a large supply of random numbers or means to generate them on demand.

Algorithmic information theory studies, among other topics, what constitutes a random sequence. The central idea is that a string of bits is random if and only if it is shorter than any computer program that can produce that string (Kolmogorov randomness)—this means that random strings are those that cannot be compressed. Pioneers of this field include Andrey Kolmogorov and his student Per Martin-Löf, Ray Solomonoff, and Gregory Chaitin. For the notion of infinite sequence, one normally uses Per Martin-Löf's definition. That is, an infinite sequence is random if and only if it withstands all recursively enumerable null sets. The other notions of random sequences include (but not limited to): recursive randomness and Schnorr randomness which are based on recursively computable martingales. It was shown by Yongge Wang that these randomness notions are generally different.

Randomness occurs in numbers such as log (2) and pi. The decimal digits of pi constitute an infinite sequence and "never repeat in a cyclical fashion." Numbers like pi are also considered likely to be normal, which means their digits are random in a certain statistical sense.

Pi certainly seems to behave this way. In the first six billion decimal places of pi, each of the digits from 0 through 9 shows up about six hundred million times. Yet such results, conceivably accidental, do not prove normality even in base 10, much less normality in other number bases.

In statistics

In statistics, randomness is commonly used to create simple random samples. This lets surveys of completely random groups of people provide realistic data. Common methods of doing this include drawing names out of a hat or using a random digit chart. A random digit chart is simply a large table of random digits.

In information science

In information science, irrelevant or meaningless data is considered noise. Noise consists of a large number of transient disturbances with a statistically randomized time distribution.

In communication theory, randomness in a signal is called "noise" and is opposed to that component of its variation that is causally attributable to the source, the signal.

In terms of the development of random networks, for communication randomness rests on the two simple assumptions of Paul Erdős and Alfréd Rényi who said that there were a fixed number of nodes and this number remained fixed for the life of the network, and that all nodes were equal and linked randomly to each other.

In finance

The random walk hypothesis considers that asset prices in an organized market evolve at random, in the sense that the expected value of their change is zero but the actual value may turn out to be positive or negative. More generally, asset prices are influenced by a variety of unpredictable events in the general economic environment.

In politics

Random selection can be an official method to resolve tied elections in some jurisdictions. Its use in politics is very old, as office holders in Ancient Athens were chosen by lot, there being no voting.

Randomness and religion

Randomness can be seen as conflicting with the deterministic ideas of some religions, such as those where the universe is created by an omniscient deity who is aware of all past and future events. If the universe is regarded to have a purpose, then randomness can be seen as impossible. This is one of the rationales for religious opposition to evolution, which states that non-random selection is applied to the results of random genetic variation.

Hindu and Buddhist philosophies state that any event is the result of previous events, as reflected in the concept of karma, and as such there is no such thing as a random event or a first event
.
In some religious contexts, procedures that are commonly perceived as randomizers are used for divination. Cleromancy uses the casting of bones or dice to reveal what is seen as the will of the gods.

Applications

In most of its mathematical, political, social and religious uses, randomness is used for its innate "fairness" and lack of bias.

Politics: Athenian democracy was based on the concept of isonomia (equality of political rights) and used complex allotment machines to ensure that the positions on the ruling committees that ran Athens were fairly allocated. Allotment is now restricted to selecting jurors in Anglo-Saxon legal systems and in situations where "fairness" is approximated by randomization, such as selecting jurors and military draft lotteries.

Games: Random numbers were first investigated in the context of gambling, and many randomizing devices, such as dice, shuffling playing cards, and roulette wheels, were first developed for use in gambling. The ability to produce random numbers fairly is vital to electronic gambling, and, as such, the methods used to create them are usually regulated by government Gaming Control Boards. Random drawings are also used to determine lottery winners. Throughout history, randomness has been used for games of chance and to select out individuals for an unwanted task in a fair way.

Sports: Some sports, including American football, use coin tosses to randomly select starting conditions for games or seed tied teams for postseason play. The National Basketball Association uses a weighted lottery to order teams in its draft.

Mathematics: Random numbers are also employed where their use is mathematically important, such as sampling for opinion polls and for statistical sampling in quality control systems. Computational solutions for some types of problems use random numbers extensively, such as in the Monte Carlo method and in genetic algorithms.

Medicine: Random allocation of a clinical intervention is used to reduce bias in controlled trials (e.g., randomized controlled trials).

Religion: Although not intended to be random, various forms of divination such as cleromancy see what appears to be a random event as a means for a divine being to communicate their will.

Generation

The ball in a roulette can be used as a source of apparent randomness, because its behavior is very sensitive to the initial conditions.

It is generally accepted that there exist three mechanisms responsible for (apparently) random behavior in systems:

1. Randomness coming from the environment (for example, Brownian motion, but also hardware random number generators)
2. Randomness coming from the initial conditions. This aspect is studied by chaos theory and is observed in systems whose behavior is very sensitive to small variations in initial conditions (such as pachinko machines and dice).
3. Randomness intrinsically generated by the system. This is also called pseudorandomness and is the kind used in pseudo-random number generators. There are many algorithms (based on arithmetics or cellular automaton) to generate pseudorandom numbers. The behavior of the system can be determined by knowing the seed state and the algorithm used. These methods are often quicker than getting "true" randomness from the environment.

The many applications of randomness have led to many different methods for generating random data. These methods may vary as to how unpredictable or statistically random they are, and how quickly they can generate random numbers.

Before the advent of computational random number generators, generating large amounts of sufficiently random numbers (important in statistics) required a lot of work. Results would sometimes be collected and distributed as random number tables.

Measures and tests

There are many practical measures of randomness for a binary sequence. These include measures based on frequency, discrete transforms, and complexity, or a mixture of these. These include tests by Kak, Phillips, Yuen, Hopkins, Beth and Dai, Mund, and Marsaglia and Zaman.

Quantum Non-Locality has been used to certify the presence of genuine randomness in a given string of numbers.

Misconceptions and logical fallacies

Popular perceptions of randomness are frequently mistaken, based on fallacious reasoning or intuitions.

A number is "due"

This argument is, "In a random selection of numbers, since all numbers eventually appear, those that have not come up yet are 'due', and thus more likely to come up soon." This logic is only correct if applied to a system where numbers that come up are removed from the system, such as when playing cards are drawn and not returned to the deck. In this case, once a jack is removed from the deck, the next draw is less likely to be a jack and more likely to be some other card. However, if the jack is returned to the deck, and the deck is thoroughly reshuffled, a jack is as likely to be drawn as any other card. The same applies in any other process where objects are selected independently, and none are removed after each event, such as the roll of a die, a coin toss, or most lottery number selection schemes. Truly random processes such as these do not have memory, making it impossible for past outcomes to affect future outcomes.

A number is "cursed" or "blessed"

In a random sequence of numbers, a number may be said to be cursed because it has come up less often in the past, and so it is thought that it will occur less often in the future. A number may be assumed to be blessed because it has occurred more often than others in the past, and so it is thought likely to come up more often in the future. This logic is valid only if the randomisation is biased, for example with a loaded die. If the die is fair, then previous rolls give no indication of future events. In nature, events rarely occur with perfectly equal frequency, so observing outcomes to determine which events are more probable makes sense. It is fallacious to apply this logic to systems designed to make all outcomes equally likely, such as shuffled cards, dice, and roulette wheels.

Odds are never dynamic

In the beginning of a scenario, one might calculate the probability of a certain event. The fact is, as soon as one gains more information about that situation, they may need to re-calculate the probability.

When the host reveals one door that contains a goat, this is new information.

Say we are told that a woman has two children. If we ask whether either of them is a girl, and are told yes, what is the probability that the other child is also a girl? Considering this new child independently, one might expect the probability that the other child is female is ½ (50%). But by building a probability space (illustrating all possible outcomes), we see that the probability is actually only ⅓ (33%). This is because the possibility space illustrates 4 ways of having these two children: boy-boy, girl-boy, boy-girl, and girl-girl. But we were given more information. Once we are told that one of the children is a female, we use this new information to eliminate the boy-boy scenario. Thus the probability space reveals that there are still 3 ways to have two children where one is a female: boy-girl, girl-boy, girl-girl. Only ⅓ of these scenarios would have the other child also be a girl. Using a probability space, we are less likely to miss one of the possible scenarios, or to neglect the importance of new information. For further information, see Boy or girl paradox.

This technique provides insights in other situations such as the Monty Hall problem, a game show scenario in which a car is hidden behind one of three doors, and two goats are hidden as booby prizes behind the others. Once the contestant has chosen a door, the host opens one of the remaining doors to reveal a goat, eliminating that door as an option. With only two doors left (one with the car, the other with another goat), the player must decide to either keep their decision, or switch and select the other door. Intuitively, one might think the player is choosing between two doors with equal probability, and that the opportunity to choose another door makes no difference. But probability spaces reveal that the contestant has received new information, and can increase their chances of winning by changing to the other door.