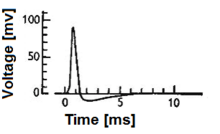
Biological neuron model

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Biological_neuron_model 

 

Fig. 1. Neuron and myelinated axon, with signal flow from inputs at dendrites to outputs at axon terminals. The signal is a short electrical pulse called action potential or 'spike'.

 

Fig 2. Time course of neuronal action potential ("spike"). Note that the amplitude and the exact shape of the action potential can vary according to the exact experimental technique used for acquiring the signal.

Biological neuron models, also known as a spiking neuron models, are mathematical descriptions of the properties of certain cells in the nervous system that generate sharp electrical potentials across their cell membrane, roughly one millisecond in duration, called action potentials or spikes (Fig. 2). Since spikes are transmitted along the axon and synapses from the sending neuron to many other neurons, spiking neurons are considered to be a major information processing unit of the nervous system. Spiking neuron models can be divided into different categories: the most detailed mathematical models are biophysical neuron models (also called Hodgkin-Huxley models) that describe the membrane voltage as a function of the input current and the activation of ion channels. Mathematically simpler are integrate-and-fire models that describe the membrane voltage as a function of the input current and predict the spike times without a description of the biophysical processes that shape the time course of an action potential. Even more abstract models only predict output spikes (but not membrane voltage) as a function of the stimulation where the stimulation can occur through sensory input or pharmacologically. This article provides a short overview of different spiking neuron models and links, whenever possible to experimental phenomena. It includes deterministic and probabilistic models.

Introduction: Biological background, classification and aims of neuron models

Non-spiking cells, spiking cells, and their measurement

Not all the cells of the nervous system produce the type of spike that define the scope of the spiking neuron models. For example, cochlear hair cells, retinal receptor cells, and retinal bipolar cells do not spike. Furthermore, many cells in the nervous system are not classified as neurons but instead are classified as glia.

Neuronal activity can be measured with different experimental techniques, such as the "Whole cell" measurement technique, which captures the spiking activity of a single neuron and produces full amplitude action potentials.

With extracellular measurement techniques an electrode (or array of several electrodes) is located in the extracellular space. Spikes, often from several spiking sources, depending on the size of the electrode and its proximity to the sources, can be identified with signal processing techniques. Extracellular measurement has several advantages: 1) Is easier to obtain experimentally; 2) Is robust and lasts for a longer time; 3) Can reflect the dominant effect, especially when conducted in an anatomical region with many similar cells.

Overview of neuron models

Neuron models can be divided into two categories according to the physical units of the interface of the model. Each category could be further divided according to the abstraction/detail level:

1. Electrical input–output membrane voltage models – These models produce a prediction for membrane output voltage as a function of electrical stimulation given as current or voltage input. The various models in this category differ in the exact functional relationship between the input current and the output voltage and in the level of details. Some models in this category predict only the moment of occurrence of output spike (also known as "action potential"); other models are more detailed and account for sub-cellular processes. The models in this category can be either deterministic or probabilistic.
2. Natural stimulus or pharmacological input neuron models – The models in this category connect between the input stimulus which can be either pharmacological or natural, to the probability of a spike event. The input stage of these models is not electrical, but rather has either pharmacological (chemical) concentration units, or physical units that characterize an external stimulus such as light, sound or other forms of physical pressure. Furthermore, the output stage represents the probability of a spike event and not an electrical voltage.

Although it is not unusual in science and engineering to have several descriptive models for different abstraction/detail levels, the number of different, sometimes contradicting, biological neuron models is exceptionally high. This situation is partly the result of the many different experimental settings, and the difficulty to separate the intrinsic properties of a single neuron from measurements effects and interactions of many cells (network effects). To accelerate the convergence to a unified theory, we list several models in each category, and where applicable, also references to supporting experiments.

Aims of neuron models

Ultimately, biological neuron models aim to explain the mechanisms underlying the operation of the nervous system. However several approaches can be distinguished from more realistic models (e.g., mechanistic models) to more pragmatic models (e.g., phenomenological models). Modeling helps to analyze experimental data and address questions such as: How are the spikes of a neuron related to sensory stimulation or motor activity such as arm movements? What is the neural code used by the nervous system? Models are also important in the context of restoring lost brain functionality through neuroprosthetic devices.

Electrical input–output membrane voltage models

The models in this category describe the relationship between neuronal membrane currents at the input stage, and membrane voltage at the output stage. This category includes (generalized) integrate-and-fire models and biophysical models inspired by the work of Hodgkin–Huxley in the early 1950s using an experimental setup that punctured the cell membrane and allowed to force a specific membrane voltage/current.

Most modern electrical neural interfaces apply extra-cellular electrical stimulation to avoid membrane puncturing which can lead to cell death and tissue damage. Hence, it is not clear to what extent the electrical neuron models hold for extra-cellular stimulation.

Hodgkin–Huxley

Main article: Hodgkin–Huxley model

The Hodgkin–Huxley model (H&H model) is a model of the relationship between the flow of ionic currents across the neuronal cell membrane and the membrane voltage of the cell. It consists of a set of nonlinear differential equations describing the behaviour of ion channels that permeate the cell membrane of the squid giant axon. Hodgkin and Huxley were awarded the 1963 Nobel Prize in Physiology or Medicine for this work.

We note the voltage-current relationship, with multiple voltage-dependent currents charging the cell membrane of capacity C_m

${\displaystyle C_{\mathrm {m} }{\frac {dV(t)}{dt}}=-\sum _{i}I_{i}(t,V).}$

The above equation is the time derivative of the law of capacitance, Q = CV where the change of the total charge must be explained as the sum over the currents. Each current is given by

${\displaystyle I(t,V)=g(t,V)\cdot (V-V_{\mathrm {eq} })}$

where g(t,V) is the conductance, or inverse resistance, which can be expanded in terms of its maximal conductance ḡ and the activation and inactivation fractions m and h, respectively, that determine how many ions can flow through available membrane channels. This expansion is given by

${\displaystyle g(t,V)={\bar {g}}\cdot m(t,V)^{p}\cdot h(t,V)^{q}}$

and our fractions follow the first-order kinetics

${\displaystyle {\frac {dm(t,V)}{dt}}={\frac {m_{\infty }(V)-m(t,V)}{\tau _{\mathrm {m} }(V)}}=\alpha _{\mathrm {m} }(V)\cdot (1-m)-\beta _{\mathrm {m} }(V)\cdot m}$

with similar dynamics for h, where we can use either τ and m_∞ or α and β to define our gate fractions.

The Hodgkin–Huxley model may be extended to include additional ionic currents. Typically, these include inward Ca²⁺ and Na⁺ input currents, as well as several varieties of K⁺ outward currents, including a "leak" current.

The end result can be at the small end 20 parameters which one must estimate or measure for an accurate model. In a model of a complex systems of neurons, numerical integration of the equations are computationally expensive. Careful simplifications of the Hodgkin–Huxley model are therefore needed.

The model can be reduced to two dimensions thanks to the dynamic relations which can be established between the gating variables. it is also possible to extend it to take into account the evolution of the concentrations (considered fixed in the original model).

Perfect Integrate-and-fire

One of the earliest models of a neuron is the perfect integrate-and-fire model (also called non-leaky integrate-and-fire), first investigated in 1907 by Louis Lapicque. A neuron is represented by its membrane voltage V which evolves in time during stimulation with an input current I(t) according

${\displaystyle I(t)=C{\frac {dV(t)}{dt}}}$

which is just the time derivative of the law of capacitance, Q = CV. When an input current is applied, the membrane voltage increases with time until it reaches a constant threshold V_th, at which point a delta function spike occurs and the voltage is reset to its resting potential, after which the model continues to run. The firing frequency of the model thus increases linearly without bound as input current increases.

The model can be made more accurate by introducing a refractory period t_ref that limits the firing frequency of a neuron by preventing it from firing during that period. For constant input I(t)=I the threshold voltage is reached after an integration time t_int=CV_thr/I after start from zero. After a reset, the refractory period introduces a dead time so that the total time until the next firing is t_ref+t_int . The firing frequency is the inverse of the total inter-spike interval (including dead time). The firing frequency as a function of a constant input current is therefore

${\displaystyle \,\!f(I)={\frac {I}{C_{\mathrm {} }V_{\mathrm {th} }+t_{\mathrm {ref} }I}}.}$

A shortcoming of this model is that it describes neither adaptation nor leakage. If the model receives a below-threshold short current pulse at some time, it will retain that voltage boost forever - until another input later makes it fire. This characteristic is clearly not in line with observed neuronal behavior. The following extensions make the integrate-and-fire model more plausible from a biological point of view.

Leaky integrate-and-fire

The leaky integrate-and-fire model which can be traced back to Louis Lapicque, contains, compared to the non-leaky integrate-and-fire model a "leak" term in the membrane potential equation, reflecting the diffusion of ions through the membrane. The model equation looks like

${\displaystyle C_{\mathrm {m} }{\frac {dV_{\mathrm {m} }(t)}{dt}}=I(t)-{\frac {V_{\mathrm {m} }(t)}{R_{\mathrm {m} }}}}$

A neuron is represented by an RC circuit with a threshold. Each input pulse (e.g. caused by a spike from a different neuron) causes a short current pulse. Voltage decays exponentially. If the threshold is reached an output spike is generated and the voltage is reset.

where V_m is the voltage across the cell membrane and R_m is the membrane resistance. (The non-leaky integrate-and-fire model is retrieved in the limit R_m to infinity, i.e. if the membrane is a perfect insulator). The model equation is valid for arbitrary time-dependent input until a threshold V_th is reached; thereafter the membrane potential is reset.

For constant input, the minimum input to reach the threshold is I_th = V_th / R_m. Assuming a reset to zero, the firing frequency thus looks like

${\displaystyle f(I)={\begin{cases}0,&I\leq I_{\mathrm {th} }\\\left[t_{\mathrm {ref} }-R_{\mathrm {m} }C_{\mathrm {m} }\log \left(1-{\tfrac {V_{\mathrm {th} }}{IR_{\mathrm {m} }}}\right)\right]^{-1},&I>I_{\mathrm {th} }\end{cases}}}$

which converges for large input currents to the previous leak-free model with refractory period. The model can also be used for inhibitory neurons.

The biggest disadvantage of the Leaky integrate-and-fire neuron is that it does not contain neuronal adaptation so that it cannot describe an experimentally measured spike train in response to constant input current. This disadvantage is removed in generalized integrate-and-fire models that also contain one or several adaptation-variables and are able to predict spike times of cortical neurons under current injection to a high degree of accuracy.

Adaptive integrate-and-fire

Neuronal adaptation refers to the fact that even in the presence of a constant current injection into the soma, the intervals between output spikes increase. An adaptive integrate-and-fire neuron model combines the leaky integration of voltage V with one or several adaptation variables w_k

${\displaystyle \tau _{\mathrm {m} }{\frac {dV_{\mathrm {m} }(t)}{dt}}=RI(t)-[V_{\mathrm {m} }(t)-E_{\mathrm {m} }]-R\sum _{k}w_{k}}$

${\displaystyle \tau _{k}{\frac {dw_{k}(t)}{dt}}=-a_{k}[V_{\mathrm {m} }(t)-E_{\mathrm {m} }]-w_{k}+b_{k}\tau _{k}\sum _{f}\delta (t-t^{f})}$

where ${\displaystyle \tau _{m}}$ is the membrane time constant , w_k is the adaptation current number, with index k, ${\displaystyle \tau _{k}}$ is the time constant of adaptation current w_k, E_m is the resting potential and t^f is the firing time of the neuron and the Greek delta denotes the Dirac delta function. Whenever the voltage reaches the firing threshold the voltage is reset to a value V_r below the firing threshold. The reset value is one of the important parameters of the model. The simplest model of adaptation has only a single adaptation variable w and the sum over k is removed.

Spike times and subthreshold voltage of cortical neuron models can be predicted by generalized integrate-and-fire models such as the adaptive integrate-and-fire model, the adaptive exponential integrate-and-fire model, or the spike response model. In the example here, adaptation is implemented by a dynamic threshold which increases after each spike.

Integrate-and-fire neurons with one or several adaptation variables can account for a variety of neuronal firing patterns in response to constant stimulation, including adaptation, bursting and initial bursting. Moreover, adaptive integrate-and-fire neurons with several adaptation variables are able to predict spike times of cortical neurons under time-dependent current injection into the soma.

Fractional-order leaky integrate-and-fire

Recent advances in computational and theoretical fractional calculus lead to a new form of model, called Fractional-order leaky integrate-and-fire. An advantage of this model is that it can capture adaptation effects with a single variable. The model has the following form

${\displaystyle I(t)-{\frac {V_{\mathrm {m} }(t)}{R_{\mathrm {m} }}}=C_{\mathrm {m} }{\frac {d^{\alpha }V_{\mathrm {m} }(t)}{d^{\alpha }t}}}$

Once the voltage hits the threshold it is reset. Fractional integration has been used to account for neuronal adaptation in experimental data.

'Exponential integrate-and-fire' and 'adaptive exponential integrate-and-fire'

Main article: Exponential integrate-and-fire

In the exponential integrate-and-fire model, spike generation is exponential, following the equation:

${\displaystyle {\frac {dV}{dt}}-{\frac {R}{\tau _{m}}}I(t)={\frac {1}{\tau _{m}}}\left[E_{m}-V+\Delta _{T}\exp \left({\frac {V-V_{T}}{\Delta _{T}}}\right)\right].}$

where ${\displaystyle V}$ is the membrane potential, ${\displaystyle V_{T}}$ is the intrinsic membrane potential threshold, ${\displaystyle \tau _{m}}$ is the membrane time constant, ${\displaystyle E_{m}}$is the resting potential, and ${\displaystyle \Delta _{T}}$ is the sharpness of action potential initiation, usually around 1 mV for cortical pyramidal neurons. Once the membrane potential crosses ${\displaystyle V_{T}}$, it diverges to infinity in finite time. In numerical simulation the integration is stopped if the membrane potential hits an arbitrary threshold (much larger than ${\displaystyle V_{T}}$) at which the membrane potential is reset to a value V_r . The voltage reset value V_r is one of the important parameters of the model. Importantly, the right-hand side of the above equation contains a nonlinearity that can be directly extracted from experimental data. In this sense the exponential nonlinearity is strongly supported by experimental evidence.

In the adaptive exponential integrate-and-fire neuron  the above exponential nonlinearity of the voltage equation is combined with an adaptation variabe w

${\displaystyle \tau _{m}{\frac {dV}{dt}}=RI(t)+\left[E_{m}-V+\Delta _{T}\exp \left({\frac {V-V_{T}}{\Delta _{T}}}\right)\right]-Rw}$

${\displaystyle \tau {\frac {dw(t)}{dt}}=-a[V_{\mathrm {m} }(t)-E_{\mathrm {m} }]-w+b\tau \delta (t-t^{f})}$

Firing pattern of initial bursting in response to a step current input generated with the Adaptive exponential integrate-and-fire model. Other Firing patterns can also be generated.

where w denotes the adaptation current with time scale ${\displaystyle \tau }$. Important model parameters are the voltage reset value V_r, the intrinsic threshold ${\displaystyle V_{T}}$, the time constants ${\displaystyle \tau }$ and ${\displaystyle \tau _{m}}$ as well as the coupling parameters a and b. The adaptive exponential integrate-and-fire model inherits the experimentally derived voltage nonlinearity of the exponential integrate-and-fire model. But going beyond this model, it can also account for a variety of neuronal firing patterns in response to constant stimulation, including adaptation, bursting and initial bursting. However, since the adaptation is in the form of a current, aberrant hyperpolarization may appear. This problem was solved by expressing it as a conductance.

Stochastic models of membrane voltage and spike timing

The models in this category are generalized integrate-and-fire models that include a certain level of stochasticity. Cortical neurons in experiments are found to respond reliably to time-dependent input, albeit with a small degree of variations between one trial and the next if the same stimulus is repeated.  Stochasticity in neurons has two important sources. First, even in a very controlled experiment where input current is injected directly into the soma, ion channels open and close stochastically and this channel noise leads to a small amount of variability in the exact value of the membrane potential and the exact timing of output spikes. Second, for a neuron embedded in a cortical network, it is hard to control the exact input because most inputs come from unobserved neurons somewhere else in the brain.

Stochasticity has been introduces into spiking neuron models in two fundamentally different forms: either (i) a noisy input current is added to the differential equation of the neuron model; or (ii) the process of spike generation is noisy. In both cases, the mathematical theory can be developed for continuous time, which is then, if desired for the use in computer simulations, transformed into a discrete-time model.

The relation of noise in neuron models to variability of spike trains and neural codes is discussed in Neural Coding and in Chapter 7 of the textbook Neuronal Dynamics.

Noisy input model (diffusive noise)

A neuron embedded in a network receives spike input from other neurons. Since the spike arrival times are not controlled by an experimentalist they can be considered as stochastic. Thus a (potentially nonlinear) integrate-and-fire model with nonlinearity f(v) receives two inputs: an input ${\displaystyle I(t)}$ controlled by the experimentalists and a noisy input current ${\displaystyle I^{\rm {noise}}(t)}$ that describes the uncontrolled background input.

${\displaystyle \tau _{m}{\frac {dV}{dt}}=f(V)+RI(t)+RI^{\text{noise}}(t)}$

Stein's model is the special case of a leaky integrate-and-fire neuron and a stationary white noise current ${\displaystyle I^{\rm {noise}}(t)=\xi (t)}$ with mean zero and unit variance. In the subthreshold regime, these assumptions yield the equation of the Ornstein–Uhlenbeck process

${\displaystyle \tau _{m}{\frac {dV}{dt}}=[E_{m}-V]+RI(t)+R\xi (t)}$

However, in contrast to the standard Ornstein–Uhlenbeck process, the membrane voltage is reset whenever V hits the firing threshold V_th . Calculating the interval distribution of the Ornstein–Uhlenbeck model for constant input with threshold leads to a first-passage time problem. Stein's neuron model and variants thereof have been used to fit interspike interval distributions of spike trains from real neurons under constant input current.

In the mathematical literature, the above equation of the Ornstein–Uhlenbeck process is written in the form

${\displaystyle dV=[E_{m}-V+RI(t)]{\frac {dt}{\tau _{m}}}+\sigma \,dW}$

where ${\displaystyle \sigma }$ is the amplitude of the noise input and dW are increments of a Wiener process. For discrete-time implementations with time step dt the voltage updates are

${\displaystyle \Delta V=[E_{m}-V+RI(t)]{\frac {\Delta t}{\tau _{m}}}+\sigma {\sqrt {\tau _{m}}}y}$

where y is drawn from a Gaussian distribution with zero mean unit variance. The voltage is reset when it hits the firing threshold V_th .

The noisy input model can also be used in generalized integrate-and-fire models. For example, the exponential integrate-and-fire model with noisy input reads

${\displaystyle \tau _{m}{\frac {dV}{dt}}=E_{m}-V+\Delta _{T}\exp \left({\frac {V-V_{T}}{\Delta _{T}}}\right)+RI(t)+R\xi (t)}$

For constant deterministic input ${\displaystyle I(t)=I_{0}}$ it is possible to calculate the mean firing rate as a function of ${\displaystyle I_{0}}$. This is important because the frequency-current relation (f-I-curve) is often used by experimentalists to characterize a neuron. It is also the transfer function in

The leaky integrate-and-fire with noisy input has been widely used in the analysis of networks of spiking neurons. Noisy input is also called 'diffusive noise' because it leads to a diffusion of the subthreshold membrane potential around the noise-free trajectory (Johannesma, The theory of spiking neurons with noisy input is reviewed in Chapter 8.2 of the textbook Neuronal Dynamics.

Noisy output model (escape noise)

In deterministic integrate-and-fire models, a spike is generated if the membrane potential V(t) hits the threshold ${\displaystyle V_{th}}$. In noisy output models the strict threshold is replaced by a noisy one as follows. At each moment in time t, a spike is generated stochastically with instantaneous stochastic intensity or 'escape rate' 

${\displaystyle \rho (t)=f(V(t)-V_{th})}$

that depends on the momentary difference between the membrane voltage V(t) and the threshold ${\displaystyle V_{th}}$. A common choice for the 'escape rate' ${\displaystyle f}$ (that is consistent with biological data) is

${\displaystyle f(V-V_{th})={\frac {1}{\tau _{0}}}\exp[\beta (V-V_{th}]}$

Stochastic spike generation (noisy output) depends on the momentary difference between the membrane potential V(t) and the threshold. The membrane potential V of the spike response model (SRM) has two contributions. First, input current I is filtered by a first filter k. Second the sequence of output spikes S(t) is filtered by a second filter η and fed back. The resulting membrane V(t) potential is used to generate output spikes by a stochastic process ρ(t) with an intensity that depends on the distance between membrane potential and threshold. The spike response model (SRM) is closely related to the Generalized Linear Model (GLM).

where ${\displaystyle \tau _{0}}$is a time constant that describes how quickly a spike is fired once the membrane potential reaches the threshold and ${\displaystyle \beta }$ is a sharpness parameter. For ${\displaystyle \beta \to \infty }$ the threshold becomes sharp and spike firing occurs deterministically at the moment when the membrane potential hits the threshold from below. The sharpness value found in experiments is ${\displaystyle 1/\beta \approx 4mV}$ which means that neuronal firing becomes non-negligible as soon the membrane potential is a few mV below the formal firing threshold.

The escape rate process via a soft threshold is reviewed in Chapter 9 of the textbook Neuronal Dynamics.

For models in discrete time, a spike is generated with probability

${\displaystyle P_{F}(t_{n})=F[V(t_{n})-V_{th}]}$

that depends on the momentary difference between the membrane voltage V at time ${\displaystyle t_{n}}$ and the threshold ${\displaystyle V_{th}}$. The function F is often taken as a standard sigmoidal ${\displaystyle F(x)=0.5[1+\tanh(\gamma x)]}$ with steepness parameter ${\displaystyle \gamma }$, similar to the update dynamics in artificial neural networks. But the functional form of F can also be derived from the stochastic intensity ${\displaystyle f}$ in continuous time introduced above as ${\displaystyle F(y_{n})\approx 1-\exp[y_{n}\Delta t]}$ where ${\displaystyle y_{n}=V(t_{n})-V_{th}}$ is the distance to threshold.

Integrate-and-fire models with output noise can be used to predict the PSTH of real neurons under arbitrary time-dependent input. For non-adaptive integrate-and-fire neurons, the interval distribution under constant stimulation can be calculated from stationary renewal theory. 

Spike response model (SRM)

main article: Spike response model

The spike response model (SRM) is a general linear model for the subthreshold membrane voltage combined with a nonlinear output noise process for spike generation. The membrane voltage V(t) at time t is

${\displaystyle V(t)=\sum _{f}\eta (t-t^{f})+\int _{0}^{\infty }\kappa (s)I(t-s)\,ds+V_{\mathrm {rest} }}$

where t^f is the firing time of spike number f of the neuron, V_rest is the resting voltage in the absence of input, I(t-s) is the input current at time t-s and ${\displaystyle \kappa (s)}$ is a linear filter (also called kernel) that describes the contribution of an input current pulse at time t-s to the voltage at time t. The contributions to the voltage caused by a spike at time ${\displaystyle t^{f}}$ are described by the refractory kernel ${\displaystyle \eta (t-t^{f})}$. In particular, ${\displaystyle \eta (t-t^{f})}$ describes the reset after the spike and the time course of the spike-afterpotential following a spike. It therefore expresses the consequences of refractoriness and adaptation. The voltage V(t) can be interpreted as the result of an integration of the differential equation of a leaky integrate-and-fire model coupled to an arbitrary number of spike-triggered adaptation variables.

Spike firing is stochastic and happens with a time-dependent stochastic intensity (instantaneous rate)

${\displaystyle f(V-\vartheta (t))={\frac {1}{\tau _{0}}}\exp[\beta (V-\vartheta (t)]}$

with parameters ${\displaystyle \tau _{0}}$ and ${\displaystyle \beta }$ and a dynamic threshold ${\displaystyle \vartheta (t)}$ given by

${\displaystyle \vartheta (t)=\vartheta _{0}+\sum _{f}\theta _{1}(t-t^{f})}$

Here ${\displaystyle \vartheta _{0}}$ is the firing threshold of an inactive neuron and ${\displaystyle \theta _{1}(t-t^{f})}$ describes the increase of the threshold after a spike at time ${\displaystyle t^{f}}$. In case of a fixed threshold, one sets ${\displaystyle \theta _{1}(t-t^{f})}$=0. For ${\displaystyle \beta \to \infty }$ the threshold process is deterministic.

The time course of the filters ${\displaystyle \eta ,\kappa ,\theta _{1}}$ that characterize the spike response model can be directly extracted from experimental data. With optimized parameters the SRM describes the time course of the subthreshold membrane voltage for time-dependent input with a precision of 2mV and can predict the timing of most output spikes with a precision of 4ms. The SRM is closely related to linear-nonlinear-Poisson cascade models (also called Generalized Linear Model). The estimation of parameters of probabilistic neuron models such as the SRM using methods developed for Generalized Linear Models is discussed in Chapter 10 of the textbook Neuronal Dynamics.

Spike arrival causes postsynaptic potentials (red lines) which are summed. If the total voltage V reaches a threshold (dashed blue line) a spike is initiated (green) which also includes a spike-afterpotential. The threshold increases after each spike. Postsynaptic potentials are the response to incoming spikes while the spike-afterpotential is the response to outgoing spikes.

The name spike response model arises because in a network, the input current for neuron i is generated by the spikes of other neurons so that in the case of a network the voltage equation becomes

${\displaystyle V_{i}(t)=\sum _{f}\eta _{i}(t-t_{i}^{f})+\sum _{j=1}^{N}w_{ij}\sum _{f'}\varepsilon _{ij}(t-t_{j}^{f'})+V_{\mathrm {rest} }}$

where ${\displaystyle t_{j}^{f'}}$ are the firing times of neuron j (i.e., its spike train) , and ${\displaystyle \eta _{i}(t-t_{i}^{f})}$ describes the time course of the spike and the spike after-potential for neuron i, ${\displaystyle w_{ij}}$ and ${\displaystyle \varepsilon _{ij}(t-t_{j}^{f'})}$ describe the amplitude and time course of an excitatory or inhibitory postsynaptic potential (PSP) caused by the spike ${\displaystyle t_{j}^{f'}}$ of the presynaptic neuron j. The time course ${\displaystyle \varepsilon _{ij}(s)}$ of the PSP results from the convolution of the postsynaptic current ${\displaystyle I(t)}$ caused by the arrival of a presynaptic spike from neuron j with the membrane filter ${\displaystyle \kappa (s)}$.

SRM0

The SRM0 is a stochastic neuron model related to time-dependent nonlinear renewal theory and a simplification of the Spike Renose Model (SRM). The main difference to the voltage equation of the SRM introduced above is that in the term containing the refractory kernel ${\displaystyle \eta (s)}$ there is no summation sign over past spikes: only the most recent spike (denoted as the time ${\displaystyle {\hat {t}}}$) matters. Another difference is that the threshold is constant. The model SRM0 can be formulated in discrete or continuous time. For example, in continuous time, the single-neuron equation is

${\displaystyle V(t)=\eta (t-{\hat {t}})+\int _{0}^{\infty }\kappa (s)I(t-s)\,ds+V_{\mathrm {rest} }}$

and the network equations of the SRM0 are

${\displaystyle V_{i}(t\mid {\hat {t}}_{i})=\eta _{i}(t-{\hat {t}}_{i})+\sum _{j}w_{ij}\sum _{f}\varepsilon _{ij}(t-{\hat {t}}_{i},t-t^{f})+V_{\mathrm {rest} }}$

where ${\displaystyle {\hat {t}}_{i}}$ is the last firing time neuron i. Note that the time course of the postsynaptic potential ${\displaystyle \varepsilon _{ij}}$ is also allowed to depend on the time since the last spike of neuron i so as to describe a change in membrane conductance during refractoriness. The instantaneous firing rate (stochastic intensity) is

${\displaystyle f(V-\vartheta )={\frac {1}{\tau _{0}}}\exp[\beta (V-V_{th})]}$

where ${\displaystyle V_{th}}$ is a fixed firing threshold. Thus spike firing of neuron i depends only on its input and the time since neuron i has fired its last spike.

With the SRM0, the interspike-interval distribution for constant input can be mathematically linked to the shape of the refractory kernel ${\displaystyle \eta }$ . Moreover the stationary frequency-current relation can be calculated from the escape rate in combination with the refractory kernel ${\displaystyle \eta }$. With an appropriate choice of the kernels, the SRM0 approximates the dynamics of the Hodgkin-Huxley model to a high degree of accuracy. Moreover, the PSTH response to arbitrary time-dependent input can be predicted.

Galves–Löcherbach model

3D visualization of the Galves–Löcherbach model for biological neural nets. This visualization is set for 4,000 neurons (4 layers with one population of inhibitory neurons and one population of excitatory neurons each) at 180 intervals of time.

 

Main article: Galves–Löcherbach model

The Galves–Löcherbach model is a stochastic neuron model closely related to the spike response model SRM0  and to the leaky integrate-and-fire model. It is inherently stochastic and, just like the SRM0 linked to time-dependent nonlinear renewal theory. Given the model specifications, the probability that a given neuron ${\displaystyle i}$ spikes in a time period ${\displaystyle t}$ may be described by

${\displaystyle \mathop {\mathrm {Prob} } (X_{t}(i)=1\mid {\mathcal {F}}_{t-1})=\varphi _{i}{\Biggl (}\sum _{j\in I}W_{j\rightarrow i}\sum _{s=L_{t}^{i}}^{t-1}g_{j}(t-s)X_{s}(j),~~~t-L_{t}^{i}{\Biggl )},}$

where ${\displaystyle W_{j\rightarrow i}}$ is a synaptic weight, describing the influence of neuron ${\displaystyle j}$ on neuron ${\displaystyle i}$, ${\displaystyle g_{j}}$ expresses the leak, and ${\displaystyle L_{t}^{i}}$ provides the spiking history of neuron ${\displaystyle i}$ before ${\displaystyle t}$, according to

${\displaystyle L_{t}^{i}=\sup\{s<t:X_{s}(i)=1\}.}$

Importantly, the spike probability of neuron i depends only on its spike input (filtered with a kernel ${\displaystyle g_{j}}$ and weighted with a factor ${\displaystyle W_{j\to i}}$) and the timing of its most recent output spike (summarized by ${\displaystyle t-L_{t}^{i}}$).

Didactic toy models of membrane voltage

The models in this category are highly simplified toy models that qualitatively describe the membrane voltage as a function of input. They are mainly used for didactic reasons in teaching but are not considered valid neuron models for large-scale simulations or data fitting.

FitzHugh–Nagumo

Main article: FitzHugh–Nagumo model

Sweeping simplifications to Hodgkin–Huxley were introduced by FitzHugh and Nagumo in 1961 and 1962. Seeking to describe "regenerative self-excitation" by a nonlinear positive-feedback membrane voltage and recovery by a linear negative-feedback gate voltage, they developed the model described by

${\displaystyle {\begin{array}{rcl}{\dfrac {dV}{dt}}&=&V-V^{3}/3-w+I_{\mathrm {ext} }\\\tau {\dfrac {dw}{dt}}&=&V-a-bw\end{array}}}$

where we again have a membrane-like voltage and input current with a slower general gate voltage w and experimentally-determined parameters a = -0.7, b = 0.8, τ = 1/0.08. Although not clearly derivable from biology, the model allows for a simplified, immediately available dynamic, without being a trivial simplification. The experimental support is weak, but the model is useful as a didactic tool to introduce dynamics of spike generation through phase plane analysis. See Chapter 7 in the textbook Methods of Neuronal Modeling.

Morris–Lecar

Main article: Morris–Lecar model

In 1981 Morris and Lecar combined the Hodgkin–Huxley and FitzHugh–Nagumo models into a voltage-gated calcium channel model with a delayed-rectifier potassium channel, represented by

${\displaystyle {\begin{aligned}&C{\frac {dV}{dt}}&=&-I_{\mathrm {ion} }(V,w)+I\\[6pt]&{\frac {dw}{dt}}&=&\varphi \cdot {\frac {w_{\infty }-w}{\tau _{w}}}\end{aligned}}}$

where ${\displaystyle I_{\mathrm {ion} }(V,w)={\bar {g}}_{\mathrm {Ca} }m_{\infty }\cdot (V-V_{\mathrm {Ca} })+{\bar {g}}_{\mathrm {K} }w\cdot (V-V_{\mathrm {K} })+{\bar {g}}_{\mathrm {L} }\cdot (V-V_{\mathrm {L} })}$. The experimental support of the model is weak, but the model is useful as a didactic tool to introduce dynamics of spike generation through phase plane analysis. See Chapter 7 in the textbook Methods of Neuronal Modeling.

A two-dimensional neuron model very similar to the Morris-Lecar model can be derived step-by-step starting from the Hodgkin-Huxley model. See Chapter 4.2 in the textbook Neuronal Dynamics.

Hindmarsh–Rose

Main article: Hindmarsh–Rose model

Building upon the FitzHugh–Nagumo model, Hindmarsh and Rose proposed in 1984 a model of neuronal activity described by three coupled first-order differential equations:

${\displaystyle {\begin{aligned}&{\frac {dx}{dt}}&=&y+3x^{2}-x^{3}-z+I\\[6pt]&{\frac {dy}{dt}}&=&1-5x^{2}-y\\[6pt]&{\frac {dz}{dt}}&=&r\cdot (4(x+{\tfrac {8}{5}})-z)\end{aligned}}}$

with r² = x² + y² + z², and r ≈ 10⁻² so that the z variable only changes very slowly. This extra mathematical complexity allows a great variety of dynamic behaviors for the membrane potential, described by the x variable of the model, which include chaotic dynamics. This makes the Hindmarsh–Rose neuron model very useful, because being still simple, allows a good qualitative description of the many different firing patterns of the action potential, in particular bursting, observed in experiments. Nevertheless, it remains a toy model and has not been fitted to experimental data. It is widely used as a reference model for bursting dynamics.

Theta model and quadratic integrate-and-fire.

The theta model, or Ermentrout–Kopell canonical Type I model, is mathematically equivalent to the quadratic integrate-and-fire model which in turn is an approximation to the exponential integrate-and-fire model and the Hodgkin-Huxley model. It is called a canonical model because it is one of the generic models for constant input close to the bifurcation point, which means close to the transition from silent to repetitive firing.

The standard formulation of the theta model is

${\displaystyle {\frac {d\theta (t)}{dt}}=(I-I_{0})[1+\cos(\theta )]+[1-\cos(\theta )]}$

The equation for the quadratic integrate-and-fire model is (see Chapter 5.3 in the textbook Neuronal Dynamics)

${\displaystyle \tau _{\mathrm {m} }{\frac {dV_{\mathrm {m} }(t)}{dt}}=(I-I_{0})R+[V_{\mathrm {m} }(t)-E_{\mathrm {m} }][V_{\mathrm {m} }(t)-V_{\mathrm {T} }]}$

The equivalence of theta model and quadratic integrate-and-fire is for example reviewed in Chapter 4.1.2.2 of spiking neuron models.

For input I(t) that changes over time or is far away from the bifurcation point, it is preferable to work with the exponential integrate-and-fire model (if one wants the stay in the class of one-dimensional neuron models), because real neurons exhibit the nonlinearity of the exponential integrate-and-fire model.

Sensory input-stimulus encoding neuron models

The models in this category were derived following experiments involving natural stimulation such as light, sound, touch, or odor. In these experiments, the spike pattern resulting from each stimulus presentation varies from trial to trial, but the averaged response from several trials often converges to a clear pattern. Consequently, the models in this category generate a probabilistic relationship between the input stimulus to spike occurrences. Importantly, the recorded neurons are often located several processing steps after the sensory neurons, so that these models summarize the effects of the sequence of processing steps in a compact form

The non-homogeneous Poisson process model (Siebert)

Siebert modeled the neuron spike firing pattern using a non-homogeneous Poisson process model, following experiments involving the auditory system. According to Siebert, the probability of a spiking event at the time interval ${\displaystyle [t,t+\Delta _{t}]}$ is proportional to a non negative function ${\displaystyle g[s(t)]}$, where ${\displaystyle s(t)}$ is the raw stimulus.:

${\displaystyle P_{\text{spike}}(t\in [t',t'+\Delta _{t}])=\Delta _{t}\cdot g[s(t)]}$

Siebert considered several functions as ${\displaystyle g[s(t)]}$, including ${\displaystyle g[s(t)]\propto s^{2}(t)}$ for low stimulus intensities.

The main advantage of Siebert's model is its simplicity. The shortcomings of the model is its inability to reflect properly the following phenomena:

• The transient enhancement of the neuronal firing activity in response to a step stimulus.
• The saturation of the firing rate.
• The values of inter-spike-interval-histogram at short intervals values (close to zero).

These shortcoming are addressed by the age-dependent point process model and the two-state Markov Model.

Refractoriness and age-dependent point process model

Berry and Meister studied neuronal refractoriness using a stochastic model that predicts spikes as a product of two terms, a function f(s(t)) that depends on the time-dependent stimulus s(t) and one a recovery function ${\displaystyle w(t-{\hat {t}})}$ that depends on the time since the last spike

${\displaystyle \rho (t)=f(s(t))w(t-{\hat {t}})}$

The model is also called an inhomogeneous Markov interval (IMI) process. Similar models have been used for many years in auditory neuroscience. Since the model keeps memory of the last spike time it is non-Poisson and falls in the class of time-dependent renewal models. It is closely related to the model SRM0 with exponential escape rate. Importantly, it is possible to fit parameters of the age-dependent point process model so as to describe not just the PSTH response, but also the interspike-interval statistics.

Linear-nonlinear Poisson cascade model and GLM

Main article: Linear-nonlinear-Poisson cascade model

The linear-nonlinear-Poisson cascade model is a cascade of a linear filtering process followed by a nonlinear spike generation step. In the case that output spikes feed back, via a linear filtering process, we arrive at a model that is known in the neurosciences as Generalized Linear Model (GLM). The GLM is mathematically equivalent to the spike response model SRM) with escape noise; but whereas in the SRM the internal variables are interpreted as the membrane potential and the firing threshold, in the GLM the internal variables are abstract quantities that summarizes the net effect of input (and recent output spikes) before spikes are generated in the final step.

The two-state Markov model (Nossenson & Messer)

The spiking neuron model by Nossenson & Messer produces the probability of the neuron to fire a spike as a function of either an external or pharmacological stimulus. The model consists of a cascade of a receptor layer model and a spiking neuron model, as shown in Fig 4. The connection between the external stimulus to the spiking probability is made in two steps: First, a receptor cell model translates the raw external stimulus to neurotransmitter concentration, then, a spiking neuron model connects between neurotransmitter concentration to the firing rate (spiking probability). Thus, the spiking neuron model by itself depends on neurotransmitter concentration at the input stage.

Fig 4: High level block diagram of the receptor layer and neuron model by Nossenson & Messer.

 

Fig 5. The prediction for the firing rate in response to a pulse stimulus as given by the model by Nossenson & Messer.

An important feature of this model is the prediction for neurons firing rate pattern which captures, using a low number of free parameters, the characteristic edge emphasized response of neurons to a stimulus pulse, as shown in Fig. 5. The firing rate is identified both as a normalized probability for neural spike firing, and as a quantity proportional to the current of neurotransmitters released by the cell. The expression for the firing rate takes the following form:

${\displaystyle R_{\text{fire}}(t)={\frac {P_{\text{spike}}(t;\Delta _{t})}{\Delta _{t}}}=[y(t)+R_{0}]\cdot P_{0}(t)}$

where,

• P0 is the probability of the neuron to be "armed" and ready to fire. It is given by the following differential equation:

${\displaystyle {\dot {P}}_{0}=-[y(t)+R_{0}+R_{1}]\cdot P_{0}(t)+R_{1}}$

P0 could be generally calculated recursively using Euler method, but in the case of a pulse of stimulus it yields a simple closed form expression.

• y(t) is the input of the model and is interpreted as the neurotransmitter concentration on the cell surrounding (in most cases glutamate). For an external stimulus it can be estimated through the receptor layer model:

${\displaystyle y(t)\simeq g_{\text{gain}}\cdot \langle s^{2}(t)\rangle ,}$

with ${\displaystyle \langle s^{2}(t)\rangle }$ being short temporal average of stimulus power (given in Watt or other energy per time unit).

• R₀ corresponds to the intrinsic spontaneous firing rate of the neuron.
• R₁ is the recovery rate of the neuron from the refractory state.

Other predictions by this model include:

1) The averaged evoked response potential (ERP) due to the population of many neurons in unfiltered measurements resembles the firing rate.

2) The voltage variance of activity due to multiple neuron activity resembles the firing rate (also known as Multi-Unit-Activity power or MUA).

3) The inter-spike-interval probability distribution takes the form a gamma-distribution like function.

Experimental evidence supporting the model by Nossenson & Messer

Property of the Model by Nossenson & Messer	Description of experimental evidence
The shape of the firing rate in response to an auditory stimulus pulse	The Firing Rate has the same shape of Fig 5.
The shape of the firing rate in response to a visual stimulus pulse	The Firing Rate has the same shape of Fig 5.
The shape of the firing rate in response to an olfactory stimulus pulse	The Firing Rate has the same shape of Fig 5.
The shape of the firing rate in response to a somato-sensory stimulus	The Firing Rate has the same shape of Fig 5.
The change in firing rate in response to neurotransmitter application (mostly glutamate)	Firing Rate change in response to neurotransmitter application (Glutamate)
Square dependence between an auditory stimulus pressure and the firing rate	Square Dependence between Auditory Stimulus pressure and the Firing Rate (- Linear dependence in pressure square (power)).
Square dependence between visual stimulus electric field (volts) and the firing rate	Square dependence between visual stimulus electric field (volts) - Linear Dependence between Visual Stimulus Power and the Firing Rate.
The shape of the Inter-Spike-Interval Statistics (ISI)	ISI shape resembles the gamma-function-like
The ERP resembles the firing rate in unfiltered measurements	The shape of the averaged evoked response potential in response to stimulus resembles the firing rate (Fig. 5).
MUA power resembles the firing rate	The shape of the empirical variance of extra-cellular measurements in response to stimulus pulse resembles the firing rate (Fig. 5).

Pharmacological input stimulus neuron models

The models in this category produce predictions for experiments involving pharmacological stimulation.

Synaptic transmission (Koch & Segev)

See also: Neurotransmission

According to the model by Koch and Segev, the response of a neuron to individual neurotransmitters can be modeled as an extension of the classical Hodgkin–Huxley model with both standard and nonstandard kinetic currents. Four neurotransmitters primarily have influence in the CNS. AMPA/kainate receptors are fast excitatory mediators while NMDA receptors mediate considerably slower currents. Fast inhibitory currents go through GABA_A receptors, while GABA_B receptors mediate by secondary G-protein-activated potassium channels. This range of mediation produces the following current dynamics:

• ${\displaystyle I_{\mathrm {AMPA} }(t,V)={\bar {g}}_{\mathrm {AMPA} }\cdot [O]\cdot (V(t)-E_{\mathrm {AMPA} })}$
• ${\displaystyle I_{\mathrm {NMDA} }(t,V)={\bar {g}}_{\mathrm {NMDA} }\cdot B(V)\cdot [O]\cdot (V(t)-E_{\mathrm {NMDA} })}$
• ${\displaystyle I_{\mathrm {GABA_{A}} }(t,V)={\bar {g}}_{\mathrm {GABA_{A}} }\cdot ([O_{1}]+[O_{2}])\cdot (V(t)-E_{\mathrm {Cl} })}$
• ${\displaystyle I_{\mathrm {GABA_{B}} }(t,V)={\bar {g}}_{\mathrm {GABA_{B}} }\cdot {\tfrac {[G]^{n}}{[G]^{n}+K_{\mathrm {d} }}}\cdot (V(t)-E_{\mathrm {K} })}$

where ḡ is the maximal conductance (around 1S) and E is the equilibrium potential of the given ion or transmitter (AMDA, NMDA, Cl, or K), while [O] describes the fraction of receptors that are open. For NMDA, there is a significant effect of magnesium block that depends sigmoidally on the concentration of intracellular magnesium by B(V). For GABA_B, [G] is the concentration of the G-protein, and K_d describes the dissociation of G in binding to the potassium gates.

The dynamics of this more complicated model have been well-studied experimentally and produce important results in terms of very quick synaptic potentiation and depression, that is, fast, short-term learning.

The stochastic model by Nossenson and Messer translates neurotransmitter concentration at the input stage to the probability of releasing neurotransmitter at the output stage. For a more detailed description of this model, see the Two state Markov model section above.

HTM neuron model

The HTM neuron model was developed by Jeff Hawkins and researchers at Numenta and is based on a theory called Hierarchical Temporal Memory, originally described in the book On Intelligence. It is based on neuroscience and the physiology and interaction of pyramidal neurons in the neocortex of the human brain.

Comparing the artificial neural network (A), the biological neuron (B), and the HTM neuron (C).

 

Artificial Neural Network (ANN)	Neocortical Pyramidal Neuron (Biological Neuron)	HTM Model Neuron
- Few synapses - No dendrites - Sum input x weights - Learns by modifying weights of synapses	- Thousands of synapses on the dendrites - Active dendrites: cell recognizes hundreds of unique patterns - Co-activation of a set of synapses on a dendritic segment causes an NMDA spike and depolarization at the soma - Sources of input to the cell: Feedforward inputs which form synapses proximal to the soma and directly lead to action potentials NMDA spikes generated in the more distal basal Apical dendrites that depolarize the soma (usually not sufficient enough to generate a somatic action potential) - Learns by growing new synapses	- Inspired by the pyramidal cells in neocortex layers 2/3 and 5 - Thousands of synapses - Active dendrites: cell recognizes hundreds of unique patterns - Models dendrites and NMDA spikes with each array of coincident detectors having a set of synapses - Learns by modeling growth of new synapses

Applications

Main article: Brain–computer interface

Spiking Neuron Models are used in a variety of applications that need encoding into or decoding from neuronal spike trains in the context of neuroprosthesis and brain-computer interfaces such as retinal prosthesis: or artificial limb control and sensation. Applications are not part of this article; for more information on this topic please refer to the main article.

Relation between artificial and biological neuron models

The most basic model of a neuron consists of an input with some synaptic weight vector and an activation function or transfer function inside the neuron determining output. This is the basic structure used for artificial neurons, which in a neural network often looks like

${\displaystyle y_{i}=\varphi \left(\sum _{j}w_{ij}x_{j}\right)}$

where y_i is the output of the i th neuron, x_j is the jth input neuron signal, w_ij is the synaptic weight (or strength of connection) between the neurons i and j, and φ is the activation function. While this model has seen success in machine-learning applications, it is a poor model for real (biological) neurons, because it lacks time-dependence in input and output.

When an input is switched on at a time t and kept constant thereafter, biological neurons emit a spike train. Importantly this spike train is not regular but exhibits a temporal structure characterized by adaptation, bursting, or initial bursting followed by regular spiking. Generalized integrate-and-fire model such as the Adaptive Exponential Integrate-and-Fire model, the spike response model, or the (linear) adaptive integrate-and-fire model are able to capture these neuronal firing patterns.

Moreover, neuronal input in the brain is time-dependent. Time-dependent input is transformed by complex linear and nonlinear filters into a spike train in the output. Again, the spike response model or the adaptive integrate-and-fire model enable to predict the spike train in the output for arbitrary time-dependent input, whereas an artificial neuron or a simple leaky integrate-and-fire does not.

If we take the Hodkgin-Huxley model as a starting point, generalized integrate-and-fire models can be derived systematically in a step-by-step simplification procedure. This has been shown explicitly for the exponential integrate-and-fire model and the spike response model.

In the case of modelling a biological neuron, physical analogues are used in place of abstractions such as "weight" and "transfer function". A neuron is filled and surrounded with water containing ions, which carry electric charge. The neuron is bound by an insulating cell membrane and can maintain a concentration of charged ions on either side that determines a capacitance C_m. The firing of a neuron involves the movement of ions into the cell that occurs when neurotransmitters cause ion channels on the cell membrane to open. We describe this by a physical time-dependent current I(t). With this comes a change in voltage, or the electrical potential energy difference between the cell and its surroundings, which is observed to sometimes result in a voltage spike called an action potential which travels the length of the cell and triggers the release of further neurotransmitters. The voltage, then, is the quantity of interest and is given by V_m(t).

If the input current is constant, most neurons emit after some time of adaptation or initial bursting a regular spike train. The frequency of regular firing in response to a constant current I is described by the frequency-current relation which corresponds to the transfer function ${\displaystyle \varphi }$ of artificial neural networks. Similarly, for all spiking neuron models the transfer function ${\displaystyle \varphi }$ can be calculated numerically (or analytically).

Cable theory and compartmental models

See also: Cable theory

All of the above deterministic models are point-neuron models because they do not consider the spatial structure of a neuron. However, the dendrite contributes to transforming input into output. Point neuron models are valid description in three cases. (i) If input current is directly injected into the soma. (ii) If synaptic input arrives predominantly at or close to the soma (closeness is defined by a length scale ${\displaystyle \lambda }$ introduced below. (iii) If synapse arrive anywhere on the dendrite, but the dendrite is completely linear. In the last case the cable acts as a linear filter; these linear filter properties can be included in the formulation of generalized integrate-and-fire models such as the spike response model.

The filter properties can be calculate from a cable equation.

Let us consider a cell membrane in the form a cylindrical cable. The position on the cable is denoted by x and the voltage across the cell membrane by V. The cable is characterized by a longitudinal resistance ${\displaystyle r_{l}}$ per unit length and a membrane resistance ${\displaystyle r_{m}}$ . If everything is linear, the voltage changes as a function of time

${\displaystyle {\frac {r_{m}}{r_{l}}}{\frac {\partial ^{2}V}{\partial x^{2}}}=c_{m}r_{m}{\frac {\partial V}{\partial t}}+V}$

(19)

We introduce a length scale ${\displaystyle \lambda ^{2}={r_{m}}/{r_{l}}}$ on the left side and time constant ${\displaystyle \tau =c_{m}r_{m}}$ on the right side. The cable equation can now be written in its perhaps best known form:

${\displaystyle \lambda ^{2}{\frac {\partial ^{2}V}{\partial x^{2}}}=\tau {\frac {\partial V}{\partial t}}+V}$

(20)

The above cable equation is valid for a single cylindrical cable.

Linear cable theory describes the dendritic arbor of a neuron as a cylindrical structure undergoing a regular pattern of bifurcation, like branches in a tree. For a single cylinder or an entire tree, the static input conductance at the base (where the tree meets the cell body, or any such boundary) is defined as

${\displaystyle G_{in}={\frac {G_{\infty }\tanh(L)+G_{L}}{1+(G_{L}/G_{\infty })\tanh(L)}}}$,

where L is the electrotonic length of the cylinder which depends on its length, diameter, and resistance. A simple recursive algorithm scales linearly with the number of branches and can be used to calculate the effective conductance of the tree. This is given by

${\displaystyle \,\!G_{D}=G_{m}A_{D}\tanh(L_{D})/L_{D}}$

where A_D = πld is the total surface area of the tree of total length l, and L_D is its total electrotonic length. For an entire neuron in which the cell body conductance is G_S and the membrane conductance per unit area is G_md = G_m / A, we find the total neuron conductance G_N for n dendrite trees by adding up all tree and soma conductances, given by

${\displaystyle G_{N}=G_{S}+\sum _{j=1}^{n}A_{D_{j}}F_{dga_{j}},}$

where we can find the general correction factor F_dga experimentally by noting G_D = G_mdA_DF_dga.

The linear cable model makes a number of simplifications to give closed analytic results, namely that the dendritic arbor must branch in diminishing pairs in a fixed pattern and that dendrites are linear. A compartmental model allows for any desired tree topology with arbitrary branches and lengths, as well as arbitrary nonlinearities. It is essentially a discretized computational implementation of nonlinear dendrites.

Each individual piece, or compartment, of a dendrite is modeled by a straight cylinder of arbitrary length l and diameter d which connects with fixed resistance to any number of branching cylinders. We define the conductance ratio of the ith cylinder as B_i = G_i / G_∞, where ${\displaystyle G_{\infty }={\tfrac {\pi d^{3/2}}{2{\sqrt {R_{i}R_{m}}}}}}$ and R_i is the resistance between the current compartment and the next. We obtain a series of equations for conductance ratios in and out of a compartment by making corrections to the normal dynamic B_out,i = B_in,i+1, as

• ${\displaystyle B_{\mathrm {out} ,i}={\frac {B_{\mathrm {in} ,i+1}(d_{i+1}/d_{i})^{3/2}}{\sqrt {R_{\mathrm {m} ,i+1}/R_{\mathrm {m} ,i}}}}}$
• ${\displaystyle B_{\mathrm {in} ,i}={\frac {B_{\mathrm {out} ,i}+\tanh X_{i}}{1+B_{\mathrm {out} ,i}\tanh X_{i}}}}$
• ${\displaystyle B_{\mathrm {out,par} }={\frac {B_{\mathrm {in,dau1} }(d_{\mathrm {dau1} }/d_{\mathrm {par} })^{3/2}}{\sqrt {R_{\mathrm {m,dau1} }/R_{\mathrm {m,par} }}}}+{\frac {B_{\mathrm {in,dau2} }(d_{\mathrm {dau2} }/d_{\mathrm {par} })^{3/2}}{\sqrt {R_{\mathrm {m,dau2} }/R_{\mathrm {m,par} }}}}+\ldots }$

where the last equation deals with parents and daughters at branches, and ${\displaystyle X_{i}={\tfrac {l_{i}{\sqrt {4R_{i}}}}{\sqrt {d_{i}R_{m}}}}}$. We can iterate these equations through the tree until we get the point where the dendrites connect to the cell body (soma), where the conductance ratio is B_in,stem. Then our total neuron conductance for static input is given by

${\displaystyle G_{N}={\frac {A_{\mathrm {soma} }}{R_{\mathrm {m,soma} }}}+\sum _{j}B_{\mathrm {in,stem} ,j}G_{\infty ,j}.}$

Importantly, static input is a very special case. In biology inputs are time dependent. Moreover, dendrites are not always linear.

Compartmental models enable to include nonlinearities via ion channels positioned at arbitrary locations along the dendrites. For static inputs, it is sometimes possible to reduce the number of compartments (increase the computational speed) and yet retain the salient electrical characteristics.

See also: Multi-compartment model

Conjectures regarding the role of the neuron in the wider context of the brain principle of operation

The neurotransmitter-based energy detection scheme

The neurotransmitter-based energy detection scheme suggests that the neural tissue chemically executes a Radar-like detection procedure.

Fig. 6 The biological neural detection scheme as suggested by Nossenson et al.

As shown in Fig. 6, the key idea of the conjecture is to account neurotransmitter concentration, neurotransmitter generation and neurotransmitter removal rates as the important quantities in executing the detection task, while referring to the measured electrical potentials as a side effect that only in certain conditions coincide with the functional purpose of each step. The detection scheme is similar to a radar-like "energy detection" because it includes signal squaring, temporal summation and a threshold switch mechanism, just like the energy detector, but it also includes a unit that emphasizes stimulus edges and a variable memory length (variable memory). According to this conjecture, the physiological equivalent of the energy test statistics is neurotransmitter concentration, and the firing rate corresponds to neurotransmitter current. The advantage of this interpretation is that it leads to a unit consistent explanation which allows to bridge between electrophysiological measurements, biochemical measurements and psychophysical results.

The evidence reviewed in suggest the following association between functionality to histological classification:

1. Stimulus squaring is likely to be performed by receptor cells.
2. Stimulus edge emphasizing and signal transduction is performed by neurons.
3. Temporal accumulation of neurotransmitters is performed by glial cells. Short term neurotransmitter accumulation is likely to occur also in some types of neurons.
4. Logical switching is executed by glial cells, and it results from exceeding a threshold level of neurotransmitter concentration. This threshold crossing is also accompanied by a change in neurotransmitter leak rate.
5. Physical all-or-non movement switching is due to muscle cells and results from exceeding a certain neurotransmitter concentration threshold on muscle surroundings.

Note that although the electrophysiological signals in Fig.6 are often similar to the functional signal (signal power / neurotransmitter concentration / muscle force), there are some stages in which the electrical observation is different from the functional purpose of the corresponding step. In particular, Nossenson et al. suggested that glia threshold crossing has a completely different functional operation compared to the radiated electrophysiological signal, and that the latter might only be a side effect of glia break.

General comments regarding the modern perspective of scientific and engineering models

• The models above are still idealizations. Corrections must be made for the increased membrane surface area given by numerous dendritic spines, temperatures significantly hotter than room-temperature experimental data, and nonuniformity in the cell's internal structure. Certain observed effects do not fit into some of these models. For instance, the temperature cycling (with minimal net temperature increase) of the cell membrane during action potential propagation not compatible with models which rely on modeling the membrane as a resistance which must dissipate energy when current flows through it. The transient thickening of the cell membrane during action potential propagation is also not predicted by these models, nor is the changing capacitance and voltage spike that results from this thickening incorporated into these models. The action of some anesthetics such as inert gases is problematic for these models as well. New models, such as the soliton model attempt to explain these phenomena, but are less developed than older models and have yet to be widely applied.
• Modern views regarding of the role of the scientific model suggest that "All models are wrong but some are useful" (Box and Draper, 1987, Gribbin, 2009; Paninski et al., 2009).
• Recent conjecture suggests that each neuron might function as a collection of independent threshold units. It is suggested that a neuron could be anisotropically activated following the origin of its arriving signals to the membrane, via its dendritic trees. The spike waveform was also proposed to be dependent on the origin of the stimulus.

Louis Farrakhan

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Louis_Farrakhan 

 

Minister Louis Farrakhan
Farrakhan in November 2018
Born	Louis Eugene Walcott May 11, 1933 New York City, U.S.
Education	English High School of Boston, Winston-Salem State University
Occupation	Leader of the Nation of Islam Former calypso music singer
Predecessor	Warith Deen Mohammed
Spouse(s)	Khadijah Farrakhan ​ (m. 1953)​
Children	9; including Mustapha and Donna

Part of a series on the
Nation of Islam
Mosque Maryam in Chicago

Influencers

Leaders

Beliefs and theology

History

Publications

Subsidiaries

Offshoots and sects

Related organizations

Islam portal  Politics portal

Louis Farrakhan (/ˈfɑːrəkɑːn/; born Louis Eugene Walcott, May 11, 1933) is an American religious leader, black supremacist, anti-white conspiracy theorist, and former singer who heads the Nation of Islam (NOI). Prior to joining the NOI, he was a calypso music singer who went by the stage name Calypso Gene. Earlier in his career, he served as the minister of mosques in Boston and Harlem and was appointed National Representative of the Nation of Islam by former NOI leader Elijah Muhammad. He adopted the name Louis X, before being named Louis Farrakhan.

After Warith Deen Mohammed reorganized the original NOI into the orthodox Sunni Islamic group American Society of Muslims, Farrakhan began to rebuild the NOI as "Final Call". In 1981, he officially adopted the name "Nation of Islam", reviving the group and establishing its headquarters at Mosque Maryam. The Nation of Islam is an organization which the Southern Poverty Law Center (SPLC) describes as a hate group. Farrakhan’s antisemitic statements and views have been condemned by the SPLC, the Anti-Defamation League (ADL), and other monitoring organizations. According to the SPLC, the NOI promotes a "fundamentally anti-white theology" amounting to an "innate black superiority over whites". Farrakhan's views and remarks have also been called homophobic. He has disputed these assertions.

In October 1995, he organized and led the Million Man March in Washington, D.C. Due to health issues, he reduced his responsibilities with the NOI in 2007. However, Farrakhan has continued to deliver sermons and speak at NOI events. In 2015, he led the 20th Anniversary of the Million Man March: Justice or Else.

Farrakhan was banned from Facebook in 2019 along with other public figures considered to be extremists.

Early life and education

Farrakhan was born Louis Eugene Walcott on May 11, 1933, in The Bronx, New York City, the younger of two sons of Sarah Mae Manning (1900–1988) and Percival Clark, immigrants from the Anglo-Caribbean islands. His mother was born in Saint Kitts, while his father was Jamaican. The couple separated before their second son was born, and Farrakhan says he never knew his biological father.

In a 1996 interview with Henry Louis Gates Jr., he speculated that his father, "Gene", may have been Jewish.

After his stepfather died in 1936, the Walcott family moved to Boston, where they settled in the largely African-American neighborhood of Roxbury.

Walcott received his first violin at the age of five and by the time he was 12 years old, he had been on tour with the Boston College Orchestra. A year later, he participated in national competitions and won them. In 1946, he was one of the first black performers to appear on the Ted Mack Original Amateur Hour, where he also won an award. Walcott and his family were active members of the Episcopal St. Cyprian's Church in Roxbury.

Walcott attended the Boston Latin School, and later the English High School, from which he graduated. He completed three years at Winston-Salem Teachers College, where he had a track scholarship.

Louis and Khadijah Farrakhan

In 1953, Walcott married Betsy Ross (later known as Khadijah Farrakhan) while he was in college. Due to complications from his new wife's first pregnancy, Walcott dropped out after completing his junior year of college to devote time to his wife and their child. Farrakhan is the father of 9 children and grandfather of basketball player Mustapha Farrakhan Jr.

Career and activities (1953–1995)

In the 1950s, Walcott began his professional music career as a singer billed as "The Charmer". At this point, earning $500 a week, Walcott was touring the northeastern and midwestern United States, sometimes also using the nickname "Calypso Gene". In 1953–1954, preceding Harry Belafonte's success with his album Calypso (released in 1956), he recorded and released a dozen cheeky, funny tunes as "The Charmer" in a mixed mento/calypso style, including "Ugly Woman", "Stone Cold Man" and calypso standards like "Zombie Jamboree", "Hol 'Em Joe", "Mary Ann" and "Brown Skin Girl". Some were reissued: "Don’t Touch Me Nylon" has mild, explicit sexual lyrics as well as "Female Boxer", which contains some sexist overtones and "Is She Is, Or Is She Ain't" (inspired by Christine Jorgensen's sex change operation).

In February 1955, he was headlining a show in Chicago, Illinois, called Calypso Follies. There he first came in contact with the teachings of the Nation of Islam (NOI) through Rodney Smith, a friend and saxophonist from Boston. Walcott and his wife Betsy were invited to the Nation of Islam's annual Saviours' Day address by Elijah Muhammad. Prior to going to Saviours' Day, due to then-Minister Malcolm X's media presence, Walcott had never heard of Elijah Muhammad, and like many outside of the Nation of Islam, he thought that Malcolm X was the leader of the Nation of Islam.

In 1955, Walcott fulfilled the requirements to be a registered Muslim/registered believer/registered laborer. He memorized and recited verbatim the 10 questions and answers of the NOI's Student Enrollment. He then wrote a Saviour's Letter that must be sent to the NOI's headquarters in Chicago. The Saviour's Letter must be copied verbatim, and have the identical handwriting of the Nation of Islam's founder, Wallace Fard Muhammad.

After having the Saviour's Letter reviewed, and approved by the NOI's headquarters in Chicago in July 1955, Walcott received a letter of approval from the Nation of Islam acknowledging his official membership as a registered Muslim/registered believer/registered laborer in the NOI. As a result, he received his "X." The "X" was considered a placeholder, used to indicate that Nation of Islam members' original African family names had been lost. They acknowledged that European surnames were slave names, assigned by the slaveowners in order to mark their ownership. Members of the NOI used the "X" while they were waiting for their Islamic names, which some NOI members received later in their conversions.

Hence, Louis Walcott became Louis X. Elijah Muhammad then replaced his "X" with the "holy name" Farrakhan, which is a corruption of the Arabic word فرقان furqan, which means "The Criterion". On a very different tone from his calypso songs, he recorded two tunes as Louis X, criticizing racism in A White Man's Heaven Is a Black Man's Hell, a record album which was issued on Boston's A Moslem Sings label in 1960.

The summer after Farrakhan's conversion, Elijah Muhammad stated that all musicians in the NOI had to choose between music and the Nation of Islam.

After nine months of being a registered Muslim in the NOI and a member of Muhammad's Temple of Islam in Boston, where Malcolm X was the minister, the former calypso-singer turned Muslim became his assistant minister. Eventually he became the official minister after Elijah Muhammad transferred Malcolm X to Muhammad's Temple of Islam No. 7 on West 116th St. in Harlem, New York City. Louis X continued to be mentored by Malcolm X, until the latter's assassination in 1965.

The day that Malcolm X died in Harlem, Farrakhan happened to be in Newark, New Jersey on rotation, 45 minutes away from where Malcolm X was assassinated. After Malcolm X's death, Elijah Muhammad appointed Farrakhan to the two prominent positions that Malcolm held before being dismissed from the NOI. Farrakhan became the national spokesman/national representative of the NOI and was appointed minister of the influential Harlem Mosque (Temple), where he served until 1975.

Farrakhan made numerous incendiary statements about Malcolm X, contributing to what was called a "climate of vilification." Three men from a Newark, NOI mosque—Thomas Hagan, Muhammad Abdul Aziz (aka Norman 3X Butler) and Kahlil Islam (aka Thomas 15X Johnson)—were convicted of the killing and served prison sentences. Only Hagan ever admitted his role.

Leadership of the Nation of Islam

Warith Deen Mohammed, the seventh son of Elijah and Clara Muhammad, was declared the new leader of the Nation of Islam at the annual Saviours' Day Convention in February 1975, a day after his father died. He made substantial changes in the organization in the late 1970s, taking most of its members into a closer relationship with orthodox Islam, and renaming the group "World Community of Islam in the West", and eventually renaming it the American Society of Muslims, to indicate the apparent changes which had occurred in the group. He rejected the deification of the Nation of Islam's founder Wallace D. Fard as Allah in person, the Mahdi of the Holy Qur'an and the messiah of the Bible, welcomed white worshipers who were once considered devils and enemies in the NOI as equal brothers, sisters, and friends. At the beginning of these changes, Chief Min. Warith Deen Mohammed gave some Euro-Americans X's, and he extended efforts at inter-religious cooperation and outreach to Christians and Jews. He changed his position and title from Chief Minister Wallace Muhammad to Imam Warith Huddin Mohammad, and finally changed them to Imam Warith Al-Deen Mohammed.

Farrakhan joined Mohammed's movement and followed Imam Warith Al-Deen Mohammed, and eventually became a Sunni Imam under him for 3+1⁄2 years from 1975 to 1978. Imam Mohammed gave Imam Farrakhan the name Abdul-Haleem. In 1978, Imam Farrakhan distanced himself from Mohammed's movement. In a 1990 interview with Emerge magazine, Farrakhan said that he had become disillusioned with Mohammed's movement and decided to "quietly walk away" from it rather than cause a schism among its members. In 1978, Farrakhan and a small number of supporters decided to rebuild what they considered the original Nation of Islam upon the foundations established by Wallace Fard Muhammad, and Elijah Muhammad. This decision was made without public announcement.

In 1979, Farrakhan's group founded a weekly newspaper entitled The Final Call, which was intended to be similar to the original Muhammad Speaks newspaper that Malcolm X claimed to have started, Farrakhan had a weekly column in The Final Call. In 1981, Farrakhan and his supporters held their first Saviours' Day convention in Chicago, Illinois, and took back the name of the Nation of Islam. The event was similar to the earlier Nation's celebrations, last held in Chicago on February 26, 1975. At the convention's keynote address, Farrakhan announced his attempt to restore the Nation of Islam under Elijah Muhammad's teachings.

On October 24, 1989, at a press conference at the J.W. Marriott Hotel in Washington, DC, Minister Farrakhan described a vision which he had on September 17, 1985 in Tepoztlán, Mexico. In this 'Vision-like' experience he was carried up to "a Wheel, or what you call an unidentified flying object", as in the Bible's Book of Ezekiel. During this experience, he heard the voice of Elijah Muhammad, the leader of the Nation of Islam.

He said in the press conference that Elijah Muhammad "spoke in short cryptic sentences and as he spoke a scroll full of cursive writing rolled down in front of my eyes, but it was a projection of what was being written in my mind. As I attempted to read the cursive writing, which was in English, the scroll disappeared and the Honorable Elijah Muhammad began to speak to me." [Elijah Muhammad said], "President Reagan has met with the Joint Chiefs of Staff to plan a war. I want you to hold a press conference in Washington, D.C., and announce their plan and say to the world that you got the information from me, Elijah Muhammad, on the Wheel."

During that same press conference Farrakhan stated that he believed his "experience" was proven: "In 1987, in The New York Times' Sunday magazine and on the front page of The Atlanta Constitution, the truth of my vision was verified, for the headlines of The Atlanta Constitution read, 'President Reagan Planned War Against Libya.'" Farrakhan added "In the article which followed, the exact words that the Honorable Elijah Muhammad spoke to me on the Wheel were found; that the President had met with the Joint Chiefs of Staff and planned a war against Libya in the early part of September 1985."

Qubilah Shabazz, the daughter of Malcolm X and Betty Shabazz, was arrested on January 12, 1995 accused of conspiracy to assassinate Farrakhan in retaliation for the murder of her father, for which she believed he was responsible. According to Stanford University historian Clayborne Carson, "[her family] resented Farrakhan and had good reason to because he was one of those in the Nation responsible for the climate of vilification that resulted in Malcolm X's assassination". Some critics later alleged that the FBI had used paid informant Michael Fitzpatrick to frame Shabazz, who was four years old when her father was killed. Nearly four months later, on May 1, Shabazz accepted a plea agreement under which she maintained her innocence but accepted responsibility for her actions.

Farrakhan in 1997

Million Man March

That year in October, Farrakhan convened a broad coalition of what he and his supporters claimed was one million men in Washington, D.C., for the Million Man March. The count however fell far below the hoped-for numbers. The National Park Service estimated that approximately 440,000 were in attendance. Farrakhan threatened to sue the National Park Service because of the low estimate from the Park Police.

Farrakhan and other speakers called for black men to renew their commitments to their families and communities. In Farrakhan's 21⁄2 hours he quoted from spirituals as well as the Old and New Testaments and termed himself a prophet sent by God to show America its evil. The event was organized by many civil rights and religious organizations and drew men and their sons from across the United States of America. Many other distinguished African Americans addressed the throng, including: Maya Angelou; Rosa Parks; Martin Luther King III, Cornel West, Jesse Jackson and Benjamin Chavis. In 2005, together with other prominent African Americans such as the New Black Panther Party leader Malik Zulu Shabazz, the activist Al Sharpton, Addis Daniel and others, Farrakhan marked the 10th anniversary of the Million Man March by holding a second gathering, the Millions More Movement, October 14–17 in Washington D.C.

Views

Racism and black nationalism

The Anti-Defamation League classifies Farrakhan as a racist and the Southern Poverty Law Center considers the Nation of Islam (NOI) as a hate group and a black nationalist organization. According to the SPLC, the NOI's theology claims black superiority over whites. According to the NOI, whites were created 6,600 years ago as a "race of devils" by an evil scientist named Yakub, a story which originated with the founder of the NOI, Wallace D. Fard.

The split in the NOI into two factions after Eljiah Muhammad died in 1975, was caused in part because new leader Warith Mohammed wished to reject the Yakub myth, while national spokesman Farrakhan wanted to reaffirm it. At an event in Milwaukee in August 2015, Farrakhan said: "White people deserve to die, and they know, so they think it’s us coming to do it".

Antisemitism

Part of a series on
Antisemitism
Part of Jewish history and discrimination
History Timeline Reference

Definitions

Manifestations

Antisemitic canards

Antisemitic publications

Antisemitism on the Internet

Prominent figures

Persecution

Opposition

Category

Southern Poverty Law Center classifies Farrakhan as an "antisemite". He disputes this label. Farrakhan has made many comments that have been deemed antisemitic by the Anti-Defamation League. The Simon Wiesenthal Center included some of Farrakhan's comments on its list of the Top 10 antisemitic slurs in 2012.

"Gutter religion" remarks

In June 1984, after returning from a visit to Libya, Farrakhan delivered a sermon that was recorded by a Chicago Sun-Times reporter. A transcript from part of the sermon was published in The New York Times:

Toward the end of that portion of his speech that was recorded, Mr. Farrakhan said: "Now that nation called Israel never has had any peace in 40 years and she will never have any peace because there can be no peace structured on injustice, thievery, lying and deceit and using the name of God to shield your dirty religion under His holy and righteous name.

Farrakhan has repeatedly denied referring to Judaism as a "gutter religion" by explaining that he was instead referring to what he believed was the Israeli Government's use of Judaism as a political tool. In a June 18, 1997, letter to a former Wall Street Journal editor Jude Wanniski he stated:

Countless times over the years I have explained that I never referred to Judaism as a gutter religion, but, clearly referred to the machinations of those who hide behind the shield of Judaism while using unjust political means to achieve their objectives. This was distilled in the New York tabloids and other media saying, 'Farrakhan calls Judaism a gutter religion.'

As a Muslim, I revere Abraham, Moses, and all the Prophets whom Allah (God) sent to the children of Israel. I believe in the scriptures brought by these Prophets and the Laws of Allah (God) as expressed in the Torah. I would never refer to the Revealed Word of Allah (God)—the basis of Jewish Faith—as 'dirty' or 'gutter.' You know, Jude, as well as I, that the Revealed Word of Allah (God) comes as a Message from Allah (God) to purify us from our evil that has divided us and caused us to fall into the gutter.

Over the centuries, the evils of Christians, Jews and Muslims have dirtied their respective religions. True Faith in the laws and Teaching of Abraham, Jesus and Muhammad is not dirty, but, practices in the name of these religions can be unclean and can cause people to look upon the misrepresented religion as being unclean.

Adolf Hitler and the Holocaust

In response to Farrakhan's speech, Nathan Pearlmutter, then Chair of the Anti-Defamation League, referred to Farrakhan as the new "Black Hitler" and Village Voice journalist Nat Hentoff also characterized the NOI leader as a "Black Hitler" while he was a guest on a New York radio talk-show.

In response, Farrakhan announced during a March 11, 1984, speech which was broadcast on a Chicago radio station:

So I said to the members of the press, 'Why won't you go and look into what we are saying about the threats on Reverend Jackson's life?' Here the Jews don't like Farrakhan and so they call me 'Hitler'. Well that's a good name. Hitler was a very great man. He wasn't great for me as a Black man but he was a great German and he rose Germany up from the ashes of her defeat by the united force of all of Europe and America after the First World War. Yet Hitler took Germany from the ashes and rose her up and made her the greatest fighting machine of the twentieth century, brothers and sisters, and even though Europe and America had deciphered the code that Hitler was using to speak to his chiefs of staff, they still had trouble defeating Hitler even after knowing his plans in advance. Now I'm not proud of Hitler's evil toward Jewish people, but that's a matter of record. He rose Germany up from nothing. Well, in a sense you could say there is a similarity in that we are rising our people up from nothing, but don't compare me with your wicked killers.

At a later meeting of the Nation of Islam at Madison Square Garden in 1985, Farrakhan said of the Jews: "And don't you forget, when it's God who puts you in the ovens, it's forever!" He has also claimed that German Jews financed the Holocaust in a speech at the Mosque Maryam, Chicago in March 1995: "German Jews financed Hitler right here in America...International bankers financed Hitler and poor Jews died while big Jews were at the root of what you call the Holocaust". Almost three years later at a Saviors' Day gathering in the same city, he said: "The Jews have been so bad at politics they lost half their population in the Holocaust. They thought they could trust in Hitler, and they helped him get the Third Reich on the road."

Incidents and comments since 2002

On March 23, 2002, Farrakhan visited Kahal Kadosh Shaare Shalom in Kingston, Jamaica, which was his first visit to a synagogue, in an attempt to repair his relationship with the Jewish community. Farrakhan was accepted to speak at Shaare Shalom in the native country of his father, after being rejected to appear at American synagogues, many of whom had fear of sending the wrong signals to the Jewish community.

Farrakhan in Iran, 2018

Farrakhan made antisemitic comments during his May 16–17, 2013 visit to Detroit in which he accused President Obama of having “surrounded himself with Satan…members of the Jewish community". Jews, according to Farrakhan, "have mastered the civilization now, but they’ve mastered it in evil". In a weekly lecture series titled "The Time and What Must Be Done", which began during January 2013, he prophesied the downfall of the United States soon and said the country faced divine punishment if his warnings were rejected.

In March 2015, Farrakhan accused "Israelis and Zionist Jews" of being involved in the September 11 attacks. (In 2012 and 2017 speeches, he said the American government were behind 9/11.) In his Saviours' Day speech in February 2018, Farrakhan described "the powerful Jews" as his enemy and approvingly cited President Richard Nixon and the Reverend Billy Graham's derogatory comments about Jews "grip on the media", and claimed they are responsible for "all of this filth and degenerate behavior that Hollywood is putting out turning men into women and women into men".

A three-hour speech by Farrakhan on July 4, 2020 was carried by Revolt TV's YouTube channel, He claimed Jonathan Greenblatt, the head of the anti-bigotry nonprofit Anti-Defamation League, is Satan, and described Alan Dershowitz as "a skillful deceiver" and "Satan masquerading as a lawyer". Greenblatt responded in a tweet: "This is routine for Farrakhan—give him a platform, he never fails to espouse hatred." Farrakhan made the factually inaccurate claim that Jews are required by their religion to poison prophets and claimed Jews had "broken their covenant relationship with God" and were the "enemy of God". However, in his speech, Farrakhan also said: “If you really think I hate the Jewish people, you don’t know me at all,” adding “[I’ve never] uttered the words of death to the Jewish people.” As of July 15, 2020, Farrakhan's speech had been viewed more than 1.2 million times on YouTube.

Activities and statements since 2005

Hurricane Katrina

In comments in 2005, Farrakhan stated that there was a 25-foot (7.6 m) hole under one of the key levees that failed in New Orleans following Hurricane Katrina. He implied that the levee's destruction was a deliberate attempt to wipe out the population of the largely black sections within the city. Farrakhan later said that New Orleans Mayor Ray Nagin told him of the crater during a meeting in Dallas, Texas.

Farrakhan further claimed that the fact the levee broke the day after Hurricane Katrina is proof that the destruction of the levee was not a natural occurrence. Farrakhan has raised additional questions and has called for federal investigations into the source of the levee break. He also asserted that the hurricane was "God's way of punishing America for its warmongering and racism".

Experts including the Independent Levee Investigation Team (ILIT) from the University of California, Berkeley have countered his accusations. The report from the ILIT said "The findings of this panel are that the over-topping of the levees by flood waters, the often sub-standard materials used to shore up the levees, and the age of the levees contributed to these scour holes found at many of the sites of levee breaks after the hurricane."

Relations with Barack Obama

In 2008, Farrakhan publicly criticized the United States and supported then-Senator Barack Obama who was campaigning at the time to become the president of the United States of America. Farrakhan and Obama had met at least once before that time.

The Obama campaign quickly responded to convey his distance from the minister. "Senator Obama has been clear in his objections to Farrakhan's past pronouncements and has not solicited the minister's support," said Obama spokesman Bill Burton. Obama "rejected and denounced" Farrakhan's support during an NBC presidential candidate debate.

Following the 2008 presidential election, Farrakhan explained, during a BET television interview, that he was "careful" never to endorse Obama during his campaign. "I talked about him—but, in very beautiful and glowing terms, stopping short of endorsing him. And unfortunately, or fortunately, however we look at it, the media said I 'endorsed' him, so he renounced my so-called endorsement and support. But that didn't stop me from supporting him."

On May 28, 2011, Farrakhan, speaking at the American Clergy Leadership Conference, lambasted Obama over the wars in Iraq and Afghanistan and the intervention in Libya, calling him an "assassin" and a "murderer." "We voted for our brother Barack, a beautiful human being with a sweet heart," Farrakhan said, in a video that was widely shared on the Internet. "But he has turned into someone else," Farrakhan told the crowd. "Now he's an assassin."

Dianetics

A connection between the Church of Scientology and the Nation of Islam is reported to date from the late 1990s when Farrakhan was introduced to its teachings by the musician Isaac Hayes, who was the Church of Scientology's International spokesman for its World Literacy Crusade.

On May 8, 2010, Farrakhan publicly announced his embrace of Dianetics and has actively encouraged Nation of Islam members to undergo auditing from the Church. Although he has stressed that he is not a Scientologist, but only a believer in Dianetics and the theories related to it, the Church honored Farrakhan previously during its 2006 Ebony Awakening awards ceremony (which he did not attend). Farrakhan has also urged European Americans to join the Church of Scientology, stating in his 2011 Saviour's Day speech, "All white people should flock to [Scientology founder] L. Ron Hubbard." Reportedly, according to the SPLC, Hubbard was a racist who supported the apartheid regime in South Africa.

Since the announcement in 2010, the Nation of Islam has been hosting its own Dianetic courses and its own graduation ceremonies. At the third such ceremony, which was held on Saviours Day 2013, it was announced that nearly 8,500 members of the organisation had undergone Dianetic auditing. The Organisation announced it had graduated 1,055 auditors and had delivered 82,424 hours of auditing. The graduation ceremony was certified by the Church of Scientology, and the Nation of Islam members received official certification. The ceremony was attended by Shane Woodruff, vice-president of the Church of Scientology's Celebrity Centre International. He stated that "The unfolding story of the Nation of Islam and Dianetics is bold, it is determined and it is absolutely committed to restoring freedom and wiping hell from the face of this planet."

Praise for Donald Trump

During the 2016 Republican Party presidential primaries, Farrakhan praised Republican candidate Donald Trump as the only candidate "who has stood in front of the Jewish community and said 'I don’t want your money.'" While he declined to endorse Trump outright, he said of Trump "I like what I'm looking at." In 2018, Farrakhan again praised Trump for "destroying every enemy that was an enemy of our rise". He included the Department of Justice and the Federal Bureau of Investigation (FBI) in this group.

Conservative pundits Candace Owens and Glenn Beck both took note of Farrakhan's position, with Owens saying, while she did not "endorse Farrakhan’s views," it remained a "really big deal" that Farrakhan had "aligned himself with Trump's administration" and Beck declaring that "the enemy of my enemy is my friend" and urged "reconciliation" between conservatives and Farrakhan.

Controversies

Farrakhan's police escort in Memphis, Tennessee, 2015

Farrakhan has been the center of much controversy with critics saying that his political views and comments are antisemitic or racist. Farrakhan has categorically denied these charges and stated that much of America's perception of him has been shaped by the media.

Malcolm X's death

Many, including Malcolm X's family, have accused Farrakhan of being involved in the plot to assassinate Malcolm X. For many years, Betty Shabazz, the widow of Malcolm X, harbored resentment toward the Nation of Islam—and Farrakhan in particular—for what she felt was their role in the assassination of her husband. In a 1993 speech, Farrakhan seemed to confirm that the Nation of Islam was responsible for the assassination:

We don't give a damn about no white man law if you attack what we love. And frankly, it ain't none of your business. What do you got to say about it? Did you teach Malcolm? Did you make Malcolm? Did you clean up Malcolm? Did you put Malcolm out before the world? Was Malcolm your traitor or ours? And if we dealt with him like a nation deals with a traitor, what the hell business is it of yours? You just shut your mouth, and stay out of it. Because in the future, we gonna become a nation. And a nation gotta be able to deal with traitors and cutthroats and turncoats. The white man deals with his. The Jews deal with theirs.

During a 1994 interview, Gabe Pressman asked Shabazz whether Farrakhan "had anything to do" with Malcolm X's death. She replied: "Of course, yes. Nobody kept it a secret. It was a badge of honor. Everybody talked about it, yes."

In a 60 Minutes interview that aired during May 2000, Farrakhan stated that some of the things he said may have led to the assassination of Malcolm X. "I may have been complicit in words that I spoke", he said. "I acknowledge that and regret that any word that I have said caused the loss of life of a human being." A few days later Farrakhan denied that he "ordered the assassination" of Malcolm X, although he again acknowledged that he "created the atmosphere that ultimately led to Malcolm X's assassination."

Allegations of sexism

Farrakhan received sexual discrimination complaints filed with a New York state agency when he banned women from attending a speech he gave in a city-owned theater in 1993. The next year he gave a speech only women could attend. In his speech for women, as The New York Times reported,

Mr. Farrakhan urged the women to embrace his formula for a successful family. He encouraged them to put husbands and children ahead of their careers, shun tight, short skirts, stay off welfare and reject abortion. He also stressed the importance of cooking and cleaning and urged women not to abandon homemaking for careers. 'You're just not going to be happy unless there is happiness in the home,' Mr. Farrakhan said at the Mason Cathedral Church of God in Christ in the Dorchester section, not far from the Roxbury neighborhood where he was raised by a single mother. 'Your professional lives can't satisfy your soul like a good, loving man.'

Muammar Gaddafi

In 1985, Farrakhan obtained working capital in the amount of $5 million, in the form of an interest-free loan from Libya's Islamic Call Society to be repaid within 18 months which was to be used to create a toiletries firm with black employees. Libyan President Muammar Gaddafi had also offered Farrahkan guns to begin a black nation. Farrakhan said that he told Gaddafi that he preferred an economic investment in black America.

In January 1996, when Farrakhan visited Libya, Gaddafi pledged giving him a gift of $1 billion and a personal award of $250,000. As economic activity between the two countries had been restricted by the US government since 1986 following allegations of Libya's connection to terrorism, the financial transfer was blocked. It was unclear if Gaddafi would have been in a position to finance the money transfer.

At the time of the wider uprisings in the Arab world and the Tsunami in Japan in a Chicago press conference on March 31, 2011, Farrakhan said President Obama's action in supporting the rebels in Libya were going to advance the arrival of UFOs, or divine spaceships, as punishments for black sufferings. Depicting Obama as engulfed by the people surrounding him, he said: "The stupid mistake that we make is to think that the president is the supreme power. Never was. Money is the power in America. … All of you know what I’m talking about, Zionist control of the government of the United States of America." When Gaddafi was killed in October 2011, Farrakhan blamed Obama's advisors whom he called "wicked demons".

Social media

Farrakhan lost his verified status on his Twitter posts in June 2018, denying him full verification, after asserting the Harvey Weinstein scandal was about "Jewish power". A contributor to the Tablet website, Yair Rosenberg, objected to a potential suspension as "erasing hate from social media doesn’t make it go away, it just makes it easier to ignore" making them more difficult to dismiss as "inconsequential". The following October, Twitter said that it would not suspend Farrakhan's account after a tweet he posted compared Jews to termites as he had not broken the site's rules. After a Twitter rule change on hateful conduct in July 2019, the tweet ("I’m not an anti-Semite. I’m anti-Termite") was removed.

At the beginning of May 2019, Farrakhan was banned from Facebook, along with other prominent individuals considered by the company to be extremists, with antisemitism believed to be the reason for Farrakhan's removal.

During a speech at Saint Sabina Catholic Church in Chicago a week later, Farrakhan stated he had "never been arrested" for "drunken driving" and asked: "What have I done that you would hate me like that?" The Nation of Islam said his speech was Farrakhan's response to the "public outrage over the unprecedented and unwarranted lifetime ban" from Facebook. He insisted he was neither a misogynist nor a homophobe and that: "I do not hate Jewish people". Archbishop of Chicago Cardinal Blase J. Cupich condemned the decision of the church in allowing Farrakhan to speak there.

Other issues

Farrakhan's home in Kenwood, Chicago

Brief return to music

When Farrakhan first joined the NOI, he was asked by Elijah Muhammad to put aside his musical career as a calypso singer. After 42 years, Farrakhan decided to take up the violin once more primarily due to the urging of prominent classical musician Sylvia Olden Lee.

On April 17, 1993, Farrakhan made his return concert debut with performances of the Violin Concerto in E Minor by Felix Mendelssohn. Farrakhan intimated that his performance of a concerto by a Jewish composer was, in part, an effort to heal a rift between him and the Jewish community. (Mendelssohn's family converted to Christianity). The New York Times music critic Bernard Holland reported that Farrakhan's performance was somewhat flawed due to years of neglect, but "nonetheless Mr. Farrakhan's sound is that of the authentic player. It is wide, deep and full of the energy that makes the violin gleam."

Health

Farrakhan announced that he was seriously ill in a letter on September 11, 2006, that was directed to his staff, Nation of Islam members, and supporters. The letter, published in The Final Call newspaper, said that doctors in Cuba had discovered a peptic ulcer. According to the letter subsequent infections caused Farrakhan to lose 35 pounds (16 kg), and he urged the Nation of Islam leadership to carry on while he recovered.

Farrakhan was released from his five-week hospital stay on January 28, 2007, after major abdominal surgery. The operation was performed to correct damage caused by side effects of a radioactive "seed" implantation procedure that he received years earlier to successfully treat prostate cancer.

Following his hospital stay, Farrakhan released a "Message of Appreciation" to supporters and well-wishers and weeks later delivered the keynote address at the Nation of Islam's annual convention in Detroit.

In December 2013, Farrakhan announced that he had not appeared publicly for two months because he had suffered a heart attack in October.

Awards

• 2005, a Black Entertainment Television (BET) poll voted Farrakhan the 'Person of the Year'.

Belief bias

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Belief_bias

Belief bias is the tendency to judge the strength of arguments based on the plausibility of their conclusion rather than how strongly they support that conclusion. A person is more likely to accept an argument that supports a conclusion that aligns with their values, beliefs and prior knowledge, while rejecting counter arguments to the conclusion. Belief bias is an extremely common and therefore significant form of error; we can easily be blinded by our beliefs and reach the wrong conclusion. Belief bias has been found to influence various reasoning tasks, including conditional reasoning, relation reasoning and transitive reasoning.

Syllogisms

Main article: Syllogism

A syllogism is a kind of logical argument in which one proposition (the conclusion) is inferred from two or more others (the premises) of a specific form. The classical example of a valid syllogism is:

All humans are mortal. (major premise)

Socrates is human. (minor premise)

Therefore, Socrates is mortal. (conclusion)

An example of an invalid syllogism is:

All teenage girls are ambitious.

Teenage girls study hard.

Therefore, girls study hard because they are ambitious.

Typically, a majority of test subjects in studies incorrectly identify this syllogism as one in which the conclusion follows from the premises. It might be true in the real world that a) girls study and b) this is because they are ambitious. However, this argument is a fallacy, because the conclusion is not supported by its premises. The validity of an argument is independent from the truth of its conclusion: there are valid arguments for false conclusions and invalid arguments for true conclusions. Hence, it is an error to judge the validity of an argument from the plausibility of its conclusion. This is the reasoning error known as belief bias.

When a person gives a response that is determined by the believability of the conclusion rather than logical validity, this is referred to as belief bias only when a syllogism is used. This phenomenon is so closely related to syllogistic reasoning that, when it does occur, in areas such as Wason's selection task or the THOG problem, it is called "memory cueing" or the "effects of content".

Dual-process theory of belief bias

Many researchers in thinking and reasoning have provided evidence for a dual-process cognitive approach to reasoning, judgment and decision making. They argue that these two mental processes (system 1 and system 2) engage in a constant battle for control over our brain to reason and make decisions. System 1 can be described as an automatic response system characterised by "unconscious", "intuitive" and "rapid" evaluation; whereas system 2 is said to be a controlled response system, characterised by “conscious”, “analytic” and “slow” evaluation; some researchers even claimed to have found a link between general intelligence and the effectiveness of decision making. It is important to note that the dual-process cognitive theory is different from the two minds hypothesis. Research done by Jonathan Evans in 2007 provided evidence for the view that System 1, which serves as a quick heuristic processor, fights for control over System 2's slower analytical approach. In the experiment, participants were asked to evaluate syllogisms that have valid arguments with unconvincing conclusions; valid arguments with convincing conclusions; invalid arguments with unconvincing conclusions; invalid arguments with convincing conclusions. The results show that when the conclusion is believable, people blindly accept invalid conclusions more so than invalid arguments are accepted.

Influencing factors of belief bias

Time

Various studies have proved that the time period for which a subject is allowed to think when evaluating arguments is related to the tendency for belief bias to take place. In a study done by Evans and Holmes in 2005, they recruited two different groups of people to answer a series of reasoning questions. One group of people were given only two seconds to answer the questions; whereas the other group of people were allowed to use as much time as they would like to answer the questions. The result obtained was that a higher percentage of incorrect answers were found in the time pressured group than the other; they concluded that this was a result of a shift in logical to belief-biased way of thinking.

Nature of content

The nature of the content presented can also affect belief bias of an individual as shown by a study done by Goel & Vartanian in 2011. In their experiment, 34 participants were presented with a syllogism upon each trial. Each trial were either neutral or carried some degree of negative content. Negative content involved in the experiment were politically incorrect social norm violations, such as the statement “Some wars are not unjustified, Some wars involve raping of women, therefore, Some raping of women is not unjustified”. For syllogisms where the content was neutral, the results were consistent with studies of belief bias; however, for syllogisms with negative emotional content, participants were more likely to reason logically on invalid syllogisms with believable conclusions instead of automatically judging them to be valid. In other words, the effect of belief bias is reduced when the content presented is of negative emotion. According to Goel and Vartanian, this is because negative emotions prompts us to reason in more careful and in more detail. This argument was supported by the observation that for questions with negative emotions, the reaction time taken was significantly longer than that of questions that a neutral.

Instructions given

In an experiment done by Evans, Newstead, Allen & Pollard in 1994, where subjects were given detailed instructions which lack specific reference to the notion of logical necessity when answering questions, it was shown that a larger proportion of answers actually rejected invalid arguments with convincing conclusions as opposed to when no further instructions were given when the subjects were asked to answer the questions. The results of the experiments reflects that when elaborated instructions were given to subjects to reason logically, the effects of belief bias is decreased.

Research

In a series of experiments by Evans, Barston and Pollard (1983), participants were presented with evaluation task paradigms, containing two premises and a conclusion. The participants were asked to make an evaluation of logical validity. The subjects, however, exhibited a belief bias, evidenced by their tendency to reject valid arguments with unbelievable conclusions, and endorse invalid arguments with believable conclusions. Instead of following directions and assessing logical validity, the subjects based their assessments on personal beliefs.

Consequently, these results demonstrated a greater acceptance of more believable (80%), than unbelievable (33%) conclusions. Participants also illustrated evidence of logical competences and the results determined an increase in acceptance of valid (73%) than invalid (41%). Additionally, there's a small difference between believable and valid (89%) in comparison to unbelievable and invalid (56%) (Evans, Barston & Pollard, 1983; Morley, Evans & Handley, 2004).

It has been argued that using more realistic content in syllogisms can facilitate more normative performance from participants. It has been suggested that the use of more abstract, artificial content will also have a biasing effect on performance. Therefore, more research is required to fully understand how and why belief bias occurs and if there are certain mechanisms that are responsible for such things. There is also evidence of clear individual differences in normative responding that are predicted by the response times of participants.

A 1989 study by Markovits and Nantel gave participants four reasoning tasks. The results indicated “a significant belief-bias effect” that existed “independently of the subjects' abstract reasoning ability.”

A 2010 study by Donna Torrens examined differences in belief bias among individuals. Torrens found that “the extent of an individual's belief bias effect was unrelated to a number of measures of reasoning competence” but was, instead, related to that person's ability “to generate alternative representations of premises: the more alternatives a person generated, the less likely they were to show a belief bias effect."

In a 2010 study, Chad Dube and Caren M. Rotello of the University of Massachusetts and Evan Heit of the University of California, Merced, showed that “the belief bias effect is simply a response bias effect.”

In a 2012 study, Adrian P. Banks of the University of Surrey explained that “belief bias is caused by the believability of a conclusion in working memory which influences its activation level, determining its likelihood of retrieval and therefore its effect on the reasoning process.”

Michelle Colleen and Elizabeth Hilscher of the University of Toronto showed in 2014 that belief bias can be affected by the difficulty level and placement of the syllogism in question.