Binomial distribution

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Binomial_distribution 

Probability mass function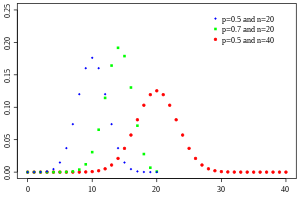
Cumulative distribution function
Notation	${\displaystyle B(n,p)}$
Parameters	${\displaystyle n\in \{0,1,2,\ldots \}}$ – number of trials ${\displaystyle p\in [0,1]}$ – success probability for each trial ${\displaystyle q=1-p}$
Support	${\displaystyle k\in \{0,1,\ldots ,n\}}$ – number of successes
PMF	${\displaystyle {\binom {n}{k}}p^{k}q^{n-k}}$
CDF	${\displaystyle I_{q}(n-k,1+k)}$
Mean	${\displaystyle np}$
Median	${\displaystyle \lfloor np\rfloor }$ or ${\displaystyle \lceil np\rceil }$
Mode	${\displaystyle \lfloor (n+1)p\rfloor }$ or ${\displaystyle \lceil (n+1)p\rceil -1}$
Variance	${\displaystyle npq}$
Skewness	${\displaystyle {\frac {q-p}{\sqrt {npq}}}}$
Ex. kurtosis	${\displaystyle {\frac {1-6pq}{npq}}}$
Entropy	${\displaystyle {\frac {1}{2}}\log _{2}(2\pi enpq)+O\left({\frac {1}{n}}\right)}$ in shannons. For nats, use the natural log in the log.
MGF	${\displaystyle (q+pe^{t})^{n}}$
CF	${\displaystyle (q+pe^{it})^{n}}$
PGF	${\displaystyle G(z)=[q+pz]^{n}}$
Fisher information	${\displaystyle g_{n}(p)={\frac {n}{pq}}}$ (for fixed ${\displaystyle n}$)

Part of a series on statistics
Probability theory
Probability Axioms Determinism System Indeterminism Randomness
Probability space Sample space Event Collectively exhaustive events Elementary event Mutual exclusivity Outcome Singleton Experiment Bernoulli trial Probability distribution Bernoulli distribution Binomial distribution Normal distribution Probability measure Random variable Bernoulli process Continuous or discrete Expected value Markov chain Observed value Random walk Stochastic process
Complementary event Joint probability Marginal probability Conditional probability
Independence Conditional independence Law of total probability Law of large numbers Bayes' theorem Boole's inequality
Venn diagram Tree diagram

Binomial distribution for ${\displaystyle p=0.5}$ with n and k as in Pascal's triangle

The probability that a ball in a Galton box with 8 layers (n = 8) ends up in the central bin (k = 4) is ${\displaystyle 70/256}$.

In probability theory and statistics, the binomial distribution with parameters n and p is the discrete probability distribution of the number of successes in a sequence of n independent experiments, each asking a yes–no question, and each with its own Boolean-valued outcome: success (with probability p) or failure (with probability q = 1 − p). A single success/failure experiment is also called a Bernoulli trial or Bernoulli experiment, and a sequence of outcomes is called a Bernoulli process; for a single trial, i.e., n = 1, the binomial distribution is a Bernoulli distribution. The binomial distribution is the basis for the popular binomial test of statistical significance.

The binomial distribution is frequently used to model the number of successes in a sample of size n drawn with replacement from a population of size N. If the sampling is carried out without replacement, the draws are not independent and so the resulting distribution is a hypergeometric distribution, not a binomial one. However, for N much larger than n, the binomial distribution remains a good approximation, and is widely used.

Definitions

Probability mass function

In general, if the random variable X follows the binomial distribution with parameters n ∈ ${\displaystyle \mathbb {N} }$ and p ∈ [0,1], we write X ~ B(n, p). The probability of getting exactly k successes in n independent Bernoulli trials is given by the probability mass function:

${\displaystyle f(k,n,p)=\Pr(k;n,p)=\Pr(X=k)={\binom {n}{k}}p^{k}(1-p)^{n-k}}$

for k = 0, 1, 2, ..., n, where

${\displaystyle {\binom {n}{k}}={\frac {n!}{k!(n-k)!}}}$

is the binomial coefficient, hence the name of the distribution. The formula can be understood as follows: k successes occur with probability p^k and n − k failures occur with probability (1 − p)^n − k. However, the k successes can occur anywhere among the n trials, and there are ${\displaystyle {\tbinom {n}{k}}}$ different ways of distributing k successes in a sequence of n trials.

In creating reference tables for binomial distribution probability, usually the table is filled in up to n/2 values. This is because for k > n/2, the probability can be calculated by its complement as

${\displaystyle f(k,n,p)=f(n-k,n,1-p).}$

Looking at the expression f(k, n, p) as a function of k, there is a k value that maximizes it. This k value can be found by calculating

${\displaystyle {\frac {f(k+1,n,p)}{f(k,n,p)}}={\frac {(n-k)p}{(k+1)(1-p)}}}$

and comparing it to 1. There is always an integer M that satisfies

${\displaystyle (n+1)p-1\leq M<(n+1)p.}$

f(k, n, p) is monotone increasing for k < M and monotone decreasing for k > M, with the exception of the case where (n + 1)p is an integer. In this case, there are two values for which f is maximal: (n + 1)p and (n + 1)p − 1. M is the most probable outcome (that is, the most likely, although this can still be unlikely overall) of the Bernoulli trials and is called the mode.

Example

Suppose a biased coin comes up heads with probability 0.3 when tossed. The probability of seeing exactly 4 heads in 6 tosses is

${\displaystyle f(4,6,0.3)={\binom {6}{4}}0.3^{4}(1-0.3)^{6-4}=0.059535.}$

Cumulative distribution function

The cumulative distribution function can be expressed as:

${\displaystyle F(k;n,p)=\Pr(X\leq k)=\sum _{i=0}^{\lfloor k\rfloor }{n \choose i}p^{i}(1-p)^{n-i},}$

where ${\displaystyle \lfloor k\rfloor }$ is the "floor" under k, i.e. the greatest integer less than or equal to k.

It can also be represented in terms of the regularized incomplete beta function, as follows:

${\displaystyle {\begin{aligned}F(k;n,p)&=\Pr(X\leq k)\\&=I_{1-p}(n-k,k+1)\\&=(n-k){n \choose k}\int _{0}^{1-p}t^{n-k-1}(1-t)^{k}\,dt.\end{aligned}}}$

which is equivalent to the cumulative distribution function of the F-distribution:

${\displaystyle F(k;n,p)=F_{F{\text{-distribution}}}\left(x={\frac {1-p}{p}}{\frac {k+1}{n-k}};d_{1}=2(n-k),d_{2}=2(k+1)\right).}$

Some closed-form bounds for the cumulative distribution function are given below.

Properties

Expected value and variance

If X ~ B(n, p), that is, X is a binomially distributed random variable, n being the total number of experiments and p the probability of each experiment yielding a successful result, then the expected value of X is:^[5]

${\displaystyle \operatorname {E} [X]=np.}$

This follows from the linearity of the expected value along with the fact that X is the sum of n identical Bernoulli random variables, each with expected value p. In other words, if ${\displaystyle X_{1},\ldots ,X_{n}}$ are identical (and independent) Bernoulli random variables with parameter p, then ${\displaystyle X=X_{1}+\cdots +X_{n}}$ and

${\displaystyle \operatorname {E} [X]=\operatorname {E} [X_{1}+\cdots +X_{n}]=\operatorname {E} [X_{1}]+\cdots +\operatorname {E} [X_{n}]=p+\cdots +p=np.}$

The variance is:

${\displaystyle \operatorname {Var} (X)=npq=np(1-p).}$

This similarly follows from the fact that the variance of a sum of independent random variables is the sum of the variances.

Higher moments

The first 6 central moments, defined as ${\displaystyle \mu _{c}=\operatorname {E} \left[(X-\operatorname {E} [X])^{c}\right]}$, are given by

${\displaystyle {\begin{aligned}\mu _{1}&=0,\\\mu _{2}&=np(1-p),\\\mu _{3}&=np(1-p)(1-2p),\\\mu _{4}&=np(1-p)(1+(3n-6)p(1-p)),\\\mu _{5}&=np(1-p)(1-2p)(1+(10n-12)p(1-p)),\\\mu _{6}&=np(1-p)(1-30p(1-p)(1-4p(1-p))+5np(1-p)(5-26p(1-p))+15n^{2}p^{2}(1-p)^{2}).\end{aligned}}}$

The non-central moments satisfy

${\displaystyle {\begin{aligned}\operatorname {E} [X]&=np,\\\operatorname {E} [X^{2}]&=np(1-p)+n^{2}p^{2},\end{aligned}}}$

and in general ^[6]

${\displaystyle \operatorname {E} [X^{c}]=\sum _{k=0}^{c}\left\{{c \atop k}\right\}n^{\underline {k}}p^{k},}$

where ${\displaystyle \textstyle \left\{{c \atop k}\right\}}$ are the Stirling numbers of the second kind, and ${\displaystyle n^{\underline {k}}=n(n-1)\cdots (n-k+1)}$ is the ${\displaystyle k}$th falling power of ${\displaystyle n}$. A simple bound  follows by bounding the Binomial moments via the higher Poisson moments:

${\displaystyle \operatorname {E} [X^{c}]\leq \left({\frac {c}{\log(c/(np)+1)}}\right)^{c}\leq (np)^{c}\exp \left({\frac {c^{2}}{2np}}\right).}$

This shows that if ${\displaystyle c=O({\sqrt {np}})}$, then ${\displaystyle \operatorname {E} [X^{c}]}$ is at most a constant factor away from ${\displaystyle \operatorname {E} [X]^{c}}$

Mode

Usually the mode of a binomial B(n, p) distribution is equal to ${\displaystyle \lfloor (n+1)p\rfloor }$, where ${\displaystyle \lfloor \cdot \rfloor }$ is the floor function. However, when (n + 1)p is an integer and p is neither 0 nor 1, then the distribution has two modes: (n + 1)p and (n + 1)p − 1. When p is equal to 0 or 1, the mode will be 0 and n correspondingly. These cases can be summarized as follows:

${\displaystyle {\text{mode}}={\begin{cases}\lfloor (n+1)\,p\rfloor &{\text{if }}(n+1)p{\text{ is 0 or a noninteger}},\\(n+1)\,p\ {\text{ and }}\ (n+1)\,p-1&{\text{if }}(n+1)p\in \{1,\dots ,n\},\\n&{\text{if }}(n+1)p=n+1.\end{cases}}}$

Proof: Let

${\displaystyle f(k)={\binom {n}{k}}p^{k}q^{n-k}.}$

For ${\displaystyle p=0}$ only ${\displaystyle f(0)}$ has a nonzero value with ${\displaystyle f(0)=1}$. For ${\displaystyle p=1}$ we find ${\displaystyle f(n)=1}$ and ${\displaystyle f(k)=0}$ for ${\displaystyle k\neq n}$. This proves that the mode is 0 for ${\displaystyle p=0}$ and ${\displaystyle n}$ for ${\displaystyle p=1}$.

Let ${\displaystyle 0<p<1}$. We find

${\displaystyle {\frac {f(k+1)}{f(k)}}={\frac {(n-k)p}{(k+1)(1-p)}}}$.

From this follows

${\displaystyle {\begin{aligned}k>(n+1)p-1\Rightarrow f(k+1)<f(k)\\k=(n+1)p-1\Rightarrow f(k+1)=f(k)\\k<(n+1)p-1\Rightarrow f(k+1)>f(k)\end{aligned}}}$

So when ${\displaystyle (n+1)p-1}$ is an integer, then ${\displaystyle (n+1)p-1}$ and ${\displaystyle (n+1)p}$ is a mode. In the case that ${\displaystyle (n+1)p-1\notin \mathbb {Z} }$, then only ${\displaystyle \lfloor (n+1)p-1\rfloor +1=\lfloor (n+1)p\rfloor }$ is a mode.

Median

In general, there is no single formula to find the median for a binomial distribution, and it may even be non-unique. However, several special results have been established:

• If np is an integer, then the mean, median, and mode coincide and equal np.
• Any median m must lie within the interval ⌊np⌋ ≤ m ≤ ⌈np⌉.
• A median m cannot lie too far away from the mean: |m − np| ≤ min{ ln 2, max{p, 1 − p} }.
• The median is unique and equal to m = round(np) when |m − np| ≤ min{p, 1 − p} (except for the case when p = 1/2 and n is odd).
• When p is a rational number (with the exception of p = 1/2 and n odd) the median is unique.
• When p = 1/2 and n is odd, any number m in the interval 1/2(n − 1) ≤ m ≤ 1/2(n + 1) is a median of the binomial distribution. If p = 1/2 and n is even, then m = n/2 is the unique median.

Tail bounds

For k ≤ np, upper bounds can be derived for the lower tail of the cumulative distribution function ${\displaystyle F(k;n,p)=\Pr(X\leq k)}$, the probability that there are at most k successes. Since ${\displaystyle \Pr(X\geq k)=F(n-k;n,1-p)}$, these bounds can also be seen as bounds for the upper tail of the cumulative distribution function for k ≥ np.

Hoeffding's inequality yields the simple bound

${\displaystyle F(k;n,p)\leq \exp \left(-2n\left(p-{\frac {k}{n}}\right)^{2}\right),\!}$

which is however not very tight. In particular, for p = 1, we have that F(k;n,p) = 0 (for fixed k, n with k < n), but Hoeffding's bound evaluates to a positive constant.

A sharper bound can be obtained from the Chernoff bound:

${\displaystyle F(k;n,p)\leq \exp \left(-nD\left({\frac {k}{n}}\parallel p\right)\right)}$

where D(a || p) is the relative entropy (or Kullback-Leibler divergence) between an a-coin and a p-coin (i.e. between the Bernoulli(a) and Bernoulli(p) distribution):

${\displaystyle D(a\parallel p)=(a)\log {\frac {a}{p}}+(1-a)\log {\frac {1-a}{1-p}}.\!}$

Asymptotically, this bound is reasonably tight.

One can also obtain lower bounds on the tail ${\displaystyle F(k;n,p)}$, known as anti-concentration bounds. By approximating the binomial coefficient with Stirling's formula it can be shown that

${\displaystyle F(k;n,p)\geq {\frac {1}{\sqrt {8n{\tfrac {k}{n}}(1-{\tfrac {k}{n}})}}}\exp \left(-nD\left({\frac {k}{n}}\parallel p\right)\right),}$

which implies the simpler but looser bound

${\displaystyle F(k;n,p)\geq {\frac {1}{\sqrt {2n}}}\exp \left(-nD\left({\frac {k}{n}}\parallel p\right)\right).}$

For p = 1/2 and k ≥ 3n/8 for even n, it is possible to make the denominator constant:

${\displaystyle F(k;n,{\tfrac {1}{2}})\geq {\frac {1}{15}}\exp \left(-16n\left({\frac {1}{2}}-{\frac {k}{n}}\right)^{2}\right).\!}$

Statistical inference

Estimation of parameters

See also: Beta distribution § Bayesian inference

When n is known, the parameter p can be estimated using the proportion of successes:

${\displaystyle {\widehat {p}}={\frac {x}{n}}.}$

This estimator is found using maximum likelihood estimator and also the method of moments. This estimator is unbiased and uniformly with minimum variance, proven using Lehmann–Scheffé theorem, since it is based on a minimal sufficient and complete statistic (i.e.: x). It is also consistent both in probability and in MSE.

A closed form Bayes estimator for p also exists when using the Beta distribution as a conjugate prior distribution. When using a general ${\displaystyle \operatorname {Beta} (\alpha ,\beta )}$ as a prior, the posterior mean estimator is:

${\displaystyle {\widehat {p}}_{b}={\frac {x+\alpha }{n+\alpha +\beta }}.}$

The Bayes estimator is asymptotically efficient and as the sample size approaches infinity (n → ∞), it approaches the MLE solution. The Bayes estimator is biased (how much depends on the priors), admissible and consistent in probability.

For the special case of using the standard uniform distribution as a non-informative prior, ${\displaystyle \operatorname {Beta} (\alpha =1,\beta =1)=U(0,1)}$, the posterior mean estimator becomes:

${\displaystyle {\widehat {p}}_{b}={\frac {x+1}{n+2}}.}$

(A posterior mode should just lead to the standard estimator.) This method is called the rule of succession, which was introduced in the 18th century by Pierre-Simon Laplace.

When estimating p with very rare events and a small n (e.g.: if x=0), then using the standard estimator leads to ${\displaystyle {\widehat {p}}=0,}$ which sometimes is unrealistic and undesirable. In such cases there are various alternative estimators. One way is to use the Bayes estimator, leading to:

${\displaystyle {\widehat {p}}_{b}={\frac {1}{n+2}}.}$

Another method is to use the upper bound of the confidence interval obtained using the rule of three:

${\displaystyle {\widehat {p}}_{\text{rule of 3}}={\frac {3}{n}}.}$

Confidence intervals

Main article: Binomial proportion confidence interval

Even for quite large values of n, the actual distribution of the mean is significantly nonnormal. Because of this problem several methods to estimate confidence intervals have been proposed.

In the equations for confidence intervals below, the variables have the following meaning:

• n₁ is the number of successes out of n, the total number of trials
• ${\displaystyle {\widehat {p\,}}={\frac {n_{1}}{n}}}$ is the proportion of successes
• ${\displaystyle z=1-{\tfrac {1}{2}}\alpha }$ is the quantile of a standard normal distribution (i.e., probit) corresponding to the target error rate ${\displaystyle \alpha }$. For example, for a 95% confidence level the error ${\displaystyle \alpha }$ = 0.05, so ${\displaystyle 1-{\tfrac {1}{2}}\alpha }$ = 0.975 and ${\displaystyle z}$ = 1.96.

Wald method

Main article: Binomial proportion confidence interval § Wald interval

${\displaystyle {\widehat {p\,}}\pm z{\sqrt {\frac {{\widehat {p\,}}(1-{\widehat {p\,}})}{n}}}.}$

A continuity correction of 0.5/n may be added.

Agresti–Coull method

Main article: Binomial proportion confidence interval § Agresti–Coull interval

${\displaystyle {\tilde {p}}\pm z{\sqrt {\frac {{\tilde {p}}(1-{\tilde {p}})}{n+z^{2}}}}}$

Here the estimate of p is modified to

${\displaystyle {\tilde {p}}={\frac {n_{1}+{\frac {1}{2}}z^{2}}{n+z^{2}}}}$

This method works well for ${\displaystyle n>10}$ and ${\displaystyle n_{1}\neq 0,n}$. See here for ${\displaystyle n\leq 10}$. For ${\displaystyle n_{1}=0,n}$ use the Wilson (score) method below.

Arcsine method

Main article: Binomial proportion confidence interval § Arcsine transformation

${\displaystyle \sin ^{2}\left(\arcsin \left({\sqrt {\widehat {p\,}}}\right)\pm {\frac {z}{2{\sqrt {n}}}}\right).}$

Wilson (score) method

Main article: Binomial proportion confidence interval § Wilson score interval

The notation in the formula below differs from the previous formulas in two respects:

• Firstly, z_x has a slightly different interpretation in the formula below: it has its ordinary meaning of 'the xth quantile of the standard normal distribution', rather than being a shorthand for 'the (1 − x)-th quantile'.
• Secondly, this formula does not use a plus-minus to define the two bounds. Instead, one may use ${\displaystyle z=z_{\alpha /2}}$ to get the lower bound, or use ${\displaystyle z=z_{1-\alpha /2}}$ to get the upper bound. For example: for a 95% confidence level the error ${\displaystyle \alpha }$ = 0.05, so one gets the lower bound by using ${\displaystyle z=z_{\alpha /2}=z_{0.025}=-1.96}$, and one gets the upper bound by using ${\displaystyle z=z_{1-\alpha /2}=z_{0.975}=1.96}$.

${\displaystyle {\frac {{\widehat {p\,}}+{\frac {z^{2}}{2n}}+z{\sqrt {{\frac {{\widehat {p\,}}(1-{\widehat {p\,}})}{n}}+{\frac {z^{2}}{4n^{2}}}}}}{1+{\frac {z^{2}}{n}}}}}$

Comparison

The so-called "exact" (Clopper–Pearson) method is the most conservative. (Exact does not mean perfectly accurate; rather, it indicates that the estimates will not be less conservative than the true value.)

The Wald method, although commonly recommended in textbooks, is the most biased.

Related distributions

Sums of binomials

If X ~ B(n, p) and Y ~ B(m, p) are independent binomial variables with the same probability p, then X + Y is again a binomial variable; its distribution is Z=X+Y ~ B(n+m, p):

${\displaystyle {\begin{aligned}\operatorname {P} (Z=k)&=\sum _{i=0}^{k}\left[{\binom {n}{i}}p^{i}(1-p)^{n-i}\right]\left[{\binom {m}{k-i}}p^{k-i}(1-p)^{m-k+i}\right]\\&={\binom {n+m}{k}}p^{k}(1-p)^{n+m-k}\end{aligned}}}$

A Binomial distributed random variable X ~ B(n, p) can be considered as the sum of n Bernoulli distributed random variables. So the sum of two Binomial distributed random variable X ~ B(n, p) and Y ~ B(m, p) is equivalent to the sum of n + m Bernoulli distributed random variables, which means Z=X+Y ~ B(n+m, p). This can also be proven directly using the addition rule.

However, if X and Y do not have the same probability p, then the variance of the sum will be smaller than the variance of a binomial variable distributed as ${\displaystyle B(n+m,{\bar {p}}).\,}$

Poisson binomial distribution

The binomial distribution is a special case of the Poisson binomial distribution, which is the distribution of a sum of n independent non-identical Bernoulli trials B(p_i).

Ratio of two binomial distributions

This result was first derived by Katz and coauthors in 1978.

Let X ~ B(n,p₁) and Y ~ B(m,p₂) be independent. Let T = (X/n)/(Y/m).

Then log(T) is approximately normally distributed with mean log(p₁/p₂) and variance ((1/p₁) − 1)/n + ((1/p₂) − 1)/m.

Conditional binomials

If X ~ B(n, p) and Y | X ~ B(X, q) (the conditional distribution of Y, given X), then Y is a simple binomial random variable with distribution Y ~ B(n, pq).

For example, imagine throwing n balls to a basket U_X and taking the balls that hit and throwing them to another basket U_Y. If p is the probability to hit U_X then X ~ B(n, p) is the number of balls that hit U_X. If q is the probability to hit U_Y then the number of balls that hit U_Y is Y ~ B(X, q) and therefore Y ~ B(n, pq).

[Proof]

Bernoulli distribution

The Bernoulli distribution is a special case of the binomial distribution, where n = 1. Symbolically, X ~ B(1, p) has the same meaning as X ~ Bernoulli(p). Conversely, any binomial distribution, B(n, p), is the distribution of the sum of n independent Bernoulli trials, Bernoulli(p), each with the same probability p.

Normal approximation

See also: Binomial proportion confidence interval § Normal approximation interval

 

Binomial probability mass function and normal probability density function approximation for n = 6 and p = 0.5

If n is large enough, then the skew of the distribution is not too great. In this case a reasonable approximation to B(n, p) is given by the normal distribution

${\displaystyle {\mathcal {N}}(np,\,np(1-p)),}$

and this basic approximation can be improved in a simple way by using a suitable continuity correction. The basic approximation generally improves as n increases (at least 20) and is better when p is not near to 0 or 1. Various rules of thumb may be used to decide whether n is large enough, and p is far enough from the extremes of zero or one:

• One rule is that for n > 5 the normal approximation is adequate if the absolute value of the skewness is strictly less than 1/3; that is, if

${\displaystyle {\frac {|1-2p|}{\sqrt {np(1-p)}}}={\frac {1}{\sqrt {n}}}\left|{\sqrt {\frac {1-p}{p}}}-{\sqrt {\frac {p}{1-p}}}\,\right|<{\frac {1}{3}}.}$

This can be made precise using the Berry–Esseen theorem.

• A stronger rule states that the normal approximation is appropriate only if everything within 3 standard deviations of its mean is within the range of possible values; that is, only if

${\displaystyle \mu \pm 3\sigma =np\pm 3{\sqrt {np(1-p)}}\in (0,n).}$

This 3-standard-deviation rule is equivalent to the following conditions, which also imply the first rule above.

${\displaystyle n>9\left({\frac {1-p}{p}}\right)\quad {\text{and}}\quad n>9\left({\frac {p}{1-p}}\right).}$

[Proof]

• Another commonly used rule is that both values ${\displaystyle np}$ and ${\displaystyle n(1-p)}$ must be greater than or equal to 5. However, the specific number varies from source to source, and depends on how good an approximation one wants. In particular, if one uses 9 instead of 5, the rule implies the results stated in the previous paragraphs.

[Proof]

The following is an example of applying a continuity correction. Suppose one wishes to calculate Pr(X ≤ 8) for a binomial random variable X. If Y has a distribution given by the normal approximation, then Pr(X ≤ 8) is approximated by Pr(Y ≤ 8.5). The addition of 0.5 is the continuity correction; the uncorrected normal approximation gives considerably less accurate results.

This approximation, known as de Moivre–Laplace theorem, is a huge time-saver when undertaking calculations by hand (exact calculations with large n are very onerous); historically, it was the first use of the normal distribution, introduced in Abraham de Moivre's book The Doctrine of Chances in 1738. Nowadays, it can be seen as a consequence of the central limit theorem since B(n, p) is a sum of n independent, identically distributed Bernoulli variables with parameter p. This fact is the basis of a hypothesis test, a "proportion z-test", for the value of p using x/n, the sample proportion and estimator of p, in a common test statistic.

For example, suppose one randomly samples n people out of a large population and ask them whether they agree with a certain statement. The proportion of people who agree will of course depend on the sample. If groups of n people were sampled repeatedly and truly randomly, the proportions would follow an approximate normal distribution with mean equal to the true proportion p of agreement in the population and with standard deviation

${\displaystyle \sigma ={\sqrt {\frac {p(1-p)}{n}}}}$

Poisson approximation

The binomial distribution converges towards the Poisson distribution as the number of trials goes to infinity while the product np remains fixed or at least p tends to zero. Therefore, the Poisson distribution with parameter λ = np can be used as an approximation to B(n, p) of the binomial distribution if n is sufficiently large and p is sufficiently small. According to two rules of thumb, this approximation is good if n ≥ 20 and p ≤ 0.05, or if n ≥ 100 and np ≤ 10.

Concerning the accuracy of Poisson approximation, see Novak, ch. 4, and references therein.

Limiting distributions

• Poisson limit theorem: As n approaches ∞ and p approaches 0 with the product np held fixed, the Binomial(n, p) distribution approaches the Poisson distribution with expected value λ = np.
• de Moivre–Laplace theorem: As n approaches ∞ while p remains fixed, the distribution of

${\displaystyle {\frac {X-np}{\sqrt {np(1-p)}}}}$

approaches the normal distribution with expected value 0 and variance 1. This result is sometimes loosely stated by saying that the distribution of X is asymptotically normal with expected value 0 and variance 1. This result is a specific case of the central limit theorem.

Beta distribution

The binomial distribution and beta distribution are different views of the same model of repeated Bernoulli trials. The binomial distribution is the PMF of k successes given n independent events each with a probability p of success. Mathematically, when α = k + 1 and β = n − k + 1, the beta distribution and the binomial distribution are related by a factor of n + 1:

${\displaystyle \operatorname {Beta} (p;\alpha ;\beta )=(n+1)B(k;n;p)}$

Beta distributions also provide a family of prior probability distributions for binomial distributions in Bayesian inference:

${\displaystyle P(p;\alpha ,\beta )={\frac {p^{\alpha -1}(1-p)^{\beta -1}}{\operatorname {Beta} (\alpha ,\beta )}}.}$

Given a uniform prior, the posterior distribution for the probability of success p given n independent events with k observed successes is a beta distribution.

Random number generation

Further information: Pseudo-random number sampling

Methods for random number generation where the marginal distribution is a binomial distribution are well-established. One way to generate random variates samples from a binomial distribution is to use an inversion algorithm. To do so, one must calculate the probability that Pr(X = k) for all values k from 0 through n. (These probabilities should sum to a value close to one, in order to encompass the entire sample space.) Then by using a pseudorandom number generator to generate samples uniformly between 0 and 1, one can transform the calculated samples into discrete numbers by using the probabilities calculated in the first step.

History

This distribution was derived by Jacob Bernoulli. He considered the case where p = r/(r + s) where p is the probability of success and r and s are positive integers. Blaise Pascal had earlier considered the case where p = 1/2.

Vitamin

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Vitamin 

Vitamin
A bottle of B-complex vitamin pills
Pronunciation	UK: /ˈvɪtəmɪn, ˈvaɪt-/ VIT-ə-min, VYTE-, US: /ˈvaɪtəmɪn/ VY-tə-min

A vitamin is an organic molecule (or a set of molecules closely related chemically, i.e. vitamers) that is an essential micronutrient that an organism needs in small quantities for the proper functioning of its metabolism. Essential nutrients cannot be synthesized in the organism, either at all or not in sufficient quantities, and therefore must be obtained through the diet. Vitamin C can be synthesized by some species but not by others; it is not a vitamin in the first instance but is in the second. The term vitamin does not include the three other groups of essential nutrients: minerals, essential fatty acids, and essential amino acids. Most vitamins are not single molecules, but groups of related molecules called vitamers. For example, there are eight vitamers of vitamin E: four tocopherols and four tocotrienols. Some sources list fourteen vitamins, by including choline, but major health organizations list thirteen: vitamin A (as all-trans-retinol, all-trans-retinyl-esters, as well as all-trans-beta-carotene and other provitamin A carotenoids), vitamin B₁ (thiamine), vitamin B₂ (riboflavin), vitamin B₃ (niacin), vitamin B₅ (pantothenic acid), vitamin B₆ (pyridoxine), vitamin B₇ (biotin), vitamin B₉ (folic acid or folate), vitamin B₁₂ (cobalamins), vitamin C (ascorbic acid), vitamin D (calciferols), vitamin E (tocopherols and tocotrienols), and vitamin K (phylloquinone and menaquinones).

Vitamins have diverse biochemical functions. Vitamin A acts as a regulator of cell and tissue growth and differentiation. Vitamin D provides a hormone-like function, regulating mineral metabolism for bones and other organs. The B complex vitamins function as enzyme cofactors (coenzymes) or the precursors for them. Vitamins C and E function as antioxidants. Both deficient and excess intake of a vitamin can potentially cause clinically significant illness, although excess intake of water-soluble vitamins is less likely to do so.

All vitamins were discovered (identified) between 1913 and 1948. Historically, when intake of vitamins from diet was lacking, the results were vitamin deficiency diseases. Then, starting in 1935, commercially produced tablets of yeast-extract vitamin B complex and semi-synthetic vitamin C became available. This was followed in the 1950s by the mass production and marketing of vitamin supplements, including multivitamins, to prevent vitamin deficiencies in the general population. Governments have mandated the addition of some vitamins to staple foods such as flour or milk, referred to as food fortification, to prevent deficiencies. Recommendations for folic acid supplementation during pregnancy reduced risk of infant neural tube defects.

Etymology

The term "vitamin" was derived from "vitamine", a compound word coined in 1912 by the biochemist Casimir Funk while working at the Lister Institute of Preventive Medicine. Funk created the name from vital and amine, because it appeared that these organic micronutrient food factors that prevent beriberi and perhaps other similar dietary-deficiency diseases were required for life, hence "vital," and were chemical amines, hence "amine.". This was true of thiamine, but after it was found that vitamin C and other such micronutrients were not amines, the word was shortened to "vitamin" in English.

List

Vitamin	Vitamers (incomplete)	Solubility	U.S. recommended dietary allowances per day ages 19–70)	Deficiency disease(s)	Overdose syndrome/symptoms	Food sources
Vitamin A	all-trans-Retinol, Retinals, and provitamin Carotenoids including all-trans-beta-carotene	Fat	900 µg/700 µg	Night blindness, hyperkeratosis, and keratomalacia	Hypervitaminosis A	from animal origin as Vitamin A / all-trans-Retinol: Fish in general, liver and dairy products; from plant origin as provitamin A / all-trans-beta-carotene: orange, ripe yellow fruits, leafy vegetables, carrots, pumpkin, squash, spinach
Vitamin B1	Thiamine	Water	1.2 mg/1.1 mg	Beriberi, Wernicke-Korsakoff syndrome	Drowsiness and muscle relaxation	Pork, wholemeal grains, brown rice, vegetables, potatoes, liver, eggs
Vitamin B2	Riboflavin	Water	1.3 mg/1.1 mg	Ariboflavinosis, glossitis, angular stomatitis		Dairy products, bananas, green beans, asparagus
Vitamin B3	Niacin, Niacinamide, Nicotinamide riboside	Water	16 mg/14 mg	Pellagra	Liver damage (doses > 2g/day) and other problems	Meat, fish, eggs, many vegetables, mushrooms, tree nuts
Vitamin B5	Pantothenic acid	Water	5 mg/5 mg	Paresthesia	Diarrhea; possibly nausea and heartburn.	Meat, broccoli, avocados
Vitamin B6	Pyridoxine, Pyridoxamine, Pyridoxal	Water	1.3–1.7 mg/1.2–1.5 mg	Anemia, Peripheral neuropathy	Impairment of proprioception, nerve damage (doses > 100 mg/day)	Meat, vegetables, tree nuts, bananas
Vitamin B7	Biotin	Water	AI: 30 µg/30 µg	Dermatitis, enteritis		Raw egg yolk, liver, peanuts, leafy green vegetables
Vitamin B9	Folates, Folic acid	Water	400 µg/400 µg	Megaloblastic anemia and deficiency during pregnancy is associated with birth defects, such as neural tube defects	May mask symptoms of vitamin B12 deficiency; other effects.	Leafy vegetables, pasta, bread, cereal, liver
Vitamin B12	Cyanocobalamin, Hydroxocobalamin, Methylcobalamin, Adenosylcobalamin	Water	2.4 µg/2.4 µg	Vitamin B12 deficiency anemia	None proven	Meat, poultry, fish, eggs, milk
Vitamin C	Ascorbic acid	Water	90 mg/75 mg	Scurvy	Stomach Pain, Diarrhoea and Flatulence.	Many fruits and vegetables, liver
Vitamin D	Cholecalciferol (D3), Ergocalciferol (D2)	Fat	15 µg/15 µg	Rickets and osteomalacia	Hypervitaminosis D	Eggs, liver, certain fish species such as sardines, certain mushroom species such as shiitake
Vitamin E	Tocopherols, Tocotrienols	Fat	15 mg/15 mg	Deficiency is very rare; mild hemolytic anemia in newborn infants	Possible increased incidence of congestive heart failure.	Many fruits and vegetables, nuts and seeds, and seed oils
Vitamin K	Phylloquinone, Menaquinones	Fat	AI: 110 µg/120 µg	Bleeding diathesis	Decreased anticoagulation effect of warfarin.	Leafy green vegetables such as spinach; egg yolks; liver

Classification

Vitamins are classified as either water-soluble or fat-soluble. In humans there are 13 vitamins: 4 fat-soluble (A, D, E, and K) and 9 water-soluble (8 B vitamins and vitamin C). Water-soluble vitamins dissolve easily in water and, in general, are readily excreted from the body, to the degree that urinary output is a strong predictor of vitamin consumption. Because they are not as readily stored, more consistent intake is important. Fat-soluble vitamins are absorbed through the intestinal tract with the help of lipids (fats). Vitamins A and D can accumulate in the body, which can result in dangerous hypervitaminosis. Fat-soluble vitamin deficiency due to malabsorption is of particular significance in cystic fibrosis.

Anti-vitamins

Main article: Antinutrient

Anti-vitamins are chemical compounds that inhibit the absorption or actions of vitamins. For example, avidin is a protein in raw egg whites that inhibits the absorption of biotin; it is deactivated by cooking. Pyrithiamine, a synthetic compound, has a molecular structure similar to thiamine, vitamin B₁, and inhibits the enzymes that use thiamine.

Biochemical functions

Each vitamin is typically used in multiple reactions, and therefore most have multiple functions.

On fetal growth and childhood development

Main article: Nutrition and pregnancy

Vitamins are essential for the normal growth and development of a multicellular organism. Using the genetic blueprint inherited from its parents, a fetus develops from the nutrients it absorbs. It requires certain vitamins and minerals to be present at certain times. These nutrients facilitate the chemical reactions that produce among other things, skin, bone, and muscle. If there is serious deficiency in one or more of these nutrients, a child may develop a deficiency disease. Even minor deficiencies may cause permanent damage.

On adult health maintenance

Once growth and development are completed, vitamins remain essential nutrients for the healthy maintenance of the cells, tissues, and organs that make up a multicellular organism; they also enable a multicellular life form to efficiently use chemical energy provided by food it eats, and to help process the proteins, carbohydrates, and fats required for cellular respiration.

Intake

Sources

For the most part, vitamins are obtained from the diet, but some are acquired by other means: for example, microorganisms in the gut flora produce vitamin K and biotin; and one form of vitamin D is synthesized in skin cells when they are exposed to a certain wavelength of ultraviolet light present in sunlight. Humans can produce some vitamins from precursors they consume: for example, vitamin A is synthesized from beta carotene; and niacin is synthesized from the amino acid tryptophan. Vitamin C can be synthesized by some species but not by others. Vitamin B₁₂ is the only vitamin or nutrient not available from plant sources. The Food Fortification Initiative lists countries which have mandatory fortification programs for vitamins folic acid, niacin, vitamin A and vitamins B₁, B₂ and B₁₂.

Deficient intake

See also: Vitamin deficiency

The body's stores for different vitamins vary widely; vitamins A, D, and B₁₂ are stored in significant amounts, mainly in the liver, and an adult's diet may be deficient in vitamins A and D for many months and B₁₂ in some cases for years, before developing a deficiency condition. However, vitamin B₃ (niacin and niacinamide) is not stored in significant amounts, so stores may last only a couple of weeks. For vitamin C, the first symptoms of scurvy in experimental studies of complete vitamin C deprivation in humans have varied widely, from a month to more than six months, depending on previous dietary history that determined body stores.

Deficiencies of vitamins are classified as either primary or secondary. A primary deficiency occurs when an organism does not get enough of the vitamin in its food. A secondary deficiency may be due to an underlying disorder that prevents or limits the absorption or use of the vitamin, due to a "lifestyle factor", such as smoking, excessive alcohol consumption, or the use of medications that interfere with the absorption or use of the vitamin. People who eat a varied diet are unlikely to develop a severe primary vitamin deficiency, but may be consuming less than the recommended amounts; a national food and supplement survey conducted in the US over 2003-2006 reported that over 90% of individuals who did not consume vitamin supplements were found to have inadequate levels of some of the essential vitamins, notably vitamins D and E.

Well-researched human vitamin deficiencies involve thiamine (beriberi), niacin (pellagra), vitamin C (scurvy), folate (neural tube defects) and vitamin D (rickets). In much of the developed world these deficiencies are rare due to an adequate supply of food and the addition of vitamins to common foods. In addition to these classical vitamin deficiency diseases, some evidence has also suggested links between vitamin deficiency and a number of different disorders.

Excess intake

Some vitamins have documented acute or chronic toxicity at larger intakes, which is referred to as hypertoxicity. The European Union and the governments of several countries have established Tolerable upper intake levels (ULs) for those vitamins which have documented toxicity (see table). The likelihood of consuming too much of any vitamin from food is remote, but excessive intake (vitamin poisoning) from dietary supplements does occur. In 2016, overdose exposure to all formulations of vitamins and multi-vitamin/mineral formulations was reported by 63,931 individuals to the American Association of Poison Control Centers with 72% of these exposures in children under the age of five. In the US, analysis of a national diet and supplement survey reported that about 7% of adult supplement users exceeded the UL for folate and 5% of those older than age 50 years exceeded the UL for vitamin A.

Effects of cooking

The USDA has conducted extensive studies on the percentage losses of various nutrients from food types and cooking methods. Some vitamins may become more "bio-available" – that is, usable by the body – when foods are cooked. The table below shows whether various vitamins are susceptible to loss from heat—such as heat from boiling, steaming, frying, etc. The effect of cutting vegetables can be seen from exposure to air and light. Water-soluble vitamins such as B and C dissolve into the water when a vegetable is boiled, and are then lost when the water is discarded.

Vitamin	Is substance susceptible to losses under given condition?
	Soluble in Water	Air Exposure	Light Exposure	Heat Exposure
Vitamin A	no	partially	partially	relatively stable
Vitamin C	very unstable	yes	no	no
Vitamin D	no	no	no	no
Vitamin E	no	yes	yes	no
Vitamin K	no	no	yes	no
Thiamine (B1)	highly	no	?	> 100 °C
Riboflavin (B2)	slightly	no	in solution	no
Niacin (B3)	yes	no	no	no
Pantothenic Acid (B5)	quite stable	no	no	yes
Vitamin B6	yes	?	yes	< 160 °C
Biotin (B7)	somewhat	?	?	no
Folic Acid (B9)	yes	?	when dry	at high temp
Cobalamin (B12)	yes	?	yes	no

Recommended levels

In setting human nutrient guidelines, government organizations do not necessarily agree on amounts needed to avoid deficiency or maximum amounts to avoid the risk of toxicity. For example, for vitamin C, recommended intakes range from 40 mg/day in India to 155 mg/day for the European Union. The table below shows U.S. Estimated Average Requirements (EARs) and Recommended Dietary Allowances (RDAs) for vitamins, PRIs for the European Union (same concept as RDAs), followed by what three government organizations deem to be the safe upper intake. RDAs are set higher than EARs to cover people with higher than average needs. Adequate Intakes (AIs) are set when there is not sufficient information to establish EARs and RDAs. Governments are slow to revise information of this nature. For the U.S. values, with the exception of calcium and vitamin D, all of the data date to 1997–2004.

All values are consumption per day:

Nutrient	U.S. EAR	Highest U.S. RDA or AI	Highest EU PRI or AI	Upper limit (UL)			Unit
				U.S.	EU	Japan
Vitamin A	625	900	1300	3000	3000	2700	µg
Vitamin C	75	90	155	2000	ND	ND	mg
Vitamin D	10	15	15	100	100	100	µg
Vitamin K	NE	120	70	ND	ND	ND	µg
α-tocopherol (Vitamin E)	12	15	13	1000	300	650-900	mg
Thiamin (Vitamin B1)	1.0	1.2	0.1 mg/MJ	ND	ND	ND	mg
Riboflavin (Vitamin B2)	1.1	1.3	2.0	ND	ND	ND	mg
Niacin (Vitamin B3)	12	16	1.6 mg/MJ	35	10	60-85	mg
Pantothenic acid (Vitamin B5)	NE	5	7	ND	ND	ND	mg
Vitamin B6	1.1	1.3	1.8	100	25	40-60	mg
Biotin (Vitamin B7)	NE	30	45	ND	ND	ND	µg
Folate (Vitamin B9)	320	400	600	1000	1000	900-1000	µg
Cyanocobalamin (Vitamin B12)	2.0	2.4	5.0	ND	ND	ND	µg

EAR US Estimated Average Requirements.

RDA US Recommended Dietary Allowances; higher for adults than for children, and may be even higher for women who are pregnant or lactating.

AI US and EFSA Adequate Intake; AIs established when there is not sufficient information to set EARs and RDAs.

PRI Population Reference Intake is European Union equivalent of RDA; higher for adults than for children, and may be even higher for women who are pregnant or lactating. For Thiamin and Niacin the PRIs are expressed as amounts per MJ of calories consumed. MJ = megajoule = 239 food calories.

UL or Upper Limit Tolerable upper intake levels.

ND ULs have not been determined.

NE EARs have not been established.

Supplementation

Calcium combined with vitamin D (as calciferol) supplement tablets with fillers.

In those who are otherwise healthy, there is little evidence that supplements have any benefits with respect to cancer or heart disease. Vitamin A and E supplements not only provide no health benefits for generally healthy individuals, but they may increase mortality, though the two large studies that support this conclusion included smokers for whom it was already known that beta-carotene supplements can be harmful. A 2018 meta-analysis found no evidence that intake of vitamin D or calcium for community-dwelling elderly people reduced bone fractures.

Europe has regulations that define limits of vitamin (and mineral) dosages for their safe use as dietary supplements. Most vitamins that are sold as dietary supplements are not supposed to exceed a maximum daily dosage referred to as the tolerable upper intake level (UL or Upper Limit). Vitamin products above these regulatory limits are not considered supplements and should be registered as prescription or non-prescription (over-the-counter drugs) due to their potential side effects. The European Union, United States and Japan establish ULs.

Dietary supplements often contain vitamins, but may also include other ingredients, such as minerals, herbs, and botanicals. Scientific evidence supports the benefits of dietary supplements for persons with certain health conditions. In some cases, vitamin supplements may have unwanted effects, especially if taken before surgery, with other dietary supplements or medicines, or if the person taking them has certain health conditions. They may also contain levels of vitamins many times higher, and in different forms, than one may ingest through food.

See also: Megavitamin therapy

Governmental regulation

Most countries place dietary supplements in a special category under the general umbrella of foods, not drugs. As a result, the manufacturer, and not the government, has the responsibility of ensuring that its dietary supplement products are safe before they are marketed. Regulation of supplements varies widely by country. In the United States, a dietary supplement is defined under the Dietary Supplement Health and Education Act of 1994. There is no FDA approval process for dietary supplements, and no requirement that manufacturers prove the safety or efficacy of supplements introduced before 1994. The Food and Drug Administration must rely on its Adverse Event Reporting System to monitor adverse events that occur with supplements.

In 2007, the US Code of Federal Regulations (CFR) Title 21, part III took effect, regulating Good Manufacturing Practices (GMPs) in the manufacturing, packaging, labeling, or holding operations for dietary supplements. Even though product registration is not required, these regulations mandate production and quality control standards (including testing for identity, purity and adulterations) for dietary supplements. In the European Union, the Food Supplements Directive requires that only those supplements that have been proven safe can be sold without a prescription. For most vitamins, pharmacopoeial standards have been established. In the United States, the United States Pharmacopeia (USP) sets standards for the most commonly used vitamins and preparations thereof. Likewise, monographs of the European Pharmacopoeia (Ph.Eur.) regulate aspects of identity and purity for vitamins on the European market.

Naming

Nomenclature of reclassified vitamins

Previous name	Chemical name	Reason for name change
Vitamin B4	Adenine	DNA metabolite; synthesized in body
Vitamin B8	Adenylic acid	DNA metabolite; synthesized in body
Vitamin BT	Carnitine	Synthesized in body
Vitamin F	Essential fatty acids	Needed in large quantities (does not fit the definition of a vitamin).
Vitamin G	Riboflavin	Reclassified as Vitamin B2
Vitamin H	Biotin	Reclassified as Vitamin B7
Vitamin J	Catechol, Flavin	Catechol nonessential; flavin reclassified as Vitamin B2
Vitamin L1	Anthranilic acid	Nonessential
Vitamin L2	5′-Methylthioadenosine	RNA metabolite; synthesized in body
Vitamin M or Bc	Folate	Reclassified as Vitamin B9
Vitamin P	Flavonoids	Many compounds, not proven essential
Vitamin PP	Niacin	Reclassified as Vitamin B3
Vitamin S	Salicylic acid	Nonessential
Vitamin U	S-Methylmethionine	Protein metabolite; synthesized in body

The reason that the set of vitamins skips directly from E to K is that the vitamins corresponding to letters F–J were either reclassified over time, discarded as false leads, or renamed because of their relationship to vitamin B, which became a complex of vitamins.

The Danish-speaking scientists who isolated and described vitamin K (in addition to naming it as such) did so because the vitamin is intimately involved in the coagulation of blood following wounding (from the Danish word Koagulation). At the time, most (but not all) of the letters from F through to J were already designated, so the use of the letter K was considered quite reasonable. The table Nomenclature of reclassified vitamins lists chemicals that had previously been classified as vitamins, as well as the earlier names of vitamins that later became part of the B-complex.

The missing B vitamins were reclassified or determined not to be vitamins. For example, B₉ is folic acid and five of the folates are in the range B₁₁ through B₁₆. Others, such as PABA (formerly B₁₀), are biologically inactive, toxic, or with unclassifiable effects in humans, or not generally recognised as vitamins by science, such as the highest-numbered, which some naturopath practitioners call B₂₁ and B₂₂. There are also nine lettered B complex vitamins (e.g., B_m). There are other D vitamins now recognised as other substances, which some sources of the same type number up to D₇. The controversial cancer treatment laetrile was at one point lettered as vitamin B₁₇. There appears to be no consensus on any vitamins Q, R, T, V, W, X, Y or Z, nor are there substances officially designated as vitamins N or I, although the latter may have been another form of one of the other vitamins or a known and named nutrient of another type.

History

The value of eating certain foods to maintain health was recognized long before vitamins were identified. The ancient Egyptians knew that feeding liver to a person may help with night blindness, an illness now known to be caused by a vitamin A deficiency. The advancement of ocean voyages during the Age of Discovery resulted in prolonged periods without access to fresh fruits and vegetables, and made illnesses from vitamin deficiency common among ships' crews.

The discovery dates of the vitamins and their sources

Year of discovery	Vitamin	Food source
1913	Vitamin A (Retinol)	Cod liver oil
1910	Vitamin B1 (Thiamine)	Rice bran
1920	Vitamin C (Ascorbic acid)	Citrus, most fresh foods
1920	Vitamin D (Calciferol)	Cod liver oil
1920	Vitamin B2 (Riboflavin)	Meat, dairy products, eggs
1922	Vitamin E (Tocopherol)	Wheat germ oil, unrefined vegetable oils
1929	Vitamin K1 (Phylloquinone)	Leaf vegetables
1931	Vitamin B5 (Pantothenic acid)	Meat, whole grains, in many foods
1934	Vitamin B6 (Pyridoxine)	Meat, dairy products
1936	Vitamin B7 (Biotin)[65]	Meat, dairy products, Eggs
1936	Vitamin B3 (Niacin)	Meat, grains
1941	Vitamin B9 (Folic acid)	Leaf vegetables
1948	Vitamin B12 (Cobalamins)	Meat, organs (Liver), Eggs

In 1747, the Scottish surgeon James Lind discovered that citrus foods helped prevent scurvy, a particularly deadly disease in which collagen is not properly formed, causing poor wound healing, bleeding of the gums, severe pain, and death. In 1753, Lind published his Treatise on the Scurvy, which recommended using lemons and limes to avoid scurvy, which was adopted by the British Royal Navy. This led to the nickname limey for British sailors. Lind's discovery, however, was not widely accepted by individuals in the Royal Navy's Arctic expeditions in the 19th century, where it was widely believed that scurvy could be prevented by practicing good hygiene, regular exercise, and maintaining the morale of the crew while on board, rather than by a diet of fresh food. As a result, Arctic expeditions continued to be plagued by scurvy and other deficiency diseases. In the early 20th century, when Robert Falcon Scott made his two expeditions to the Antarctic, the prevailing medical theory at the time was that scurvy was caused by "tainted" canned food.

During the late 18th and early 19th centuries, the use of deprivation studies allowed scientists to isolate and identify a number of vitamins. Lipid from fish oil was used to cure rickets in rats, and the fat-soluble nutrient was called "antirachitic A". Thus, the first "vitamin" bioactivity ever isolated, which cured rickets, was initially called "vitamin A"; however, the bioactivity of this compound is now called vitamin D. In 1881, Russian medical doctor Nikolai I. Lunin [ru] studied the effects of scurvy at the University of Tartu. He fed mice an artificial mixture of all the separate constituents of milk known at that time, namely the proteins, fats, carbohydrates, and salts. The mice that received only the individual constituents died, while the mice fed by milk itself developed normally. He made a conclusion that "a natural food such as milk must therefore contain, besides these known principal ingredients, small quantities of unknown substances essential to life." However, his conclusions were rejected by his advisor, Gustav von Bunge. A similar result by Cornelius Pekelharing appeared in a Dutch medical journal in 1905, but it was not widely reported.

In East Asia, where polished white rice was the common staple food of the middle class, beriberi resulting from lack of vitamin B₁ was endemic. In 1884, Takaki Kanehiro, a British-trained medical doctor of the Imperial Japanese Navy, observed that beriberi was endemic among low-ranking crew who often ate nothing but rice, but not among officers who consumed a Western-style diet. With the support of the Japanese navy, he experimented using crews of two battleships; one crew was fed only white rice, while the other was fed a diet of meat, fish, barley, rice, and beans. The group that ate only white rice documented 161 crew members with beriberi and 25 deaths, while the latter group had only 14 cases of beriberi and no deaths. This convinced Takaki and the Japanese Navy that diet was the cause of beriberi, but they mistakenly believed that sufficient amounts of protein prevented it. That diseases could result from some dietary deficiencies was further investigated by Christiaan Eijkman, who in 1897 discovered that feeding unpolished rice instead of the polished variety to chickens helped to prevent a kind of polyneuritis that was the equivalent of beriberi. The following year, Frederick Hopkins postulated that some foods contained "accessory factors" — in addition to proteins, carbohydrates, fats etc. — that are necessary for the functions of the human body.

Jack Drummond's single-paragraph article in 1920 which provided structure and nomenclature used today for vitamins

"Vitamine" to vitamin

In 1910, the first vitamin complex was isolated by Japanese scientist Umetaro Suzuki, who succeeded in extracting a water-soluble complex of micronutrients from rice bran and named it aberic acid (later Orizanin). He published this discovery in a Japanese scientific journal. When the article was translated into German, the translation failed to state that it was a newly discovered nutrient, a claim made in the original Japanese article, and hence his discovery failed to gain publicity. In 1912 Polish-born biochemist Casimir Funk, working in London, isolated the same complex of micronutrients and proposed the complex be named "vitamine". It was later to be known as vitamin B₃ (niacin), though he described it as "anti-beri-beri-factor" (which would today be called thiamine or vitamin B₁). Funk proposed the hypothesis that other diseases, such as rickets, pellagra, coeliac disease, and scurvy could also be cured by vitamins. Max Nierenstein a friend and Reader of Biochemistry at Bristol University reportedly suggested the "vitamine" name (from "vital amine"). The name soon became synonymous with Hopkins' "accessory factors", and, by the time it was shown that not all vitamins are amines, the word was already ubiquitous. In 1920, Jack Cecil Drummond proposed that the final "e" be dropped to deemphasize the "amine" reference, hence "vitamin," after researchers began to suspect that not all "vitamines" (in particular, vitamin A) have an amine component.

Nobel Prizes for vitamin research

The Nobel Prize for Chemistry for 1928 was awarded to Adolf Windaus "for his studies on the constitution of the sterols and their connection with vitamins", the first person to receive an award mentioning vitamins, even though it was not specifically about vitamin D.

The Nobel Prize in Physiology or Medicine for 1929 was awarded to Christiaan Eijkman and Frederick Gowland Hopkins for their contributions to the discovery of vitamins. Thirty-five years earlier, Eijkman had observed that chickens fed polished white rice developed neurological symptoms similar to those observed in military sailors and soldiers fed a rice-based diet, and that the symptoms were reversed when the chickens were switched to whole-grain rice. He called this "the anti-beriberi factor", which was later identified as vitamin B₁, thiamine.

In 1930, Paul Karrer elucidated the correct structure for beta-carotene, the main precursor of vitamin A, and identified other carotenoids. Karrer and Norman Haworth confirmed Albert Szent-Györgyi's discovery of ascorbic acid and made significant contributions to the chemistry of flavins, which led to the identification of lactoflavin. For their investigations on carotenoids, flavins and vitamins A and B₂, they both received the Nobel Prize in Chemistry in 1937.

In 1931, Albert Szent-Györgyi and a fellow researcher Joseph Svirbely suspected that "hexuronic acid" was actually vitamin C, and gave a sample to Charles Glen King, who proved its anti-scorbutic activity in his long-established guinea pig scorbutic assay. In 1937, Szent-Györgyi was awarded the Nobel Prize in Physiology or Medicine for his discovery. In 1943, Edward Adelbert Doisy and Henrik Dam were awarded the Nobel Prize in Physiology or Medicine for their discovery of vitamin K and its chemical structure.

In 1938, Richard Kuhn was awarded the Nobel Prize in Chemistry for his work on carotenoids and vitamins, specifically B₂ and B₆.

Five people have been awarded Nobel Prizes for direct and indirect studies of vitamin B₁₂: George Whipple, George Minot and William P. Murphy (1934), Alexander R. Todd (1957), and Dorothy Hodgkin (1964).

In 1967, George Wald, Ragnar Granit and Haldan Keffer Hartline were awarded the Nobel Prize in Physiology and Medicine "...for their discoveries concerning the primary physiological and chemical visual processes in the eye." Wald's contribution was discovering the role vitamin A had in the process.

History of promotional marketing

Once discovered, vitamins were actively promoted in articles and advertisements in McCall's, Good Housekeeping, and other media outlets. Marketers enthusiastically promoted cod-liver oil, a source of vitamin D, as "bottled sunshine", and bananas as a "natural vitality food". They promoted foods such as yeast cakes, a source of B vitamins, on the basis of scientifically determined nutritional value, rather than taste or appearance. In 1942, when flour enrichment with nicotinic acid began, a headline in the popular press said "Tobacco in Your Bread." In response, the Council on Foods and Nutrition of the American Medical Association approved of the Food and Nutrition Board's new names niacin and niacin amide for use primarily by non-scientists. It was thought appropriate to choose a name to dissociate nicotinic acid from nicotine, to avoid the perception that vitamins or niacin-rich food contains nicotine, or that cigarettes contain vitamins. The resulting name niacin was derived from nicotinic acid + vitamin. Researchers also focused on the need to ensure adequate nutrition, especially to compensate for what was lost in the manufacture of processed foods.

Robert W. Yoder is credited with first using the term vitamania, in 1942, to describe the appeal of relying on nutritional supplements rather than on obtaining vitamins from a varied diet of foods. The continuing preoccupation with a healthy lifestyle led to an obsessive consumption of vitamins and multi-vitamins, the beneficial effects of which are questionable. As one example, in the 1950s, the Wonder Bread company sponsored the Howdy Doody television show, with host Buffalo Bob Smith telling the audience, "Wonder Bread builds strong bodies 8 ways", referring to the number of added nutrients.