Fermat's principle

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Fermat%27s_principle 

 

Fig. 1: Fermat's principle in the case of refraction of light at a flat surface between (say) air and water. Given an object-point A in the air, and an observation point B in the water, the refraction point P is that which minimizes the time taken by the light to travel the path APB. If we seek the required value of x, we find that the angles α and β satisfy Snell's law.

Fermat's principle, also known as the principle of least time, is the link between ray optics and wave optics. In its original "strong" form, Fermat's principle states that the path taken by a ray between two given points is the path that can be traveled in the least time. In order to be true in all cases, this statement must be weakened by replacing the "least" time with a time that is "stationary" with respect to variations of the path — so that a deviation in the path causes, at most, a second-order change in the traversal time. To put it loosely, a ray path is surrounded by close paths that can be traversed in very close times. It can be shown that this technical definition corresponds to more intuitive notions of a ray, such as a line of sight or the path of a narrow beam.

First proposed by the French mathematician Pierre de Fermat in 1662, as a means of explaining the ordinary law of refraction of light (Fig. 1), Fermat's principle was initially controversial because it seemed to ascribe knowledge and intent to nature. Not until the 19th century was it understood that nature's ability to test alternative paths is merely a fundamental property of waves. If points A and B are given, a wavefront expanding from A sweeps all possible ray paths radiating from A, whether they pass through B or not. If the wavefront reaches point B, it sweeps not only the ray path(s) from A to B, but also an infinitude of nearby paths with the same endpoints. Fermat's principle describes any ray that happens to reach point B; there is no implication that the ray "knew" the quickest path or "intended" to take that path.

Fig. 2: Two points P and P′ on a path from A to B. For the purposes of Fermat's principle, the propagation time from P to P′ is taken as for a point-source at P, not (e.g.) for an arbitrary wavefront W passing through P. The surface Σ  (with unit normal n̂ at P′) is the locus of points that a disturbance at P can reach in the same time that it takes to reach P′; in other words, Σ is the secondary wavefront with radius PP′. (The medium is not assumed to be homogeneous or isotropic.)

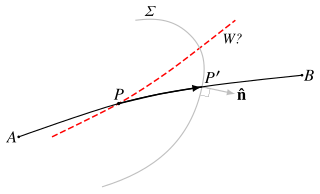
For the purpose of comparing traversal times, the time from one point to the next nominated point is taken as if the first point were a point-source. Without this condition, the traversal time would be ambiguous; for example, if the propagation time from P to P′ were reckoned from an arbitrary wavefront W containing P  (Fig. 2), that time could be made arbitrarily small by suitably angling the wavefront.

Treating a point on the path as a source is the minimum requirement of Huygens' principle, and is part of the explanation of Fermat's principle. But it can also be shown that the geometric construction by which Huygens tried to apply his own principle (as distinct from the principle itself) is simply an invocation of Fermat's principle. Hence all the conclusions that Huygens drew from that construction — including, without limitation, the laws of rectilinear propagation of light, ordinary reflection, ordinary refraction, and the extraordinary refraction of "Iceland crystal" (calcite) — are also consequences of Fermat's principle.

Derivation

Sufficient conditions

Let us suppose that:

1. A disturbance propagates sequentially through a medium (a vacuum or some material, not necessarily homogeneous or isotropic), without action at a distance;
2. During propagation, the influence of the disturbance at any intermediate point P upon surrounding points has a non-zero angular spread (as if P were a source), so that a disturbance originating at any point A arrives at any other point B via an infinitude of paths, by which B receives an infinitude of delayed versions of the disturbance at A; and
3. These delayed versions of the disturbance will reinforce each other at B if they are synchronized within some tolerance.

Then the various propagation paths from A to B will help each other if their traversal times agree within the said tolerance. For a small tolerance (in the limiting case), the permissible range of variations of the path is maximized if the path is such that its traversal time is stationary with respect to the variations, so that a variation of the path causes at most a second-order change in the traversal time.

The most obvious example of a stationarity in traversal time is a (local or global) minimum — that is, a path of least time, as in the "strong" form of Fermat's principle. But that condition is not essential to the argument.

Having established that a path of stationary traversal time is reinforced by a maximally wide corridor of neighboring paths, we still need to explain how this reinforcement corresponds to intuitive notions of a ray. But, for brevity in the explanations, let us first define a ray path as a path of stationary traversal time.

A ray as a signal path (line of sight)

If the corridor of paths reinforcing a ray path from A to B is substantially obstructed, this will significantly alter the disturbance reaching B from A — unlike a similar-sized obstruction outside any such corridor, blocking paths that do not reinforce each other. The former obstruction will significantly disrupt the signal reaching B from A, while the latter will not; thus the ray path marks a signal path. If the signal is visible light, the former obstruction will significantly affect the appearance of an object at A as seen by an observer at B, while the latter will not; so the ray path marks a line of sight.

In optical experiments, a line of sight is routinely assumed to be a ray path.

A ray as an energy path (beam)

Fig. 3: An experiment demonstrating refraction (and partial reflection) of rays — approximated by, or contained in, narrow beams

If the corridor of paths reinforcing a ray path from A to B is substantially obstructed, this will significantly affect the energy reaching B from A — unlike a similar-sized obstruction outside any such corridor. Thus the ray path marks an energy path — as does a beam.

Suppose that a wavefront expanding from point A passes point P, which lies on a ray path from point A to point B. By definition, all points on the wavefront have the same propagation time from A. Now let the wavefront be blocked except for a window, centered on P, and small enough to lie within the corridor of paths that reinforce the ray path from A to B. Then all points on the unobstructed portion of the wavefront will have, nearly enough, equal propagation times to B, but not to points in other directions, so that B will be in the direction of peak intensity of the beam admitted through the window. So the ray path marks the beam. And in optical experiments, a beam is routinely considered as a collection of rays or (if it is narrow) as an approximation to a ray (Fig. 3).

Analogies

According to the "strong" form of Fermat's principle, the problem of finding the path of a light ray from point A in a medium of faster propagation, to point B in a medium of slower propagation (Fig. 1), is analogous to the problem faced by a lifeguard in deciding where to enter the water in order to reach a drowning swimmer as soon as possible, given that the lifeguard can run faster than (s)he can swim. But that analogy falls short of explaining the behavior of the light, because the lifeguard can think about the problem (even if only for an instant) whereas the light presumably cannot. The discovery that ants are capable of similar calculations does not bridge the gap between the animate and the inanimate.

In contrast, the above assumptions (1) to (3) hold for any wavelike disturbance and explain Fermat's principle in purely mechanistic terms, without any imputation of knowledge or purpose.

The principle applies to waves in general, including (e.g.) sound waves in fluids and elastic waves in solids. In a modified form, it even works for matter waves: in quantum mechanics, the classical path of a particle is obtainable by applying Fermat's principle to the associated wave — except that, because the frequency may vary with the path, the stationarity is in the phase shift (or number of cycles) and not necessarily in the time.

Fermat's principle is most familiar, however, in the case of visible light: it is the link between geometrical optics, which describes certain optical phenomena in terms of rays, and the wave theory of light, which explains the same phenomena on the hypothesis that light consists of waves.

Equivalence to Huygens' construction

Fig. 4: Two iterations of Huygens' construction. In the first iteration, the later wavefront W′ is derived from the earlier wavefront W by taking the envelope of all the secondary wavefronts (gray arcs) expanding in a given time from all the points (e.g., P) on W. The arrows show the ray directions.

In this article we distinguish between Huygens' principle, which states that every point crossed by a traveling wave becomes the source of a secondary wave, and Huygens' construction, which is described below.

Let the surface W be a wavefront at time t, and let the surface W′ be the same wavefront at the later time t + Δt (Fig. 4). Let P be a general point on W. Then, according to Huygens' construction,

1. W′ is the envelope (common tangent surface), on the forward side of W, of all the secondary wavefronts each of which would expand in time Δt from a point on W, and
2. if the secondary wavefront expanding from point P in time Δt touches the surface W′ at point P′, then P and P′ lie on a ray.

The construction may be repeated in order to find successive positions of the primary wavefront, and successive points on the ray.

The ray direction given by this construction is the radial direction of the secondary wavefront, and may differ from the normal of the secondary wavefront (cf. Fig. 2), and therefore from the normal of the primary wavefront at the point of tangency. Hence the ray velocity, in magnitude and direction, is the radial velocity of an infinitesimal secondary wavefront, and is generally a function of location and direction.

Now let Q be a point on W close to P, and let Q′ be a point on W′ close to P′. Then, by the construction,

1.   the time taken for a secondary wavefront from P to reach Q′ has at most a second-order dependence on the displacement P′Q′, and
2. the time taken for a secondary wavefront to reach P′ from Q has at most a second-order dependence on the displacement PQ.

By (i), the ray path is a path of stationary traversal time from P to W′; and by (ii), it is a path of stationary traversal time from a point on W to P′.

So Huygens' construction implicitly defines a ray path as a path of stationary traversal time between successive positions of a wavefront, the time being reckoned from a point-source on the earlier wavefront. This conclusion remains valid if the secondary wavefronts are reflected or refracted by surfaces of discontinuity in the properties of the medium, provided that the comparison is restricted to the affect paths and the affected portions of the wavefronts.

Fermat's principle, however, is conventionally expressed in point-to-point terms, not wavefront-to-wavefront terms. Accordingly, let us modify the example by supposing that the wavefront which becomes surface W at time t, and which becomes surface W′ at the later time t + Δt, is emitted from point A at time 0. Let P be a point on W (as before), and B a point on W′. And let A, W, W′, and B be given, so that the problem is to find P.

If P satisfies Huygens' construction, so that the secondary wavefront from P is tangential to W′ at B, then PB is a path of stationary traversal time from W to B. Adding the fixed time from A to W, we find that APB is the path of stationary traversal time from A to B (possibly with a restricted domain of comparison, as noted above), in accordance with Fermat's principle. The argument works just as well in the converse direction, provided that W′ has a well-defined tangent plane at B. Thus Huygens' construction and Fermat's principle are geometrically equivalent.

Through this equivalence, Fermat's principle sustains Huygens' construction and thence all the conclusions that Huygens was able to draw from that construction. In short, "The laws of geometrical optics may be derived from Fermat's principle". With the exception of the Fermat-Huygens principle itself, these laws are special cases in the sense that they depend on further assumptions about the media. Two of them are mentioned under the next heading.

Special cases

Isotropic media: Rays normal to wavefronts

In an isotropic medium, because the propagation speed is independent of direction, the secondary wavefronts that expand from points on a primary wavefront in a given infinitesimal time are spherical, so that their radii are normal to their common tangent surface at the points of tangency. But their radii mark the ray directions, and their common tangent surface is a general wavefront. Thus the rays are normal (orthogonal) to the wavefronts.

Because much of the teaching of optics concentrates on isotropic media, treating anisotropic media as an optional topic, the assumption that the rays are normal to the wavefronts can become so pervasive that even Fermat's principle is explained under that assumption, although in fact Fermat's principle is more general.

Homogeneous media: Rectilinear propagation

In a homogeneous medium (also called a uniform medium), all the secondary wavefronts that expand from a given primary wavefront W in a given time Δt are congruent and similarly oriented, so that their envelope W′ may be considered as the envelope of a single secondary wavefront which preserves its orientation while its center (source) moves over W. If P is its center while P′ is its point of tangency with W′, then P′ moves parallel to P, so that the plane tangential to W′ at P′ is parallel to the plane tangential to W at P. Let another (congruent and similarly orientated) secondary wavefront be centered on P′, moving with P, and let it meet its envelope W″ at point P″. Then, by the same reasoning, the plane tangential to W″ at P″ is parallel to the other two planes. Hence, due to the congruence and similar orientations, the ray directions PP′ and P′P″ are the same (but not necessarily normal to the wavefronts, since the secondary wavefronts are not necessarily spherical). This construction can be repeated any number of times, giving a straight ray of any length. Thus a homogeneous medium admits rectilinear rays.

Modern version

Formulation in terms of refractive index

Let a path Γ extend from point A to point B. Let s be the arc length measured along the path from A, and let t be the time taken to traverse that arc length at the ray speed ${\displaystyle v_{\mathrm {r} }}$ (that is, at the radial speed of the local secondary wavefront, for each location and direction on the path). Then the traversal time of the entire path Γ is

$${\displaystyle T=\int _{A}^{B}\!dt=\int _{A}^{B}{\frac {ds}{\,v_{\mathrm {r} }\,}}}$$

(1)

(where A and B simply denote the endpoints and are not to be construed as values of t or s). The condition for Γ to be a ray path is that the first-order change in T due to a change in Γ is zero; that is,

$${\displaystyle \delta T=\,\delta \int _{A}^{B}{\frac {ds}{\,v_{\mathrm {r} }\,}}\,=\,0\,.}$$

Now let us define the optical length of a given path (optical path length, OPL) as the distance traversed by a ray in a homogeneous isotropic reference medium (e.g., a vacuum) in the same time that it takes to traverse the given path at the local ray velocity.^[24] Then, if c denotes the propagation speed in the reference medium (e.g., the speed of light in a vacuum), the optical length of a path traversed in time dt is dS = c dt, and the optical length of a path traversed in time T is S = cT.  So, multiplying equation (1) through by c, we obtain

$${\displaystyle S\,=\int _{A}^{B}\!dS\,=\int _{A}^{B}{\frac {\,c\,}{v_{\mathrm {r} }}}\,ds=\int _{A}^{B}\!n_{\mathrm {r} }\,ds\,,}$$

where ${\displaystyle \,n_{\mathrm {r} }=c/v_{\mathrm {r} }\,}$ is the ray index — that is, the refractive index calculated on the ray velocity instead of the usual phase velocity (wave-normal velocity). For an infinitesimal path, we have  ${\displaystyle dS=n_{\mathrm {r} \,}ds\,,\,}$ indicating that the optical length is the physical length multiplied by the ray index: the OPL is a notional geometric quantity, from which time has been factored out. In terms of OPL, the condition for Γ to be a ray path (Fermat's principle) becomes

$${\displaystyle \delta S=\delta \int _{A}^{B}\!n_{\mathrm {r} }\,ds=0.}$$

(2)

This has the form of Maupertuis's principle in classical mechanics (for a single particle), with the ray index in optics taking the role of momentum or velocity in mechanics.

In an isotropic medium, for which the ray velocity is also the phase velocity, we may substitute the usual refractive index n for n_r. 

Relation to Hamilton's principle

If x,y,z are Cartesian coordinates and an overdot denotes differentiation with respect to s , Fermat's principle (2) may be written

$${\displaystyle {\begin{aligned}\delta S&=\,\delta \int _{A}^{B}\!n_{\mathrm {r} }\,{\sqrt {dx^{2}+dy^{2}+dz^{2}}}\\&=\,\delta \int _{A}^{B}\!n_{\mathrm {r} }\,{\sqrt {{\dot {x}}^{2}+{\dot {y}}^{2}+{\dot {z}}^{2}}}~ds\,=\,0\,.\end{aligned}}}$$

In the case of an isotropic medium, we may replace n_r with the normal refractive index  n(x,y,z), which is simply a scalar field. If we then define the optical Lagrangian as

$${\displaystyle L(x,y,z,{\dot {x}},{\dot {y}},{\dot {z}})\,=\,n(x,y,z)\,{\sqrt {{\dot {x}}^{2}+{\dot {y}}^{2}+{\dot {z}}^{2}}}\,,}$$

Fermat's principle becomes

$${\displaystyle \delta S=\,\delta \int _{A}^{B}\!L(x,y,z,{\dot {x}},{\dot {y}},{\dot {z}})\,ds\,=\,0\,.}$$

If the direction of propagation is always such that we can use z instead of s as the parameter of the path (and the overdot to denote differentiation w.r.t. z instead of s), the optical Lagrangian can instead be written

$${\displaystyle L{\big (}x(z),y(z),{\dot {x}}(z),{\dot {y}}(z),z{\big )}=n(x,y,z)\,{\sqrt {1+{\dot {x}}^{2}+{\dot {y}}^{2}}}\,,}$$

so that Fermat's principle becomes

$${\displaystyle \delta S=\,\delta \int _{A}^{B}\!L{\big (}x(z),y(z),{\dot {x}}(z),{\dot {y}}(z),z{\big )}\,dz\,=\,0.}$$

This has the form of Hamilton's principle in classical mechanics, except that the time dimension is missing: the third spatial coordinate in optics takes the role of time in mechanics. The optical Lagrangian is the function which, when integrated w.r.t. the parameter of the path, yields the OPL; it is the foundation of Lagrangian and Hamiltonian optics.

History

Fermat vs. the Cartesians

Pierre de Fermat (1607 –1665)

If a ray follows a straight line, it obviously takes the path of least length. Hero of Alexandria, in his Catoptrics (1st century CE), showed that the ordinary law of reflection off a plane surface follows from the premise that the total length of the ray path is a minimum. In 1657, Pierre de Fermat received from Marin Cureau de la Chambre a copy of newly published treatise, in which La Chambre noted Hero's principle and complained that it did not work for refraction.

Fermat replied that refraction might be brought into the same framework by supposing that light took the path of least resistance, and that different media offered different resistances. His eventual solution, described in a letter to La Chambre dated 1 January 1662, construed "resistance" as inversely proportional to speed, so that light took the path of least time. That premise yielded the ordinary law of refraction, provided that light traveled more slowly in the optically denser medium.

Fermat's solution was a landmark in that it unified the then-known laws of geometrical optics under a variational principle or action principle, setting the precedent for the principle of least action in classical mechanics and the corresponding principles in other fields (see History of variational principles in physics). It was the more notable because it used the method of adequality, which may be understood in retrospect as finding the point where the slope of an infinitesimally short chord is zero, without the intermediate step of finding a general expression for the slope (the derivative).

It was also immediately controversial. The ordinary law of refraction was at that time attributed to René Descartes (d. 1650), who had tried to explain it by supposing that light was a force that propagated instantaneously, or that light was analogous to a tennis ball that traveled faster in the denser medium, either premise being inconsistent with Fermat's.  Descartes' most prominent defender, Claude Clerselier, criticized Fermat for apparently ascribing knowledge and intent to nature, and for failing to explain why nature should prefer to economize on time rather than distance. Clerselier wrote in part:

1. The principle that you take as the basis of your demonstration, namely that nature always acts in the shortest and simplest ways, is merely a moral principle and not a physical one; it is not, and cannot be, the cause of any effect in nature.... For otherwise we would attribute knowledge to nature; but here, by "nature", we understand only this order and this law established in the world as it is, which acts without foresight, without choice, and by a necessary determination.

2. This same principle would make nature irresolute... For I ask you... when a ray of light must pass from a point in a rare medium to a point in a dense one, is there not reason for nature to hesitate if, by your principle, it must choose the straight line as soon as the bent one, since if the latter proves shorter in time, the former is shorter and simpler in length? Who will decide and who will pronounce? 

Fermat, being unaware of the mechanistic foundations of his own principle, was not well placed to defend it, except as a purely geometric and kinematic proposition.  The wave theory of light, first proposed by Robert Hooke in the year of Fermat's death, and rapidly improved by Ignace-Gaston Pardies and (especially) Christiaan Huygens, contained the necessary foundations; but the recognition of this fact was surprisingly slow.

Huygens's oversight

Christiaan Huygens (1629–1695)

Huygens repeatedly referred to the envelope of his secondary wavefronts as the termination of the movement, meaning that the later wavefront was the outer boundary that the disturbance could reach in a given time, which was therefore the minimum time in which each point on the later wavefront could be reached. But he did not argue that the direction of minimum time was that from the secondary source to the point of tangency; instead, he deduced the ray direction from the extent of the common tangent surface corresponding to a given extent of the initial wavefront. His only endorsement of Fermat's principle was limited in scope: having derived the law of ordinary refraction, for which the rays are normal to the wavefronts, Huygens gave a geometric proof that a ray refracted according to this law takes the path of least time. He would hardly have thought this necessary if he had known that the principle of least time followed directly from the same common-tangent construction by which he had deduced not only the law of ordinary refraction, but also the laws of rectilinear propagation and ordinary reflection (which were also known to follow from Fermat's principle), and a previously unknown law of extraordinary refraction — the last by means of secondary wavefronts that were spheroidal rather than spherical, with the result that the rays were generally oblique to the wavefronts. It was as if Huygens had not noticed that his construction implied Fermat's principle, and even as if he thought he had found an exception to that principle. Manuscript evidence cited by Alan E. Shapiro tends to confirm that Huygens believed the principle of least time to be invalid "in double refraction, where the rays are not normal to the wave fronts".

Shapiro further reports that the only three authorities who accepted "Huygens' principle" in the 17th and 18th centuries, namely Philippe de La Hire, Denis Papin, and Gottfried Wilhelm Leibniz, did so because it accounted for the extraordinary refraction of "Iceland crystal" (calcite) in the same manner as the previously known laws of geometrical optics. But, for the time being, the corresponding extension of Fermat's principle went unnoticed.

Laplace, Young, Fresnel, and Lorentz

Pierre-Simon Laplace (1749–1827)

On 30 January 1809, Pierre-Simon Laplace, reporting on the work of his protégé Étienne-Louis Malus, claimed that the extraordinary refraction of calcite could be explained under the corpuscular theory of light with the aid of Maupertuis's principle of least action: that the integral of speed with respect to distance was a minimum. The corpuscular speed that satisfied this principle was proportional to the reciprocal of the ray speed given by the radius of Huygens' spheroid. Laplace continued:

According to Huygens, the velocity of the extraordinary ray, in the crystal, is simply expressed by the radius of the spheroid; consequently his hypothesis does not agree with the principle of the least action: but it is remarkable that it agrees with the principle of Fermat, which is, that light passes, from a given point without the crystal, to a given point within it, in the least possible time; for it is easy to see that this principle coincides with that of the least action, if we invert the expression of the velocity.

Thomas Young (1773–1829)

Laplace's report was the subject of a wide-ranging rebuttal by Thomas Young, who wrote in part:

The principle of Fermat, although it was assumed by that mathematician on hypothetical, or even imaginary grounds, is in fact a fundamental law with respect to undulatory motion, and is explicitly [sic] the basis of every determination in the Huygenian theory...  Mr. Laplace seems to be unacquainted with this most essential principle of one of the two theories which he compares; for he says, that "it is remarkable," that the Huygenian law of extraordinary refraction agrees with the principle of Fermat; which he would scarcely have observed, if he had been aware that the law was an immediate consequence of the principle.

In fact Laplace was aware that Fermat's principle follows from Huygens' construction in the case of refraction from an isotropic medium to an anisotropic one; a geometric proof was contained in the long version of Laplace's report, printed in 1810.

Young's claim was more general than Laplace's, and likewise upheld Fermat's principle even in the case of extraordinary refraction, in which the rays are generally not perpendicular to the wavefronts. Unfortunately, however, the omitted middle sentence of the quoted paragraph by Young began "The motion of every undulation must necessarily be in a direction perpendicular to its surface..." (emphasis added), and was therefore bound to sow confusion rather than clarity.

Augustin-Jean Fresnel (1788–1827)

No such confusion subsists in Augustin-Jean Fresnel's "Second Memoir" on double refraction (Fresnel, 1827), which addresses Fermat's principle in several places (without naming Fermat), proceeding from the special case in which rays are normal to wavefronts, to the general case in which rays are paths of least time or stationary time. (In the following summary, page numbers refer to Alfred W. Hobson's translation.)

• For refraction of a plane wave at parallel incidence on one face of an anisotropic crystalline wedge (pp. 291–2), in order to find the "first ray arrived" at an observation point beyond the other face of the wedge, it suffices to treat the rays outside the crystal as normal to the wavefronts, and within the crystal to consider only the parallel wavefronts (whatever the ray direction). So in this case, Fresnel does not attempt to trace the complete ray path.
• Next, Fresnel considers a ray refracted from a point-source M inside a crystal, through a point A on the surface, to an observation point B outside (pp. 294–6). The surface passing through B and given by the "locus of the disturbances which arrive first" is, according to Huygens' construction, normal to "the ray AB of swiftest arrival". But this construction requires knowledge of the "surface of the wave" (that is, the secondary wavefront) within the crystal.
• Then he considers a plane wavefront propagating in a medium with non-spherical secondary wavefronts, oriented so that the ray path given by Huygens' construction — from the source of the secondary wavefront to its point of tangency with the subsequent primary wavefront — is not normal to the primary wavefronts (p. 296). He shows that this path is nevertheless "the path of quickest arrival of the disturbance" from the earlier primary wavefront to the point of tangency.
• In a later heading (p. 305) he declares that "The construction of Huygens, which determines the path of swiftest arrival," is applicable to secondary wavefronts of any shape. He then notes that when we apply Huygens' construction to refraction into a crystal with a two-sheeted secondary wavefront, and draw the lines from the two points of tangency to the center of the secondary wavefront, "we shall have the directions of the two paths of swiftest arrival, and consequently of the ordinary and of the extraordinary ray."
• Under the heading "Definition of the word Ray" (p. 309), he concludes that this term must be applied to the line which joins the center of the secondary wave to a point on its surface, whatever the inclination of this line to the surface.
• As a "new consideration" (pp. 310–11), he notes that if a plane wavefront is passed through a small hole centered on point E, then the direction ED of maximum intensity of the resulting beam will be that in which the secondary wave starting from E will "arrive there the first", and the secondary wavefronts from opposite sides of the hole (equidistant from E) will "arrive at D in the same time" as each other. This direction is not assumed to be normal to any wavefront.

Thus Fresnel showed, even for anisotropic media, that the ray path given by Huygens' construction is the path of least time between successive positions of a plane or diverging wavefront, that the ray velocities are the radii of the secondary "wave surface" after unit time, and that a stationary traversal time accounts for the direction of maximum intensity of a beam. However, establishing the general equivalence between Huygens' construction and Fermat's principle would have required further consideration of Fermat's principle in point-to-point terms.

Hendrik Lorentz, in a paper written in 1886 and republished in 1907, deduced the principle of least time in point-to-point form from Huygens' construction. But the essence of his argument was somewhat obscured by an apparent dependence on aether and aether drag.

Lorentz's work was cited in 1959 by Adriaan J. de Witte, who then offered his own argument, which "although in essence the same, is believed to be more cogent and more general." De Witte's treatment is more original than that description might suggest, although limited to two dimensions; it uses calculus of variations to show that Huygens' construction and Fermat's principle lead to the same differential equation for the ray path, and that in the case of Fermat's principle, the converse holds. De Witte also noted that "The matter seems to have escaped treatment in textbooks."

Forced labour

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Forced_labour 

 

Clergy on forced labour, by Ivan Vladimirov (Soviet Russia, 1919)

 

Unfree labour workers from Plovdiv during WW2

Forced labour, or unfree labour, is any work relation, especially in modern or early modern history, in which people are employed against their will with the threat of destitution, detention, violence including death, or other forms of extreme hardship to either themselves or members of their families.^{[note 1]}

Unfree labour includes all forms of slavery, penal labour and the corresponding institutions, such as debt slavery, serfdom, corvée and labour camps.

Definition

Many forms of unfree labour are also covered by the term forced labour, which is defined by the International Labour Organization (ILO) as all involuntary work or service exacted under the menace of a penalty.

However, under the ILO Forced Labour Convention of 1930, the term forced or compulsory labour does not include:

• "any work or service exacted in virtue of compulsory military service laws for work of a purely military character;"
• "any work or service which forms part of the normal civic obligations of the citizens of a fully self-governing country;"
• "any work or service exacted from any person as a consequence of a conviction in a court of law, provided that the said work or service is carried out under the supervision and control of a public authority and that the said person is not hired to or placed at the disposal of private individuals, companies or associations (requiring that prison farms no longer do convict leasing)";
• "any work or service exacted in cases of emergency, that is to say, in the event of war, of a calamity or threatened calamity, such as fire, flood, famine, earthquake, violent epidemic or epizootic diseases, invasion by: animal, insect or vegetable pests, and in general any circumstance that would endanger the existence or the well-being of the whole or part of the population";

Payment for unfree labour

See also: Labour economics § Wage slavery, Labor theory of value, and Productive and unproductive labour

 

Convict labourers in Australia in the early 19th century.

If payment occurs, it may be in one or more of the following forms:

• The payment does not exceed subsistence or barely exceeds it;
• The payment is in goods which are not desirable and/or cannot be exchanged or are difficult to exchange; or
• The payment wholly or mostly consists of cancellation of a debt or liability that was itself coerced, or belongs to someone else.

Unfree labour is often more easily instituted and enforced on migrant workers, who have travelled far from their homelands and who are easily identified because of their physical, ethnic, linguistic, or cultural differences from the general population, since they are unable or unlikely to report their conditions to the authorities.

Industrial involvement

Trenching with hand tools and scant protective gear in rail construction, early 20th century.

In many contexts, the use of unfree labour is prohibited under the law and is mainly associated with the underground economy. In other contexts, established industries have embraced the use of unfree labour as a socially accepted practice in that time and place. Use of compelled labour is especially common when the labour involved can not be performed without risk of death, disfigurement, disability, or diminished life expectancy; in the extreme, these detriments render the voluntary labour market uneconomic, and the industry in question is forced to either adopt compelled labour or discontinue operations altogether.

Industries which continue to employ unfree labour worldwide include agriculture, domestic work, manufacture, and hospitality. Mining, defence, the merchant marine and transport infrastructure, which employed questionable practices during the heyday of railway track construction (often involving the use of high explosives or constructing high wooden trestle bridges in sheer mountain canyons), and of canal excavation (sometimes in conditions of permafrost) also have historical ties.

Modern day unfree labour

Unfree labour re-emerged as an issue in the debate about rural development during the years following the end of the Second World War, when a political concern of Keynesian theory was not just economic reconstruction (mainly in Europe and Asia) but also planning (in developing "Third World" nations). A crucial aspect of the ensuing discussion concerned the extent to which different relational forms constituted obstacles to capitalist development, and why.

During the 1960s and 1970s unfree labour was regarded as incompatible with capitalist accumulation, and thus an obstacle to economic growth, an interpretation advanced by exponents of the then-dominant semi-feudal thesis. From the 1980s onwards, however, another and very different Marxist view emerged, arguing that evidence from Latin America and India suggested agribusiness enterprises, commercial farmers and rich peasants reproduced, introduced or reintroduced unfree relations.

However, recent contributions to this debate have attempted to exclude Marxism from the discussion. These contributions maintain that, because Marxist theory failed to understand the centrality of unfreedom to modern capitalism, a new explanation of this link is needed. This claim has been questioned by Tom Brass (2014), ‘Debating Capitalist Dynamics and Unfree Labour: A Missing Link?’, The Journal of Development Studies, 50:4, 570–82. He argues that many of these new characteristics are in fact no different from those identified earlier by Marxist theory and that the exclusion of the latter approach from the debate is thus unwarranted.

The International Labour Organization (ILO) estimates that at least 12.3 million people are victims of forced labour worldwide; of these, 9.8 million are exploited by private agents and more than 2.4 million are trafficked. Another 2.5 million are forced to work by the state or by rebel military groups. From an international law perspective, countries that allow forced labour are violating international labour standards as set forth in the Abolition of Forced Labour Convention (C105), one of the fundamental conventions of the ILO.

See also: Global Slavery Index

According to the ILO Special Action Programme to Combat Forced Labour (SAP-FL), global profits from forced trafficked labour exploited by private agents are estimated at US$44,3 billion per year. About 70% of this value (US$31.6 billion) come from trafficked victims. At least the half of this sum (more than US$15 billion) comes from industrialized countries.

Freedom from forced labour by country (V-Dem Institute, 2021)

Trafficking

Main article: Human trafficking

Trafficking is a term to define the recruiting, harbouring, obtaining and transportation of a person by use of force, fraud, or coercion for the purpose of subjecting them to involuntary acts, such as acts related to commercial sexual exploitation (including forced prostitution) or involuntary labour.

Forms of unfree labour

Illustration of Native woman panning for gold

Slavery

Main article: Slavery

The archetypal and best-known form of unfree labour is chattel slavery, in which individual workers are legally owned throughout their lives, and may be bought, sold or otherwise exchanged by owners, while never or rarely receiving any personal benefit from their labour. Slavery was common in many ancient societies, including ancient Egypt, Babylon, Persia, ancient Greece, Rome, ancient Israel, ancient China, classical Arab states, as well as many societies in Africa and the Americas. Being sold into slavery was a common fate of populations that were conquered in wars. Perhaps the most prominent example of chattel slavery was the enslavement of many millions of black people in Africa, as well as their forced transportation to the Americas, Asia, or Europe, where their status as slaves was almost always inherited by their descendants.

The term "slavery" is often applied to situations which do not meet the above definitions, but which are other, closely related forms of unfree labour, such as debt slavery or debt-bondage (although not all repayment of debts through labour constitutes unfree labour). Examples are the Repartimiento system in the Spanish Empire, or the work of Indigenous Australians in northern Australia on sheep or cattle stations (ranches), from the mid-19th to the mid-20th century. In the latter case, workers were rarely or never paid, and were restricted by regulations and/or police intervention to regions around their places of work.

In late 16th century Japan, "unfree labour" or slavery was officially banned; but forms of contract and indentured labour persisted alongside the period's penal codes' forced labour. Somewhat later, the Edo period's penal laws prescribed "non-free labour" for the immediate families of executed criminals in Article 17 of the Gotōke reijō (Tokugawa House Laws), but the practice never became common. The 1711 Gotōke reijō was compiled from over 600 statutes that were promulgated between 1597 and 1696.

According to Kevin Bales in Disposable People: New Slavery in the Global Economy (1999), there are now an estimated 27 million slaves in the world.

See also: Global Slavery Index

Blackbirding

Blackbirding involves kidnapping or trickery to transport people to another country or far away from home, to work as a slave or low-paid involuntary worker. In some cases, workers were returned home after a period of time.

Serfdom

Serfdom bonds labourers to the land they farm, typically in a feudal society. Serfs typically have no legal right to leave, change employers, or seek paid work, though depending on economic conditions many did so anyway. Unlike chattel slaves, they typically cannot be sold separately from the land, and have rights such as the military protection of the lord.

Truck system

Main article: Truck system

A truck system, in the specific sense in which the term is used by labour historians, refers to an unpopular or even exploitative form of payment associated with small, isolated and/or rural communities, in which workers or self-employed small producers are paid in either: goods, a form of payment known as truck wages, or tokens, private currency ("scrip") or direct credit, to be used at a company store, owned by their employers. A specific kind of truck system, in which credit advances are made against future work, is known in the U.S. as debt bondage.

Many scholars have suggested that employers use such systems to exploit workers and/or indebt them. This could occur, for example, if employers were able to pay workers with goods which had a market value below the level of subsistence, or by selling items to workers at inflated prices. Others argue that truck wages were a convenient way for isolated communities, such as during the early colonial settlement of North America, to operate when official currency was scarce.

By the early 20th century, truck systems were widely seen, in industrialized countries, as exploitative; perhaps the most well-known example of this view was a 1947 U.S. hit song "Sixteen Tons". Many countries have Truck Act legislation that outlaws truck systems and requires payment in cash.

Mandatory services due to social status

Corvée

Main articles: Corvée and Socage

Though most closely associated with Medieval Europe, governments throughout human history have imposed regular short stints of unpaid labour upon lower social classes. These might be annual obligations of a few weeks or something similarly regular that lasted for the labourer's entire working life. As the system developed in the Philippines and elsewhere, the labourer could pay an appropriate fee and be exempted from the obligation.

Vetti-chakiri

Main article: Veth (India)

A form of forced labour in which peasants and members of lower castes were required to work for free existed in India before independence. This form of labour was known by several names, including veth, vethi, vetti-chakiri and begar.

Penal labour

Main article: Penal labour

See also: Convicts in Australia, Katorga, and Devil's Island

Labour camps

Main article: Labour camp

See also: The Holocaust, Japanese war crimes, Slavery in Japan, Gulag, Laogai, Kwalliso, Arbeitslager, Nazi concentration camps, Forced labor of Germans after World War II, and History of Germany (1945–90) § Forced labor reparations

 

Female forced labourers wearing "OST" (Ost-Arbeiter) badges are liberated from a camp near Lodz, January 1945.

Another historically significant example of forced labour was that of political prisoners, people from conquered or occupied countries, members of persecuted minorities, and prisoners of war, especially during the 20th century. The best-known example of this are the concentration camp system run by Nazi Germany in Europe during World War II, the Gulag camps run by the Soviet Union, and the forced labour used by the military of the Empire of Japan, especially during the Pacific War (such as the Burma Railway). Roughly 4,000,000 German POWs were used as "reparations labour" by the Allies for several years after the German surrender; this was permitted under the Third Geneva Convention provided they were accorded proper treatment. China's laogai ("labour reform") system and North Korea's kwalliso camps are current examples.

About 12 million forced labourers, most of whom were Poles and Soviet citizens (Ost-Arbeiter) were employed in the German war economy inside Nazi Germany. More than 2000 German companies profited from slave labour during the Nazi era, including Daimler, Deutsche Bank, Siemens, Volkswagen, Hoechst, Dresdner Bank, Krupp, Allianz, BASF, Bayer, BMW, and Degussa. In particular, Germany's Jewish population was subject to slave labour prior to their extermination.

In Asia, according to a joint study of historians featuring Zhifen Ju, Mark Peattie, Toru Kubo, and Mitsuyoshi Himeta, more than 10 million Chinese were mobilized by the Japanese army and enslaved by the Kōa-in for slave labour in Manchukuo and north China. The U.S. Library of Congress estimates that in Java, between 4 and 10 million romusha (Japanese: "manual labourer") were forced to work by the Japanese military. About 270,000 of these Javanese labourers were sent to other Japanese-held areas in South East Asia. Only 52,000 were repatriated to Java, meaning that there was a death rate of 80%.

Kerja rodi (Heerendiensten), was the term for forced labour in Indonesia under Dutch colonial rule.

The Khmer Rouge attempted to turn Cambodia into a classless society by depopulating cities and forcing the urban population ("New People") into agricultural communes. The entire population was forced to become farmers in labour camps.

Prison labour

American prisoner "chain gang" labourers, 2006. Notice the shackles on the feet of the prisoners.

Convict or prison labour is another classic form of unfree labour. The forced labour of convicts has often been regarded with lack of sympathy, because of the social stigma attached to people regarded as "common criminals". In some countries and historical periods, however, including the modern United States, prison labour has been forced upon people who have been victims of prejudice, convicted of political crimes, convicted of "victimless crimes", or people who committed theft or related offences because they lacked any other means of subsistence—categories of people who typically are entitled to compassion according to current ethical ideas.

Three British colonies in Australia— New South Wales, Van Diemen's Land and Western Australia—are examples of the state use of convict labour. Australia received thousands of convict labourers in the eighteenth and nineteenth centuries who were given sentences for crimes ranging from those now considered to be minor misdemeanors to such serious offences as murder, rape and incest. A considerable number of Irish convicts were sentenced to transportation for treason while fighting against British rule in Ireland.

More than 165,000 convicts were transported to Australian colonies from 1788 to 1868. Most British or Irish convicts who were sentenced to transportation, however, completed their sentences in British jails and were not transported at all.

It is estimated that in the last 50 years more than 50 million people have been sent to Chinese laogai camps.

Indentured and bonded labour

Main articles: Indenture and bonded labour

A more common form in modern society is indenture, or bonded labour, under which workers sign contracts to work for a specific period of time, for which they are paid only with accommodation and sustenance, or these essentials in addition to limited benefits such as cancellation of a debt, or transportation to a desired country.

Permitted exceptions of unfree labour

As mentioned above, there are several exceptions of unfree or forced labour recognized by the International Labour Organization:

Civil conscription

Main article: Civil conscription

Some countries practice forms of civil conscription for different major occupational groups or inhabitants under different denominations like civil conscription, civil mobilization, political mobilization etc. This obligatory services on the one hand has been implemented due to long-lasting labour strikes, during wartimes or economic crisis, to provide basic services like medical care, food supply or supply of the defence industry. On the other hand, this service can be obligatory to provide recurring and inevitable services to the population, like fire services, due to lack of volunteers.

Temporary civil conscription

Between December 1943 and March 1948 young men in the United Kingdom, the so-called Bevin Boys, had been conscripted for the work in coal mines. In Belgium in 1964, in Portugal and in Greece from 2010 to 2014 due to the severe economic crisis, a system of civil mobilization was implemented to provide public services as a national interest.

Recurring civil conscription

In Switzerland in most communities for all inhabitants, no matter if they are Swiss or not, it is mandatory to join the so-called Militia Fire Brigades, as well as the obligatory service in Swiss civil defence and protection force. Conscripts in Singapore are providing the personnel of the country's fire service as part of the national service in the Civil Defence Force. In Austria and Germany citizens have to join a compulsory fire brigade if a volunteer fire service can not be provided, due to lack of volunteers. In 2018 this regulation is executed only in a handful of communities in Germany and currently none in Austria.

Conscription for military service and security forces

Main articles: Conscription and Impressment

Beside the conscription for military services, some countries draft citizens for paramilitary or security forces, like internal troops, border guards or police forces. While sometimes paid, conscripts are not free to decline enlistment. Draft dodging or desertion are often met with severe punishment. Even in countries which prohibit other forms of unfree labour, conscription is generally justified as being necessary in the national interest and therefore is one of the five exceptions to the Forced Labour Convention, signed by the most countries in the world.

Mandatory community service

Community services

Community service is a non-paying job performed by one person or a group of people for the benefit of their community or its institutions. Community service is distinct from volunteering, since it is not always performed on a voluntary basis. Although personal benefits may be realized, it may be performed for a variety of reasons including citizenship requirements, a substitution of criminal justice sanctions, requirements of a school or class, and requisites for the receipt of certain benefits.

De facto obligatory community work

During the Cold War in some communist countries like Czechoslovakia, the German Democratic Republic or the Soviet Union the originally voluntary work on Saturday for the community called Subbotnik, Voskresnik or Akce Z became de facto obligatory for the members of a community.

Hand and hitch-up services

In some German states it is feasible for communities to draft citizens for public services, called hand and hitch-up services. This mandatory service is still executed to maintain the infrastructure of small communities.

International conventions

• ILO Forced Labour Convention, 1930 (No. 29)
• ILO Abolition of Forced Labour Convention, 1957 (No. 105)
• ILO Minimum Age Convention, 1973 (No. 138)
• Worst Forms of Child Labour Convention, 1999 (No. 182)