Kepler's laws of planetary motion

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Kepler%27s_laws_of_planetary_motion 

Figure 1: Illustration of Kepler's three laws with two planetary orbits.

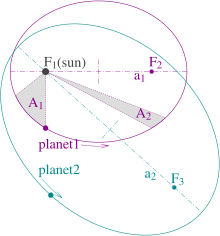
1. The orbits are ellipses, with focal points F₁ and F₂ for the first planet and F₁ and F₃ for the second planet. The Sun is placed at focal point F₁.
2. The two shaded sectors A₁ and A₂ have the same surface area and the time for planet 1 to cover segment A₁ is equal to the time to cover segment A₂.
3. The total orbit times for planet 1 and planet 2 have a ratio ${\left(\frac{a_1}{a_2}\right)}^{\frac{3}{2}}$.

In astronomy, Kepler's laws of planetary motion, published by Johannes Kepler between 1609 and 1619, describe the orbits of planets around the Sun. The laws modified the heliocentric theory of Nicolaus Copernicus, replacing its circular orbits and epicycles with elliptical trajectories, and explaining how planetary velocities vary. The three laws state that:

1. The orbit of a planet is an ellipse with the Sun at one of the two foci.
2. A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time.
3. The square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit.

The elliptical orbits of planets were indicated by calculations of the orbit of Mars. From this, Kepler inferred that other bodies in the Solar System, including those farther away from the Sun, also have elliptical orbits. The second law helps to establish that when a planet is closer to the Sun, it travels faster. The third law expresses that the farther a planet is from the Sun, the slower its orbital speed, and vice versa.

Isaac Newton showed in 1687 that relationships like Kepler's would apply in the Solar System as a consequence of his own laws of motion and law of universal gravitation.

Comparison to Copernicus

Johannes Kepler's laws improved the model of Copernicus. According to Copernicus:

1. The planetary orbit is a circle with epicycles.
2. The Sun is approximately at the center of the orbit.
3. The speed of the planet in the main orbit is constant.

Despite being correct in saying that the planets revolved around the Sun, Copernicus was incorrect in defining their orbits. It was Kepler who correctly defined the orbit of planets as follows:

1. The planetary orbit is not a circle with epicycles, but an ellipse.
2. The Sun is not at the center but at a focal point of the elliptical orbit.
3. Neither the linear speed nor the angular speed of the planet in the orbit is constant, but the area speed (closely linked historically with the concept of angular momentum) is constant.

The eccentricity of the orbit of the Earth makes the time from the March equinox to the September equinox, around 186 days, unequal to the time from the September equinox to the March equinox, around 179 days. A diameter would cut the orbit into equal parts, but the plane through the Sun parallel to the equator of the Earth cuts the orbit into two parts with areas in a 186 to 179 ratio, so the eccentricity of the orbit of the Earth is approximately

${\displaystyle e\approx \frac{\pi }{4}\frac{186-179}{186+179}\approx 0.015,}$

which is close to the correct value (0.016710218). The accuracy of this calculation requires that the two dates chosen be along the elliptical orbit's minor axis and that the midpoints of each half be along the major axis. As the two dates chosen here are equinoxes, this will be correct when perihelion, the date the Earth is closest to the Sun, falls on a solstice. The current perihelion, near January 4, is fairly close to the solstice of December 21 or 22.

Nomenclature

It took nearly two centuries for current formulation of Kepler's work to take on its settled form. Voltaire's Eléments de la philosophie de Newton (Elements of Newton's Philosophy) of 1738 was the first publication to use the terminology of "laws". The Biographical Encyclopedia of Astronomers in its article on Kepler (p. 620) states that the terminology of scientific laws for these discoveries was current at least from the time of Joseph de Lalande. It was the exposition of Robert Small, in An account of the astronomical discoveries of Kepler (1814) that made up the set of three laws, by adding in the third. Small also claimed, against the history, that these were empirical laws, based on inductive reasoning.

Further, the current usage of "Kepler's Second Law" is something of a misnomer. Kepler had two versions, related in a qualitative sense: the "distance law" and the "area law". The "area law" is what became the Second Law in the set of three; but Kepler did himself not privilege it in that way.

History

Kepler published his first two laws about planetary motion in 1609, having found them by analyzing the astronomical observations of Tycho Brahe. Kepler's third law was published in 1619. Kepler had believed in the Copernican model of the Solar System, which called for circular orbits, but he could not reconcile Brahe's highly precise observations with a circular fit to Mars' orbit – Mars coincidentally having the highest eccentricity of all planets except Mercury. His first law reflected this discovery.

In 1621, Kepler noted that his third law applies to the four brightest moons of Jupiter. Godefroy Wendelin also made this observation in 1643. The second law, in the "area law" form, was contested by Nicolaus Mercator in a book from 1664, but by 1670 his Philosophical Transactions were in its favour. As the century proceeded it became more widely accepted. The reception in Germany changed noticeably between 1688, the year in which Newton's Principia was published and was taken to be basically Copernican, and 1690, by which time work of Gottfried Leibniz on Kepler had been published.

Newton was credited with understanding that the second law is not special to the inverse square law of gravitation, being a consequence just of the radial nature of that law, whereas the other laws do depend on the inverse square form of the attraction. Carl Runge and Wilhelm Lenz much later identified a symmetry principle in the phase space of planetary motion (the orthogonal group O(4) acting) which accounts for the first and third laws in the case of Newtonian gravitation, as conservation of angular momentum does via rotational symmetry for the second law.

Formulary

The mathematical model of the kinematics of a planet subject to the laws allows a large range of further calculations.

First law

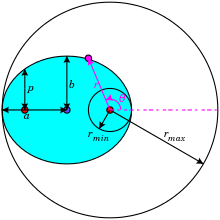
The orbit of every planet is an ellipse with the Sun at one of the two foci.

 Figure 2: Kepler's first law placing the Sun at the focus of an elliptical orbit 

Figure 3: Heliocentric coordinate system (r, θ) for ellipse. Also shown are: semi-major axis a, semi-minor axis b and semi-latus rectum p; center of ellipse and its two foci marked by large dots. For θ = 0°, r = r_min and for θ = 180°, r = r_max.

Mathematically, an ellipse can be represented by the formula:

${\displaystyle r=\frac{p}{1+\epsilon \cos \theta },}$

where ${\displaystyle p}$ is the semi-latus rectum, ε is the eccentricity of the ellipse, r is the distance from the Sun to the planet, and θ is the angle to the planet's current position from its closest approach, as seen from the Sun. So (r, θ) are polar coordinates.

For an ellipse 0 < ε < 1 ; in the limiting case ε = 0, the orbit is a circle with the Sun at the centre (i.e. where there is zero eccentricity).

At θ = 0°, perihelion, the distance is minimum

${\displaystyle r_{min}=\frac{p}{1+\epsilon }}$

At θ = 90° and at θ = 270° the distance is equal to ${\displaystyle p}$.

At θ = 180°, aphelion, the distance is maximum (by definition, aphelion is – invariably – perihelion plus 180°)

${\displaystyle r_{max}=\frac{p}{1-\epsilon }}$

The semi-major axis a is the arithmetic mean between r_min and r_max:

${\displaystyle \begin{array}{rl}a& =\frac{r_{max}+r_{min}}{2}\\ a& =\frac{p}{1-{\epsilon }^2}\end{array}}$

The semi-minor axis b is the geometric mean between r_min and r_max:

${\displaystyle \begin{array}{rl}b& =\sqrt{r_{max}{r}_{min}}\\ b& =\frac{p}{\sqrt{1-{\epsilon }^2}}\end{array}}$

The semi-latus rectum p is the harmonic mean between r_min and r_max:

${\displaystyle \begin{array}{rl}p& ={\left(\frac{r_{max}^{-1}+r_{min}^{-1}}{2}\right)}^{-1}\\ pa& =r_{max}{r}_{min}=b^2\end{array}}$

The eccentricity ε is the coefficient of variation between r_min and r_max:

${\displaystyle \epsilon =\frac{r_{max}-r_{min}}{r_{max}+r_{min}}.}$

The area of the ellipse is

${\displaystyle A=\pi ab.}$

The special case of a circle is ε = 0, resulting in r = p = r_min = r_max = a = b and A = πr².

Second law

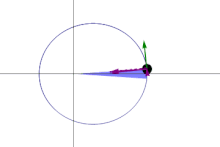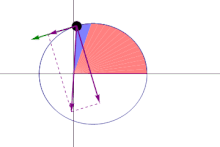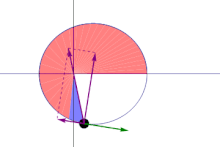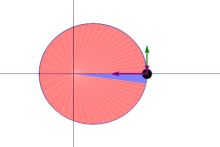
A line joining a planet and the Sun sweeps out equal areas during equal intervals of time.

The same (blue) area is swept out in a fixed time period. The green arrow is velocity. The purple arrow directed towards the Sun is the acceleration. The other two purple arrows are acceleration components parallel and perpendicular to the velocity.

The orbital radius and angular velocity of the planet in the elliptical orbit will vary. This is shown in the animation: the planet travels faster when closer to the Sun, then slower when farther from the Sun. Kepler's second law states that the blue sector has constant area.

In a small time ${\displaystyle dt}$ the planet sweeps out a small triangle having base line ${\displaystyle r}$ and height ${\displaystyle rd\theta }$ and area $dA=\frac{1}{2}\cdot r\cdot rd\theta$, so the constant areal velocity is

$${\displaystyle \frac{dA}{dt}=\frac{r^2}{2}\frac{d\theta }{dt}.}$$

The area enclosed by the elliptical orbit is ${\displaystyle \pi ab}$. So the period ${\displaystyle T}$ satisfies

${\displaystyle T\cdot \frac{r^2}{2}\frac{d\theta }{dt}=\pi ab}$

and the mean motion of the planet around the Sun

${\displaystyle n=\frac{2\pi }{T}}$

satisfies

${\displaystyle r^{2}d\theta =abndt.}$

And so,

$${\displaystyle \frac{dA}{dt}=\frac{abn}{2}=\frac{\pi ab}{T}.}$$

Orbits of planets with varying eccentricities. The red ray rotates at a constant angular velocity and with the same orbital time period as the planet, ${\displaystyle T=1}$. S: Sun at the primary focus, C: Centre of ellipse, S': The secondary focus. In each case, the area of all sectors depicted is identical.

Low	High

Planet orbiting the Sun in a circular orbit (e=0.0)

Planet orbiting the Sun in an orbit with e=0.5

Planet orbiting the Sun in an orbit with e=0.2

Planet orbiting the Sun in an orbit with e=0.8

Third law

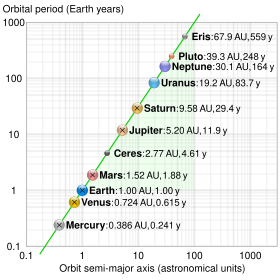
The ratio of the square of an object's orbital period with the cube of the semi-major axis of its orbit is the same for all objects orbiting the same primary.

This captures the relationship between the distance of planets from the Sun, and their orbital periods.

Kepler enunciated in 1619 this third law in a laborious attempt to determine what he viewed as the "music of the spheres" according to precise laws, and express it in terms of musical notation. It was therefore known as the harmonic law.

Using Newton's law of gravitation (published 1687), this relation can be found in the case of a circular orbit by setting the centripetal force equal to the gravitational force:

${\displaystyle mr{\omega }^2=G\frac{mM}{r^2}}$

Then, expressing the angular velocity ω in terms of the orbital period ${\displaystyle T}$ and then rearranging, results in Kepler's Third Law:

${\displaystyle mr{\left(\frac{2\pi }{T}\right)}^2=G\frac{mM}{r^2}\to T^2=\left(\frac{4{\pi }^2}{GM}\right)r^3\to T^2\propto r^3}$

A more detailed derivation can be done with general elliptical orbits, instead of circles, as well as orbiting the center of mass, instead of just the large mass. This results in replacing a circular radius, ${\displaystyle r}$, with the semi-major axis, ${\displaystyle a}$, of the elliptical relative motion of one mass relative to the other, as well as replacing the large mass ${\displaystyle M}$ with ${\displaystyle M+m}$. However, with planet masses being so much smaller than the Sun, this correction is often ignored. The full corresponding formula is:

${\displaystyle \frac{a^3}{T^2}=\frac{G(M+m)}{4{\pi }^2}\approx \frac{GM}{4{\pi }^2}\approx 7.496\times {10}^{-6}\frac{{\text{AU}}^3}{{\text{days}}^2}\text{ is constant}}$

where ${\displaystyle M}$ is the mass of the Sun, ${\displaystyle m}$ is the mass of the planet, ${\displaystyle G}$ is the gravitational constant, ${\displaystyle T}$ is the orbital period and ${\displaystyle a}$ is the elliptical semi-major axis, and ${\displaystyle \text{AU}}$ is the astronomical unit, the average distance from earth to the sun.

Table

The following table shows the data used by Kepler to empirically derive his law:

Data used by Kepler (1618)

Planet	Mean distance to sun (AU)	Period (days)	$\frac{R^3}{T^2}$ (10-6 AU3/day2)
Mercury	0.389	87.77	7.64
Venus	0.724	224.70	7.52
Earth	1	365.25	7.50
Mars	1.524	686.95	7.50
Jupiter	5.20	4332.62	7.49
Saturn	9.510	10759.2	7.43

Upon finding this pattern Kepler wrote:

I first believed I was dreaming... But it is absolutely certain and exact that the ratio which exists between the period times of any two planets is precisely the ratio of the 3/2th power of the mean distance.

— translated from Harmonies of the World by Kepler (1619)

Log-log plot of period T vs semi-major axis a (average of aphelion and perihelion) of some Solar System orbits (crosses denoting Kepler's values) showing that a³/T² is constant (green line)

For comparison, here are modern estimates:

Modern data (Wolfram Alpha Knowledgebase 2018)

Planet	Semi-major axis (AU)	Period (days)	$\frac{R^3}{T^2}$ (10-6 AU3/day2)
Mercury	0.38710	87.9693	7.496
Venus	0.72333	224.7008	7.496
Earth	1	365.2564	7.496
Mars	1.52366	686.9796	7.495
Jupiter	5.20336	4332.8201	7.504
Saturn	9.53707	10775.599	7.498
Uranus	19.1913	30687.153	7.506
Neptune	30.0690	60190.03	7.504

Planetary acceleration

Isaac Newton computed in his Philosophiæ Naturalis Principia Mathematica the acceleration of a planet moving according to Kepler's first and second laws.

1. The direction of the acceleration is towards the Sun.
2. The magnitude of the acceleration is inversely proportional to the square of the planet's distance from the Sun (the inverse square law).

This implies that the Sun may be the physical cause of the acceleration of planets. However, Newton states in his Principia that he considers forces from a mathematical point of view, not a physical, thereby taking an instrumentalist view. Moreover, he does not assign a cause to gravity.

Newton defined the force acting on a planet to be the product of its mass and the acceleration (see Newton's laws of motion). So:

1. Every planet is attracted towards the Sun.
2. The force acting on a planet is directly proportional to the mass of the planet and is inversely proportional to the square of its distance from the Sun.

The Sun plays an unsymmetrical part, which is unjustified. So he assumed, in Newton's law of universal gravitation:

1. All bodies in the Solar System attract one another.
2. The force between two bodies is in direct proportion to the product of their masses and in inverse proportion to the square of the distance between them.

As the planets have small masses compared to that of the Sun, the orbits conform approximately to Kepler's laws. Newton's model improves upon Kepler's model, and fits actual observations more accurately. (See two-body problem.)

Below comes the detailed calculation of the acceleration of a planet moving according to Kepler's first and second laws.

Acceleration vector

See also: Polar coordinate § Vector calculus, and Mechanics of planar particle motion

From the heliocentric point of view consider the vector to the planet ${\displaystyle \mathbf{r}=r\hat{\mathbf{r}}}$ where ${\displaystyle r}$ is the distance to the planet and ${\displaystyle \hat{\mathbf{r}}}$ is a unit vector pointing towards the planet.

$${\displaystyle \frac{d\hat{\mathbf{r}}}{dt}=\dot{\hat{\mathbf{r}}}=\dot{\theta }\hat{\mathit{\theta }},\frac{d\hat{\mathit{\theta }}}{dt}=\dot{\hat{\mathit{\theta }}}=-\dot{\theta }\hat{\mathbf{r}}}$$

where ${\displaystyle \hat{\mathit{\theta }}}$ is the unit vector whose direction is 90 degrees counterclockwise of ${\displaystyle \hat{\mathbf{r}}}$, and ${\displaystyle \theta }$ is the polar angle, and where a dot on top of the variable signifies differentiation with respect to time.

Differentiate the position vector twice to obtain the velocity vector and the acceleration vector:

$${\displaystyle \begin{array}{rl}\dot{\mathbf{r}}& =\dot{r}\hat{\mathbf{r}}+r\dot{\hat{\mathbf{r}}}=\dot{r}\hat{\mathbf{r}}+r\dot{\theta }\hat{\mathit{\theta }},\\ \ddot{\mathbf{r}}& =\left(\ddot{r}\hat{\mathbf{r}}+\dot{r}\dot{\hat{\mathbf{r}}}\right)+\left(\dot{r}\dot{\theta }\hat{\mathit{\theta }}+r\ddot{\theta }\hat{\mathit{\theta }}+r\dot{\theta }\dot{\hat{\mathit{\theta }}}\right)=\left(\ddot{r}-r{\dot{\theta }}^2\right)\hat{\mathbf{r}}+\left(r\ddot{\theta }+2\dot{r}\dot{\theta }\right)\hat{\mathit{\theta }}.\end{array}}$$

So

$${\displaystyle \ddot{\mathbf{r}}=a_r\hat{\mathit{r}}+a_{\theta }\hat{\mathit{\theta }}}$$

where the radial acceleration is

$${\displaystyle a_r=\ddot{r}-r{\dot{\theta }}^2}$$

and the transversal acceleration is

$${\displaystyle a_{\theta }=r\ddot{\theta }+2\dot{r}\dot{\theta }.}$$

Inverse square law

Kepler's second law says that

$${\displaystyle r^2\dot{\theta }=nab}$$

is constant.

The transversal acceleration ${\displaystyle a_{\theta }}$ is zero:

$${\displaystyle \frac{d\left(r^2\dot{\theta }\right)}{dt}=r\left(2\dot{r}\dot{\theta }+r\ddot{\theta }\right)=r{a}_{\theta }=0.}$$

So the acceleration of a planet obeying Kepler's second law is directed towards the Sun.

The radial acceleration ${\displaystyle a_{\text{r}}}$ is

$${\displaystyle a_{\text{r}}=\ddot{r}-r{\dot{\theta }}^2=\ddot{r}-r{\left(\frac{nab}{r^2}\right)}^2=\ddot{r}-\frac{n^2{a}^2{b}^2}{r^3}.}$$

Kepler's first law states that the orbit is described by the equation:

$${\displaystyle \frac{p}{r}=1+\epsilon \cos (\theta ).}$$

Differentiating with respect to time

$${\displaystyle -\frac{p\dot{r}}{r^2}=-\epsilon \sin (\theta )\dot{\theta }}$$

or

$${\displaystyle p\dot{r}=nab\epsilon \sin (\theta ).}$$

Differentiating once more

$${\displaystyle p\ddot{r}=nab\epsilon \cos (\theta )\dot{\theta }=nab\epsilon \cos (\theta )\frac{nab}{r^2}=\frac{n^2{a}^2{b}^2}{r^2}\epsilon \cos (\theta ).}$$

The radial acceleration ${\displaystyle a_{\text{r}}}$ satisfies

$${\displaystyle p{a}_{\text{r}}=\frac{n^2{a}^2{b}^2}{r^2}\epsilon \cos (\theta )-p\frac{n^2{a}^2{b}^2}{r^3}=\frac{n^2{a}^2{b}^2}{r^2}\left(\epsilon \cos (\theta )-\frac{p}{r}\right).}$$

Substituting the equation of the ellipse gives

$${\displaystyle p{a}_{\text{r}}=\frac{n^2{a}^2{b}^2}{r^2}\left(\frac{p}{r}-1-\frac{p}{r}\right)=-\frac{n^2{a}^2}{r^2}{b}^2.}$$

The relation ${\displaystyle b^2=pa}$ gives the simple final result

$${\displaystyle a_{\text{r}}=-\frac{n^2{a}^3}{r^2}.}$$

This means that the acceleration vector ${\displaystyle \ddot{\mathbf{r}}}$ of any planet obeying Kepler's first and second law satisfies the inverse square law

$${\displaystyle \ddot{\mathbf{r}}=-\frac{\alpha }{r^2}\hat{\mathbf{r}}}$$

where

$${\displaystyle \alpha =n^2{a}^3}$$

is a constant, and ${\displaystyle \hat{\mathbf{r}}}$ is the unit vector pointing from the Sun towards the planet, and ${\displaystyle r}$ is the distance between the planet and the Sun.

Since mean motion ${\displaystyle n=\frac{2\pi }{T}}$ where ${\displaystyle T}$ is the period, according to Kepler's third law, ${\displaystyle \alpha }$ has the same value for all the planets. So the inverse square law for planetary accelerations applies throughout the entire Solar System.

The inverse square law is a differential equation. The solutions to this differential equation include the Keplerian motions, as shown, but they also include motions where the orbit is a hyperbola or parabola or a straight line. (See Kepler orbit.)

Newton's law of gravitation

By Newton's second law, the gravitational force that acts on the planet is:

$${\displaystyle \mathbf{F}=m_{\text{planet}}\ddot{\mathbf{r}}=-m_{\text{planet}}\alpha r^{-2}\hat{\mathbf{r}}}$$

where ${\displaystyle m_{\text{planet}}}$ is the mass of the planet and ${\displaystyle \alpha }$ has the same value for all planets in the Solar System. According to Newton's third law, the Sun is attracted to the planet by a force of the same magnitude. Since the force is proportional to the mass of the planet, under the symmetric consideration, it should also be proportional to the mass of the Sun, ${\displaystyle m_{\text{Sun}}}$. So

$${\displaystyle \alpha =G{m}_{\text{Sun}}}$$

where ${\displaystyle G}$ is the gravitational constant.

The acceleration of solar system body number i is, according to Newton's laws:

$${\displaystyle {\ddot{\mathbf{r}}}_i=G\sum _{j\ne i}{m}_j{r}_{ij}^{-2}{\hat{\mathbf{r}}}_{ij}}$$

where ${\displaystyle m_j}$ is the mass of body j, ${\displaystyle r_{ij}}$ is the distance between body i and body j, ${\displaystyle {\hat{\mathbf{r}}}_{ij}}$ is the unit vector from body i towards body j, and the vector summation is over all bodies in the Solar System, besides i itself.

In the special case where there are only two bodies in the Solar System, Earth and Sun, the acceleration becomes

$${\displaystyle {\ddot{\mathbf{r}}}_{\text{Earth}}=G{m}_{\text{Sun}}{r}_{\text{Earth},\text{Sun}}^{-2}{\hat{\mathbf{r}}}_{\text{Earth},\text{Sun}}}$$

which is the acceleration of the Kepler motion. So this Earth moves around the Sun according to Kepler's laws.

If the two bodies in the Solar System are Moon and Earth the acceleration of the Moon becomes

$${\displaystyle {\ddot{\mathbf{r}}}_{\text{Moon}}=G{m}_{\text{Earth}}{r}_{\text{Moon},\text{Earth}}^{-2}{\hat{\mathbf{r}}}_{\text{Moon},\text{Earth}}}$$

So in this approximation, the Moon moves around the Earth according to Kepler's laws.

In the three-body case the accelerations are

$${\displaystyle \begin{array}{rl}{\ddot{\mathbf{r}}}_{\text{Sun}}& =G{m}_{\text{Earth}}{r}_{\text{Sun},\text{Earth}}^{-2}{\hat{\mathbf{r}}}_{\text{Sun},\text{Earth}}+G{m}_{\text{Moon}}{r}_{\text{Sun},\text{Moon}}^{-2}{\hat{\mathbf{r}}}_{\text{Sun},\text{Moon}}\\ {\ddot{\mathbf{r}}}_{\text{Earth}}& =G{m}_{\text{Sun}}{r}_{\text{Earth},\text{Sun}}^{-2}{\hat{\mathbf{r}}}_{\text{Earth},\text{Sun}}+G{m}_{\text{Moon}}{r}_{\text{Earth},\text{Moon}}^{-2}{\hat{\mathbf{r}}}_{\text{Earth},\text{Moon}}\\ {\ddot{\mathbf{r}}}_{\text{Moon}}& =G{m}_{\text{Sun}}{r}_{\text{Moon},\text{Sun}}^{-2}{\hat{\mathbf{r}}}_{\text{Moon},\text{Sun}}+G{m}_{\text{Earth}}{r}_{\text{Moon},\text{Earth}}^{-2}{\hat{\mathbf{r}}}_{\text{Moon},\text{Earth}}\end{array}}$$

These accelerations are not those of Kepler orbits, and the three-body problem is complicated. But Keplerian approximation is the basis for perturbation calculations. (See Lunar theory.)

Position as a function of time

Kepler used his two first laws to compute the position of a planet as a function of time. His method involves the solution of a transcendental equation called Kepler's equation.

The procedure for calculating the heliocentric polar coordinates (r,θ) of a planet as a function of the time t since perihelion, is the following five steps:

1. Compute the mean motion n = (2π rad)/P, where P is the period.
2. Compute the mean anomaly M = nt, where t is the time since perihelion.
3. Compute the eccentric anomaly E by solving Kepler's equation:
   $${\displaystyle M=E-\epsilon \sin E,}$$

   
   where ${\displaystyle \epsilon }$ is the eccentricity.
4. Compute the true anomaly θ by solving the equation:
   $${\displaystyle (1-\epsilon ){\tan }^2\frac{\theta }{2}=(1+\epsilon ){\tan }^2\frac{E}{2}}$$

   
5. Compute the heliocentric distance r:
   $${\displaystyle r=a(1-\epsilon \cos E),}$$

   
   where ${\displaystyle a}$ is the semimajor axis.

The Cartesian velocity vector can then be calculated as ${\displaystyle \mathbf{v}=\frac{\sqrt{\mu a}}{r}⟨-\sin E,\sqrt{1-{\epsilon }^2}\cos E⟩}$, where ${\displaystyle \mu }$ is the standard gravitational parameter.

The important special case of circular orbit, ε = 0, gives θ = E = M. Because the uniform circular motion was considered to be normal, a deviation from this motion was considered an anomaly.

The proof of this procedure is shown below.

Mean anomaly, M

Main article: Mean anomaly

Figure 5: Geometric construction for Kepler's calculation of θ. The Sun (located at the focus) is labeled S and the planet P. The auxiliary circle is an aid to calculation. Line xd is perpendicular to the base and through the planet P. The shaded sectors are arranged to have equal areas by positioning of point y.

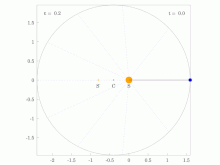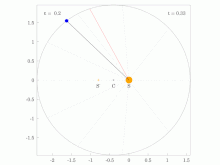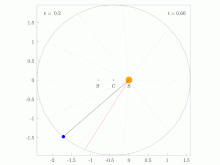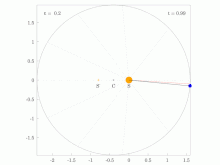
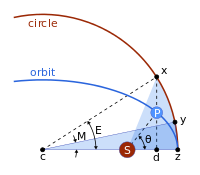
The Keplerian problem assumes an elliptical orbit and the four points:

• s the Sun (at one focus of ellipse);
• z the perihelion
• c the center of the ellipse
• p the planet

and

• ${\displaystyle a=|cz|,}$ distance between center and perihelion, the semimajor axis,
• ${\displaystyle \epsilon =\frac{|cs|}{a},}$ the eccentricity,
• ${\displaystyle b=a\sqrt{1-{\epsilon }^2},}$ the semiminor axis,
• ${\displaystyle r=|sp|,}$ the distance between Sun and planet.
• ${\displaystyle \theta =\mathrm{\angle }zsp,}$ the direction to the planet as seen from the Sun, the true anomaly.

The problem is to compute the polar coordinates (r,θ) of the planet from the time since perihelion, t.

It is solved in steps. Kepler considered the circle with the major axis as a diameter, and

• ${\displaystyle x,}$ the projection of the planet to the auxiliary circle
• ${\displaystyle y,}$ the point on the circle such that the sector areas |zcy| and |zsx| are equal,
• ${\displaystyle M=\mathrm{\angle }zcy,}$ the mean anomaly.

The sector areas are related by ${\displaystyle |zsp|=\frac{b}{a}\cdot |zsx|.}$

The circular sector area ${\displaystyle |zcy|=\frac{a^{2}M}{2}.}$

The area swept since perihelion,

$${\displaystyle |zsp|=\frac{b}{a}\cdot |zsx|=\frac{b}{a}\cdot |zcy|=\frac{b}{a}\cdot \frac{a^{2}M}{2}=\frac{abM}{2},}$$

is by Kepler's second law proportional to time since perihelion. So the mean anomaly, M, is proportional to time since perihelion, t.

$${\displaystyle M=nt,}$$

where n is the mean motion.

Eccentric anomaly, E

When the mean anomaly M is computed, the goal is to compute the true anomaly θ. The function θ = f(M) is, however, not elementary. Kepler's solution is to use

$${\displaystyle E=\mathrm{\angle }zcx,}$$

x as seen from the centre, the eccentric anomaly as an intermediate variable, and first compute E as a function of M by solving Kepler's equation below, and then compute the true anomaly θ from the eccentric anomaly E. Here are the details.

$${\displaystyle \begin{array}{rl}|zcy|& =|zsx|=|zcx|-|scx|\\ \frac{a^{2}M}{2}& =\frac{a^{2}E}{2}-\frac{a\epsilon \cdot a\sin E}{2}\end{array}}$$

Division by a²/2 gives Kepler's equation

$${\displaystyle M=E-\epsilon \sin E.}$$

This equation gives M as a function of E. Determining E for a given M is the inverse problem. Iterative numerical algorithms are commonly used.

Having computed the eccentric anomaly E, the next step is to calculate the true anomaly θ.

But note: Cartesian position coordinates with reference to the center of ellipse are (a cos E, b sin E)

With reference to the Sun (with coordinates (c,0) = (ae,0) ), r = (a cos E – ae, b sin E)

True anomaly would be arctan(r_y/rx), magnitude of r would be √r · r.

True anomaly, θ

Note from the figure that

$${\displaystyle \overrightarrow{cd}=\overrightarrow{cs}+\overrightarrow{sd}}$$

so that

$${\displaystyle a\cos E=a\epsilon +r\cos \theta .}$$

Dividing by ${\displaystyle a}$ and inserting from Kepler's first law

$${\displaystyle \frac{r}{a}=\frac{1-{\epsilon }^2}{1+\epsilon \cos \theta }}$$

to get

$${\displaystyle \cos E=\epsilon +\frac{1-{\epsilon }^2}{1+\epsilon \cos \theta }\cos \theta =\frac{\epsilon (1+\epsilon \cos \theta )+\left(1-{\epsilon }^2\right)\cos \theta }{1+\epsilon \cos \theta }=\frac{\epsilon +\cos \theta }{1+\epsilon \cos \theta }.}$$

The result is a usable relationship between the eccentric anomaly E and the true anomaly θ.

A computationally more convenient form follows by substituting into the trigonometric identity:

$${\displaystyle {\tan }^2\frac{x}{2}=\frac{1-\cos x}{1+\cos x}.}$$

Get

$${\displaystyle \begin{array}{rl}{\tan }^2\frac{E}{2}& =\frac{1-\cos E}{1+\cos E}=\frac{1-\frac{\epsilon +\cos \theta }{1+\epsilon \cos \theta }}{1+\frac{\epsilon +\cos \theta }{1+\epsilon \cos \theta }}\\ & =\frac{(1+\epsilon \cos \theta )-(\epsilon +\cos \theta )}{(1+\epsilon \cos \theta )+(\epsilon +\cos \theta )}=\frac{1-\epsilon }{1+\epsilon }\cdot \frac{1-\cos \theta }{1+\cos \theta }=\frac{1-\epsilon }{1+\epsilon }{\tan }^2\frac{\theta }{2}.\end{array}}$$

Multiplying by 1 + ε gives the result

$${\displaystyle (1-\epsilon ){\tan }^2\frac{\theta }{2}=(1+\epsilon ){\tan }^2\frac{E}{2}}$$

This is the third step in the connection between time and position in the orbit.

Distance, r

The fourth step is to compute the heliocentric distance r from the true anomaly θ by Kepler's first law:

$${\displaystyle r(1+\epsilon \cos \theta )=a\left(1-{\epsilon }^2\right)}$$

Using the relation above between θ and E the final equation for the distance r is:

$${\displaystyle r=a(1-\epsilon \cos E).}$$

Argumentation theory

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Argumentation_theory 

 

Two men argue at a political protest in New York City.

Argumentation theory, or argumentation, is the interdisciplinary study of how conclusions can be supported or undermined by premises through logical reasoning. With historical origins in logic, dialectic, and rhetoric, argumentation theory includes the arts and sciences of civil debate, dialogue, conversation, and persuasion. It studies rules of inference, logic, and procedural rules in both artificial and real-world settings. Argumentation includes various forms of dialogue such as deliberation and negotiation which are concerned with collaborative decision-making procedures. It also encompasses eristic dialog, the branch of social debate in which victory over an opponent is the primary goal, and didactic dialogue used for teaching. This discipline also studies the means by which people can express and rationally resolve or at least manage their disagreements.

Argumentation is a daily occurrence, such as in public debate, science, and law. For example in law, in courts by the judge, the parties and the prosecutor, in presenting and testing the validity of evidences. Also, argumentation scholars study the post hoc rationalizations by which organizational actors try to justify decisions they have made irrationally.

Argumentation is one of four rhetorical modes (also known as modes of discourse), along with exposition, description, and narration.

Key components of argumentation

Some key components of argumentation are:

• Understanding and identifying arguments, either explicit or implied, and the goals of the participants in the different types of dialogue.
• Identifying the premises from which conclusions are derived.
• Establishing the "burden of proof" – determining who made the initial claim and is thus responsible for providing evidence why his/her position merits acceptance.
• For the one carrying the "burden of proof", the advocate, to marshal evidence for his/her position in order to convince or force the opponent's acceptance. The method by which this is accomplished is producing valid, sound, and cogent arguments, devoid of weaknesses, and not easily attacked.
• In a debate, fulfillment of the burden of proof creates a burden of rejoinder. One must try to identify faulty reasoning in the opponent's argument, to attack the reasons/premises of the argument, to provide counterexamples if possible, to identify any fallacies, and to show why a valid conclusion cannot be derived from the reasons provided for his/her argument.

For example, consider the following exchange, illustrated by the No true Scotsman fallacy:

Argument: "No Scotsman puts sugar on his porridge."

Reply: "But my friend Angus likes sugar with his porridge."

Rebuttal: "Ah yes, but no true Scotsman puts sugar on his porridge."

In this dialogue, the proposer first offers a premise, the premise is challenged by the interlocutor, and finally the proposer offers a modification of the premise. This exchange could be part of a larger discussion, for example a murder trial, in which the defendant is a Scotsman, and it had been established earlier that the murderer was eating sugared porridge when he or she committed the murder.

Internal structure of arguments

Typically an argument has an internal structure, comprising the following:

1. a set of assumptions or premises,
2. a method of reasoning or deduction, and
3. a conclusion or point.

An argument has one or more premises and one conclusion.

Often classical logic is used as the method of reasoning so that the conclusion follows logically from the assumptions or support. One challenge is that if the set of assumptions is inconsistent then anything can follow logically from inconsistency. Therefore, it is common to insist that the set of assumptions be consistent. It is also good practice to require the set of assumptions to be the minimal set, with respect to set inclusion, necessary to infer the consequent. Such arguments are called MINCON arguments, short for minimal consistent. Such argumentation has been applied to the fields of law and medicine.

A non-classical approach to argumentation investigates abstract arguments, where 'argument' is considered a primitive term, so no internal structure of arguments is taken into account.

Types of dialogue

In its most common form, argumentation involves an individual and an interlocutor or opponent engaged in dialogue, each contending differing positions and trying to persuade each other, but there are various types of dialogue:

• Persuasion dialogue aims to resolve conflicting points of view of different positions.
• Negotiation aims to resolve conflicts of interests by cooperation and dealmaking.
• Inquiry aims to resolve general ignorance by the growth of knowledge.
• Deliberation aims to resolve a need to take action by reaching a decision.
• Information seeking aims to reduce one party's ignorance by requesting information from another party that is in a position to know something.
• Eristic aims to resolve a situation of antagonism through verbal fighting.

Argumentation and the grounds of knowledge

Argumentation theory had its origins in foundationalism, a theory of knowledge (epistemology) in the field of philosophy. It sought to find the grounds for claims in the forms (logic) and materials (factual laws) of a universal system of knowledge. The dialectical method was made famous by Plato and his use of Socrates critically questioning various characters and historical figures. But argument scholars gradually rejected Aristotle's systematic philosophy and the idealism in Plato and Kant. They questioned and ultimately discarded the idea that argument premises take their soundness from formal philosophical systems. The field thus broadened.

One of the original contributors to this trend was the philosopher Chaïm Perelman, who together with Lucie Olbrechts-Tyteca introduced the French term la nouvelle rhetorique in 1958 to describe an approach to argument which is not reduced to application of formal rules of inference. Perelman's view of argumentation is much closer to a juridical one, in which rules for presenting evidence and rebuttals play an important role.

Karl R. Wallace's seminal essay, "The Substance of Rhetoric: Good Reasons" in the Quarterly Journal of Speech (1963) 44, led many scholars to study "marketplace argumentation" – the ordinary arguments of ordinary people. The seminal essay on marketplace argumentation is Ray Lynn Anderson's and C. David Mortensen's "Logic and Marketplace Argumentation" Quarterly Journal of Speech 53 (1967): 143–150. This line of thinking led to a natural alliance with late developments in the sociology of knowledge. Some scholars drew connections with recent developments in philosophy, namely the pragmatism of John Dewey and Richard Rorty. Rorty has called this shift in emphasis "the linguistic turn".

In this new hybrid approach argumentation is used with or without empirical evidence to establish convincing conclusions about issues which are moral, scientific, epistemic, or of a nature in which science alone cannot answer. Out of pragmatism and many intellectual developments in the humanities and social sciences, "non-philosophical" argumentation theories grew which located the formal and material grounds of arguments in particular intellectual fields. These theories include informal logic, social epistemology, ethnomethodology, speech acts, the sociology of knowledge, the sociology of science, and social psychology. These new theories are not non-logical or anti-logical. They find logical coherence in most communities of discourse. These theories are thus often labeled "sociological" in that they focus on the social grounds of knowledge.

Approaches to argumentation in communication and informal logic

In general, the label "argumentation" is used by communication scholars such as (to name only a few) Wayne E. Brockriede, Douglas Ehninger, Joseph W. Wenzel, Richard Rieke, Gordon Mitchell, Carol Winkler, Eric Gander, Dennis S. Gouran, Daniel J. O'Keefe, Mark Aakhus, Bruce Gronbeck, James Klumpp, G. Thomas Goodnight, Robin Rowland, Dale Hample, C. Scott Jacobs, Sally Jackson, David Zarefsky, and Charles Arthur Willard, while the term "informal logic" is preferred by philosophers, stemming from University of Windsor philosophers Ralph H. Johnson and J. Anthony Blair. Harald Wohlrapp developed a criterion for validness (Geltung, Gültigkeit) as freedom of objections.

Trudy Govier, Douglas N. Walton, Michael Gilbert, Harvey Seigal, Michael Scriven, and John Woods (to name only a few) are other prominent authors in this tradition. Over the past thirty years, however, scholars from several disciplines have co-mingled at international conferences such as that hosted by the University of Amsterdam (the Netherlands) and the International Society for the Study of Argumentation (ISSA). Other international conferences are the biannual conference held at Alta, Utah sponsored by the (US) National Communication Association and American Forensics Association and conferences sponsored by the Ontario Society for the Study of Argumentation (OSSA).

Some scholars (such as Ralph H. Johnson) construe the term "argument" narrowly, as exclusively written discourse or even discourse in which all premises are explicit. Others (such as Michael Gilbert) construe the term "argument" broadly, to include spoken and even nonverbal discourse, for instance the degree to which a war memorial or propaganda poster can be said to argue or "make arguments". The philosopher Stephen Toulmin has said that an argument is a claim on our attention and belief, a view that would seem to authorize treating, say, propaganda posters as arguments. The dispute between broad and narrow theorists is of long standing and is unlikely to be settled. The views of the majority of argumentation theorists and analysts fall somewhere between these two extremes.

Kinds of argumentation

Conversational argumentation

Main articles: Conversation analysis and Discourse analysis

The study of naturally occurring conversation arose from the field of sociolinguistics. It is usually called conversation analysis (CA). Inspired by ethnomethodology, it was developed in the late 1960s and early 1970s principally by the sociologist Harvey Sacks and, among others, his close associates Emanuel Schegloff and Gail Jefferson. Sacks died early in his career, but his work was championed by others in his field, and CA has now become an established force in sociology, anthropology, linguistics, speech-communication and psychology. It is particularly influential in interactional sociolinguistics, discourse analysis and discursive psychology, as well as being a coherent discipline in its own right. Recently CA techniques of sequential analysis have been employed by phoneticians to explore the fine phonetic details of speech.

Empirical studies and theoretical formulations by Sally Jackson and Scott Jacobs, and several generations of their students, have described argumentation as a form of managing conversational disagreement within communication contexts and systems that naturally prefer agreement.

Mathematical argumentation

Main article: Philosophy of mathematics

The basis of mathematical truth has been the subject of long debate. Frege in particular sought to demonstrate (see Gottlob Frege, The Foundations of Arithmetic, 1884, and Begriffsschrift, 1879) that arithmetical truths can be derived from purely logical axioms and therefore are, in the end, logical truths. The project was developed by Russell and Whitehead in their Principia Mathematica. If an argument can be cast in the form of sentences in symbolic logic, then it can be tested by the application of accepted proof procedures. This was carried out for arithmetic using Peano axioms, and the foundation most commonly used for most modern mathematics is Zermelo-Fraenkel set theory, with or without the Axiom of Choice. Be that as it may, an argument in mathematics, as in any other discipline, can be considered valid only if it can be shown that it cannot have true premises and a false conclusion.

Scientific argumentation

Main articles: Philosophy of science and Rhetoric of science

Perhaps the most radical statement of the social grounds of scientific knowledge appears in Alan G.Gross's The Rhetoric of Science (Cambridge: Harvard University Press, 1990). Gross holds that science is rhetorical "without remainder", meaning that scientific knowledge itself cannot be seen as an idealized ground of knowledge. Scientific knowledge is produced rhetorically, meaning that it has special epistemic authority only insofar as its communal methods of verification are trustworthy. This thinking represents an almost complete rejection of the foundationalism on which argumentation was first based.

Interpretive argumentation

Main article: Interpretive discussion

Interpretive argumentation is a dialogical process in which participants explore and/or resolve interpretations often of a text of any medium containing significant ambiguity in meaning.

Interpretive argumentation is pertinent to the humanities, hermeneutics, literary theory, linguistics, semantics, pragmatics, semiotics, analytic philosophy and aesthetics. Topics in conceptual interpretation include aesthetic, judicial, logical and religious interpretation. Topics in scientific interpretation include scientific modeling.

Legal argumentation

By lawyers

Main articles: Oral argument and Closing argument

Legal arguments are spoken presentations to a judge or appellate court by a lawyer, or parties when representing themselves of the legal reasons why they should prevail. Oral argument at the appellate level accompanies written briefs, which also advance the argument of each party in the legal dispute. A closing argument, or summation, is the concluding statement of each party's counsel reiterating the important arguments for the trier of fact, often the jury, in a court case. A closing argument occurs after the presentation of evidence.

By judges

Main articles: Judicial opinion, Legal opinion, and Ratio decidendi

A judicial opinion or legal opinion is in certain jurisdictions a written explanation by a judge or group of judges that accompanies an order or ruling in a case, laying out the rationale (justification) and legal principles for the ruling. It cites the decision reached to resolve the dispute. A judicial opinion usually includes the reasons behind the decision. Where there are three or more judges, it may take the form of a majority opinion, minority opinion or a concurring opinion.

Political argumentation

Main article: Political argument

Political arguments are used by academics, media pundits, candidates for political office and government officials. Political arguments are also used by citizens in ordinary interactions to comment about and understand political events. The rationality of the public is a major question in this line of research. Political scientist Samuel L. Popkin coined the expression "low information voters" to describe most voters who know very little about politics or the world in general.

In practice, a "low information voter" may not be aware of legislation that their representative has sponsored in Congress. A low-information voter may base their ballot box decision on a media sound-bite, or a flier received in the mail. It is possible for a media sound-bite or campaign flier to present a political position for the incumbent candidate that completely contradicts the legislative action taken in the Capitol on behalf of the constituents. It may only take a small percentage of the overall voting group who base their decision on the inaccurate information to form a voter bloc large enough to swing an overall election result. When this happens, the constituency at large may have been duped or fooled. Nevertheless, the election result is legal and confirmed. Savvy Political consultants will take advantage of low-information voters and sway their votes with disinformation and fake news because it can be easier and sufficiently effective. Fact checkers have come about in recent years to help counter the effects of such campaign tactics.

Psychological aspects

Psychology has long studied the non-logical aspects of argumentation. For example, studies have shown that simple repetition of an idea is often a more effective method of argumentation than appeals to reason. Propaganda often utilizes repetition. "Repeat a lie often enough and it becomes the truth" is a law of propaganda often attributed to the Nazi politician Joseph Goebbels. Nazi rhetoric has been studied extensively as, inter alia, a repetition campaign.

Empirical studies of communicator credibility and attractiveness, sometimes labeled charisma, have also been tied closely to empirically-occurring arguments. Such studies bring argumentation within the ambit of persuasion theory and practice.

Some psychologists such as William J. McGuire believe that the syllogism is the basic unit of human reasoning. They have produced a large body of empirical work around McGuire's famous title "A Syllogistic Analysis of Cognitive Relationships". A central line of this way of thinking is that logic is contaminated by psychological variables such as "wishful thinking", in which subjects confound the likelihood of predictions with the desirability of the predictions. People hear what they want to hear and see what they expect to see. If planners want something to happen they see it as likely to happen. If they hope something will not happen, they see it as unlikely to happen. Thus smokers think that they personally will avoid cancer, promiscuous people practice unsafe sex, and teenagers drive recklessly.

Theories

Argument fields

Stephen Toulmin and Charles Arthur Willard have championed the idea of argument fields, the former drawing upon Ludwig Wittgenstein's notion of language games, (Sprachspiel) the latter drawing from communication and argumentation theory, sociology, political science, and social epistemology. For Toulmin, the term "field" designates discourses within which arguments and factual claims are grounded. For Willard, the term "field" is interchangeable with "community", "audience", or "readership". Similarly, G. Thomas Goodnight has studied "spheres" of argument and sparked a large literature created by younger scholars responding to or using his ideas. The general tenor of these field theories is that the premises of arguments take their meaning from social communities.

Stephen E. Toulmin's contributions

The most influential theorist has been Stephen Toulmin, the Cambridge educated philosopher and educator, best known for his Toulmin model of argument. What follows below is a sketch of his ideas.

An alternative to absolutism and relativism

This section is transcluded from Stephen Toulmin. (edit | history)

Throughout many of his works, Toulmin pointed out that absolutism (represented by theoretical or analytic arguments) has limited practical value. Absolutism is derived from Plato's idealized formal logic, which advocates universal truth; accordingly, absolutists believe that moral issues can be resolved by adhering to a standard set of moral principles, regardless of context. By contrast, Toulmin contends that many of these so-called standard principles are irrelevant to real situations encountered by human beings in daily life.

To develop his contention, Toulmin introduced the concept of argument fields. In The Uses of Argument (1958), Toulmin claims that some aspects of arguments vary from field to field, and are hence called "field-dependent", while other aspects of argument are the same throughout all fields, and are hence called "field-invariant". The flaw of absolutism, Toulmin believes, lies in its unawareness of the field-dependent aspect of argument; absolutism assumes that all aspects of argument are field invariant.

In Human Understanding (1972), Toulmin suggests that anthropologists have been tempted to side with relativists because they have noticed the influence of cultural variations on rational arguments. In other words, the anthropologist or relativist overemphasizes the importance of the "field-dependent" aspect of arguments, and neglects or is unaware of the "field-invariant" elements. In order to provide solutions to the problems of absolutism and relativism, Toulmin attempts throughout his work to develop standards that are neither absolutist nor relativist for assessing the worth of ideas.

In Cosmopolis (1990), he traces philosophers' "quest for certainty" back to René Descartes and Thomas Hobbes, and lauds John Dewey, Wittgenstein, Martin Heidegger, and Richard Rorty for abandoning that tradition.

Toulmin model of argument

Toulmin argumentation can be diagrammed as a conclusion established, more or less, on the basis of a fact supported by a warrant (with backing), and a possible rebuttal.

 

Further information: Practical arguments

Arguing that absolutism lacks practical value, Toulmin aimed to develop a different type of argument, called practical arguments (also known as substantial arguments). In contrast to absolutists' theoretical arguments, Toulmin's practical argument is intended to focus on the justificatory function of argumentation, as opposed to the inferential function of theoretical arguments. Whereas theoretical arguments make inferences based on a set of principles to arrive at a claim, practical arguments first find a claim of interest, and then provide justification for it. Toulmin believed that reasoning is less an activity of inference, involving the discovering of new ideas, and more a process of testing and sifting already existing ideas—an act achievable through the process of justification.

Toulmin believed that for a good argument to succeed, it needs to provide good justification for a claim. This, he believed, will ensure it stands up to criticism and earns a favourable verdict. In The Uses of Argument (1958), Toulmin proposed a layout containing six interrelated components for analyzing arguments:

Claim (Conclusion)

A conclusion whose merit must be established. In argumentative essays, it may be called the thesis. For example, if a person tries to convince a listener that he is a British citizen, the claim would be "I am a British citizen" (1).

Ground (Fact, Evidence, Data)

A fact one appeals to as a foundation for the claim. For example, the person introduced in 1 can support his claim with the supporting data "I was born in Bermuda" (2).

Warrant

A statement authorizing movement from the ground to the claim. In order to move from the ground established in 2, "I was born in Bermuda", to the claim in 1, "I am a British citizen", the person must supply a warrant to bridge the gap between 1 and 2 with the statement "A man born in Bermuda will legally be a British citizen" (3).

Backing

Credentials designed to certify the statement expressed in the warrant; backing must be introduced when the warrant itself is not convincing enough to the readers or the listeners. For example, if the listener does not deem the warrant in 3 as credible, the speaker will supply the legal provisions: "I trained as a barrister in London, specialising in citizenship, so I know that a man born in Bermuda will legally be a British citizen".

Rebuttal (Reservation)

Statements recognizing the restrictions which may legitimately be applied to the claim. It is exemplified as follows: "A man born in Bermuda will legally be a British citizen, unless he has betrayed Britain and has become a spy for another country".

Qualifier

Words or phrases expressing the speaker's degree of force or certainty concerning the claim. Such words or phrases include "probably", "possible", "impossible", "certainly", "presumably", "as far as the evidence goes", and "necessarily". The claim "I am definitely a British citizen" has a greater degree of force than the claim "I am a British citizen, presumably". (See also: Defeasible reasoning.)

The first three elements, claim, ground, and warrant, are considered as the essential components of practical arguments, while the second triad, qualifier, backing, and rebuttal, may not be needed in some arguments.

When Toulmin first proposed it, this layout of argumentation was based on legal arguments and intended to be used to analyze the rationality of arguments typically found in the courtroom. Toulmin did not realize that this layout could be applicable to the field of rhetoric and communication until his works were introduced to rhetoricians by Wayne Brockriede and Douglas Ehninger. Their Decision by Debate (1963) streamlined Toulmin's terminology and broadly introduced his model to the field of debate. Only after Toulmin published Introduction to Reasoning (1979) were the rhetorical applications of this layout mentioned in his works.

One criticism of the Toulmin model is that it does not fully consider the use of questions in argumentation. The Toulmin model assumes that an argument starts with a fact or claim and ends with a conclusion, but ignores an argument's underlying questions. In the example "Harry was born in Bermuda, so Harry must be a British subject", the question "Is Harry a British subject?" is ignored, which also neglects to analyze why particular questions are asked and others are not. (See Issue mapping for an example of an argument-mapping method that emphasizes questions.)

Toulmin's argument model has inspired research on, for example, goal structuring notation (GSN), widely used for developing safety cases, and argument maps and associated software.

The evolution of knowledge

This section is transcluded from Stephen Toulmin. (edit | history)

In 1972, Toulmin published Human Understanding, in which he asserts that conceptual change is an evolutionary process. In this book, Toulmin attacks Thomas Kuhn's account of conceptual change in his seminal work The Structure of Scientific Revolutions (1962). Kuhn believed that conceptual change is a revolutionary process (as opposed to an evolutionary process), during which mutually exclusive paradigms compete to replace one another. Toulmin criticized the relativist elements in Kuhn's thesis, arguing that mutually exclusive paradigms provide no ground for comparison, and that Kuhn made the relativists' error of overemphasizing the "field variant" while ignoring the "field invariant" or commonality shared by all argumentation or scientific paradigms.

In contrast to Kuhn's revolutionary model, Toulmin proposed an evolutionary model of conceptual change comparable to Darwin's model of biological evolution. Toulmin states that conceptual change involves the process of innovation and selection. Innovation accounts for the appearance of conceptual variations, while selection accounts for the survival and perpetuation of the soundest conceptions. Innovation occurs when the professionals of a particular discipline come to view things differently from their predecessors; selection subjects the innovative concepts to a process of debate and inquiry in what Toulmin considers as a "forum of competitions". The soundest concepts will survive the forum of competition as replacements or revisions of the traditional conceptions.

From the absolutists' point of view, concepts are either valid or invalid regardless of contexts. From the relativists' perspective, one concept is neither better nor worse than a rival concept from a different cultural context. From Toulmin's perspective, the evaluation depends on a process of comparison, which determines whether or not one concept will improve explanatory power more than its rival concepts.

Pragma-dialectics

Main article: Pragma-dialectics

Scholars at the University of Amsterdam in the Netherlands have pioneered a rigorous modern version of dialectic under the name pragma-dialectics. The intuitive idea is to formulate clear-cut rules that, if followed, will yield reasonable discussion and sound conclusions. Frans H. van Eemeren, the late Rob Grootendorst, and many of their students and co-authors have produced a large body of work expounding this idea.

The dialectical conception of reasonableness is given by ten rules for critical discussion, all being instrumental for achieving a resolution of the difference of opinion (from Van Eemeren, Grootendorst, & Snoeck Henkemans, 2002, p. 182-183). The theory postulates this as an ideal model, and not something one expects to find as an empirical fact. The model can however serve as an important heuristic and critical tool for testing how reality approximates this ideal and point to where discourse goes wrong, that is, when the rules are violated. Any such violation will constitute a fallacy. Albeit not primarily focused on fallacies, pragma-dialectics provides a systematic approach to deal with them in a coherent way.

Van Eemeren and Grootendorst identified four stages of argumentative dialogue. These stages can be regarded as an argument protocol. In a somewhat loose interpretation, the stages are as follows:

• Confrontation stage: Presentation of the difference of opinion, such as a debate question or a political disagreement.
• Opening stage: Agreement on material and procedural starting points, the mutually acceptable common ground of facts and beliefs, and the rules to be followed during the discussion (such as, how evidence is to be presented, and determination of closing conditions).
• Argumentation stage: Presentation of reasons for and against the standpoint(s) at issue, through application of logical and common-sense principles according to the agreed-upon rules
• Concluding stage: Determining whether the standpoint has withstood reasonable criticism, and accepting it is justified. This occurs when the termination conditions are met (Among these could be, for example, a time limitation or the determination of an arbiter.)

Van Eemeren and Grootendorst provide a detailed list of rules that must be applied at each stage of the protocol. Moreover, in the account of argumentation given by these authors, there are specified roles of protagonist and antagonist in the protocol which are determined by the conditions which set up the need for argument.

Walton's logical argumentation method

Douglas N. Walton developed a distinctive philosophical theory of logical argumentation built around a set of practical methods to help a user identify, analyze and evaluate arguments in everyday conversational discourse and in more structured areas such as debate, law and scientific fields. There are four main components: argumentation schemes, dialogue structures, argument mapping tools, and formal argumentation systems. The method uses the notion of commitment in dialogue as the fundamental tool for the analysis and evaluation of argumentation rather than the notion of belief. Commitments are statements that the agent has expressed or formulated, and has pledged to carry out, or has publicly asserted. According to the commitment model, agents interact with each other in a dialogue in which each takes its turn to contribute speech acts. The dialogue framework uses critical questioning as a way of testing plausible explanations and finding weak points in an argument that raise doubt concerning the acceptability of the argument.

Walton's logical argumentation model took a view of proof and justification different from analytic philosophy's dominant epistemology, which was based on a justified true belief framework. In the logical argumentation approach, knowledge is seen as form of belief commitment firmly fixed by an argumentation procedure that tests the evidence on both sides, and uses standards of proof to determine whether a proposition qualifies as knowledge. In this evidence-based approach, knowledge must be seen as defeasible.

Artificial intelligence

See also: Argument technology, Argument mapping, and Argumentation framework

Efforts have been made within the field of artificial intelligence to perform and analyze the act of argumentation with computers. Argumentation has been used to provide a proof-theoretic semantics for non-monotonic logic, starting with the influential work of Dung (1995). Computational argumentation systems have found particular application in domains where formal logic and classical decision theory are unable to capture the richness of reasoning, domains such as law and medicine. In Elements of Argumentation, Philippe Besnard and Anthony Hunter show how classical logic-based techniques can be used to capture key elements of practical argumentation.

Within computer science, the ArgMAS workshop series (Argumentation in Multi-Agent Systems), the CMNA workshop series, and now the COMMA Conference, are regular annual events attracting participants from every continent. The journal Argument & Computation is dedicated to exploring the intersection between argumentation and computer science. ArgMining is a workshop series dedicated specifically to the related argument mining task.

Socratic method

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Socratic_method 

 

Marcello Bacciarelli's Alcibiades Being Taught by Socrates (1776)

The Socratic method (also known as method of Elenchus, elenctic method, or Socratic debate) is a form of cooperative argumentative dialogue between individuals, based on asking and answering questions to stimulate critical thinking and to draw out ideas and underlying presuppositions. It is named after the Classical Greek philosopher Socrates and is introduced by him in Plato's Theaetetus as midwifery (maieutics) because it is employed to bring out definitions implicit in the interlocutors' beliefs, or to help them further their understanding.

The Socratic method is a method of hypothesis elimination, in that better hypotheses are found by steadily identifying and eliminating those that lead to contradictions.

The Socratic method searches for general commonly held truths that shape beliefs and scrutinizes them to determine their consistency with other beliefs. The basic form is a series of questions formulated as tests of logic and fact intended to help a person or group discover their beliefs about some topic, explore definitions, and characterize general characteristics shared by various particular instances.

Development

In the second half of the 5th century BCE, sophists were teachers who specialized in using the tools of philosophy and rhetoric to entertain, impress, or persuade an audience to accept the speaker's point of view. Socrates promoted an alternative method of teaching, which came to be called the Socratic method.

Socrates began to engage in such discussions with his fellow Athenians after his friend from youth, Chaerephon, visited the Oracle of Delphi, which asserted that no man in Greece was wiser than Socrates. Socrates saw this as a paradox, and began using the Socratic method to answer his conundrum. Diogenes Laërtius, however, wrote that Protagoras invented the "Socratic" method.

Plato famously formalized the Socratic elenctic style in prose—presenting Socrates as the curious questioner of some prominent Athenian interlocutor—in some of his early dialogues, such as Euthyphro and Ion, and the method is most commonly found within the so-called "Socratic dialogues", which generally portray Socrates engaging in the method and questioning his fellow citizens about moral and epistemological issues. But in his later dialogues, such as Theaetetus or Sophist, Plato had a different method to philosophical discussions, namely dialectic.

Method

Elenchus (Ancient Greek: ἔλεγχος, romanized: elenkhos, lit. 'argument of disproof or refutation; cross-examining, testing, scrutiny esp. for purposes of refutation') is the central technique of the Socratic method. The Latin form elenchus (plural elenchi) is used in English as the technical philosophical term. The most common adjectival form in English is elenctic; elenchic and elenchtic are also current. This was also very important in Plato's early dialogues.

In Plato's early dialogues, the elenchus is the technique Socrates uses to investigate, for example, the nature or definition of ethical concepts such as justice or virtue. According to Vlastos, it has the following steps:

1. Socrates' interlocutor asserts a thesis, for example "Courage is endurance of the soul".
2. Socrates decides whether the thesis is false and targets for refutation.
3. Socrates secures his interlocutor's agreement to further premises, for example "Courage is a fine thing" and "Ignorant endurance is not a fine thing".
4. Socrates then argues, and the interlocutor agrees, these further premises imply the contrary of the original thesis; in this case, it leads to: "courage is not endurance of the soul".
5. Socrates then claims he has shown his interlocutor's thesis is false and its negation is true.

One elenctic examination can lead to a new, more refined, examination of the concept being considered, in this case it invites an examination of the claim: "Courage is wise endurance of the soul". Most Socratic inquiries consist of a series of elenchi and typically end in puzzlement known as aporia.

Frede^[7] points out Vlastos' conclusion in step #5 above makes nonsense of the aporetic nature of the early dialogues. Having shown a proposed thesis is false is insufficient to conclude some other competing thesis must be true. Rather, the interlocutors have reached aporia, an improved state of still not knowing what to say about the subject under discussion.

The exact nature of the elenchus is subject to a great deal of debate, in particular concerning whether it is a positive method, leading to knowledge, or a negative method used solely to refute false claims to knowledge.

W. K. C. Guthrie in The Greek Philosophers sees it as an error to regard the Socratic method as a means by which one seeks the answer to a problem, or knowledge. Guthrie claims that the Socratic method actually aims to demonstrate one's ignorance. Socrates, unlike the Sophists, did believe that knowledge was possible, but believed that the first step to knowledge was recognition of one's ignorance. Guthrie writes, "[Socrates] was accustomed to say that he did not himself know anything, and that the only way in which he was wiser than other men was that he was conscious of his own ignorance, while they were not. The essence of the Socratic method is to convince the interlocutor that whereas he thought he knew something, in fact he does not."

Application

Socrates generally applied his method of examination to concepts that seem to lack any concrete definition; e.g., the key moral concepts at the time, the virtues of piety, wisdom, temperance, courage, and justice. Such an examination challenged the implicit moral beliefs of the interlocutors, bringing out inadequacies and inconsistencies in their beliefs, and usually resulting in aporia. In view of such inadequacies, Socrates himself professed his ignorance, but others still claimed to have knowledge. Socrates believed that his awareness of his ignorance made him wiser than those who, though ignorant, still claimed knowledge. While this belief seems paradoxical at first glance, it in fact allowed Socrates to discover his own errors where others might assume they were correct. This claim was based on a reported Delphic oracular pronouncement that no man was wiser than Socrates.

Socrates used this claim of wisdom as the basis of his moral exhortation. Accordingly, he claimed that the chief goodness consists in the caring of the soul concerned with moral truth and moral understanding, that "wealth does not bring goodness, but goodness brings wealth and every other blessing, both to the individual and to the state", and that "life without examination [dialogue] is not worth living". It is with this in mind that the Socratic method is employed.

The motive for the modern usage of this method and Socrates' use are not necessarily equivalent. Socrates rarely used the method to actually develop consistent theories, instead using myth to explain them. The Parmenides dialogue shows Parmenides using the Socratic method to point out the flaws in the Platonic theory of forms, as presented by Socrates; it is not the only dialogue in which theories normally expounded by Plato/Socrates are broken down through dialectic. Instead of arriving at answers, the method was used to break down the theories we hold, to go "beyond" the axioms and postulates we take for granted. Therefore, myth and the Socratic method are not meant by Plato to be incompatible; they have different purposes, and are often described as the "left hand" and "right hand" paths to good and wisdom.

Socratic seminar

A Socratic seminar (also known as a Socratic circle) is a pedagogical approach based on the Socratic method and uses a dialogic approach to understand information in a text. Its systematic procedure is used to examine a text through questions and answers founded on the beliefs that all new knowledge is connected to prior knowledge, that all thinking comes from asking questions, and that asking one question should lead to asking further questions. A Socratic seminar is not a debate. The goal of this activity is to have participants work together to construct meaning and arrive at an answer, not for one student or one group to "win the argument".

This approach is based on the belief that participants seek and gain deeper understanding of concepts in the text through thoughtful dialogue rather than memorizing information that has been provided for them. While Socratic seminars can differ in structure, and even in name, they typically involve a passage of text that students must read beforehand and facilitate dialogue. Sometimes, a facilitator will structure two concentric circles of students: an outer circle and an inner circle. The inner circle focuses on exploring and analysing the text through the act of questioning and answering. During this phase, the outer circle remains silent. Students in the outer circle are much like scientific observers watching and listening to the conversation of the inner circle. When the text has been fully discussed and the inner circle is finished talking, the outer circle provides feedback on the dialogue that took place. This process alternates with the inner circle students going to the outer circle for the next meeting and vice versa. The length of this process varies depending on the text used for the discussion. The teacher may decide to alternate groups within one meeting, or they may alternate at each separate meeting.

The most significant difference between this activity and most typical classroom activities involves the role of the teacher. In Socratic seminar, the students lead the discussion and questioning. The teacher's role is to ensure the discussion advances regardless of the particular direction the discussion takes.

Various approaches to Socratic seminar

Teachers use Socratic seminar in different ways. The structure it takes may look different in each classroom. While this is not an exhaustive list, teachers may use one of the following structures to administer Socratic seminar:

1. Inner/outer circle or fishbowl: Students need to be arranged in inner and outer circles. The inner circle engages in discussion about the text. The outer circle observes the inner circle, while taking notes. The outer circle shares their observations and questions the inner circle with guidance from the teacher/facilitator. Students use constructive criticism as opposed to making judgements. The students on the outside keep track of topics they would like to discuss as part of the debrief. Participants in the outer circle can use an observation checklist or notes form to monitor the participants in the inner circle. These tools will provide structure for listening and give the outside members specific details to discuss later in the seminar. The teacher may also sit in the circle but at the same height as the students.
2. Triad: Students are arranged so that each participant (called a "pilot") in the inner circle has two "co-pilots" sitting behind them on either side. Pilots are the speakers because they are in the inner circle; co-pilots are in the outer circle and only speak during consultation. The seminar proceeds as any other seminar. At a point in the seminar, the facilitator pauses the discussion and instructs the triad to talk to each other. Conversation will be about topics that need more in-depth discussion or a question posed by the leader. Sometimes triads will be asked by the facilitator to come up with a new question. Any time during a triad conversation, group members can switch seats and one of the co-pilots can sit in the pilot's seat. Only during that time is the switching of seats allowed. This structure allows for students to speak, who may not yet have the confidence to speak in the large group. This type of seminar involves all students instead of just the students in the inner and outer circles.
3. Simultaneous seminars: Students are arranged in multiple small groups and placed as far as possible from each other. Following the guidelines of the Socratic seminar, students engage in small group discussions. Simultaneous seminars are typically done with experienced students who need little guidance and can engage in a discussion without assistance from a teacher/facilitator. According to the literature, this type of seminar is beneficial for teachers who want students to explore a variety of texts around a main issue or topic. Each small group may have a different text to read/view and discuss. A larger Socratic seminar can then occur as a discussion about how each text corresponds with one another. Simultaneous Seminars can also be used for a particularly difficult text. Students can work through different issues and key passages from the text.

No matter what structure the teacher employs, the basic premise of the seminar/circles is to turn partial control and direction of the classroom over to the students. The seminars encourage students to work together, creating meaning from the text and to stay away from trying to find a correct interpretation. The emphasis is on critical and creative thinking.

Text selection

Socratic seminar texts

A Socratic seminar text is a tangible document that creates a thought-provoking discussion. The text ought to be appropriate for the participants' current level of intellectual and social development. It provides the anchor for dialogue whereby the facilitator can bring the participants back to the text if they begin to digress. Furthermore, the seminar text enables the participants to create a level playing field – ensuring that the dialogical tone within the classroom remains consistent and pure to the subject or topic at hand. Some practitioners argue that "texts" do not have to be confined to printed texts, but can include artifacts such as objects, physical spaces, and the like.

Pertinent elements of an effective Socratic text

Socratic seminar texts are able to challenge participants' thinking skills by having these characteristics:

1. Ideas and values: The text must introduce ideas and values that are complex and difficult to summarize. Powerful discussions arise from personal connections to abstract ideas and from implications to personal values.
2. Complexity and challenge: The text must be rich in ideas and complexity  and open to interpretation. Ideally it should require multiple readings, but should be neither far above the participants' intellectual level nor very long.
3. Relevance to participants' curriculum: An effective text has identifiable themes that are recognizable and pertinent to the lives of the participants. Themes in the text should relate to the curriculum.
4. Ambiguity: The text must be approachable from a variety of different perspectives, including perspectives that seem mutually exclusive, thus provoking critical thinking and raising important questions. The absence of right and wrong answers promotes a variety of discussion and encourages individual contributions.

Two different ways to select a text

Socratic texts can be divided into two main categories:

1. Print texts (e.g., short stories, poems, and essays) and non-print texts (e.g. photographs, sculptures, and maps); and
2. Subject area, which can draw from print or non-print artifacts. As examples, language arts can be approached through poems, history through written or oral historical speeches, science through policies on environmental issues, math through mathematical proofs, health through nutrition labels, and physical education through fitness guidelines.

Questioning methods

Socratic seminars are based upon the interaction of peers. The focus is to explore multiple perspectives on a given issue or topic. Socratic questioning is used to help students apply the activity to their learning. The pedagogy of Socratic questions is open-ended, focusing on broad, general ideas rather than specific, factual information. The questioning technique emphasizes a level of questioning and thinking where there is no single right answer.

Socratic seminars generally start with an open-ended question proposed either by the leader or by another participant. There is no designated first speaker; as individuals participate in Socratic dialogue, they gain experience that enables them to be effective in this role of initial questioner.

The leader keeps the topic focused by asking a variety of questions about the text itself, as well as questions to help clarify positions when arguments become confused. The leader also seeks to coax reluctant participants into the discussion, and to limit contributions from those who tend to dominate. She or he prompts participants to elaborate on their responses and to build on what others have said. The leader guides participants to deepen, clarify, and paraphrase, and to synthesize a variety of different views.

The participants share the responsibility with the leader to maintain the quality of the Socratic circle. They listen actively in order to respond effectively to what others have contributed. This teaches the participants to think and speak persuasively using the discussion to support their position. Participants must demonstrate respect for different ideas, thoughts and values, and must not interrupt each other.

Questions can be created individually or in small groups. All participants are given the opportunity to take part in the discussion. Socratic circles specify three types of questions to prepare:

1. Opening questions generate discussion at the beginning of the seminar in order to elicit dominant themes.
2. Guiding questions help deepen and elaborate the discussion, keeping contributions on topic and encouraging a positive atmosphere and consideration for others.
3. Closing questions lead participants to summarize their thoughts and learning and personalize what they've discussed.

Psychotherapy

The Socratic method, in the form of Socratic questioning, has been adapted for psychotherapy, most prominently in classical Adlerian psychotherapy, logotherapy, rational emotive behavior therapy, cognitive therapy and reality therapy. It can be used to clarify meaning, feeling, and consequences, as well as to gradually unfold insight, or explore alternative actions.

The Socratic method has also recently inspired a new form of applied philosophy: Socratic dialogue, also called philosophical counseling. In Europe Gerd B. Achenbach is probably the best known practitioner, and Michel Weber has also proposed another variant of the practice.

Challenges and disadvantages

Scholars such as Peter Boghossian suggest that although the method improves creative and critical thinking, there is a flip side to the method. He states that the teachers who use this method wait for the students to make mistakes, thus creating negative feelings in the class, exposing the student to possible ridicule and humiliation.

Some have countered this thought by stating that the humiliation and ridicule is not caused by the method, rather it is due to the lack of knowledge of the student. Boghossian mentions that even though the questions may be perplexing, they are not originally meant for it, in fact such questions provoke the students and can be countered by employing counterexamples.