Degenerate energy levels

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Degenerate_energy_levels

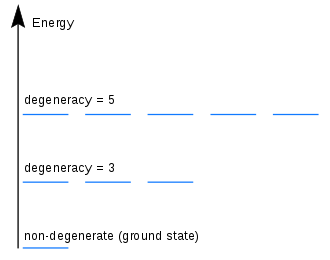
In quantum mechanics, an energy level is degenerate if it corresponds to two or more different measurable states of a quantum system. Conversely, two or more different states of a quantum mechanical system are said to be degenerate if they give the same value of energy upon measurement. The number of different states corresponding to a particular energy level is known as the degree of degeneracy of the level. It is represented mathematically by the Hamiltonian for the system having more than one linearly independent eigenstate with the same energy eigenvalue. When this is the case, energy alone is not enough to characterize what state the system is in, and other quantum numbers are needed to characterize the exact state when distinction is desired. In classical mechanics, this can be understood in terms of different possible trajectories corresponding to the same energy.

Degeneracy plays a fundamental role in quantum statistical mechanics. For an N-particle system in three dimensions, a single energy level may correspond to several different wave functions or energy states. These degenerate states at the same level all have an equal probability of being filled. The number of such states gives the degeneracy of a particular energy level.

Degenerate states in a quantum system

Mathematics

The possible states of a quantum mechanical system may be treated mathematically as abstract vectors in a separable, complex Hilbert space, while the observables may be represented by linear Hermitian operators acting upon them. By selecting a suitable basis, the components of these vectors and the matrix elements of the operators in that basis may be determined. If A is a N × N matrix, X a non-zero vector, and λ is a scalar, such that ${\displaystyle AX=\lambda X}$, then the scalar λ is said to be an eigenvalue of A and the vector X is said to be the eigenvector corresponding to λ. Together with the zero vector, the set of all eigenvectors corresponding to a given eigenvalue λ form a subspace of Cⁿ, which is called the eigenspace of λ. An eigenvalue λ which corresponds to two or more different linearly independent eigenvectors is said to be degenerate, i.e., ${\displaystyle A{X}_1=\lambda X_1}$ and ${\displaystyle A{X}_2=\lambda X_2}$, where ${\displaystyle X_1}$ and ${\displaystyle X_2}$ are linearly independent eigenvectors. The dimension of the eigenspace corresponding to that eigenvalue is known as its degree of degeneracy, which can be finite or infinite. An eigenvalue is said to be non-degenerate if its eigenspace is one-dimensional.

The eigenvalues of the matrices representing physical observables in quantum mechanics give the measurable values of these observables while the eigenstates corresponding to these eigenvalues give the possible states in which the system may be found, upon measurement. The measurable values of the energy of a quantum system are given by the eigenvalues of the Hamiltonian operator, while its eigenstates give the possible energy states of the system. A value of energy is said to be degenerate if there exist at least two linearly independent energy states associated with it. Moreover, any linear combination of two or more degenerate eigenstates is also an eigenstate of the Hamiltonian operator corresponding to the same energy eigenvalue. This clearly follows from the fact that the eigenspace of the energy value eigenvalue λ is a subspace (being the kernel of the Hamiltonian minus λ times the identity), hence is closed under linear combinations.

Proof of the above theorem.

If ${\displaystyle \hat{H}}$ represents the Hamiltonian operator and ${\displaystyle |{\psi }_1⟩}$ and ${\displaystyle |{\psi }_2⟩}$ are two eigenstates corresponding to the same eigenvalue E, then

$${\displaystyle \hat{H}|{\psi }_1⟩=E|{\psi }_1⟩}$$

$${\displaystyle \hat{H}|{\psi }_2⟩=E|{\psi }_2⟩}$$

Let ${\displaystyle |\psi ⟩=c_1|{\psi }_1⟩+c_2|{\psi }_2⟩}$, where ${\displaystyle c_1}$ and ${\displaystyle c_2}$ are complex(in general) constants, be any linear combination of ${\displaystyle |{\psi }_1⟩}$ and ${\displaystyle |{\psi }_2⟩}$. Then,

$${\displaystyle \begin{array}{rl}\hat{H}|\psi ⟩& =\hat{H}(c_1|{\psi }_1⟩+c_2|{\psi }_2⟩)\\ & =c_1\hat{H}|{\psi }_1⟩+c_2\hat{H}|{\psi }_2⟩\\ & =E(c_1|{\psi }_1⟩+c_2|{\psi }_2⟩)\\ & =E|\psi ⟩\end{array}}$$

which shows that ${\displaystyle |\psi ⟩}$ is an eigenstate of ${\displaystyle \hat{H}}$ with the same eigenvalue E.

Effect of degeneracy on the measurement of energy

In the absence of degeneracy, if a measured value of energy of a quantum system is determined, the corresponding state of the system is assumed to be known, since only one eigenstate corresponds to each energy eigenvalue. However, if the Hamiltonian ${\displaystyle \hat{H}}$ has a degenerate eigenvalue ${\displaystyle E_n}$ of degree g_n, the eigenstates associated with it form a vector subspace of dimension g_n. In such a case, several final states can be possibly associated with the same result ${\displaystyle E_n}$, all of which are linear combinations of the g_n orthonormal eigenvectors ${\displaystyle |E_{n,i}⟩}$.

In this case, the probability that the energy value measured for a system in the state ${\displaystyle |\psi ⟩}$ will yield the value ${\displaystyle E_n}$ is given by the sum of the probabilities of finding the system in each of the states in this basis, i.e.

${\displaystyle P(E_n)=\sum _{i=1}^{g_n}|⟨E_{n,i}|\psi ⟩{|}^2}$

Degeneracy in different dimensions

This section intends to illustrate the existence of degenerate energy levels in quantum systems studied in different dimensions. The study of one and two-dimensional systems aids the conceptual understanding of more complex systems.

Degeneracy in one dimension

In several cases, analytic results can be obtained more easily in the study of one-dimensional systems. For a quantum particle with a wave function ${\displaystyle |\psi ⟩}$ moving in a one-dimensional potential ${\displaystyle V(x)}$, the time-independent Schrödinger equation can be written as

${\displaystyle -\frac{{\hslash }^2}{2m}\frac{d^2\psi }{d{x}^2}+V\psi =E\psi }$

Since this is an ordinary differential equation, there are two independent eigenfunctions for a given energy ${\displaystyle E}$ at most, so that the degree of degeneracy never exceeds two. It can be proven that in one dimension, there are no degenerate bound states for normalizable wave functions. A sufficient condition on a piecewise continuous potential ${\displaystyle V}$ and the energy ${\displaystyle E}$ is the existence of two real numbers ${\displaystyle M,x_0}$ with ${\displaystyle M\ne 0}$ such that ${\displaystyle \mathrm{\forall }x>x_0}$ we have ${\displaystyle V(x)-E\ge M^2}$. In particular, ${\displaystyle V}$ is bounded below in this criterion.

Degeneracy in two-dimensional quantum systems

Two-dimensional quantum systems exist in all three states of matter and much of the variety seen in three dimensional matter can be created in two dimensions. Real two-dimensional materials are made of monoatomic layers on the surface of solids. Some examples of two-dimensional electron systems achieved experimentally include MOSFET, two-dimensional superlattices of Helium, Neon, Argon, Xenon etc. and surface of liquid Helium. The presence of degenerate energy levels is studied in the cases of particle in a box and two-dimensional harmonic oscillator, which act as useful mathematical models for several real world systems.

Particle in a rectangular plane

Consider a free particle in a plane of dimensions ${\displaystyle L_x}$ and ${\displaystyle L_y}$ in a plane of impenetrable walls. The time-independent Schrödinger equation for this system with wave function ${\displaystyle |\psi ⟩}$ can be written as

${\displaystyle -\frac{{\hslash }^2}{2m}\left(\frac{{\mathrm{\partial }}^2\psi }{{\mathrm{\partial }x}^2}+\frac{{\mathrm{\partial }}^2\psi }{{\mathrm{\partial }y}^2}\right)=E\psi }$

The permitted energy values are

${\displaystyle E_{n_x,n_y}=\frac{{\pi }^2{\hslash }^2}{2m}\left(\frac{n_x^2}{L_x^2}+\frac{n_y^2}{L_y^2}\right)}$

The normalized wave function is

${\displaystyle {\psi }_{n_x,n_y}(x,y)=\frac{2}{\sqrt{L_x{L}_y}}\sin \left(\frac{n_x\pi x}{L_x}\right)\sin \left(\frac{n_y\pi y}{L_y}\right)}$

where ${\displaystyle n_x,n_y=1,2,\mathrm{3...}}$

So, quantum numbers ${\displaystyle n_x}$ and ${\displaystyle n_y}$ are required to describe the energy eigenvalues and the lowest energy of the system is given by

${\displaystyle E_{1,1}={\pi }^2\frac{{\hslash }^2}{2m}\left(\frac{1}{L_x^2}+\frac{1}{L_y^2}\right)}$

For some commensurate ratios of the two lengths ${\displaystyle L_x}$ and ${\displaystyle L_y}$, certain pairs of states are degenerate. If ${\displaystyle L_x/L_y=p/q}$, where p and q are integers, the states ${\displaystyle (n_x,n_y)}$ and ${\displaystyle (p{n}_y/q,q{n}_x/p)}$ have the same energy and so are degenerate to each other.

Particle in a square box

In this case, the dimensions of the box ${\displaystyle L_x=L_y=L}$ and the energy eigenvalues are given by

${\displaystyle E_{n_x,n_y}=\frac{{\pi }^2{\hslash }^2}{2m{L}^2}(n_x^2+n_y^2)}$

Since ${\displaystyle n_x}$ and ${\displaystyle n_y}$ can be interchanged without changing the energy, each energy level has a degeneracy of at least two when ${\displaystyle n_x}$ and ${\displaystyle n_y}$ are different. Degenerate states are also obtained when the sum of squares of quantum numbers corresponding to different energy levels are the same. For example, the three states (n_x = 7, n_y = 1), (n_x = 1, n_y = 7) and (n_x = n_y = 5) all have ${\displaystyle E=50\frac{{\pi }^2{\hslash }^2}{2m{L}^2}}$ and constitute a degenerate set.

Degrees of degeneracy of different energy levels for a particle in a square box:

${\displaystyle n_x}$	${\displaystyle n_y}$	${\displaystyle E\left(\frac{{\hslash }^2{\pi }^2}{2m{L}^2}\right)}$	Degeneracy
1	1	2	1
2 1	1 2	5 5	2
2	2	8	1
3 1	1 3	10 10	2
3 2	2 3	13 13	2
4 1	1 4	17 17	2
3	3	18	1
...	...	...	...
7 5 1	1 5 7	50 50 50	3
...	...	...	...
8 7 4 1	1 4 7 8	65 65 65 65	4
...	...	...	...
9 7 6 2	2 6 7 9	85 85 85 85	4
...	...	...	...
11 10 5 2	2 5 10 11	125 125 125 125	4
...	...	...	...
14 10 2	2 10 14	200 200 200	3
...	...	...	...
17 13 7	7 13 17	338 338 338	3

Particle in a cubic box

In this case, the dimensions of the box ${\displaystyle L_x=L_y=L_z=L}$ and the energy eigenvalues depend on three quantum numbers.

${\displaystyle E_{n_x,n_y,n_z}=\frac{{\pi }^2{\hslash }^2}{2m{L}^2}(n_x^2+n_y^2+n_z^2)}$

Since ${\displaystyle n_x}$, ${\displaystyle n_y}$ and ${\displaystyle n_z}$ can be interchanged without changing the energy, each energy level has a degeneracy of at least three when the three quantum numbers are not all equal.

Finding a unique eigenbasis in case of degeneracy

If two operators ${\displaystyle \hat{A}}$ and ${\displaystyle \hat{B}}$ commute, i.e. ${\displaystyle [\hat{A},\hat{B}]=0}$, then for every eigenvector ${\displaystyle |\psi ⟩}$ of ${\displaystyle \hat{A}}$, ${\displaystyle \hat{B}|\psi ⟩}$ is also an eigenvector of ${\displaystyle \hat{A}}$ with the same eigenvalue. However, if this eigenvalue, say ${\displaystyle \lambda }$, is degenerate, it can be said that ${\displaystyle \hat{B}|\psi ⟩}$ belongs to the eigenspace ${\displaystyle E_{\lambda }}$ of ${\displaystyle \hat{A}}$, which is said to be globally invariant under the action of ${\displaystyle \hat{B}}$.

For two commuting observables A and B, one can construct an orthonormal basis of the state space with eigenvectors common to the two operators. However, ${\displaystyle \lambda }$ is a degenerate eigenvalue of ${\displaystyle \hat{A}}$, then it is an eigensubspace of ${\displaystyle \hat{A}}$ that is invariant under the action of ${\displaystyle \hat{B}}$, so the representation of ${\displaystyle \hat{B}}$ in the eigenbasis of ${\displaystyle \hat{A}}$ is not a diagonal but a block diagonal matrix, i.e. the degenerate eigenvectors of ${\displaystyle \hat{A}}$ are not, in general, eigenvectors of ${\displaystyle \hat{B}}$. However, it is always possible to choose, in every degenerate eigensubspace of ${\displaystyle \hat{A}}$, a basis of eigenvectors common to ${\displaystyle \hat{A}}$ and ${\displaystyle \hat{B}}$.

Choosing a complete set of commuting observables

If a given observable A is non-degenerate, there exists a unique basis formed by its eigenvectors. On the other hand, if one or several eigenvalues of ${\displaystyle \hat{A}}$ are degenerate, specifying an eigenvalue is not sufficient to characterize a basis vector. If, by choosing an observable ${\displaystyle \hat{B}}$, which commutes with ${\displaystyle \hat{A}}$, it is possible to construct an orthonormal basis of eigenvectors common to ${\displaystyle \hat{A}}$ and ${\displaystyle \hat{B}}$, which is unique, for each of the possible pairs of eigenvalues {a,b}, then ${\displaystyle \hat{A}}$ and ${\displaystyle \hat{B}}$ are said to form a complete set of commuting observables. However, if a unique set of eigenvectors can still not be specified, for at least one of the pairs of eigenvalues, a third observable ${\displaystyle \hat{C}}$, which commutes with both ${\displaystyle \hat{A}}$ and ${\displaystyle \hat{B}}$ can be found such that the three form a complete set of commuting observables.

It follows that the eigenfunctions of the Hamiltonian of a quantum system with a common energy value must be labelled by giving some additional information, which can be done by choosing an operator that commutes with the Hamiltonian. These additional labels required naming of a unique energy eigenfunction and are usually related to the constants of motion of the system.

Degenerate energy eigenstates and the parity operator

The parity operator is defined by its action in the ${\displaystyle |r⟩}$ representation of changing r to −r, i.e.

${\displaystyle ⟨r|P|\psi ⟩=\psi (-r)}$

The eigenvalues of P can be shown to be limited to ${\displaystyle \pm 1}$, which are both degenerate eigenvalues in an infinite-dimensional state space. An eigenvector of P with eigenvalue +1 is said to be even, while that with eigenvalue −1 is said to be odd.

Now, an even operator ${\displaystyle \hat{A}}$ is one that satisfies,

${\displaystyle \tilde{A}=P\hat{A}P}$

${\displaystyle [P,\hat{A}]=0}$

while an odd operator ${\displaystyle \hat{B}}$ is one that satisfies

${\displaystyle P\hat{B}+\hat{B}P=0}$

Since the square of the momentum operator ${\displaystyle {\hat{p}}^2}$ is even, if the potential V(r) is even, the Hamiltonian ${\displaystyle \hat{H}}$ is said to be an even operator. In that case, if each of its eigenvalues are non-degenerate, each eigenvector is necessarily an eigenstate of P, and therefore it is possible to look for the eigenstates of ${\displaystyle \hat{H}}$ among even and odd states. However, if one of the energy eigenstates has no definite parity, it can be asserted that the corresponding eigenvalue is degenerate, and ${\displaystyle P|\psi ⟩}$ is an eigenvector of ${\displaystyle \hat{H}}$ with the same eigenvalue as ${\displaystyle |\psi ⟩}$.

Degeneracy and symmetry

The physical origin of degeneracy in a quantum-mechanical system is often the presence of some symmetry in the system. Studying the symmetry of a quantum system can, in some cases, enable us to find the energy levels and degeneracies without solving the Schrödinger equation, hence reducing effort.

Mathematically, the relation of degeneracy with symmetry can be clarified as follows. Consider a symmetry operation associated with a unitary operator S. Under such an operation, the new Hamiltonian is related to the original Hamiltonian by a similarity transformation generated by the operator S, such that ${\displaystyle H^{\prime }=SH{S}^{-1}=SH{S}^{†}}$, since S is unitary. If the Hamiltonian remains unchanged under the transformation operation S, we have

${\displaystyle SH{S}^{†}=H}$

${\displaystyle SH{S}^{-1}=H}$

${\displaystyle SH=HS}$

${\displaystyle [S,H]=0}$

Now, if ${\displaystyle |\alpha ⟩}$ is an energy eigenstate,

${\displaystyle H|\alpha ⟩=E|\alpha ⟩}$

where E is the corresponding energy eigenvalue.

${\displaystyle HS|\alpha ⟩=SH|\alpha ⟩=SE|\alpha ⟩=ES|\alpha ⟩}$

which means that ${\displaystyle S|\alpha ⟩}$ is also an energy eigenstate with the same eigenvalue E. If the two states ${\displaystyle |\alpha ⟩}$ and ${\displaystyle S|\alpha ⟩}$ are linearly independent (i.e. physically distinct), they are therefore degenerate.

In cases where S is characterized by a continuous parameter ${\displaystyle ϵ}$, all states of the form ${\displaystyle S(ϵ)|\alpha ⟩}$ have the same energy eigenvalue.

Symmetry group of the Hamiltonian

The set of all operators which commute with the Hamiltonian of a quantum system are said to form the symmetry group of the Hamiltonian. The commutators of the generators of this group determine the algebra of the group. An n-dimensional representation of the Symmetry group preserves the multiplication table of the symmetry operators. The possible degeneracies of the Hamiltonian with a particular symmetry group are given by the dimensionalities of the irreducible representations of the group. The eigenfunctions corresponding to a n-fold degenerate eigenvalue form a basis for a n-dimensional irreducible representation of the Symmetry group of the Hamiltonian.

Types of degeneracy

Degeneracies in a quantum system can be systematic or accidental in nature.

Systematic or essential degeneracy

This is also called a geometrical or normal degeneracy and arises due to the presence of some kind of symmetry in the system under consideration, i.e. the invariance of the Hamiltonian under a certain operation, as described above. The representation obtained from a normal degeneracy is irreducible and the corresponding eigenfunctions form a basis for this representation.

Accidental degeneracy

It is a type of degeneracy resulting from some special features of the system or the functional form of the potential under consideration, and is related possibly to a hidden dynamical symmetry in the system.^[4] It also results in conserved quantities, which are often not easy to identify. Accidental symmetries lead to these additional degeneracies in the discrete energy spectrum. An accidental degeneracy can be due to the fact that the group of the Hamiltonian is not complete. These degeneracies are connected to the existence of bound orbits in classical Physics.

Examples: Coulomb and Harmonic Oscillator potentials

For a particle in a central 1/r potential, the Laplace–Runge–Lenz vector is a conserved quantity resulting from an accidental degeneracy, in addition to the conservation of angular momentum due to rotational invariance.

For a particle moving on a cone under the influence of 1/r and r² potentials, centred at the tip of the cone, the conserved quantities corresponding to accidental symmetry will be two components of an equivalent of the Runge-Lenz vector, in addition to one component of the angular momentum vector. These quantities generate SU(2) symmetry for both potentials.

Example: Particle in a constant magnetic field

A particle moving under the influence of a constant magnetic field, undergoing cyclotron motion on a circular orbit is another important example of an accidental symmetry. The symmetry multiplets in this case are the Landau levels which are infinitely degenerate.

Examples

The hydrogen atom

Main article: Hydrogen Atom

In atomic physics, the bound states of an electron in a hydrogen atom show us useful examples of degeneracy. In this case, the Hamiltonian commutes with the total orbital angular momentum ${\displaystyle \widehat{L^2}}$, its component along the z-direction, ${\displaystyle \widehat{L_z}}$, total spin angular momentum ${\displaystyle \widehat{S^2}}$ and its z-component ${\displaystyle \widehat{S_z}}$. The quantum numbers corresponding to these operators are ${\displaystyle l}$, ${\displaystyle m_l}$, ${\displaystyle s}$ (always 1/2 for an electron) and ${\displaystyle m_s}$ respectively.

The energy levels in the hydrogen atom depend only on the principal quantum number n. For a given n, all the states corresponding to ${\displaystyle l=0,\dots ,n-1}$ have the same energy and are degenerate. Similarly for given values of n and l, the ${\displaystyle (2l+1)}$, states with ${\displaystyle m_l=-l,\dots ,l}$ are degenerate. The degree of degeneracy of the energy level E_n is therefore :${\displaystyle \sum _{l=0}^{n-1}(2l+1)=n^2}$, which is doubled if the spin degeneracy is included.

The degeneracy with respect to ${\displaystyle m_l}$ is an essential degeneracy which is present for any central potential, and arises from the absence of a preferred spatial direction. The degeneracy with respect to ${\displaystyle l}$ is often described as an accidental degeneracy, but it can be explained in terms of special symmetries of the Schrödinger equation which are only valid for the hydrogen atom in which the potential energy is given by Coulomb's law.

Isotropic three-dimensional harmonic oscillator

It is a spinless particle of mass m moving in three-dimensional space, subject to a central force whose absolute value is proportional to the distance of the particle from the centre of force.

${\displaystyle F=-kr}$

It is said to be isotropic since the potential ${\displaystyle V(r)}$ acting on it is rotationally invariant, i.e. :${\displaystyle V(r)=1/2\left(m{\omega }^2{r}^2\right)}$

where ${\displaystyle \omega }$ is the angular frequency given by $\sqrt{k/m}$.

Since the state space of such a particle is the tensor product of the state spaces associated with the individual one-dimensional wave functions, the time-independent Schrödinger equation for such a system is given by-

${\displaystyle -\frac{{\hslash }^2}{2m}\left(\frac{{\mathrm{\partial }}^2\psi }{\mathrm{\partial }{x}^2}+\frac{{\mathrm{\partial }}^2\psi }{\mathrm{\partial }{y}^2}+\frac{{\mathrm{\partial }}^2\psi }{\mathrm{\partial }{z}^2}\right)+\frac{1}{2}m{\omega }^2(x^2+y^2+z^2)\psi =E\psi }$

So, the energy eigenvalues are ${\displaystyle E_{n_x,n_y,n_z}=(n_x+n_y+n_z+3/2)\hslash \omega }$

or, ${\displaystyle E_n=(n+3/2)\hslash \omega }$

where n is a non-negative integer. So, the energy levels are degenerate and the degree of degeneracy is equal to the number of different sets ${\displaystyle \{n_x,n_y,n_z\}}$ satisfying

${\displaystyle n_x+n_y+n_z=n}$

The degeneracy of the ${\displaystyle n}$-th state can be found by considering the distribution of ${\displaystyle n}$ quanta across ${\displaystyle n_x}$, ${\displaystyle n_y}$ and ${\displaystyle n_z}$. Having 0 in ${\displaystyle n_x}$ gives ${\displaystyle n+1}$ possibilities for distribution across ${\displaystyle n_y}$ and ${\displaystyle n_z}$. Having 1 quanta in ${\displaystyle n_x}$ gives ${\displaystyle n}$ possibilities across ${\displaystyle n_y}$ and ${\displaystyle n_z}$ and so on. This leads to the general result of ${\displaystyle n-n_x+1}$ and summing over all ${\displaystyle n}$ leads to the degeneracy of the ${\displaystyle n}$-th state,

${\displaystyle \sum _{n_x=0}^n(n-n_x+1)=\frac{(n+1)(n+2)}{2}}$

As shown, only the ground state where ${\displaystyle n=0}$ is non-degenerate (ie, has a degeneracy of ${\displaystyle 1}$).

Removing degeneracy

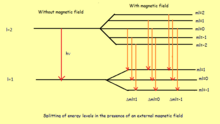
The degeneracy in a quantum mechanical system may be removed if the underlying symmetry is broken by an external perturbation. This causes splitting in the degenerate energy levels. This is essentially a splitting of the original irreducible representations into lower-dimensional such representations of the perturbed system.

Mathematically, the splitting due to the application of a small perturbation potential can be calculated using time-independent degenerate perturbation theory. This is an approximation scheme that can be applied to find the solution to the eigenvalue equation for the Hamiltonian H of a quantum system with an applied perturbation, given the solution for the Hamiltonian H₀ for the unperturbed system. It involves expanding the eigenvalues and eigenkets of the Hamiltonian H in a perturbation series. The degenerate eigenstates with a given energy eigenvalue form a vector subspace, but not every basis of eigenstates of this space is a good starting point for perturbation theory, because typically there would not be any eigenstates of the perturbed system near them. The correct basis to choose is one that diagonalizes the perturbation Hamiltonian within the degenerate subspace.

Physical examples of removal of degeneracy by a perturbation

Some important examples of physical situations where degenerate energy levels of a quantum system are split by the application of an external perturbation are given below.

Symmetry breaking in two-level systems

See also: Avoided crossing § In two state systems

A two-level system essentially refers to a physical system having two states whose energies are close together and very different from those of the other states of the system. All calculations for such a system are performed on a two-dimensional subspace of the state space.

If the ground state of a physical system is two-fold degenerate, any coupling between the two corresponding states lowers the energy of the ground state of the system, and makes it more stable.

If ${\displaystyle E_1}$ and ${\displaystyle E_2}$ are the energy levels of the system, such that ${\displaystyle E_1=E_2=E}$, and the perturbation ${\displaystyle W}$ is represented in the two-dimensional subspace as the following 2×2 matrix

${\displaystyle \mathbf{W}=\left[\begin{array}{cc}0& W_{12}\\ W_{12}^{\ast }& 0\end{array}\right].}$

then the perturbed energies are

${\displaystyle E_{+}=E+|W_{12}|}$

${\displaystyle E_{-}=E-|W_{12}|}$

Examples of two-state systems in which the degeneracy in energy states is broken by the presence of off-diagonal terms in the Hamiltonian resulting from an internal interaction due to an inherent property of the system include:

• Benzene, with two possible dispositions of the three double bonds between neighbouring Carbon atoms.
• Ammonia molecule, where the Nitrogen atom can be either above or below the plane defined by the three Hydrogen atoms.
• H⁺
  ₂ molecule, in which the electron may be localized around either of the two nuclei.

Fine-structure splitting

Main article: Fine structure

The corrections to the Coulomb interaction between the electron and the proton in a Hydrogen atom due to relativistic motion and spin–orbit coupling result in breaking the degeneracy in energy levels for different values of l corresponding to a single principal quantum number n.

The perturbation Hamiltonian due to relativistic correction is given by

${\displaystyle H_r=-p^4/8m^3{c}^2}$

where ${\displaystyle p}$ is the momentum operator and ${\displaystyle m}$ is the mass of the electron. The first-order relativistic energy correction in the ${\displaystyle |nlm⟩}$ basis is given by

${\displaystyle E_r=(-1/8m^3{c}^2)⟨nlm|p^4|nlm⟩}$

Now ${\displaystyle p^4=4m^2(H^0+e^2/r{)}^2}$

${\displaystyle \begin{array}{rl}{E}_r& =(-1/2m{c}^2)[E_n^2+2E_n{e}^2⟨1/r⟩+e^4⟨1/r^2⟩]\\ & =(-1/2)m{c}^2{\alpha }^4[-3/(4n^4)+1/n^3(l+1/2)]\end{array}}$

where ${\displaystyle \alpha }$ is the fine structure constant.

The spin–orbit interaction refers to the interaction between the intrinsic magnetic moment of the electron with the magnetic field experienced by it due to the relative motion with the proton. The interaction Hamiltonian is

${\displaystyle H_{so}=-(e/mc)\overrightarrow{m}\cdot \overrightarrow{L}/r^3=[(e^2/(m^2{c}^2{r}^3))\overrightarrow{S}\cdot \overrightarrow{L}]}$

which may be written as

${\displaystyle H_{so}=(e^2/(4m^2{c}^2{r}^3))[{\overrightarrow{J}}^2-{\overrightarrow{L}}^2-{\overrightarrow{S}}^2]}$

The first order energy correction in the ${\displaystyle |j,m,l,1/2⟩}$ basis where the perturbation Hamiltonian is diagonal, is given by

${\displaystyle E_{so}=({\hslash }^2{e}^2)/(4m^2{c}^2)[j(j+1)-l(l+1)-3/4]/((a_0{)}^3{n}^3(l(l+1/2)(l+1))]}$

where ${\displaystyle a_0}$ is the Bohr radius. The total fine-structure energy shift is given by

${\displaystyle E_{fs}=-(m{c}^2{\alpha }^4/(2n^3))[1/(j+1/2)-3/4n]}$

for ${\displaystyle j=l\pm 1/2}$.

Zeeman effect

Main article: Zeeman effect

The splitting of the energy levels of an atom when placed in an external magnetic field because of the interaction of the magnetic moment ${\displaystyle \overrightarrow{m}}$ of the atom with the applied field is known as the Zeeman effect.

Taking into consideration the orbital and spin angular momenta, ${\displaystyle \overrightarrow{L}}$ and ${\displaystyle \overrightarrow{S}}$, respectively, of a single electron in the Hydrogen atom, the perturbation Hamiltonian is given by

${\displaystyle \hat{V}=-(\overrightarrow{m_l}+\overrightarrow{m_s})\cdot \overrightarrow{B}}$

where ${\displaystyle m_l=-e\overrightarrow{L}/2m}$ and ${\displaystyle m_s=-e\overrightarrow{S}/m}$. Thus,

${\displaystyle \hat{V}=e(\overrightarrow{L}+2\overrightarrow{S})\cdot \overrightarrow{B}/2m}$

Now, in case of the weak-field Zeeman effect, when the applied field is weak compared to the internal field, the spin–orbit coupling dominates and ${\displaystyle \overrightarrow{L}}$ and ${\displaystyle \overrightarrow{S}}$ are not separately conserved. The good quantum numbers are n, l, j and m_j, and in this basis, the first order energy correction can be shown to be given by

${\displaystyle E_z=-{\mu }_B{g}_{j}B{m}_j}$, where

${\displaystyle {\mu }_B=e\hslash /2m}$ is called the Bohr Magneton.Thus, depending on the value of ${\displaystyle m_j}$, each degenerate energy level splits into several levels.

Lifting of degeneracy by an external magnetic field

In case of the strong-field Zeeman effect, when the applied field is strong enough, so that the orbital and spin angular momenta decouple, the good quantum numbers are now n, l, m_l, and m_s. Here, L_z and S_z are conserved, so the perturbation Hamiltonian is given by-

${\displaystyle \hat{V}=eB(L_z+2S_z)/2m}$

assuming the magnetic field to be along the z-direction. So,

${\displaystyle \hat{V}=eB(m_l+2m_s)/2m}$

For each value of m_l, there are two possible values of m_s, ${\displaystyle \pm 1/2}$.

Stark effect

Main article: Stark effect

The splitting of the energy levels of an atom or molecule when subjected to an external electric field is known as the Stark effect.

For the hydrogen atom, the perturbation Hamiltonian is

${\displaystyle {\hat{H}}_s=-|e|Ez}$

if the electric field is chosen along the z-direction.

The energy corrections due to the applied field are given by the expectation value of ${\displaystyle {\hat{H}}_s}$ in the ${\displaystyle |nlm⟩}$ basis. It can be shown by the selection rules that ${\displaystyle ⟨nl{m}_l|z|n_1{l}_1{m}_{l1}⟩\ne 0}$ when ${\displaystyle l=l_1\pm 1}$ and ${\displaystyle m_l=m_{l1}}$.

The degeneracy is lifted only for certain states obeying the selection rules, in the first order. The first-order splitting in the energy levels for the degenerate states ${\displaystyle |2,0,0⟩}$ and ${\displaystyle |2,1,0⟩}$, both corresponding to n = 2, is given by ${\displaystyle \mathrm{\Delta }{E}_{2,1,m_l}=\pm |e|({\hslash }^2)/(m_e{e}^2)E}$.

Electrical network

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Electrical_network

 

A simple electric circuit made up of a voltage source and a resistor. Here, ${\displaystyle v=iR}$, according to Ohm's law.

An electrical network is an interconnection of electrical components (e.g., batteries, resistors, inductors, capacitors, switches, transistors) or a model of such an interconnection, consisting of electrical elements (e.g., voltage sources, current sources, resistances, inductances, capacitances). An electrical circuit is a network consisting of a closed loop, giving a return path for the current. Thus all circuits are networks, but not all networks are circuits (although networks without a closed loop are often imprecisely referred to as "circuits"). Linear electrical networks, a special type consisting only of sources (voltage or current), linear lumped elements (resistors, capacitors, inductors), and linear distributed elements (transmission lines), have the property that signals are linearly superimposable. They are thus more easily analyzed, using powerful frequency domain methods such as Laplace transforms, to determine DC response, AC response, and transient response.

A resistive network is a network containing only resistors and ideal current and voltage sources. Analysis of resistive networks is less complicated than analysis of networks containing capacitors and inductors. If the sources are constant (DC) sources, the result is a DC network. The effective resistance and current distribution properties of arbitrary resistor networks can be modeled in terms of their graph measures and geometrical properties.

A network that contains active electronic components is known as an electronic circuit. Such networks are generally nonlinear and require more complex design and analysis tools.

Classification

By passivity

An active network contains at least one voltage source or current source that can supply energy to the network indefinitely. A passive network does not contain an active source.

An active network contains one or more sources of electromotive force. Practical examples of such sources include a battery or a generator. Active elements can inject power to the circuit, provide power gain, and control the current flow within the circuit.

Passive networks do not contain any sources of electromotive force. They consist of passive elements like resistors and capacitors.

By linearity

A network is linear if its signals obey the principle of superposition; otherwise it is non-linear. Passive networks are generally taken to be linear, but there are exceptions. For instance, an inductor with an iron core can be driven into saturation if driven with a large enough current. In this region, the behaviour of the inductor is very non-linear.

By lumpiness

Discrete passive components (resistors, capacitors and inductors) are called lumped elements because all of their, respectively, resistance, capacitance and inductance is assumed to be located ("lumped") at one place. This design philosophy is called the lumped-element model and networks so designed are called lumped-element circuits. This is the conventional approach to circuit design. At high enough frequencies, or for long enough circuits (such as power transmission lines), the lumped assumption no longer holds because there is a significant fraction of a wavelength across the component dimensions. A new design model is needed for such cases called the distributed-element model. Networks designed to this model are called distributed-element circuits.

A distributed-element circuit that includes some lumped components is called a semi-lumped design. An example of a semi-lumped circuit is the combline filter.

Classification of sources

Sources can be classified as independent sources and dependent sources.

Independent

An ideal independent source maintains the same voltage or current regardless of the other elements present in the circuit. Its value is either constant (DC) or sinusoidal (AC). The strength of voltage or current is not changed by any variation in the connected network.

Dependent

Dependent sources depend upon a particular element of the circuit for delivering the power or voltage or current depending upon the type of source it is.

Applying electrical laws

A number of electrical laws apply to all linear resistive networks. These include:

• Kirchhoff's current law: The sum of all currents entering a node is equal to the sum of all currents leaving the node.

• Kirchhoff's voltage law: The directed sum of the electrical potential differences around a loop must be zero.

• Ohm's law: The voltage across a resistor is equal to the product of the resistance and the current flowing through it.

• Norton's theorem: Any network of voltage or current sources and resistors is electrically equivalent to an ideal current source in parallel with a single resistor.

• Thévenin's theorem: Any network of voltage or current sources and resistors is electrically equivalent to a single voltage source in series with a single resistor.

• Superposition theorem: In a linear network with several independent sources, the response in a particular branch when all the sources are acting simultaneously is equal to the linear sum of individual responses calculated by taking one independent source at a time.

Applying these laws results in a set of simultaneous equations that can be solved either algebraically or numerically. The laws can generally be extended to networks containing reactances. They cannot be used in networks that contain nonlinear or time-varying components.

Design methods

Linear network analysis
Elements
Components
Series and parallel circuits
Impedance transforms
Generator theorems	Network theorems
Network analysis methods
Two-port parameters

See also: Network analysis (electrical circuits)

To design any electrical circuit, either analog or digital, electrical engineers need to be able to predict the voltages and currents at all places within the circuit. Simple linear circuits can be analyzed by hand using complex number theory. In more complex cases the circuit may be analyzed with specialized computer programs or estimation techniques such as the piecewise-linear model.

Circuit simulation software, such as HSPICE (an analog circuit simulator), and languages such as VHDL-AMS and verilog-AMS allow engineers to design circuits without the time, cost and risk of error involved in building circuit prototypes.

Network simulation software

More complex circuits can be analyzed numerically with software such as SPICE or GNUCAP, or symbolically using software such as SapWin.

Linearization around operating point

When faced with a new circuit, the software first tries to find a steady state solution, that is, one where all nodes conform to Kirchhoff's current law and the voltages across and through each element of the circuit conform to the voltage/current equations governing that element.

Once the steady state solution is found, the operating points of each element in the circuit are known. For a small signal analysis, every non-linear element can be linearized around its operation point to obtain the small-signal estimate of the voltages and currents. This is an application of Ohm's Law. The resulting linear circuit matrix can be solved with Gaussian elimination.

Piecewise-linear approximation

Software such as the PLECS interface to Simulink uses piecewise-linear approximation of the equations governing the elements of a circuit. The circuit is treated as a completely linear network of ideal diodes. Every time a diode switches from on to off or vice versa, the configuration of the linear network changes. Adding more detail to the approximation of equations increases the accuracy of the simulation, but also increases its running time.

Prototype

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Prototype

 

Prototype signage on the Boise Greenbelt, testing for rust, paint-fastness, durability, etc.

 

A sign explaining prototype signage

A prototype is an early sample, model, or release of a product built to test a concept or process. It is a term used in a variety of contexts, including semantics, design, electronics, and software programming. A prototype is generally used to evaluate a new design to enhance precision by system analysts and usersPrototyping serves to provide specifications for a real, working system rather than a theoretical one. In some design workflow models, creating a prototype (a process sometimes called materialization) is the step between the formalization and the evaluation of an idea.

A prototype can also mean a typical example of something such as in the use of the derivation 'prototypical'. This is a useful term in identifying objects, behaviours and concepts which are considered the accepted norm and is analogous with terms such as stereotypes and archetypes.

The word prototype derives from the Greek πρωτότυπον prototypon, "primitive form", neutral of πρωτότυπος prototypos, "original, primitive", from πρῶτος protos, "first" and τύπος typos, "impression" (originally in the sense of a mark left by a blow, then by a stamp struck by a die (note "typewriter"); by implication a scar or mark; by analogy a shape i.e. a statue, (figuratively) style, or resemblance; a model for imitation or illustrative example—note "typical").

Types

Prototypes explore different aspects of an intended design:

• A proof-of-principle prototype serves to verify some key functional aspects of the intended design, but usually does not have all the functionality of the final product.
• A working prototype represents all or nearly all of the functionality of the final product.
• A visual prototype represents the size and appearance, but not the functionality, of the intended design. A form study prototype is a preliminary type of visual prototype in which the geometric features of a design are emphasized, with less concern for color, texture, or other aspects of the final appearance.
• A user experience prototype represents enough of the appearance and function of the product that it can be used for user research.
• A functional prototype captures both function and appearance of the intended design, though it may be created with different techniques and even different scale from final design.
• A paper prototype is a printed or hand-drawn representation of the user interface of a software product. Such prototypes are commonly used for early testing of a software design, and can be part of a software walkthrough to confirm design decisions before more costly levels of design effort are expended.

Differences in creating a prototype vs. a final product

In general, the creation of prototypes will differ from creation of the final product in some fundamental ways:

• Material: The materials that will be used in a final product may be expensive or difficult to fabricate, so prototypes may be made from different materials than the final product. In some cases, the final production materials may still be undergoing development themselves and not yet available for use in a prototype.
• Process: Mass-production processes are often unsuitable for making a small number of parts, so prototypes may be made using different fabrication processes than the final product. For example, a final product that will be made by plastic injection molding will require expensive custom tooling, so a prototype for this product may be fabricated by machining or stereolithography instead. Differences in fabrication process may lead to differences in the appearance of the prototype as compared to the final product.
• Verification: The final product may be subject to a number of quality assurance tests to verify conformance with drawings or specifications. These tests may involve custom inspection fixtures, statistical sampling methods, and other techniques appropriate for ongoing production of a large quantity of the final product. Prototypes are generally made with much closer individual inspection and the assumption that some adjustment or rework will be part of the fabrication process. Prototypes may also be exempted from some requirements that will apply to the final product.

Engineers and prototype specialists attempt to minimize the impact of these differences on the intended role for the prototype. For example, if a visual prototype is not able to use the same materials as the final product, they will attempt to substitute materials with properties that closely simulate the intended final materials.

Characteristics and limitations of prototypes

A prototype of the Polish economy hatchback car Beskid 106 designed in the 1980s

Engineers and prototyping specialists seek to understand the limitations of prototypes to exactly simulate the characteristics of their intended design.

It is important to recognize that by their very nature, prototypes represent some compromise from the final production design. This is due to not just the skill and choices of the designer(s), but the inevitable inherent limitations of a prototype due to the "map-territory relation". Just as a map is a reduced abstraction representing far more detailed actual territory, or "the menu represents the meal" but cannot capture all the detail of the actual delivered food: a prototype is a necessarily inexact and limited approximation of a "real" final product.

Further, prototypers make both deliberate and unintended choices and tradeoffs for reasons ranging from cost/time savings to what they consider "important" vs. "trivial" aspects to focus design attention and execution on. Due to differences in materials, processes and design fidelity, it is possible that a prototype may fail to perform acceptably although the production design may have been sound. Conversely, and somewhat counter-intuitively: prototypes may actually perform acceptably but the production design and outcome may prove unsuccessful, as prototyping materials and processes may actually outperform their production counterparts.

In general, it can be expected that individual prototype costs will be substantially greater than the final production costs due to inefficiencies in materials and processes. Prototypes are also used to revise the design for the purposes of reducing costs through optimization and refinement.

It is possible to use prototype testing to reduce the risk that a design may not perform as intended, however prototypes generally cannot eliminate all risk. There are pragmatic and practical limitations to the ability of a prototype to match the intended final performance of the product and some allowances and engineering judgement are often required before moving forward with a production design.

Building the full design is often expensive and can be time-consuming, especially when repeated several times—building the full design, figuring out what the problems are and how to solve them, then building another full design. As an alternative, rapid prototyping or rapid application development techniques are used for the initial prototypes, which implement part, but not all, of the complete design. This allows designers and manufacturers to rapidly and inexpensively test the parts of the design that are most likely to have problems, solve those problems, and then build the full design.

This counter-intuitive idea—that the quickest way to build something is, first to build something else—is shared by scaffolding and Thomson's telescope rule.

Engineering sciences

In technology research, a technology demonstrator is a prototype serving as proof-of-concept and demonstration model for a new technology or future product, proving its viability and illustrating conceivable applications.

In large development projects, a testbed is a platform and prototype development environment for rigorous experimentation and testing of new technologies, components, scientific theories and computational tools.

With recent advances in computer modeling it is becoming practical to eliminate the creation of a physical prototype (except possibly at greatly reduced scales for promotional purposes), instead modeling all aspects of the final product as a computer model. An example of such a development can be seen in Boeing 787 Dreamliner, in which the first full sized physical realization is made on the series production line. Computer modeling is now being extensively used in automotive design, both for form (in the styling and aerodynamics of the vehicle) and in function—especially for improving vehicle crashworthiness and in weight reduction to improve mileage.

Mechanical and electrical engineering

The most common use of the word prototype is a functional, although experimental, version of a non-military machine (e.g., automobiles, domestic appliances, consumer electronics) whose designers would like to have built by mass production means, as opposed to a mockup, which is an inert representation of a machine's appearance, often made of some non-durable substance.

An electronics designer often builds the first prototype from breadboard or stripboard or perfboard, typically using "DIP" packages.

However, more and more often the first functional prototype is built on a "prototype PCB" almost identical to the production PCB, as PCB manufacturing prices fall and as many components are not available in DIP packages, but only available in SMT packages optimized for placing on a PCB.

Builders of military machines and aviation prefer the terms "experimental" and "service test".

Electronics

A simple electronic circuit prototype on a breadboard

 

Example of prototype in optoelectronics (Texas Instruments, DLP Cinema Prototype System)

In electronics, prototyping means building an actual circuit to a theoretical design to verify that it works, and to provide a physical platform for debugging it if it does not. The prototype is often constructed using techniques such as wire wrapping or using a breadboard, stripboard or perfboard, with the result being a circuit that is electrically identical to the design but not physically identical to the final product.

Open-source tools like Fritzing exist to document electronic prototypes (especially the breadboard-based ones) and move toward physical production. Prototyping platforms such as Arduino also simplify the task of programming and interacting with a microcontroller. The developer can choose to deploy their invention as-is using the prototyping platform, or replace it with only the microcontroller chip and the circuitry that is relevant to their product.

A technician can quickly build a prototype (and make additions and modifications) using these techniques, but for volume production it is much faster and usually cheaper to mass-produce custom printed circuit boards than to produce these other kinds of prototype boards. The proliferation of quick-turn PCB fabrication and assembly companies has enabled the concepts of rapid prototyping to be applied to electronic circuit design. It is now possible, even with the smallest passive components and largest fine-pitch packages, to have boards fabricated, assembled, and even tested in a matter of days.

Computer programming and computer science

Main articles: Software prototyping and Software release cycle

Prototype software is often referred to as alpha grade, meaning it is the first version to run. Often only a few functions are implemented, the primary focus of the alpha is to have a functional base code on to which features may be added. Once alpha grade software has most of the required features integrated into it, it becomes beta software for testing of the entire software and to adjust the program to respond correctly during situations unforeseen during development.

Often the end users may not be able to provide a complete set of application objectives, detailed input, processing, or output requirements in the initial stage. After the user evaluation, another prototype will be built based on feedback from users, and again the cycle returns to customer evaluation. The cycle starts by listening to the user, followed by building or revising a mock-up, and letting the user test the mock-up, then back. There is now a new generation of tools called Application Simulation Software which help quickly simulate application before their development.

Extreme programming uses iterative design to gradually add one feature at a time to the initial prototype.

Other programming/computing concepts

In many programming languages, a function prototype is the declaration of a subroutine or function (and should not be confused with software prototyping). This term is rather C/C++-specific; other terms for this notion are signature, type and interface. In prototype-based programming (a form of object-oriented programming), new objects are produced by cloning existing objects, which are called prototypes.

The term may also refer to the Prototype Javascript Framework.

Additionally, the term may refer to the prototype design pattern.

Continuous learning approaches within organizations or businesses may also use the concept of business or process prototypes through software models.

The concept of prototypicality is used to describe how much a website deviates from the expected norm, and leads to a lowering of user preference for that site's design.

Data prototyping

A data prototype is a form of functional or working prototype. The justification for its creation is usually a data migration, data integration or application implementation project and the raw materials used as input are an instance of all the relevant data which exists at the start of the project.

The objectives of data prototyping are to produce:

• A set of data cleansing and transformation rules which have been seen to produce data which is all fit for purpose.
• A dataset which is the result of those rules being applied to an instance of the relevant raw (source) data.

To achieve this, a data architect uses a graphical interface to interactively develop and execute transformation and cleansing rules using raw data. The resultant data is then evaluated and the rules refined. Beyond the obvious visual checking of the data on-screen by the data architect, the usual evaluation and validation approaches are to use Data profiling software and then to insert the resultant data into a test version of the target application and trial its use.

Prototyping for Human-Computer Interaction

When developing software or digital tools that humans interact with, a prototype is an artifact that is used to ask and answer a design question. Prototypes provide the means for examining design problems and evaluating solutions.

HCI practitioners can employ several different types of prototypes:

• 'Wizard of Oz' prototype: named after the Wizard of Oz in the film The Wizard of Oz. This is a prototyping method with which the computer-side of the interaction is faked by an offsite or hidden human. This prototyping technique is particularly useful for demonstrating functionality that is difficult or lengthy to engineer, such as applications like voice user interface.
• role prototype: this prototype may not be engineered or look and feel like a finished product, but the purpose of this type of prototype is to investigate and evaluation a user need, or what the prototype could do for the user. They can present features and functionality that the user might benefit from, to demonstrate what role an artifact like the prototype might fulfill for the user. A famous example of this kind of prototype would be the block of wood carried by Jeff Hawkins, when developing the Palm Pilot.
• paper prototype: this prototype may use cut paper, cardboard, or other inexpensive materials to demonstrate an interface. The purpose of this prototype is to test with users, without having to use a digital tool or develop a program to test functionality. Recently, paper prototyping has fallen out of favor within certain design circles, particularly because the low-fidelity nature of this method and the lack of effectiveness when testing with users.

Scale modeling

A scale model of an Douglas SB2D Destroyer in a wind tunnel for testing

In the field of scale modeling (which includes model railroading, vehicle modeling, airplane modeling, military modeling, etc.), a prototype is the real-world basis or source for a scale model—such as the real EMD GP38-2 locomotive—which is the prototype of Athearn's (among other manufacturers) locomotive model. Technically, any non-living object can serve as a prototype for a model, including structures, equipment, and appliances, and so on, but generally prototypes have come to mean full-size real-world vehicles including automobiles (the prototype 1957 Chevy has spawned many models), military equipment (such as M4 Shermans, a favorite among US Military modelers), railroad equipment, motor trucks, motorcycles, and space-ships (real-world such as Apollo/Saturn Vs, or the ISS). As of 2014, basic rapid prototype machines (such as 3D printers) cost about $2,000, but larger and more precise machines can cost as much as $500,000.

Architecture

In architecture, prototyping refers to either architectural model making (as form of scale modelling) or as part of aesthetic or material experimentation, such as the Forty Wall House open source material prototyping centre in Australia.

Architects prototype to test ideas structurally, aesthetically and technically. Whether the prototype works or not is not the primary focus: architectural prototyping is the revelatory process through which the architect gains insight.

Metrology

In the science and practice of metrology, a prototype is a human-made object that is used as the standard of measurement of some physical quantity to base all measurement of that physical quantity against. Sometimes this standard object is called an artifact. In the International System of Units (SI), there remains no prototype standard since May 20, 2019. Before that date, the last prototype used was the international prototype of the kilogram, a solid platinum-iridium cylinder kept at the Bureau International des Poids et Mesures (International Bureau of Weights and Measures) in Sèvres France (a suburb of Paris) that by definition was the mass of exactly one kilogram. Copies of this prototype are fashioned and issued to many nations to represent the national standard of the kilogram and are periodically compared to the Paris prototype. Now the kilogram is redefined in such a way that the Planck constant h is prescribed a value of exactly 6.62607015×10⁻³⁴ joule-second (J⋅s)

Until 1960, the meter was defined by a platinum-iridium prototype bar with two marks on it (that were, by definition, spaced apart by one meter), the international prototype of the metre, and in 1983 the meter was redefined to be the distance in free space covered by light in 1/299,792,458 of a second (thus defining the speed of light to be 299,792,458 meters per second).

Natural sciences

In many sciences, from pathology to taxonomy, prototype refers to a disease, species, etc. which sets a good example for the whole category. In biology, prototype is the ancestral or primitive form of a species or other group; an archetype. For example, the Senegal bichir is regarded as the prototypes of its genus, Polypterus.