Smoothness

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Smoothness

A bump function is a smooth function with compact support.

In mathematical analysis, the smoothness of a function is a property measured by the number of continuous derivatives it has over some domain, called differentiability class. At the very minimum, a function could be considered smooth if it is differentiable everywhere (hence continuous). At the other end, it might also possess derivatives of all orders in its domain, in which case it is said to be infinitely differentiable and referred to as a C-infinity function (or ${\displaystyle C^{\mathrm{\infty }}}$ function).

Differentiability classes

Differentiability class is a classification of functions according to the properties of their derivatives. It is a measure of the highest order of derivative that exists and is continuous for a function.

Consider an open set ${\displaystyle U}$ on the real line and a function ${\displaystyle f}$ defined on ${\displaystyle U}$ with real values. Let k be a non-negative integer. The function ${\displaystyle f}$ is said to be of differentiability class ${\displaystyle C^k}$ if the derivatives ${\displaystyle f^{\prime },f^{″},\dots ,f^{(k)}}$ exist and are continuous on ${\displaystyle U}$. If ${\displaystyle f}$ is ${\displaystyle k}$-differentiable on ${\displaystyle U}$, then it is at least in the class ${\displaystyle C^{k-1}}$ since ${\displaystyle f^{\prime },f^{″},\dots ,f^{(k-1)}}$ are continuous on ${\displaystyle U}$. The function ${\displaystyle f}$ is said to be infinitely differentiable, smooth, or of class ${\displaystyle C^{\mathrm{\infty }}}$, if it has derivatives of all orders on ${\displaystyle U}$. (So all these derivatives are continuous functions over ${\displaystyle U}$.) The function ${\displaystyle f}$ is said to be of class ${\displaystyle C^{\omega }}$, or analytic, if ${\displaystyle f}$ is smooth (i.e., ${\displaystyle f}$ is in the class ${\displaystyle C^{\mathrm{\infty }}}$) and its Taylor series expansion around any point in its domain converges to the function in some neighborhood of the point. ${\displaystyle C^{\omega }}$ is thus strictly contained in ${\displaystyle C^{\mathrm{\infty }}}$. Bump functions are examples of functions in ${\displaystyle C^{\mathrm{\infty }}}$ but not in ${\displaystyle C^{\omega }}$.

To put it differently, the class ${\displaystyle C^0}$ consists of all continuous functions. The class ${\displaystyle C^1}$ consists of all differentiable functions whose derivative is continuous; such functions are called continuously differentiable. Thus, a ${\displaystyle C^1}$ function is exactly a function whose derivative exists and is of class ${\displaystyle C^0}$. In general, the classes ${\displaystyle C^k}$ can be defined recursively by declaring ${\displaystyle C^0}$ to be the set of all continuous functions, and declaring ${\displaystyle C^k}$ for any positive integer ${\displaystyle k}$ to be the set of all differentiable functions whose derivative is in ${\displaystyle C^{k-1}}$. In particular, ${\displaystyle C^k}$ is contained in ${\displaystyle C^{k-1}}$ for every ${\displaystyle k>0}$, and there are examples to show that this containment is strict (${\displaystyle C^k⊊C^{k-1}}$). The class ${\displaystyle C^{\mathrm{\infty }}}$ of infinitely differentiable functions, is the intersection of the classes ${\displaystyle C^k}$ as ${\displaystyle k}$ varies over the non-negative integers.

Examples

Example: Continuous (C⁰) But Not Differentiable

The C⁰ function f(x) = x for x ≥ 0 and 0 otherwise.

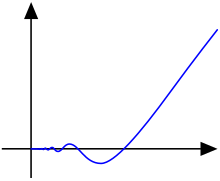
The function g(x) = x² sin(1/x) for x > 0.

The function ${\displaystyle f:\mathbb{R}\to \mathbb{R}}$ with ${\displaystyle f(x)=x^2\sin \left(\frac{1}{x}\right)}$ for ${\displaystyle x\ne 0}$ and ${\displaystyle f(0)=0}$ is differentiable. However, this function is not continuously differentiable.

A smooth function that is not analytic.

The function

$${\displaystyle f(x)=\{\begin{array}{ll}x& \text{if }x\ge 0,\\ 0& \text{if }x<0\end{array}}$$

is continuous, but not differentiable at x = 0, so it is of class C⁰, but not of class C¹.

Example: Finitely-times Differentiable (C^k)

For each even integer k, the function

$${\displaystyle f(x)=|x{|}^{k+1}}$$

is continuous and k times differentiable at all x. At x = 0, however, ${\displaystyle f}$ is not (k + 1) times differentiable, so ${\displaystyle f}$ is of class C^k, but not of class C^j where j > k.

Example: Differentiable But Not Continuously Differentiable (not C¹)

The function

$${\displaystyle g(x)=\{\begin{array}{ll}{x}^2\sin \left(\frac{1}{x}\right)& \text{if }x\ne 0,\\ 0& \text{if }x=0\end{array}}$$

is differentiable, with derivative

$${\displaystyle g^{\prime }(x)=\{\begin{array}{ll}-\cos \left(\frac{1}{x}\right)+2x\sin \left(\frac{1}{x}\right)& \text{if }x\ne 0,\\ 0& \text{if }x=0.\end{array}}$$

Because ${\displaystyle \cos (1/x)}$ oscillates as x → 0, ${\displaystyle g^{\prime }(x)}$ is not continuous at zero. Therefore, ${\displaystyle g(x)}$ is differentiable but not of class C¹.

Example: Differentiable But Not Lipschitz Continuous

The function

$${\displaystyle h(x)=\{\begin{array}{ll}{x}^{4/3}\sin \left(\frac{1}{x}\right)& \text{if }x\ne 0,\\ 0& \text{if }x=0\end{array}}$$

is differentiable but its derivative is unbounded on a compact set. Therefore, ${\displaystyle h}$ is an example of a function that is differentiable but not locally Lipschitz continuous.

Example: Analytic (C^ω)

The exponential function ${\displaystyle e^x}$ is analytic, and hence falls into the class C^ω. The trigonometric functions are also analytic wherever they are defined as they are linear combinations of complex exponential functions ${\displaystyle e^{ix}}$ and ${\displaystyle e^{-ix}}$.

Example: Smooth (C^∞) but not Analytic (C^ω)

The bump function

$${\displaystyle f(x)=\{\begin{array}{ll}{e}^{-\frac{1}{1-x^2}}& \text{ if }|x|<1,\\ 0& \text{ otherwise }\end{array}}$$

is smooth, so of class C^∞, but it is not analytic at x = ±1, and hence is not of class C^ω. The function f is an example of a smooth function with compact support.

Multivariate differentiability classes

A function ${\displaystyle f:U\subset {\mathbb{R}}^n\to \mathbb{R}}$ defined on an open set ${\displaystyle U}$ of ${\displaystyle {\mathbb{R}}^n}$ is said to be of class ${\displaystyle C^k}$ on ${\displaystyle U}$, for a positive integer ${\displaystyle k}$, if all partial derivatives

$${\displaystyle \frac{{\mathrm{\partial }}^{\alpha }f}{\mathrm{\partial }{x}_1^{{\alpha }_1}\mathrm{\partial }{x}_2^{{\alpha }_2}\cdots \mathrm{\partial }{x}_n^{{\alpha }_n}}(y_1,y_2,\dots ,y_n)}$$

exist and are continuous, for every ${\displaystyle {\alpha }_1,{\alpha }_2,\dots ,{\alpha }_n}$ non-negative integers, such that ${\displaystyle \alpha ={\alpha }_1+{\alpha }_2+\cdots +{\alpha }_n\le k}$, and every ${\displaystyle (y_1,y_2,\dots ,y_n)\in U}$. Equivalently, ${\displaystyle f}$ is of class ${\displaystyle C^k}$ on ${\displaystyle U}$ if the ${\displaystyle k}$-th order Fréchet derivative of ${\displaystyle f}$ exists and is continuous at every point of ${\displaystyle U}$. The function ${\displaystyle f}$ is said to be of class ${\displaystyle C}$ or ${\displaystyle C^0}$ if it is continuous on ${\displaystyle U}$. Functions of class ${\displaystyle C^1}$ are also said to be continuously differentiable.

A function ${\displaystyle f:U\subset {\mathbb{R}}^n\to {\mathbb{R}}^m}$, defined on an open set ${\displaystyle U}$ of ${\displaystyle {\mathbb{R}}^n}$, is said to be of class ${\displaystyle C^k}$ on ${\displaystyle U}$, for a positive integer ${\displaystyle k}$, if all of its components

$${\displaystyle f_i(x_1,x_2,\dots ,x_n)=({\pi }_i\circ f)(x_1,x_2,\dots ,x_n)={\pi }_i(f(x_1,x_2,\dots ,x_n))\text{ for }i=1,2,3,\dots ,m}$$

are of class ${\displaystyle C^k}$, where ${\displaystyle {\pi }_i}$ are the natural projections ${\displaystyle {\pi }_i:{\mathbb{R}}^m\to \mathbb{R}}$ defined by ${\displaystyle {\pi }_i(x_1,x_2,\dots ,x_m)=x_i}$. It is said to be of class ${\displaystyle C}$ or ${\displaystyle C^0}$ if it is continuous, or equivalently, if all components ${\displaystyle f_i}$ are continuous, on ${\displaystyle U}$.

The space of C^k functions

Let ${\displaystyle D}$ be an open subset of the real line. The set of all ${\displaystyle C^k}$ real-valued functions defined on ${\displaystyle D}$ is a Fréchet vector space, with the countable family of seminorms

$${\displaystyle p_{K,m}=\underset{x\in K}{sup}\left|f^{(m)}(x)\right|}$$

where ${\displaystyle K}$ varies over an increasing sequence of compact sets whose union is ${\displaystyle D}$, and ${\displaystyle m=0,1,\dots ,k}$.

The set of ${\displaystyle C^{\mathrm{\infty }}}$ functions over ${\displaystyle D}$ also forms a Fréchet space. One uses the same seminorms as above, except that ${\displaystyle m}$ is allowed to range over all non-negative integer values.

The above spaces occur naturally in applications where functions having derivatives of certain orders are necessary; however, particularly in the study of partial differential equations, it can sometimes be more fruitful to work instead with the Sobolev spaces.

Continuity

The terms parametric continuity (C^k) and geometric continuity (Gⁿ) were introduced by Brian Barsky, to show that the smoothness of a curve could be measured by removing restrictions on the speed, with which the parameter traces out the curve.

Parametric continuity

Parametric continuity (C^k) is a concept applied to parametric curves, which describes the smoothness of the parameter's value with distance along the curve. A (parametric) curve ${\displaystyle s:[0,1]\to {\mathbb{R}}^n}$ is said to be of class C^k, if ${\displaystyle \frac{d^{k}s}{d{t}^k}}$ exists and is continuous on ${\displaystyle [0,1]}$, where derivatives at the end-points ${\displaystyle 0,1\in [0,1]}$ are taken to be one sided derivatives (i.e., at ${\displaystyle 0}$ from the right, and at ${\displaystyle 1}$ from the left).

As a practical application of this concept, a curve describing the motion of an object with a parameter of time must have C¹ continuity and its first derivative is differentiable—for the object to have finite acceleration. For smoother motion, such as that of a camera's path while making a film, higher orders of parametric continuity are required.

Order of parametric continuity

Two Bézier curve segments attached that is only C⁰ continuous

Two Bézier curve segments attached in such a way that they are C¹ continuous

The various order of parametric continuity can be described as follows:

• ${\displaystyle C^0}$: zeroth derivative is continuous (curves are continuous)
• ${\displaystyle C^1}$: zeroth and first derivatives are continuous
• ${\displaystyle C^2}$: zeroth, first and second derivatives are continuous
• ${\displaystyle C^n}$: 0-th through ${\displaystyle n}$-th derivatives are continuous

Geometric continuity

Curves with G¹-contact (circles,line)

${\displaystyle (1-{\epsilon }^2)x^2-2px+y^2=0,p>0,\epsilon \ge 0}$
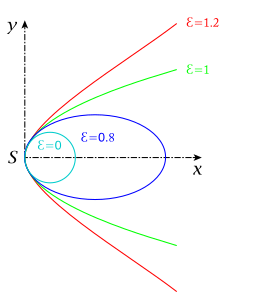
pencil of conic sections with G²-contact: p fix, ${\displaystyle \epsilon }$ variable
(${\displaystyle \epsilon =0}$: circle,${\displaystyle \epsilon =0.8}$: ellipse, ${\displaystyle \epsilon =1}$: parabola, ${\displaystyle \epsilon =1.2}$: hyperbola)

The concept of geometrical continuity or geometric continuity (Gⁿ) was primarily applied to the conic sections (and related shapes) by mathematicians such as Leibniz, Kepler, and Poncelet. The concept was an early attempt at describing, through geometry rather than algebra, the concept of continuity as expressed through a parametric function.

The basic idea behind geometric continuity was that the five conic sections were really five different versions of the same shape. An ellipse tends to a circle as the eccentricity approaches zero, or to a parabola as it approaches one; and a hyperbola tends to a parabola as the eccentricity drops toward one; it can also tend to intersecting lines. Thus, there was continuity between the conic sections. These ideas led to other concepts of continuity. For instance, if a circle and a straight line were two expressions of the same shape, perhaps a line could be thought of as a circle of infinite radius. For such to be the case, one would have to make the line closed by allowing the point ${\displaystyle x=\mathrm{\infty }}$ to be a point on the circle, and for ${\displaystyle x=+\mathrm{\infty }}$ and ${\displaystyle x=\mathrm{\neg }\mathrm{\infty }}$ to be identical. Such ideas were useful in crafting the modern, algebraically defined, idea of the continuity of a function and of ${\displaystyle \mathrm{\infty }}$ (see projectively extended real line for more).

Order of geometric continuity

A curve or surface can be described as having ${\displaystyle G^n}$ continuity, with ${\displaystyle n}$ being the increasing measure of smoothness. Consider the segments either side of a point on a curve:

• ${\displaystyle G^0}$: The curves touch at the join point.
• ${\displaystyle G^1}$: The curves also share a common tangent direction at the join point.
• ${\displaystyle G^2}$: The curves also share a common center of curvature at the join point.

In general, ${\displaystyle G^n}$ continuity exists if the curves can be reparameterized to have ${\displaystyle C^n}$ (parametric) continuity. A reparametrization of the curve is geometrically identical to the original; only the parameter is affected.

Equivalently, two vector functions ${\displaystyle f(t)}$ and ${\displaystyle g(t)}$ have ${\displaystyle G^n}$ continuity if ${\displaystyle f^{(n)}(t)\ne 0}$ and ${\displaystyle f^{(n)}(t)\equiv k{g}^{(n)}(t)}$, for a scalar ${\displaystyle k>0}$ (i.e., if the direction, but not necessarily the magnitude, of the two vectors is equal).

While it may be obvious that a curve would require ${\displaystyle G^1}$ continuity to appear smooth, for good aesthetics, such as those aspired to in architecture and sports car design, higher levels of geometric continuity are required. For example, reflections in a car body will not appear smooth unless the body has ${\displaystyle G^2}$ continuity.

A rounded rectangle (with ninety degree circular arcs at the four corners) has ${\displaystyle G^1}$ continuity, but does not have ${\displaystyle G^2}$ continuity. The same is true for a rounded cube, with octants of a sphere at its corners and quarter-cylinders along its edges. If an editable curve with ${\displaystyle G^2}$ continuity is required, then cubic splines are typically chosen; these curves are frequently used in industrial design.

Other concepts

Relation to analyticity

While all analytic functions are "smooth" (i.e. have all derivatives continuous) on the set on which they are analytic, examples such as bump functions (mentioned above) show that the converse is not true for functions on the reals: there exist smooth real functions that are not analytic. Simple examples of functions that are smooth but not analytic at any point can be made by means of Fourier series; another example is the Fabius function. Although it might seem that such functions are the exception rather than the rule, it turns out that the analytic functions are scattered very thinly among the smooth ones; more rigorously, the analytic functions form a meagre subset of the smooth functions. Furthermore, for every open subset A of the real line, there exist smooth functions that are analytic on A and nowhere else.

It is useful to compare the situation to that of the ubiquity of transcendental numbers on the real line. Both on the real line and the set of smooth functions, the examples we come up with at first thought (algebraic/rational numbers and analytic functions) are far better behaved than the majority of cases: the transcendental numbers and nowhere analytic functions have full measure (their complements are meagre).

The situation thus described is in marked contrast to complex differentiable functions. If a complex function is differentiable just once on an open set, it is both infinitely differentiable and analytic on that set.

Smooth partitions of unity

Smooth functions with given closed support are used in the construction of smooth partitions of unity (see partition of unity and topology glossary); these are essential in the study of smooth manifolds, for example to show that Riemannian metrics can be defined globally starting from their local existence. A simple case is that of a bump function on the real line, that is, a smooth function f that takes the value 0 outside an interval [a,b] and such that

$${\displaystyle f(x)>0\text{ for }a<x<b.}$$

Given a number of overlapping intervals on the line, bump functions can be constructed on each of them, and on semi-infinite intervals ${\displaystyle (-\mathrm{\infty },c]}$ and ${\displaystyle [d,+\mathrm{\infty })}$ to cover the whole line, such that the sum of the functions is always 1.

From what has just been said, partitions of unity don't apply to holomorphic functions; their different behavior relative to existence and analytic continuation is one of the roots of sheaf theory. In contrast, sheaves of smooth functions tend not to carry much topological information.

Smooth functions on and between manifolds

Given a smooth manifold ${\displaystyle M}$, of dimension ${\displaystyle m,}$ and an atlas ${\displaystyle \mathfrak{U}=\{(U_{\alpha },{\varphi }_{\alpha }){\}}_{\alpha },}$ then a map ${\displaystyle f:M\to \mathbb{R}}$ is smooth on ${\displaystyle M}$ if for all ${\displaystyle p\in M}$ there exists a chart ${\displaystyle (U,\varphi )\in \mathfrak{U},}$ such that ${\displaystyle p\in U,}$ and ${\displaystyle f\circ {\varphi }^{-1}:\varphi (U)\to \mathbb{R}}$ is a smooth function from a neighborhood of ${\displaystyle \varphi (p)}$ in ${\displaystyle {\mathbb{R}}^m}$ to ${\displaystyle \mathbb{R}}$ (all partial derivatives up to a given order are continuous). Smoothness can be checked with respect to any chart of the atlas that contains ${\displaystyle p,}$ since the smoothness requirements on the transition functions between charts ensure that if ${\displaystyle f}$ is smooth near ${\displaystyle p}$ in one chart it will be smooth near ${\displaystyle p}$ in any other chart.

If ${\displaystyle F:M\to N}$ is a map from ${\displaystyle M}$ to an ${\displaystyle n}$-dimensional manifold ${\displaystyle N}$, then ${\displaystyle F}$ is smooth if, for every ${\displaystyle p\in M,}$ there is a chart ${\displaystyle (U,\varphi )}$ containing ${\displaystyle p,}$ and a chart ${\displaystyle (V,\psi )}$ containing ${\displaystyle F(p)}$ such that ${\displaystyle F(U)\subset V,}$ and ${\displaystyle \psi \circ F\circ {\varphi }^{-1}:\varphi (U)\to \psi (V)}$ is a smooth function from ${\displaystyle {\mathbb{R}}^n.}$

Smooth maps between manifolds induce linear maps between tangent spaces: for ${\displaystyle F:M\to N}$, at each point the pushforward (or differential) maps tangent vectors at ${\displaystyle p}$ to tangent vectors at ${\displaystyle F(p)}$: ${\displaystyle F_{\ast ,p}:T_{p}M\to T_{F(p)}N,}$ and on the level of the tangent bundle, the pushforward is a vector bundle homomorphism: ${\displaystyle F_{\ast }:TM\to TN.}$ The dual to the pushforward is the pullback, which "pulls" covectors on ${\displaystyle N}$ back to covectors on ${\displaystyle M,}$ and ${\displaystyle k}$-forms to ${\displaystyle k}$-forms: ${\displaystyle F^{\ast }:{\mathrm{\Omega }}^k(N)\to {\mathrm{\Omega }}^k(M).}$ In this way smooth functions between manifolds can transport local data, like vector fields and differential forms, from one manifold to another, or down to Euclidean space where computations like integration are well understood.

Preimages and pushforwards along smooth functions are, in general, not manifolds without additional assumptions. Preimages of regular points (that is, if the differential does not vanish on the preimage) are manifolds; this is the preimage theorem. Similarly, pushforwards along embeddings are manifolds.

Smooth functions between subsets of manifolds

There is a corresponding notion of smooth map for arbitrary subsets of manifolds. If ${\displaystyle f:X\to Y}$ is a function whose domain and range are subsets of manifolds ${\displaystyle X\subseteq M}$ and ${\displaystyle Y\subseteq N}$ respectively. ${\displaystyle f}$ is said to be smooth if for all ${\displaystyle x\in X}$ there is an open set ${\displaystyle U\subseteq M}$ with ${\displaystyle x\in U}$ and a smooth function ${\displaystyle F:U\to N}$ such that ${\displaystyle F(p)=f(p)}$ for all ${\displaystyle p\in U\cap X.}$

Organofluorine chemistry

From Wikipedia, the free encyclopedia

Some important organofluorine compounds. A: fluoromethane
B: isoflurane
C: a CFC
D: an HFC
E: triflic acid
F: Teflon
G: PFOS
H: fluorouracil
I: fluoxetine

Organofluorine chemistry describes the chemistry of organofluorine compounds, organic compounds that contain a carbon–fluorine bond. Organofluorine compounds find diverse applications ranging from oil and water repellents to pharmaceuticals, refrigerants, and reagents in catalysis. In addition to these applications, some organofluorine compounds are pollutants because of their contributions to ozone depletion, global warming, bioaccumulation, and toxicity. The area of organofluorine chemistry often requires special techniques associated with the handling of fluorinating agents.

The carbon–fluorine bond

Main article: Carbon-fluorine bond

Fluorine has several distinctive differences from all other substituents encountered in organic molecules. As a result, the physical and chemical properties of organofluorines can be distinctive in comparison to other organohalogens.

1. The carbon–fluorine bond is one of the strongest in organic chemistry (an average bond energy around 480 kJ/mol). This is significantly stronger than the bonds of carbon with other halogens (an average bond energy of e.g. C-Cl bond is around 320 kJ/mol) and is one of the reasons why fluoroorganic compounds have high thermal and chemical stability.
2. The carbon–fluorine bond is relatively short (around 1.4 Å).
3. The Van der Waals radius of the fluorine substituent is only 1.47 Å, which is shorter than in any other substituent and is close to that of hydrogen (1.2 Å). This, together with the short bond length, is the reason why there is no steric strain in polyfluorinated compounds. This is another reason for their high thermal stability. In addition, the fluorine substituents in polyfluorinated compounds efficiently shield the carbon skeleton from possible attacking reagents. This is another reason for the high chemical stability of polyfluorinated compounds.
4. Fluorine has the highest electronegativity of all elements: 3.98. This causes the high dipole moment of C-F bond (1.41 D).
5. Fluorine has the lowest polarizability of all atoms: 0.56 ${\displaystyle \times }$ 10⁻²⁴ cm³. This causes very weak dispersion forces between polyfluorinated molecules and is the reason for the often-observed boiling point reduction on fluorination as well as for the simultaneous hydrophobicity and lipophobicity of polyfluorinated compounds whereas other perhalogenated compounds are more lipophilic.

In comparison to aryl chlorides and bromides, aryl fluorides form Grignard reagents only reluctantly. On the other hand, aryl fluorides, e.g. fluoroanilines and fluorophenols, often undergo nucleophilic substitution efficiently.

Types of organofluorine compounds

Fluorocarbons

Main article: Perfluorocarbon

Formally, fluorocarbons only contain carbon and fluorine. Sometimes they are called perfluorocarbons. They can be gases, liquids, waxes, or solids, depending upon their molecular weight. The simplest fluorocarbon is the gas tetrafluoromethane (CF₄). Liquids include perfluorooctane and perfluorodecalin. While fluorocarbons with single bonds are stable, unsaturated fluorocarbons are more reactive, especially those with triple bonds. Fluorocarbons are more chemically and thermally stable than hydrocarbons, reflecting the relative inertness of the C-F bond. They are also relatively lipophobic. Because of the reduced intermolecular van der Waals interactions, fluorocarbon-based compounds are sometimes used as lubricants or are highly volatile. Fluorocarbon liquids have medical applications as oxygen carriers.

The structure of organofluorine compounds can be distinctive. As shown below, perfluorinated aliphatic compounds tend to segregate from hydrocarbons. This "like dissolves like effect" is related to the usefulness of fluorous phases and the use of PFOA in processing of fluoropolymers. In contrast to the aliphatic derivatives, perfluoroaromatic derivatives tend to form mixed phases with nonfluorinated aromatic compounds, resulting from donor-acceptor interactions between the pi-systems.

Segregation of alkyl and perfluoroalkyl substituents.

Packing in a crystal pentafluorotolan (C₆F₅CCC₆H₅), illustrating the donor-acceptor interactions between the fluorinated and nonfluorinated rings.

Fluoropolymers

Main article: Fluoropolymer

Polymeric organofluorine compounds are numerous and commercially significant. They range from fully fluorinated species, e.g. PTFE to partially fluorinated, e.g. polyvinylidene fluoride ([CH₂CF₂]_n) and polychlorotrifluoroethylene ([CFClCF₂]_n). The fluoropolymer polytetrafluoroethylene (PTFE/Teflon) is a solid.

Hydrofluorocarbons

Main article: Hydrofluorocarbon

Hydrofluorocarbons (HFCs), organic compounds that contain fluorine and hydrogen atoms, are the most common type of organofluorine compounds. They are commonly used in air conditioning and as refrigerants in place of the older chlorofluorocarbons such as R-12 and hydrochlorofluorocarbons such as R-21. They do not harm the ozone layer as much as the compounds they replace; however, they do contribute to global warming. Their atmospheric concentrations and contribution to anthropogenic greenhouse gas emissions are rapidly increasing, causing international concern about their radiative forcing.

Fluorocarbons with few C-F bonds behave similarly to the parent hydrocarbons, but their reactivity can be altered significantly. For example, both uracil and 5-fluorouracil are colourless, high-melting crystalline solids, but the latter is a potent anti-cancer drug. The use of the C-F bond in pharmaceuticals is predicated on this altered reactivity. Several drugs and agrochemicals contain only one fluorine center or one trifluoromethyl group.

Unlike other greenhouse gases in the Paris Agreement, hydrofluorocarbons have other international negotiations.

In September 2016, the so-called New York Declaration urged a global reduction in the use of HFCs. On 15 October 2016, due to these chemicals contribution to climate change, negotiators from 197 nations meeting at the summit of the United Nations Environment Programme in Kigali, Rwanda reached a legally-binding accord to phase out hydrofluorocarbons (HFCs) in an amendment to the Montreal Protocol.

Fluorocarbenes

As indicated throughout this article, fluorine-substituents lead to reactivity that differs strongly from classical organic chemistry. The premier example is difluorocarbene, CF₂, which is a singlet whereas carbene (CH₂) has a triplet ground state. This difference is significant because difluorocarbene is a precursor to tetrafluoroethylene.

Perfluorinated compounds

Main article: Perfluorinated compounds

Perfluorinated compounds are fluorocarbon derivatives, as they are closely structurally related to fluorocarbons. However, they also possess new atoms such as nitrogen, iodine, or ionic groups, such as perfluorinated carboxylic acids.

Methods for preparation of C–F bonds

Organofluorine compounds are prepared by numerous routes, depending on the degree and regiochemistry of fluorination sought and the nature of the precursors. The direct fluorination of hydrocarbons with F₂, often diluted with N₂, is useful for highly fluorinated compounds:

R
₃CH + F
₂ → R
₃CF + HF

Such reactions however are often unselective and require care because hydrocarbons can uncontrollably "burn" in F
₂, analogous to the combustion of hydrocarbon in O
₂. For this reason, alternative fluorination methodologies have been developed. Generally, such methods are classified into two classes.

Electrophilic fluorination

See also: electrophilic fluorination

Electrophilic fluorination rely on sources of "F⁺". Often such reagents feature N-F bonds, for example F-TEDA-BF₄. Asymmetric fluorination, whereby only one of two possible enantiomeric products are generated from a prochiral substrate, rely on electrophilic fluorination reagents. Illustrative of this approach is the preparation of a precursor to anti-inflammatory agents:

Electrosynthetic methods

Main article: Electrofluorination

A specialized but important method of electrophilic fluorination involves electrosynthesis. The method is mainly used to perfluorinate, i.e. replace all C–H bonds by C–F bonds. The hydrocarbon is dissolved or suspended in liquid HF, and the mixture is electrolyzed at 5–6 V using Ni anodes. The method was first demonstrated with the preparation of perfluoropyridine (C
₅F
₅N) from pyridine (C
₅H
₅N). Several variations of this technique have been described, including the use of molten potassium bifluoride or organic solvents.

Nucleophilic fluorination

The major alternative to electrophilic fluorination is, naturally, nucleophilic fluorination using reagents that are sources of "F⁻," for Nucleophilic displacement typically of chloride and bromide. Metathesis reactions employing alkali metal fluorides are the simplest. For aliphatic compounds this is sometimes called the Finkelstein reaction, while for aromatic compounds it is known as the Halex process.

R
₃CCl + MF → R
₃CF + MCl (M = Na, K, Cs)

Alkyl monofluorides can be obtained from alcohols and Olah reagent (pyridinium fluoride) or another fluoridating agents.

The decomposition of aryldiazonium tetrafluoroborates in the Sandmeyer or Schiemann reactions exploit fluoroborates as F⁻ sources.

ArN
₂BF
₄ → ArF + N
₂ + BF
₃

Although hydrogen fluoride may appear to be an unlikely nucleophile, it is the most common source of fluoride in the synthesis of organofluorine compounds. Such reactions are often catalysed by metal fluorides such as chromium trifluoride. 1,1,1,2-Tetrafluoroethane, a replacement for CFC's, is prepared industrially using this approach:

Cl₂C=CClH + 4 HF → F₃CCFH₂ + 3 HCl

Notice that this transformation entails two reaction types, metathesis (replacement of Cl⁻ by F⁻) and hydrofluorination of an alkene.

Deoxofluorination

Deoxofluorination agents effect the replacement hydroxyl and carbonyl groups with one and two fluorides, respectively. One such reagent, useful for fluoride for oxide exchange in carbonyl compounds, is sulfur tetrafluoride:

RCO₂H + SF₄ → RCF₃ + SO₂ + HF

Alternates to SF₄ include the diethylaminosulfur trifluoride (DAST, NEt₂SF₃) and bis(2-methoxyethyl)aminosulfur trifluoride (Deoxofluor). These organic reagents are easier to handle and more selective:

Apart from DAST and Deoxofluor, a wide variety of similar reagents exist, including, but not limited to, 2-pyridinesulfonyl fluoride (PyFluor) and N-tosyl-4-chlorobenzenesulfonimidoyl fluoride (SulfoxFluor). Many of these display improved properties such as better safety profile, higher thermodynamic stability, ease of handling, high enantioselectivity, and selectivity over elimination side-reactions.

From fluorinated building blocks

Many organofluorine compounds are generated from reagents that deliver perfluoroalkyl and perfluoroaryl groups. (Trifluoromethyl)trimethylsilane, CF₃Si(CH₃)₃, is used as a source of the trifluoromethyl group, for example. Among the available fluorinated building blocks are CF₃X (X = Br, I), C₆F₅Br, and C₃F₇I. These species form Grignard reagents that then can be treated with a variety of electrophiles. The development of fluorous technologies (see below, under solvents) is leading to the development of reagents for the introduction of "fluorous tails".

A special but significant application of the fluorinated building block approach is the synthesis of tetrafluoroethylene, which is produced on a large-scale industrially via the intermediacy of difluorocarbene. The process begins with the thermal (600-800 °C) dehydrochlorination of chlorodifluoromethane:

CHClF₂ → CF₂ + HCl

2 CF₂ → C₂F₄

Sodium fluorodichloroacetate (CAS# 2837-90-3) is used to generate chlorofluorocarbene, for cyclopropanations.

¹⁸F-Delivery methods

The usefulness of fluorine-containing radiopharmaceuticals in ¹⁸F-positron emission tomography has motivated the development of new methods for forming C–F bonds. Because of the short half-life of ¹⁸F, these syntheses must be highly efficient, rapid, and easy. Illustrative of the methods is the preparation of fluoride-modified glucose by displacement of a triflate by a labeled fluoride nucleophile:

Biological role

Biologically synthesized organofluorines have been found in microorganisms and plants, but not animals. The most common example is fluoroacetate, which occurs as a plant defence against herbivores in at least 40 plants in Australia, Brazil and Africa. Other biologically synthesized organofluorines include ω-fluoro fatty acids, fluoroacetone, and 2-fluorocitrate which are all believed to be biosynthesized in biochemical pathways from the intermediate fluoroacetaldehyde. Adenosyl-fluoride synthase is an enzyme capable of biologically synthesizing the carbon–fluorine bond. Man made carbon–fluorine bonds are commonly found in pharmaceuticals and agrichemicals because it adds stability to the carbon framework; also, the relatively small size of fluorine is convenient as fluorine acts as an approximate bioisostere of the hydrogen. Introducing the carbon–fluorine bond to organic compounds is the major challenge for medicinal chemists using organofluorine chemistry, as the carbon–fluorine bond increases the probability of having a successful drug by about a factor of ten. An estimated 20% of pharmaceuticals, and 30–40% of agrichemicals are organofluorines, including several of the top drugs. Examples include 5-fluorouracil, fluoxetine (Prozac), paroxetine (Paxil), ciprofloxacin (Cipro), mefloquine, and fluconazole.

Applications

Organofluorine chemistry impacts many areas of everyday life and technology. The C-F bond is found in pharmaceuticals, agrichemicals, fluoropolymers, refrigerants, surfactants, anesthetics, oil-repellents, catalysis, and water-repellents, among others.

Pharmaceuticals and agrochemicals

The carbon-fluorine bond is commonly found in pharmaceuticals and agrochemicals because it is generally metabolically stable and fluorine acts as a bioisostere of the hydrogen atom. An estimated 1/5 of pharmaceuticals contain fluorine, including several of the top drugs. Examples include 5-fluorouracil, flunitrazepam (Rohypnol), fluoxetine (Prozac), paroxetine (Paxil), ciprofloxacin (Cipro), mefloquine, and fluconazole. Fluorine-substituted ethers are volatile anesthetics, including the commercial products methoxyflurane, enflurane, isoflurane, sevoflurane and desflurane. Fluorocarbon anesthetics reduce the hazard of flammability with diethyl ether and cyclopropane. Perfluorinated alkanes are used as blood substitutes.

Inhaler propellant

Fluorocarbons are also used as a propellant for metered-dose inhalers used to administer some asthma medications. The current generation of propellant consists of hydrofluoroalkanes (HFA), which have replaced CFC-propellant-based inhalers. CFC inhalers were banned as of 2008 as part of the Montreal Protocol because of environmental concerns with the ozone layer. HFA propellant inhalers like FloVent and ProAir ( Salbutamol ) have no generic versions available as of October 2014.

Fluorosurfactants

Fluorosurfactants, which have a polyfluorinated "tail" and a hydrophilic "head", serve as surfactants because they concentrate at the liquid-air interface due to their lipophobicity. Fluorosurfactants have low surface energies and dramatically lower surface tension. The fluorosurfactants perfluorooctanesulfonic acid (PFOS) and perfluorooctanoic acid (PFOA) are two of the most studied because of their ubiquity, toxicity, and long residence times in humans and wildlife.

Solvents

Fluorinated compounds often display distinct solubility properties. Dichlorodifluoromethane and chlorodifluoromethane were at one time widely used refrigerants. CFCs have potent ozone depletion potential due to the homolytic cleavage of the carbon-chlorine bonds; their use is largely prohibited by the Montreal Protocol. Hydrofluorocarbons (HFCs), such as tetrafluoroethane, serve as CFC replacements because they do not catalyze ozone depletion. Oxygen exhibits a high solubility in perfluorocarbon compounds, reflecting on their lipophilicity. Perfluorodecalin has been demonstrated as a blood substitute transporting oxygen to the lungs.

The solvent 1,1,1,2-tetrafluoroethane has been used for extraction of natural products such as taxol, evening primrose oil, and vanillin. 2,2,2-trifluoroethanol is an oxidation-resistant polar solvent.

Organofluorine reagents

The development of organofluorine chemistry has contributed many reagents of value beyond organofluorine chemistry. Triflic acid (CF₃SO₃H) and trifluoroacetic acid (CF₃CO₂H) are useful throughout organic synthesis. Their strong acidity is attributed to the electronegativity of the trifluoromethyl group that stabilizes the negative charge. The triflate-group (the conjugate base of the triflic acid) is a good leaving group in substitution reactions.

Fluorous phases

Of topical interest in the area of "Green Chemistry," highly fluorinated substituents, e.g. perfluorohexyl (C₆F₁₃) confer distinctive solubility properties to molecules, which facilitates purification of products in organic synthesis. This area, described as "fluorous chemistry," exploits the concept of like-dissolves-like in the sense that fluorine-rich compounds dissolve preferentially in fluorine-rich solvents. Because of the relative inertness of the C-F bond, such fluorous phases are compatible with even harsh reagents. This theme has spawned techniques of "fluorous tagging and fluorous protection. Illustrative of fluorous technology is the use of fluoroalkyl-substituted tin hydrides for reductions, the products being easily separated from the spent tin reagent by extraction using fluorinated solvents.

Hydrophobic fluorinated ionic liquids, such as organic salts of bistriflimide or hexafluorophosphate, can form phases that are insoluble in both water and organic solvents, producing multiphasic liquids.

Organofluorine ligands in transition metal chemistry

Organofluorine ligands have long been featured in organometallic and coordination chemistry. One advantage to F-containing ligands is the convenience of ¹⁹F NMR spectroscopy for monitoring reactions. The organofluorine compounds can serve as a "sigma-donor ligand," as illustrated by the titanium(III) derivative [(C₅Me₅)₂Ti(FC₆H₅)]BPh₄. Most often, however, fluorocarbon substituents are used to enhance the Lewis acidity of metal centers. A premier example is "Eufod," a coordination complex of europium(III) that features a perfluoroheptyl modified acetylacetonate ligand. This and related species are useful in organic synthesis and as "shift reagents" in NMR spectroscopy.

Structure of [(C₅Me₅)₂Ti(FC₆H₅)]⁺, a coordination complex of an organofluorine ligand.

In an area where coordination chemistry and materials science overlap, the fluorination of organic ligands is used to tune the properties of component molecules. For example, the degree and regiochemistry of fluorination of metalated 2-phenylpyridine ligands in platinum(II) complexes significantly modifies the emission properties of the complexes.

The coordination chemistry of organofluorine ligands also embraces fluorous technologies. For example, triphenylphosphine has been modified by attachment of perfluoroalkyl substituents that confer solubility in perfluorohexane as well as supercritical carbon dioxide. As a specific example, [(C₈F₁₇C₃H₆-4-C₆H₄)₃P.

C-F bond activation

An active area of organometallic chemistry encompasses the scission of C-F bonds by transition metal-based reagents. Both stoichiometric and catalytic reactions have been developed and are of interest from the perspectives of organic synthesis and remediation of xenochemicals. C-F bond activation has been classified as follows "(i) oxidative addition of fluorocarbon, (ii) M–C bond formation with HF elimination, (iii) M–C bond formation with fluorosilane elimination, (iv) hydrodefluorination of fluorocarbon with M–F bond formation, (v) nucleophilic attack on fluorocarbon, and (vi) defluorination of fluorocarbon". An illustrative metal-mediated C-F activation reaction is the defluorination of fluorohexane by a zirconium dihydride, an analogue of Schwartz's reagent:

(C₅Me₅)₂ZrH₂ + 1-FC₆H₁₃ → (C₅Me₅)₂ZrH(F) + C₆H₁₄

Fluorocarbon anions in Ziegler-Natta catalysis

Fluorine-containing compounds are often featured in noncoordinating or weakly coordinating anions. Both tetrakis(pentafluorophenyl)borate, B(C₆F₅)₄⁻, and the related tetrakis[3,5-bis(trifluoromethyl)phenyl]borate, are useful in Ziegler-Natta catalysis and related alkene polymerization methodologies. The fluorinated substituents render the anions weakly basic and enhance the solubility in weakly basic solvents, which are compatible with strong Lewis acids.

Materials science

Organofluorine compounds enjoy many niche applications in materials science. With a low coefficient of friction, fluid fluoropolymers are used as specialty lubricants. Fluorocarbon-based greases are used in demanding applications. Representative products include Fomblin and Krytox, made by Solvay Solexis and DuPont, respectively. Certain firearm lubricants such as "Tetra Gun" contain fluorocarbons. Capitalizing on their nonflammability, fluorocarbons are used in fire fighting foam. Organofluorine compounds are components of liquid crystal displays. The polymeric analogue of triflic acid, nafion is a solid acid that is used as the membrane in most low temperature fuel cells. The bifunctional monomer 4,4'-difluorobenzophenone is a precursor to PEEK-class polymers.

Biosynthesis of organofluorine compounds

In contrast to the many naturally-occurring organic compounds containing the heavier halides, chloride, bromide, and iodide, only a handful of biologically synthesized carbon-fluorine bonds are known. The most common natural organofluorine species is fluoroacetate, a toxin found in a few species of plants. Others include fluorooleic acid, fluoroacetone, nucleocidin (4'-fluoro-5'-O-sulfamoyladenosine), fluorothreonine, and 2-fluorocitrate. Several of these species are probably biosynthesized from fluoroacetaldehyde. The enzyme fluorinase catalyzed the synthesis of 5'-deoxy-5'-fluoroadenosine (see scheme to right).

History

Organofluorine chemistry began in the 1800s with the development of organic chemistry. The first organofluorine compounds were prepared using antimony trifluoride as the F⁻ source. The nonflammability and nontoxicity of the chlorofluorocarbons CCl₃F and CCl₂F₂ attracted industrial attention in the 1920s. On April 6, 1938, Roy J. Plunkett a young research chemist who worked at DuPont's Jackson Laboratory in Deepwater, New Jersey, accidentally discovered polytetrafluoroethylene (PTFE). Subsequent major developments, especially in the US, benefited from expertise gained in the production of uranium hexafluoride. Starting in the late 1940s, a series of electrophilic fluorinating methodologies were introduced, beginning with CoF₃. Electrochemical fluorination ("electrofluorination") was announced, which Joseph H. Simons had developed in the 1930s to generate highly stable perfluorinated materials compatible with uranium hexafluoride. These new methodologies allowed the synthesis of C-F bonds without using elemental fluorine and without relying on metathetical methods.

In 1957, the anticancer activity of 5-fluorouracil was described. This report provided one of the first examples of rational design of drugs. This discovery sparked a surge of interest in fluorinated pharmaceuticals and agrichemicals. The discovery of the noble gas compounds, e.g. XeF₄, provided a host of new reagents starting in the early 1960s. In the 1970s, fluorodeoxyglucose was established as a useful reagent in ¹⁸F positron emission tomography. In Nobel Prize-winning work, CFC's were shown to contribute to the depletion of atmospheric ozone. This discovery alerted the world to the negative consequences of organofluorine compounds and motivated the development of new routes to organofluorine compounds. In 2002, the first C-F bond-forming enzyme, fluorinase, was reported.

Environmental and health concerns

Only a few organofluorine compounds are acutely bioactive and highly toxic, such as fluoroacetate and perfluoroisobutene.

Some organofluorine compounds pose significant risks and dangers to health and the environment. CFCs and HCFCs (hydrochlorofluorocarbon) deplete the ozone layer and are potent greenhouse gases. HFCs are potent greenhouse gases and are facing calls for stricter international regulation and phase out schedules as a fast-acting greenhouse emission abatement measure, as are perfluorocarbons (PFCs), and sulfur hexafluoride (SF₆).

Because of the compound's effect on climate, the G-20 major economies agreed in 2013 to support initiatives to phase out use of HCFCs. They affirmed the roles of the Montreal Protocol and the United Nations Framework Convention on Climate Change in global HCFC accounting and reduction. The U.S. and China at the same time announced a bilateral agreement to similar effect.

Persistence and bioaccumulation

Because of the strength of the carbon–fluorine bond, many synthetic fluorocarbons and fluorocarbon-based compounds are persistent in the environment. Fluorosurfactants, such as PFOS and PFOA, are persistent global contaminants. Fluorocarbon based CFCs and tetrafluoromethane have been reported in igneous and metamorphic rock. PFOS is a persistent organic pollutant and may be harming the health of wildlife; the potential health effects of PFOA to humans are under investigation by the C8 Science Panel.