Harmonic

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Harmonic

The nodes of a vibrating string are harmonics.

Two different notations of natural harmonics on the cello. First as sounded (more common), then as fingered (easier to sightread).

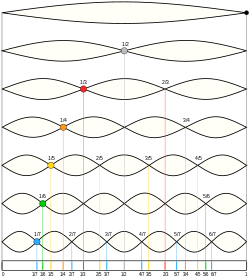
In physics, acoustics, and telecommunications, a harmonic is a sinusoidal wave with a frequency that is a positive integer multiple of the fundamental frequency of a periodic signal. The fundamental frequency is also called the 1st harmonic; the other harmonics are known as higher harmonics. As all harmonics are periodic at the fundamental frequency, the sum of harmonics is also periodic at that frequency. The set of harmonics forms a harmonic series.

The term is employed in various disciplines, including music, physics, acoustics, electronic power transmission, radio technology, and other fields. For example, if the fundamental frequency is 50 Hz, a common AC power supply frequency, the frequencies of the first three higher harmonics are 100 Hz (2nd harmonic), 150 Hz (3rd harmonic), 200 Hz (4th harmonic) and any addition of waves with these frequencies is periodic at 50 Hz.

An ${\displaystyle n}$^th characteristic mode, for ${\displaystyle n>1,}$ will have nodes that are not vibrating. For example, the 3^rd characteristic mode will have nodes at ${\displaystyle \frac{1}{3}L}$ and ${\displaystyle \frac{2}{3}L,}$ where ${\displaystyle L}$ is the length of the string. In fact, each ${\displaystyle n}$^th characteristic mode, for ${\displaystyle n}$ not a multiple of 3, will not have nodes at these points. These other characteristic modes will be vibrating at the positions ${\displaystyle \frac{1}{3}L}$ and ${\displaystyle \frac{2}{3}L.}$ If the player gently touches one of these positions, then these other characteristic modes will be suppressed. The tonal harmonics from these other characteristic modes will then also be suppressed. Consequently, the tonal harmonics from the ${\displaystyle n}$^th characteristic characteristic modes, where ${\displaystyle n}$ is a multiple of 3, will be made relatively more prominent.

In music, harmonics are used on string instruments and wind instruments as a way of producing sound on the instrument, particularly to play higher notes and, with strings, obtain notes that have a unique sound quality or "tone colour". On strings, bowed harmonics have a "glassy", pure tone. On stringed instruments, harmonics are played by touching (but not fully pressing down the string) at an exact point on the string while sounding the string (plucking, bowing, etc.); this allows the harmonic to sound, a pitch which is always higher than the fundamental frequency of the string.

Terminology

Harmonics may be called "overtones", "partials", or "upper partials", and in some music contexts, the terms "harmonic", "overtone" and "partial" are used fairly interchangeably. But more precisely, the term "harmonic" includes all pitches in a harmonic series (including the fundamental frequency) while the term "overtone" only includes pitches above the fundamental.

Characteristics

A whizzing, whistling tonal character, distinguishes all the harmonics both natural and artificial from the firmly stopped intervals; therefore their application in connection with the latter must always be carefully considered.

— Richard Scholz (c. 1888–1912)

Most acoustic instruments emit complex tones containing many individual partials (component simple tones or sinusoidal waves), but the untrained human ear typically does not perceive those partials as separate phenomena. Rather, a musical note is perceived as one sound, the quality or timbre of that sound being a result of the relative strengths of the individual partials. Many acoustic oscillators, such as the human voice or a bowed violin string, produce complex tones that are more or less periodic, and thus are composed of partials that are nearly matched to the integer multiples of fundamental frequency and therefore resemble the ideal harmonics and are called "harmonic partials" or simply "harmonics" for convenience (although it's not strictly accurate to call a  partial  a  harmonic,  the first being actual and the second being theoretical).

Oscillators that produce harmonic partials behave somewhat like one-dimensional resonators, and are often long and thin, such as a guitar string or a column of air open at both ends (as with the metallic modern orchestral transverse flute). Wind instruments whose air column is open at only one end, such as trumpets and clarinets, also produce partials resembling harmonics. However they only produce partials matching the odd harmonics—at least in theory. In practical use, no real acoustic instrument behaves as perfectly as the simplified physical models predict; for example, instruments made of non-linearly elastic wood, instead of metal, or strung with gut instead of brass or steel strings, tend to have not-quite-integer partials.

Partials whose frequencies are not integer multiples of the fundamental are referred to as inharmonic partials. Some acoustic instruments emit a mix of harmonic and inharmonic partials but still produce an effect on the ear of having a definite fundamental pitch, such as pianos, strings plucked pizzicato, vibraphones, marimbas, and certain pure-sounding bells or chimes. Antique singing bowls are known for producing multiple harmonic partials or multiphonics.  Other oscillators, such as cymbals, drum heads, and most percussion instruments, naturally produce an abundance of inharmonic partials and do not imply any particular pitch, and therefore cannot be used melodically or harmonically in the same way other instruments can.

Building on of Sethares (2004), dynamic tonality introduces the notion of pseudo-harmonic partials, in which the frequency of each partial is aligned to match the pitch of a corresponding note in a pseudo-just tuning, thereby maximizing the consonance of that pseudo-harmonic timbre with notes of that pseudo-just tuning.

Partials, overtones, and harmonics

An overtone is any partial higher than the lowest partial in a compound tone. The relative strengths and frequency relationships of the component partials determine the timbre of an instrument. The similarity between the terms overtone and partial sometimes leads to their being loosely used interchangeably in a musical context, but they are counted differently, leading to some possible confusion. In the special case of instrumental timbres whose component partials closely match a harmonic series (such as with most strings and winds) rather than being inharmonic partials (such as with most pitched percussion instruments), it is also convenient to call the component partials "harmonics", but not strictly correct, because harmonics are numbered the same even when missing, while partials and overtones are only counted when present. This chart demonstrates how the three types of names (partial, overtone, and harmonic) are counted (assuming that the harmonics are present):

Frequency	Order (n)	Name 1	Name 2	Name 3	Standing wave representation	Longitudinal wave representation
1 × f = 440 Hz	n = 1	1st partial	fundamental tone	1st harmonic
2 × f = 880 Hz	n = 2	2nd partial	1st overtone	2nd harmonic
3 × f = 1320 Hz	n = 3	3rd partial	2nd overtone	3rd harmonic
4 × f = 1760 Hz	n = 4	4th partial	3rd overtone	4th harmonic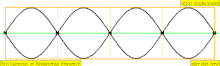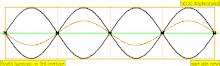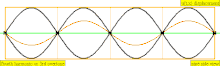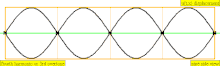

In many musical instruments, it is possible to play the upper harmonics without the fundamental note being present. In a simple case (e.g., recorder) this has the effect of making the note go up in pitch by an octave, but in more complex cases many other pitch variations are obtained. In some cases it also changes the timbre of the note. This is part of the normal method of obtaining higher notes in wind instruments, where it is called overblowing. The extended technique of playing multiphonics also produces harmonics. On string instruments it is possible to produce very pure sounding notes, called harmonics or flageolets by string players, which have an eerie quality, as well as being high in pitch. Harmonics may be used to check at a unison the tuning of strings that are not tuned to the unison. For example, lightly fingering the node found halfway down the highest string of a cello produces the same pitch as lightly fingering the node ⁠ 1 / 3 ⁠ of the way down the second highest string. For the human voice see Overtone singing, which uses harmonics.

While it is true that electronically produced periodic tones (e.g. square waves or other non-sinusoidal waves) have "harmonics" that are whole number multiples of the fundamental frequency, practical instruments do not all have this characteristic. For example, higher "harmonics" of piano notes are not true harmonics but are "overtones" and can be very sharp, i.e. a higher frequency than given by a pure harmonic series. This is especially true of instruments other than strings, brass, or woodwinds. Examples of these "other" instruments are xylophones, drums, bells, chimes, etc.; not all of their overtone frequencies make a simple whole number ratio with the fundamental frequency. (The fundamental frequency is the reciprocal of the longest time period of the collection of vibrations in some single periodic phenomenon.)

On stringed instruments

Playing a harmonic on a string

Main article: String harmonic

Harmonics may be singly produced [on stringed instruments] (1) by varying the point of contact with the bow, or (2) by slightly pressing the string at the nodes, or divisions of its aliquot parts (${\displaystyle \frac{1}{2}}$, ${\displaystyle \frac{1}{3}}$, ${\displaystyle \frac{1}{4}}$, etc.). (1) In the first case, advancing the bow from the usual place where the fundamental note is produced, towards the bridge, the whole scale of harmonics may be produced in succession, on an old and highly resonant instrument. The employment of this means produces the effect called 'sul ponticello.' (2) The production of harmonics by the slight pressure of the finger on the open string is more useful. When produced by pressing slightly on the various nodes of the open strings they are called 'natural harmonics'. ... Violinists are well aware that the longer the string in proportion to its thickness, the greater the number of upper harmonics it can be made to yield.

— Grove's Dictionary of Music and Musicians (1879)

The following table displays the stop points on a stringed instrument at which gentle touching of a string will force it into a harmonic mode when vibrated. String harmonics (flageolet tones) are described as having a "flutelike, silvery quality" that can be highly effective as a special color or tone color (timbre) when used and heard in orchestration. It is unusual to encounter natural harmonics higher than the fifth partial on any stringed instrument except the double bass, on account of its much longer strings.

Harmonic order	Stop note	Note sounded (relative to open string)	Audio frequency (Hz)	Cents above fundamental (offset by octave)	Audio (octave shifted)
1st	fundamental, perfect unison	P 1	600Hz	0.0 ¢	Playⓘ
2nd	first perfect octave	P 8	1200Hz	0.0 ¢	Playⓘ
3rd	perfect fifth	P 8 + P 5	1800Hz	702.0 ¢	Playⓘ
4th	doubled perfect octave	2 · P 8	2400Hz	0.0 ¢	Playⓘ
5th	just major third, major third	2 · P 8 + M 3	3000Hz	386.3 ¢	Playⓘ
6th	perfect fifth	2 · P 8 + P 5	3600Hz	702.0 ¢	Playⓘ
7th	harmonic seventh, septimal minor seventh (‘the lost chord’)	2 · P 8 + m 7↓	4200Hz	968.8 ¢	Playⓘ
8th	third perfect octave	3 · P 8	4800Hz	0.0 ¢	Playⓘ
9th	Pythagorean major second harmonic ninth	3 · P 8 + M 2	5400Hz	203.9 ¢	Playⓘ
10th	just major third	3 · P 8 + M 3	6000Hz	386.3 ¢	Playⓘ
11th	lesser undecimal tritone, undecimal semi-augmented fourth	3 · P 8 + A 4	6600Hz	551.3 ¢	Playⓘ
12th	perfect fifth	3 · P 8 + P 5	7200Hz	702.0 ¢	Playⓘ
13th	tridecimal neutral sixth	3 · P 8 + n 6↓	7800Hz	840.5 ¢	Playⓘ
14th	harmonic seventh, septimal minor seventh (‘the lost chord’)	3 · P 8 + m 7⤈	8400Hz	968.8 ¢	Playⓘ
15th	just major seventh	3 · P 8 + M 7	9000Hz	1088.3 ¢	Playⓘ
16th	fourth perfect octave	4 · P 8	9600Hz	0.0 ¢	Playⓘ
17th	septidecimal semitone	4 · P 8 + m 2⇟	10200Hz	105.0 ¢	Playⓘ
18th	Pythagorean major second	4 · P 8 + M 2	10800Hz	203.9 ¢	Playⓘ
19th	nanodecimal minor third	4 · P 8 + m 3	11400Hz	297.5 ¢	Playⓘ
20th	just major third	4 · P 8 + M 3	12000Hz	386.3 ¢	Playⓘ

Notation key

P	perfect interval
A	augmented interval (sharpened)
M	major interval
m	minor interval (flattened major)
n	neutral interval (between major and minor)
	half-flattened (approximate) (≈ −38 ¢ for just, −50 ¢ for 12 TET)
↓	flattened by a syntonic comma (approximate) (≈ −21 ¢ )
⤈	flattened by a half-comma (approximate) (≈ −10 ¢ )
⇟	flattened by a quarter-comma (approximate) (≈ −5 ¢ )

Artificial harmonics

Occasionally a score will call for an artificial harmonic, produced by playing an overtone on an already stopped string. As a performance technique, it is accomplished by using two fingers on the fingerboard, the first to shorten the string to the desired fundamental, with the second touching the node corresponding to the appropriate harmonic.

Other information

Harmonics may be either used in or considered as the basis of just intonation systems. Composer Arnold Dreyblatt is able to bring out different harmonics on the single string of his modified double bass by slightly altering his unique bowing technique halfway between hitting and bowing the strings. Composer Lawrence Ball uses harmonics to generate music electronically.

Change detection

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Change_detection

Yearly volume of the Nile river at Aswan, an example of time series data commonly used in change detection. Dotted line denotes a detected change point when Old Aswan Dam was built in 1902.

In statistical analysis, change detection or change point detection tries to identify times when the probability distribution of a stochastic process or time series changes. In general the problem concerns both detecting whether or not a change has occurred, or whether several changes might have occurred, and identifying the times of any such changes.

Specific applications, like step detection and edge detection, may be concerned with changes in the mean, variance, correlation, or spectral density of the process. More generally change detection also includes the detection of anomalous behavior: anomaly detection.

In offline change point detection it is assumed that a sequence of length ${\displaystyle T}$ is available and the goal is to identify whether any change point(s) occurred in the series. This is an example of post hoc analysis and is often approached using hypothesis testing methods. By contrast, online change point detection is concerned with detecting change points in an incoming data stream.

Background

A time series measures the progression of one or more quantities over time. For instance, the figure above shows the level of water in the Nile river between 1870 and 1970. Change point detection is concerned with identifying whether, and if so when, the behavior of the series changes significantly. In the Nile river example, the volume of water changes significantly after a dam was built in the river. Importantly, anomalous observations that differ from the ongoing behavior of the time series are not generally considered change points as long as the series returns to its previous behavior afterwards.

Mathematically, we can describe a time series as an ordered sequence of observations ${\displaystyle (x_1,x_2,\dots )}$. We can write the joint distribution of a subset ${\displaystyle x_{a:b}=(x_a,x_{a+1},\dots ,x_b)}$ of the time series as ${\displaystyle p(x_{a:b})}$. If the goal is to determine whether a change point occurred at a time ${\displaystyle \tau }$ in a finite time series of length ${\displaystyle T}$, then we really ask whether ${\displaystyle p(x_{1:\tau })}$ equals ${\displaystyle p(x_{\tau +1:T})}$. This problem can be generalized to the case of more than one change point.

Algorithms

Online change detection

Using the sequential analysis ("online") approach, any change test must make a trade-off between these common metrics:

• False alarm rate
• Misdetection rate
• Detection delay

In a Bayes change-detection problem, a prior distribution is available for the change time.

Online change detection is also done using streaming algorithms.

Offline change detection

Basseville (1993, Section 2.6) discusses offline change-in-mean detection with hypothesis testing based on the works of Page and Picard and maximum-likelihood estimation of the change time, related to two-phase regression. Other approaches employ clustering based on maximum likelihood estimation,, use optimization to infer the number and times of changes, via spectral analysis, or singular spectrum analysis.

Detection of changepoints in the Nile River flow data using a Bayesian method 

Statistically speaking, change detection is often considered as a model selection problem. Models with more changepoints fit data better but with more parameters. The best trade-off can be found by optimizing a model selection criterion such as Akaike information criterion and Bayesian information criterion. Bayesian model selection has also been used. Bayesian methods often quantify uncertainties of all sorts and answer questions hard to tackle by classical methods, such as what is the probability of having a change at a given time and what is the probability of the data having a certain number of changepoints.

"Offline" approaches cannot be used on streaming data because they need to compare to statistics of the complete time series, and cannot react to changes in real-time but often provide a more accurate estimation of the change time and magnitude.

Applications

Change detection tests are often used in manufacturing for quality control, intrusion detection, spam filtering, website tracking, and medical diagnostics.

Linguistic change detection

Linguistic change detection refers to the ability to detect word-level changes across multiple presentations of the same sentence. Researchers have found that the amount of semantic overlap (i.e., relatedness) between the changed word and the new word influences the ease with which such a detection is made (Sturt, Sanford, Stewart, & Dawydiak, 2004). Additional research has found that focussing one's attention to the word that will be changed during the initial reading of the original sentence can improve detection. This was shown using italicized text to focus attention, whereby the word that will be changing is italicized in the original sentence (Sanford, Sanford, Molle, & Emmott, 2006), as well as using clefting constructions such as "It was the tree that needed water." (Kennette, Wurm, & Van Havermaet, 2010). These change-detection phenomena appear to be robust, even occurring cross-linguistically when bilinguals read the original sentence in their native language and the changed sentence in their second language (Kennette, Wurm & Van Havermaet, 2010). Recently, researchers have detected word-level changes in semantics across time by computationally analyzing temporal corpora (for example: the word "gay" has acquired a new meaning over time) using change point detection. This is also applicable to reading non-words such as music. Even though music is not a language, it is still written and people to comprehend its meaning which involves perception and attention, allowing change detection to be present.

Visual change detection

Visual change detection is one's ability to detect differences between two or more images or scenes. This is essential in many everyday tasks. One example is detecting changes on the road to drive safely and successfully. Change detection is crucial in operating motor vehicles to detect other vehicles, traffic control signals, pedestrians, and more. Another example of utilizing visual change detection is facial recognition. When noticing one's appearance, change detection is vital, as faces are "dynamic" and can change in appearance due to different factors such as "lighting conditions, facial expressions, aging, and occlusion". Change detection algorithms use various techniques, such as "feature tracking, alignment, and normalization," to capture and compare different facial features and patterns across individuals in order to correctly identify people. Visual change detection involves the integration of "multiple sensors inputs, cognitive processes, and attentional mechanisms," often focusing on multiple stimuli at once. The brain processes visual information from the eyes, compares it with previous knowledge stored in memory, and identifies differences between the two stimuli. This process occurs rapidly and unconsciously, allowing individuals to respond to changing environments and make necessary adjustments to their behavior.

Cognitive change detection

There have been several studies conducted to analyze the cognitive functions of change detection. With cognitive change detection, researchers have found that most people overestimate their change detection, when in reality, they are more susceptible to change blindness than they think. Cognitive change detection has many complexities based on external factors, and sensory pathways play a key role in determining one's success in detecting changes. One study proposes and proves that the multi-sensory pathway network, which consists of three sensory pathways, significantly increases the effectiveness of change detection. Sensory pathway one fuses the stimuli together, sensory pathway two involves using the middle concatenation strategy to learn the changed behavior, and sensory pathway three involves using the middle difference strategy to learn the changed behavior. With all three of these working together, change detection has a significantly increased success rate. It was previously believed that the posterior parietal cortex (PPC) played a role in enhancing change detection due to its focus on "sensory and task-related activity". However, studies have also disproven that the PPC is necessary for change detection; although these have high functional correlation with each other, the PPC's mechanistic involvement in change detection is insignificant. Moreover, top-down processing plays an important role in change detection because it enables people to resort to background knowledge which then influences perception, which is also common in children. Researchers have conducted a longitudinal study surrounding children's development and the change detection throughout infancy to adulthood. In this, it was found that change detection is stronger in young infants compared to older children, with top-down processing being a main contributor to this outcome.

Apologetics

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Apologetics

Apologetics (from Greek ἀπολογία, apología, 'speaking in defense') is the religious discipline of defending religious doctrines through systematic argumentation and discourse. Early Christian writers (c. 120–220) who defended their beliefs against critics and recommended their faith to outsiders were called Christian apologists. In 21st-century usage, apologetics is often identified with debates over religion and theology.

Etymology

The term apologetics derives from the Ancient Greek word apologia (ἀπολογία). In the Classical Greek legal system, the prosecution delivered the kategoria (κατηγορία), the accusation or charge, and the defendant replied with an apologia, the defence. The apologia was a formal speech or explanation to reply to and rebut the charges. A famous example is Socrates' Apologia defense, as chronicled in Plato's Apology.

In the Koine Greek of the New Testament, the Apostle Paul employs the term apologia in his trial speech to Festus and Agrippa when he says "I make my defense" in Acts 26:2. A cognate form appears in Paul's Letter to the Philippians as he is "defending the gospel" in Philippians 1:7, and in "giving an answer" in 1 Peter 3:15.

Although the term apologetics has Western, primarily Christian origins and is most frequently associated with the defense of Christianity, the term is sometimes used referring to the defense of any religion in formal debate involving religion.

Apologetic positions

Baháʼí Faith

Main article: Baháʼí apologetics

Many apologetic books have been written in defence of the history or teachings of the Baháʼí Faith. The religion's founders wrote several books presenting proofs of their religion; among them are the Báb's Seven Proofs and Bahá'u'lláh's Kitáb-i-Íqán. Later Baháʼí authors wrote prominent apologetic texts, such as Mírzá Abu'l-Fadl's The Brilliant Proof and Udo Schaefer et al.'s Making the Crooked Straight.

Buddhism

One of the earliest Buddhist apologetic texts is The Questions of King Milinda, which deals with the Buddhist metaphysics such as the "no-self" nature of the individual and characteristics such as wisdom, perception, volition, feeling, consciousness and the soul. In the Meiji Era (1868-1912), encounters between Buddhists and Christians in Japan as a result of increasing contact between Japan and other nations may have prompted the formation of Japanese New Buddhism, including the apologetic Shin Bukkyō (新仏教) magazine. In recent times, A. L. De Silva, an Australian convert to Buddhism, has written a book, Beyond Belief, providing Buddhist apologetic responses and a critique of Christian Fundamentalist doctrine. Gunapala Dharmasiri wrote an apologetic critique of the Christian concept of God from a Theravadin Buddhist perspective.

Christianity

Main article: Christian apologetics

The Shield of the Trinity, a diagram frequently used by Christian apologists to explain the Trinity

Christian apologetics combines Christian theology, natural theology, and philosophy in an attempt to present a rational basis for the Christian faith, to defend the faith against objections and misrepresentation, and to show that the Christian doctrine is the only world-view that is faultless and consistent with all fundamental knowledge and questions.

Christian apologetics has taken many forms over the centuries. In the Roman Empire, Christians were severely persecuted, and many charges were brought against them. Examples in the Bible include the Apostle Paul's address to the Athenians in the Areopagus (Acts 17: 22-34). J. David Cassel gives several examples: Tacitus wrote that Nero fabricated charges that Christians started the burning of Rome. Other charges included cannibalism (due to a literal interpretation of the Eucharist) and incest (due to early Christians' practice of addressing each other as "brother" and "sister"). Paul the Apostle, Justin Martyr, Irenaeus, and others often defended Christianity against charges that were brought to justify persecution.

Later apologists have focused on providing reasons to accept various aspects of Christian belief. Christian apologists of many traditions, in common with Jews, Muslims, and some others, argue for the existence of a unique and personal God. Theodicy is one important aspect of such arguments, and Alvin Plantinga's arguments have been highly influential in this area. Many prominent Christian apologists are scholarly philosophers or theologians, frequently with additional doctoral work in physics, cosmology, comparative religions, and other fields. Others take a more popular or pastoral approach. Some prominent modern apologists are Douglas Groothuis, Frederick Copleston, John Lennox, Walter R. Martin, Dinesh D'Souza, Douglas Wilson, Cornelius Van Til, Gordon Clark, Francis Schaeffer, Greg Bahnsen, Edward John Carnell, James White, R. C. Sproul, Hank Hanegraaff, Alister McGrath, Lee Strobel, Josh McDowell, Peter Kreeft, G. K. Chesterton, William Lane Craig, J. P. Moreland, Hugh Ross, David Bentley Hart, Gary Habermas, Norman Geisler, Scott Hahn, RC Kunst, Trent Horn, and Jimmy Akin.

Apologists in the Catholic Church include Bishop Robert Barron, G. K. Chesterton, Dr. Scott Hahn, Trent Horn, Jimmy Akin, Patrick Madrid, Kenneth Hensley, Karl Keating, Ronald Knox, and Peter Kreeft.

John Henry Newman (1801–1890) was an English convert to Roman Catholicism, later made a cardinal, and beatified in 2010. In early life he was a major figure in the Oxford Movement to bring the Church of England back to its Catholic roots. Eventually his studies in history persuaded him to become a Roman Catholic. When John Henry Newman entitled his spiritual autobiography Apologia Pro Vita Sua',' in 1864, he was playing upon both this connotation, and the more commonly understood meaning of an expression of contrition or regret.

Christian apologists employ a variety of philosophical and formal approaches, including ontological, cosmological, and teleological arguments. The Christian presuppositionalist approach to apologetics uses the transcendental argument for the existence of God.

Tertullian was an early Christian apologist. He was born, lived, and died in Carthage. He is sometimes known as the "Father of the Latin Church". He introduced the term Trinity (Latin: trinitas) to the Christian vocabulary and probably the formula "three Persons, one Substance" as the Latin "tres Personae, una Substantia" (from the Koine Greek "treis Hypostaseis, Homoousios"), and the terms Vetus Testamentum (Old Testament) and Novum Testamentum (New Testament).

Latter-day Saints

Further information: Mormon studies § Apologetics

There are Latter-day Saint apologists who focus on the defense of Mormonism, including early church leaders, such as Parley P. Pratt, John Taylor, B. H. Roberts, and James E. Talmage, and modern figures, such as Hugh Nibley, Daniel C. Peterson, John L. Sorenson, John Gee, Orson Scott Card, and Jeff Lindsay.

Several well known apologetic organizations of the Church of Jesus Christ of Latter-day Saints, such as the Foundation for Ancient Research and Mormon Studies (a group of scholars at Brigham Young University) and FairMormon (an independent, not-for-profit group run by Latter Day Saints), have been formed to defend the doctrines and history of the Latter Day Saint movement in general and the Church of Jesus Christ of Latter-day Saints in particular.

Deism

Deism is a form of theism in which God created the universe and established rationally comprehensible moral and natural laws but no longer intervenes in human affairs. Deism is a natural religion where belief in God is based on application of reason and evidence observed in the designs and laws found in nature. The World Order of Deists maintains a web site presenting deist apologetics that demonstrate the existence of God based on evidence and reason, absent divine revelation.

Hinduism

Hindu apologetics began developing during the British colonial period. A number of Indian intellectuals had become critical of the British tendency to devalue the Hindu religious tradition. As a result, these Indian intellectuals, as well as a handful of British Indologists, were galvanized to examine the roots of the religion as well as to study its vast arcana and corpus in an analytical fashion. This endeavor drove the deciphering and preservation of Sanskrit. Many translations of Hindu texts were produced which made them accessible to a broader reading audience.

In the early 18th century, Christian missionary Bartholomäus Ziegenbalg engaged in dialogues with several Tamil-speaking Malabarian Hindu priests, and recorded arguments of these Hindu apologists. These records include German-language reports submitted to the Lutheran headquarters in Halle, and 99 letters written by the Hindu priests to him (later translated into German under the title Malabarische Korrespondenz from 1718 onwards).

During 1830–1831, missionary John Wilson engaged in debates with Hindu apologists in Bombay. In 1830, his protege Ram Chandra, a Hindu convert to Christianity, debated with several Hindu Brahmin apologists in public. Hindu pandit Morobhatt Dandekar summarized his arguments from his 1831 debate with Wilson in a Marathi-language work titled Shri-Hindu-dharma-sthapana. Narayana Rao, another Hindu apologist, wrote Svadesha-dharma-abhimani in response to Wilson.

In the mid-19th century, several Hindu apologist works were written in response to John Muir's Mataparīkṣā. These include Mata-parīkṣā-śikṣā (1839) by Somanatha of Central India, Mataparīkṣottara (1840) by Harachandra Tarkapanchanan of Calcutta, Śāstra-tattva-vinirṇaya (1844-1845) by Nilakantha Gore of Benares, and a critique (published later in 1861 as part of Dharmādharma-parīkṣā-patra) by an unknown Vaishnava writer.

A range of Indian philosophers, including Swami Vivekananda and Aurobindo Ghose, have written rational explanations regarding the values of the Hindu religious tradition. More modern proponents such as the Maharishi Mahesh Yogi have also tried to correlate recent developments from quantum physics and consciousness research with Hindu concepts. The late Reverend Pandurang Shastri Athavale has given a plethora of discourses regarding the symbolism and rational basis for many principles in the Vedic tradition. In his book The Cradle of Civilization, David Frawley, an American who has embraced the Vedic tradition, has characterized the ancient texts of the Hindu heritage as being like "pyramids of the spirit".

Islam

See also: Kalam

'Ilm al-Kalām, literally "science of discourse", usually foreshortened to kalam and sometimes called Islamic scholastic theology, is an Islamic undertaking born out of the need to establish and defend the tenets of Islamic faith against skeptics and detractors. A scholar of kalam is referred to as a mutakallim (plural mutakallimūn) as distinguished from philosophers, jurists, and scientists.

Judaism

See also: Jewish polemics and apologetics in the Middle Ages

Jewish apologetic literature can be traced back as far as Aristobulus of Paneas, though some discern it in the works of Demetrius the chronographer (3rd century BCE) traces of the style of "questions" and "solutions" typical of the genre. Aristobulus was a Jewish philosopher of Alexandria and the author of an apologetic work addressed to Ptolemy VI Philometor. Josephus's Contra Apion is a wide-ranging defense of Judaism against many charges laid against Judaism at that time, as too are some of the works of Philo of Alexandria.

In response to modern Christian missionaries, and congregations that "are designed to appear Jewish, but are actually fundamentalist Christian churches, which use traditional Jewish symbols to lure the most vulnerable of our Jewish people into their ranks", Jews for Judaism is the largest counter-missionary organization in existence, today. Kiruv Organization (Mizrachi), founded by Rabbi Yosef Mizrachi, and Outreach Judaism, founded by Rabbi Tovia Singer, are other prominent international organizations that respond "directly to the issues raised by missionaries and cults, by exploring Judaism in contradistinction to fundamentalist Christianity."

Pantheism

Some pantheists have formed organizations such as the World Pantheist Movement and the Universal Pantheist Society to promote and defend the belief in pantheism.

Native Americans

In a famous speech called "Red Jacket on Religion for the White Man and the Red" in 1805, Seneca chief Red Jacket gave an apologetic for Native American religion.

In literature

Plato's Apology may be read as both a religious and literary apology; however, more specifically literary examples may be found in the prefaces and dedications, which proceed many Early Modern plays, novels, and poems. Eighteenth century authors such as Colley Cibber, Frances Burney, and William Congreve, to name but a few, prefaced the majority of their poetic work with such apologies. In addition to the desire to defend their work, the apologetic preface often suggests the author's attempt to humble his- or herself before the audience.