Geophysics

From Wikipedia, the free encyclopedia

Age of the sea floor. Much of the dating information comes from magnetic anomalies.

Computer simulation of the Earth's magnetic field in a period of normal polarity between reversals.

Geophysics /dʒiːoʊfɪzɪks/ is a subject of natural science concerned with the physical processes and physical properties of the Earth and its surrounding space environment, and the use of quantitative methods for their analysis. The term geophysics sometimes refers only to the geological applications: Earth's shape; its gravitational and magnetic fields; its internal structure and composition; its dynamics and their surface expression in plate tectonics, the generation of magmas, volcanism and rock formation.^[1] However, modern geophysics organizations use a broader definition that includes the water cycle including snow and ice; fluid dynamics of the oceans and the atmosphere; electricity and magnetism in the ionosphere and magnetosphere and solar-terrestrial relations; and analogous problems associated with the Moon and other planets.^[1][2][3]

Although geophysics was only recognized as a separate discipline in the 19th century, its origins date back to ancient times. The first magnetic compasses were made from lodestones, while more modern magnetic compasses played an important role in the history of navigation. The first seismic instrument was built in 132 AD. Isaac Newton applied his theory of mechanics to the tides and the precession of the equinox; and instruments were developed to measure the Earth's shape, density and gravity field, as well as the components of the water cycle. In the 20th century, geophysical methods were developed for remote exploration of the solid Earth and the ocean, and geophysics played an essential role in the development of the theory of plate tectonics.

Geophysics is applied to societal needs, such as mineral resources, mitigation of natural hazards and environmental protection.^[2] In Exploration Geophysics, Geophysical survey data are used to analyze potential petroleum reservoirs and mineral deposits, locate groundwater, find archaeological relics, determine the thickness of glaciers and soils, and assess sites for environmental remediation.

Physical phenomena

Geophysics is a highly interdisciplinary subject, and geophysicists contribute to every area of the Earth sciences. To provide a clearer idea of what constitutes geophysics, this section describes phenomena that are studied in physics and how they relate to the Earth and its surroundings.

Gravity

A map of deviations in gravity from a perfectly smooth, idealized Earth.

The gravitational pull of the Moon and Sun give rise to two high tides and two low tides every lunar day, or every 24 hours and 50 minutes. Therefore, there is a gap of 12 hours and 25 minutes between every high tide and between every low tide.^[4]

Gravitational forces make rocks press down on deeper rocks, increasing their density as the depth increases.^[5] Measurements of gravitational acceleration and gravitational potential at the Earth's surface and above it can be used to look for mineral deposits (see gravity anomaly and gravimetry).^[6] The surface gravitational field provides information on the dynamics of tectonic plates. The geopotential surface called the geoid is one definition of the shape of the Earth. The geoid would be the global mean sea level if the oceans were in equilibrium and could be extended through the continents (such as with very narrow canals).^[7]

Heat flow

A model of thermal convection in the Earth's mantle. The thin red columns are mantle plumes.

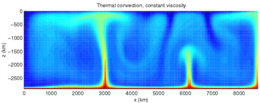
The Earth is cooling, and the resulting heat flow generates the Earth's magnetic field through the geodynamo and plate tectonics through mantle convection.^[8] The main sources of heat are the primordial heat and radioactivity, although there are also contributions from phase transitions. Heat is mostly carried to the surface by thermal convection, although there are two thermal boundary layers – the core-mantle boundary and the lithosphere – in which heat is transported by conduction.^[9] Some heat is carried up from the bottom of the mantle by mantle plumes. The heat flow at the Earth's surface is about 4.2 × 10¹³ W, and it is a potential source of geothermal energy.^[10]

Vibrations

Illustration of the deformations of a block by body waves and surface waves (see seismic wave).

Seismic waves are vibrations that travel through the Earth's interior or along its surface. The entire Earth can also oscillate in forms that are called normal modes or free oscillations of the Earth. Ground motions from waves or normal modes are measured using seismographs. If the waves come from a localized source such as an earthquake or explosion, measurements at more than one location can be used to locate the source. The locations of earthquakes provide information on plate tectonics and mantle convection.^[11][12]

Measurements of seismic waves are a source of information on the region that the waves travel through. If the density or composition of the rock changes suddenly, some waves are reflected. Reflections can provide information on near-surface structure.^[6] Changes in the travel direction, called refraction, can be used to infer the deep structure of the Earth.^[12]

Earthquakes pose a risk to humans. Understanding their mechanisms, which depend on the type of earthquake (e.g., intraplate or deep focus), can lead to better estimates of earthquake risk and improvements in earthquake engineering.^[13]

Electricity

Although we mainly notice electricity during thunderstorms, there is always a downward electric field near the surface that averages 120 volts per meter.^[14] Relative to the solid Earth, the atmosphere has a net positive charge due to bombardment by cosmic rays. A current of about 1800 amperes flows in the global circuit.^[14] It flows downward from the ionosphere over most of the Earth and back upwards through thunderstorms. The flow is manifested by lightning below the clouds and sprites above.

A variety of electric methods are used in geophysical survey. Some measure spontaneous potential, a potential that arises in the ground because of man-made or natural disturbances. Telluric currents flow in Earth and the oceans. They have two causes: electromagnetic induction by the time-varying, external-origin geomagnetic field and motion of conducting bodies (such as seawater) across the Earth's permanent magnetic field.^[15] The distribution of telluric current density can be used to detect variations in electrical resistivity of underground structures. Geophysicists can also provide the electric current themselves (see induced polarization and electrical resistivity tomography).

Electromagnetic waves

Electromagnetic waves occur in the ionosphere and magnetosphere as well as the Earth's outer core. Dawn chorus is believed to be caused by high-energy electrons that get caught in the Van Allen radiation belt. Whistlers are produced by lightning strikes. Hiss may be generated by both. Electromagnetic waves may also be generated by earthquakes (see seismo-electromagnetics).

In the Earth's outer core, electric currents in the highly conductive liquid iron create magnetic fields by electromagnetic induction (see geodynamo). Alfvén waves are magnetohydrodynamic waves in the magnetosphere or the Earth's core. In the core, they probably have little observable effect on the geomagnetic field, but slower waves such as magnetic Rossby waves may be one source of geomagnetic secular variation.^[16]

Electromagnetic methods that are used for geophysical survey include transient electromagnetics and magnetotellurics.

Magnetism

The Earth's magnetic field protects the Earth from the deadly solar wind and has long been used for navigation. It originates in the fluid motions of the Earth's outer core (see geodynamo).^[16] The magnetic field in the upper atmosphere gives rise to the auroras.^[17]

Earth's dipole axis (pink line) is tilted away from the rotational axis (blue line).

The Earth's field is roughly like a tilted dipole, but it changes over time (a phenomenon called geomagnetic secular variation). Mostly the geomagnetic pole stays near the geographic pole, but at random intervals averaging 440,000 to a million years or so, the polarity of the Earth's field reverses. These geomagnetic reversals, analyzed within a Geomagnetic Polarity Time Scale, contain 184 polarity intervals in the last 83 million years, with change in frequency over time, with the most recent brief complete reversal of the Laschamp event occurring 41,000 years ago during the last glacial period. Geologists observed geomagnetic reversal recorded in volcanic rocks, through magnetostratigraphy correlation (see natural remanent magnetization) and their signature can be seen as parallel linear magnetic anomaly stripes on the seafloor. These stripes provide quantitative information on seafloor spreading, a part of plate tectonics. They are the basis of magnetostratigraphy, which correlates magnetic reversals with other stratigraphies to construct geologic time scales.^[18] In addition, the magnetization in rocks can be used to measure the motion of continents.^[16]

Radioactivity

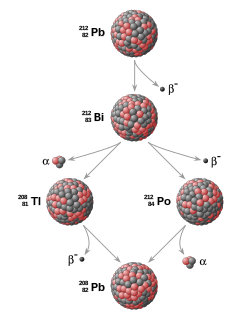
Example of a radioactive decay chain (see Radiometric dating).

Radioactive decay accounts for about 80% of the Earth's internal heat, powering the geodynamo and plate tectonics.^[19] The main heat-producing isotopes are potassium-40, uranium-238, uranium-235, and thorium-232.^[20] Radioactive elements are used for radiometric dating, the primary method for establishing an absolute time scale in geochronology. Unstable isotopes decay at predictable rates, and the decay rates of different isotopes cover several orders of magnitude, so radioactive decay can be used to accurately date both recent events and events in past geologic eras.^[21] Radiometric mapping using ground and airborne gamma spectrometry can be used to map the concentration and distribution of radioisotopes near the Earth's surface, which is useful for mapping lithology and alteration.^[22][23]

Fluid dynamics

Fluid motions occur in the magnetosphere, atmosphere, ocean, mantle and core. Even the mantle, though it has an enormous viscosity, flows like a fluid over long time intervals (see geodynamics). This flow is reflected in phenomena such as isostasy, post-glacial rebound and mantle plumes. The mantle flow drives plate tectonics and the flow in the Earth's core drives the geodynamo.^[16]

Geophysical fluid dynamics is a primary tool in physical oceanography and meteorology. The rotation of the Earth has profound effects on the Earth's fluid dynamics, often due to the Coriolis effect. In the atmosphere it gives rise to large-scale patterns like Rossby waves and determines the basic circulation patterns of storms. In the ocean they drive large-scale circulation patterns as well as Kelvin waves and Ekman spirals at the ocean surface.^[24] In the Earth's core, the circulation of the molten iron is structured by Taylor columns.^[16]

Waves and other phenomena in the magnetosphere can be modeled using magnetohydrodynamics.

Mineral physics

The physical properties of minerals must be understood to infer the composition of the Earth's interior from seismology, the geothermal gradient and other sources of information. Mineral physicists study the elastic properties of minerals; their high-pressure phase diagrams, melting points and equations of state at high pressure; and the rheological properties of rocks, or their ability to flow. Deformation of rocks by creep make flow possible, although over short times the rocks are brittle. The viscosity of rocks is affected by temperature and pressure, and in turn determines the rates at which tectonic plates move (see geodynamics).^[5]

Water is a very complex substance and its unique properties are essential for life.^[25] Its physical properties shape the hydrosphere and are an essential part of the water cycle and climate. Its thermodynamic properties determine evaporation and the thermal gradient in the atmosphere. The many types of precipitation involve a complex mixture of processes such as coalescence, supercooling and supersaturation.^[26] Some precipitated water becomes groundwater, and groundwater flow includes phenomena such as percolation, while the conductivity of water makes electrical and electromagnetic methods useful for tracking groundwater flow. Physical properties of water such as salinity have a large effect on its motion in the oceans.^[24]

The many phases of ice form the cryosphere and come in forms like ice sheets, glaciers, sea ice, freshwater ice, snow, and frozen ground (or permafrost).^[27]

Regions of the Earth

Size and form of the Earth

The Earth is roughly spherical, but it bulges towards the Equator, so it is roughly in the shape of an ellipsoid (see Earth ellipsoid). This bulge is due to its rotation and is nearly consistent with an Earth in hydrostatic equilibrium. The detailed shape of the Earth, however, is also affected by the distribution of continents and ocean basins, and to some extent by the dynamics of the plates.^[7]

Structure of the interior

Seismic velocities and boundaries in the interior of the Earth sampled by seismic waves.

Evidence from seismology, heat flow at the surface, and mineral physics is combined with the Earth's mass and moment of inertia to infer models of the Earth's interior – its composition, density, temperature, pressure. For example, the Earth's mean specific gravity (5.515) is far higher than the typical specific gravity of rocks at the surface (2.7–3.3), implying that the deeper material is denser. This is also implied by its low moment of inertia ( 0.33 M R², compared to 0.4 M R² for a sphere of constant density). However, some of the density increase is compression under the enormous pressures inside the Earth. The effect of pressure can be calculated using the Adams–Williamson equation. The conclusion is that pressure alone cannot account for the increase in density. Instead, we know that the Earth's core is composed of an alloy of iron and other minerals.^[5]

Reconstructions of seismic waves in the deep interior of the Earth show that there are no S-waves in the outer core. This indicates that the outer core is liquid, because liquids cannot support shear. The outer core is liquid, and the motion of this highly conductive fluid generates the Earth's field (see geodynamo). The inner core, however, is solid because of the enormous pressure.^[7]

Reconstruction of seismic reflections in the deep interior indicate some major discontinuities in seismic velocities that demarcate the major zones of the Earth: inner core, outer core, mantle, lithosphere and crust. The mantle itself is divided into the upper mantle, transition zone, lower mantle and D′′ layer. Between the crust and the mantle is the Mohorovičić discontinuity.^[7]

The seismic model of the Earth does not by itself determine the composition of the layers. For a complete model of the Earth, mineral physics is needed to interpret seismic velocities in terms of composition. The mineral properties are temperature-dependent, so the geotherm must also be determined. This requires physical theory for thermal conduction and convection and the heat contribution of radioactive elements. The main model for the radial structure of the interior of the Earth is the preliminary reference Earth model (PREM). Some parts of this model have been updated by recent findings in mineral physics (see post-perovskite) and supplemented by seismic tomography. The mantle is mainly composed of silicates, and the boundaries between layers of the mantle are consistent with phase transitions.^[5]

The mantle acts as a solid for seismic waves, but under high pressures and temperatures it deforms so that over millions of years it acts like a liquid. This makes plate tectonics possible. Geodynamics is the study of the fluid flow in the mantle and core.

Magnetosphere

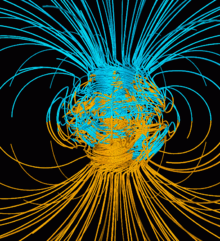
Schematic of Earth's magnetosphere. The solar wind flows from left to right.

If a planet's magnetic field is strong enough, its interaction with the solar wind forms a magnetosphere. Early space probes mapped out the gross dimensions of the Earth's magnetic field, which extends about 10 Earth radii towards the Sun. The solar wind, a stream of charged particles, streams out and around the terrestrial magnetic field, and continues behind the magnetic tail, hundreds of Earth radii downstream. Inside the magnetosphere, there are relatively dense regions of solar wind particles called the Van Allen radiation belts.^[17]

Methods

Geodesy

Geophysical measurements are generally at a particular time and place. Accurate measurements of position, along with earth deformation and gravity, are the province of geodesy. While geodesy and geophysics are separate fields, the two are so closely connected that many scientific organizations such as the American Geophysical Union, the Canadian Geophysical Union and the International Union of Geodesy and Geophysics encompass both.^[28]

Absolute positions are most frequently determined using the global positioning system (GPS). A three-dimensional position is calculated using messages from four or more visible satellites and referred to the 1980 Geodetic Reference System. An alternative, optical astronomy, combines astronomical coordinates and the local gravity vector to get geodetic coordinates. This method only provides the position in two coordinates and is more difficult to use than GPS. However, it is useful for measuring motions of the Earth such as nutation and Chandler wobble. Relative positions of two or more points can be determined using very-long-baseline interferometry.^[28][29][30]

Gravity measurements became part of geodesy because they were needed to related measurements at the surface of the Earth to the reference coordinate system. Gravity measurements on land can be made using gravimeters deployed either on the surface or in helicopter flyovers. Since the 1960s, the Earth's gravity field has been measured by analyzing the motion of satellites. Sea level can also be measured by satellites using radar altimetry, contributing to a more accurate geoid.^[28] In 2002, NASA launched the Gravity Recovery and Climate Experiment (GRACE), wherein two twin satellites map variations in Earth's gravity field by making measurements of the distance between the two satellites using GPS and a microwave ranging system. Gravity variations detected by GRACE include those caused by changes in ocean currents; runoff and ground water depletion; melting ice sheets and glaciers.^[31]

Satellites and space probes

Satellites in space have made it possible to collect data from not only the visible light region, but in other areas of the electromagnetic spectrum. The planets can be characterized by their force fields: gravity and their magnetic fields, which are studied through geophysics and space physics.

Measuring the changes in acceleration experienced by spacecraft as they orbit has allowed fine details of the gravity fields of the planets to be mapped. For example, in the 1970s, the gravity field disturbances above lunar maria were measured through lunar orbiters, which led to the discovery of concentrations of mass, mascons, beneath the Imbrium, Serenitatis, Crisium, Nectaris and Humorum basins.^[32]

History

Geophysics emerged as a separate discipline only in the 19th century, from the intersection of physical geography, geology, astronomy, meteorology, and physics.^[33][34] However, many geophysical phenomena – such as the Earth's magnetic field and earthquakes – have been investigated since the ancient era.

Ancient and classical eras

Replica of Zhang Heng's seismoscope, possibly the first contribution to seismology.

The magnetic compass existed in China back as far as the fourth century BC. It was used as much for feng shui as for navigation on land. It was not until good steel needles could be forged that compasses were used for navigation at sea; before that, they could not retain their magnetism long enough to be useful. The first mention of a compass in Europe was in 1190 AD.^[35]

In circa 240 BC, Eratosthenes of Cyrene deduced that the Earth was round and measured the circumference of the Earth, using trigonometry and the angle of the Sun at more than one latitude in Egypt. He developed a system of latitude and longitude.^[36]

Perhaps the earliest contribution to seismology was the invention of a seismoscope by the prolific inventor Zhang Heng in 132 AD.^[37] This instrument was designed to drop a bronze ball from the mouth of a dragon into the mouth of a toad. By looking at which of eight toads had the ball, one could determine the direction of the earthquake. It was 1571 years before the first design for a seismoscope was published in Europe, by Jean de la Hautefeuille. It was never built.^[38]

Beginnings of modern science

One of the publications that marked the beginning of modern science was William Gilbert's De Magnete (1600), a report of a series of meticulous experiments in magnetism. Gilbert deduced that compasses point north because the Earth itself is magnetic.^[16]

In 1687 Isaac Newton published his Principia, which not only laid the foundations for classical mechanics and gravitation but also explained a variety of geophysical phenomena such as the tides and the precession of the equinox.^[39]

The first seismometer, an instrument capable of keeping a continuous record of seismic activity, was built by James Forbes in 1844.^[38]

Torque

From Wikipedia, the free encyclopedia

Torque
Common symbols	${\displaystyle \tau }$, M
SI unit	N⋅m

Relationship between force F, torque τ, linear momentum p, and angular momentum L in a system which has rotation constrained in one plane only (forces and moments due to gravity and friction not considered).

Torque, moment, or moment of force is rotational force.^[1] Just as a linear force is a push or a pull, a torque can be thought of as a twist to an object. In three dimensions, the torque is a pseudovector; for point particles, it is given by the cross product of the position vector (distance vector) and the force vector.

The symbol for torque is typically ${\displaystyle \tau }$, the lowercase Greek letter tau. When it is called moment of force, it is commonly denoted by M.

The magnitude of torque of a rigid body depends on three quantities: the force applied, the lever arm vector^[2] connecting the origin to the point of force application, and the angle between the force and lever arm vectors. In symbols:

${\displaystyle {\boldsymbol {\tau }}=\mathbf {r} \times \mathbf {F} \,\!}$

${\displaystyle \tau =\|\mathbf {r} \|\,\|\mathbf {F} \|\sin \theta \,\!}$

where

${\displaystyle {\boldsymbol {\tau }}}$ is the torque vector and ${\displaystyle \tau }$ is the magnitude of the torque,

r is the position vector (a vector from the origin of the coordinate system defined to the point where the force is applied)

F is the force vector,

× denotes the cross product,which is defined as magnitudes of the respective vectors times sin θ.

θ is the angle between the force vector and the lever arm vector.

The SI unit for torque is N⋅m.

Defining terminology

Torque is referred to using different vocabulary depending on geographical location and field of study. This article refers to the definition used in US physics in its usage of the word torque.^[3] In the UK and in US mechanical engineering, torque is referred to as moment of force, usually shortened to moment.^[4] In US physics^[3] and UK physics terminology these terms are interchangeable, unlike in US mechanical engineering, where the term torque is used for the closely related "resultant moment of a couple".^[4]

Torque is defined mathematically as the rate of change of angular momentum of an object. The definition of torque states that one or both of the angular velocity or the moment of inertia of an object are changing. Moment is the general term used for the tendency of one or more applied forces to rotate an object about an axis, but not necessarily to change the angular momentum of the object (the concept which is called torque in physics).^[4] For example, a rotational force applied to a shaft causing acceleration, such as a drill bit accelerating from rest, results in a moment called a torque. By contrast, a lateral force on a beam produces a moment (called a bending moment), but since the angular momentum of the beam is not changing, this bending moment is not called a torque. Similarly with any force couple on an object that has no change to its angular momentum, such moment is also not called a torque.

This article follows the US physics terminology by calling all moments by the term torque, whether or not they cause the angular momentum of an object to change.

History

The concept of torque, also called moment or couple, originated with the studies of Archimedes on levers. The term torque was apparently introduced into English scientific literature by James Thomson, the brother of Lord Kelvin, in 1884.^[5]

Definition and relation to angular momentum

A particle is located at position r relative to its axis of rotation. When a force F is applied to the particle, only the perpendicular component F_⊥ produces a torque. This torque τ = r × F has magnitude τ = |r| |F_⊥| = |r| |F| sin θ and is directed outward from the page.

A force applied at a right angle to a lever multiplied by its distance from the lever's fulcrum (the length of the lever arm) is its torque. A force of three newtons applied two metres from the fulcrum, for example, exerts the same torque as a force of one newton applied six metres from the fulcrum. The direction of the torque can be determined by using the right hand grip rule: if the fingers of the right hand are curled from the direction of the lever arm to the direction of the force, then the thumb points in the direction of the torque.^[6]

More generally, the torque on a particle (which has the position r in some reference frame) can be defined as the cross product:

${\displaystyle {\boldsymbol {\tau }}=\mathbf {r} \times \mathbf {F} ,}$

where r is the particle's position vector relative to the fulcrum, and F is the force acting on the particle. The magnitude τ of the torque is given by

${\displaystyle \tau =rF\sin \theta ,\!}$

where r is the distance from the axis of rotation to the particle, F is the magnitude of the force applied, and θ is the angle between the position and force vectors. Alternatively,

${\displaystyle \tau =rF_{\perp },}$

where F_⊥ is the amount of force directed perpendicularly to the position of the particle. Any force directed parallel to the particle's position vector does not produce a torque.^[7][8]

It follows from the properties of the cross product that the torque vector is perpendicular to both the position and force vectors. The torque vector points along the axis of the rotation that the force vector (starting from rest) would initiate. The resulting torque vector direction is determined by the right-hand rule.^[7]

The unbalanced torque on a body along axis of rotation determines the rate of change of the body's angular momentum,

${\displaystyle {\boldsymbol {\tau }}={\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}}$

where L is the angular momentum vector and t is time. If multiple torques are acting on the body, it is instead the net torque which determines the rate of change of the angular momentum:

${\displaystyle {\boldsymbol {\tau }}_{1}+\cdots +{\boldsymbol {\tau }}_{n}={\boldsymbol {\tau }}_{\mathrm {net} }={\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}.}$

For the motion of a point particle,

${\displaystyle \mathbf {L} =I{\boldsymbol {\omega }},}$

where I is the moment of inertia and ω is the angular velocity. It follows that

${\displaystyle {\boldsymbol {\tau }}_{\mathrm {net} }={\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}={\frac {\mathrm {d} (I{\boldsymbol {\omega }})}{\mathrm {d} t}}=I{\frac {\mathrm {d} {\boldsymbol {\omega }}}{\mathrm {d} t}}+{\frac {\mathrm {d} I}{\mathrm {d} t}}{\boldsymbol {\omega }}=I{\boldsymbol {\alpha }}+{\frac {\mathrm {d} (mr^{2})}{\mathrm {d} t}}{\boldsymbol {\omega }}=I{\boldsymbol {\alpha }}+2rp_{||}{\boldsymbol {\omega }},}$

where α is the angular acceleration of the particle, and p_|| is the radial component of its linear momentum. This equation is the rotational analogue of Newton's Second Law for point particles, and is valid for any type of trajectory. Note that although force and acceleration are always parallel and directly proportional, the torque τ need not be parallel or directly proportional to the angular acceleration α. This arises from the fact that although mass is always conserved, the moment of inertia in general is not.

Proof of the equivalence of definitions

The definition of angular momentum for a single particle is:

${\displaystyle \mathbf {L} =\mathbf {r} \times {\boldsymbol {p}}}$

where "×" indicates the vector cross product, p is the particle's linear momentum, and r is the displacement vector from the origin (the origin is assumed to be a fixed location anywhere in space). The time-derivative of this is:

${\displaystyle {\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}=\mathbf {r} \times {\frac {\mathrm {d} {\boldsymbol {p}}}{\mathrm {d} t}}+{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\times {\boldsymbol {p}}.}$

This result can easily be proven by splitting the vectors into components and applying the product rule. Now using the definition of force ${\displaystyle \mathbf {F} ={\frac {\mathrm {d} {\boldsymbol {p}}}{\mathrm {d} t}}}$ (whether or not mass is constant) and the definition of velocity ${\displaystyle {\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}=\mathbf {v} }$

${\displaystyle {\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}=\mathbf {r} \times \mathbf {F} +\mathbf {v} \times {\boldsymbol {p}}.}$

The cross product of momentum ${\displaystyle {\boldsymbol {p}}}$ with its associated velocity ${\displaystyle \mathbf {v} }$ is zero because velocity and momentum are parallel, so the second term vanishes.

By definition, torque τ = r × F. Therefore, torque on a particle is equal to the first derivative of its angular momentum with respect to time.

If multiple forces are applied, Newton's second law instead reads F_net = ma, and it follows that

${\displaystyle {\frac {\mathrm {d} \mathbf {L} }{\mathrm {d} t}}=\mathbf {r} \times \mathbf {F} _{\mathrm {net} }={\boldsymbol {\tau }}_{\mathrm {net} }.}$

This is a general proof.

Units

Torque has dimension force times distance, symbolically L²MT⁻². Official SI literature suggests using the unit newton metre (N⋅m) or the unit joule per radian.^[9] The unit newton metre is properly denoted N⋅m or N m.^[10] This avoids ambiguity with mN, millinewtons.

The SI unit for energy or work is the joule. It is dimensionally equivalent to a force of one newton acting over a distance of one metre, but it is not used for torque. Energy and torque are entirely different concepts, so the practice of using different unit names (i.e., reserving newton metres for torque and using only joules for energy) helps avoid mistakes and misunderstandings.^[9] The dimensional equivalence of these units is not simply a coincidence: a torque of 1 N⋅m applied through a full revolution will require an energy of exactly 2π joules. Mathematically,

${\displaystyle E=\tau \theta \ }$

where E is the energy, τ is magnitude of the torque, and θ is the angle moved (in radians). This equation motivates the alternate unit name joules per radian.^[9]

In Imperial units, "pound-force-feet" (lbf⋅ft), "foot-pounds-force", "inch-pounds-force", "ounce-force-inches" (ozf⋅in)^{[citation needed]} are used, and other non-SI units of torque includes "metre-kilograms-force". For all these units, the word "force" is often left out.^[11] For example, abbreviating "pound-force-foot" to simply "pound-foot" (in this case, it would be implicit that the "pound" is pound-force and not pound-mass). This is an example of the confusion caused by the use of English units that may be avoided with SI units because of the careful distinction in SI between force (in newtons) and mass (in kilograms).

Torque is sometimes listed with units that do not make dimensional sense, such as the gram-centimeter. In this case, "gram" should be understood as the force given by the weight of 1 gram on the surface of the Earth (i.e. 0.00980665 N). The surface of the Earth has a standard gravitational field strength of 9.80665 N/kg.

Special cases and other facts

Moment arm formula

Moment arm diagram

A very useful special case, often given as the definition of torque in fields other than physics, is as follows:

${\displaystyle \tau =({\text{moment arm}})({\text{force}}).}$

The construction of the "moment arm" is shown in the figure to the right, along with the vectors r and F mentioned above. The problem with this definition is that it does not give the direction of the torque but only the magnitude, and hence it is difficult to use in three-dimensional cases. If the force is perpendicular to the displacement vector r, the moment arm will be equal to the distance to the centre, and torque will be a maximum for the given force. The equation for the magnitude of a torque, arising from a perpendicular force:

${\displaystyle \tau =({\text{distance to centre}})({\text{force}}).}$

For example, if a person places a force of 10 N at the terminal end of a wrench that is 0.5 m long (or a force of 10 N exactly 0.5 m from the twist point of a wrench of any length), the torque will be 5 N.m – assuming that the person moves the wrench by applying force in the plane of movement and perpendicular to the wrench.

The torque caused by the two opposing forces F_g and −F_g causes a change in the angular momentum L in the direction of that torque. This causes the top to precess.

Static equilibrium

For an object to be in static equilibrium, not only must the sum of the forces be zero, but also the sum of the torques (moments) about any point. For a two-dimensional situation with horizontal and vertical forces, the sum of the forces requirement is two equations: ΣH = 0 and ΣV = 0, and the torque a third equation: Στ = 0. That is, to solve statically determinate equilibrium problems in two-dimensions, three equations are used.

Net force versus torque

When the net force on the system is zero, the torque measured from any point in space is the same. For example, the torque on a current-carrying loop in a uniform magnetic field is the same regardless of your point of reference. If the net force ${\displaystyle \mathbf {F} }$ is not zero, and ${\displaystyle {\boldsymbol {\tau }}_{1}}$ is the torque measured from ${\displaystyle \mathbf {r} _{1}}$, then the torque measured from ${\displaystyle \mathbf {r} _{2}}$ is … ${\displaystyle {\boldsymbol {\tau }}_{2}={\boldsymbol {\tau }}_{1}+(\mathbf {r} _{1}-\mathbf {r} _{2})\times \mathbf {F} }$

Machine torque

Torque curve of a motorcycle ("BMW K 1200 R 2005"). The horizontal axis is the speed (in rpm) that the crankshaft is turning, and the vertical axis is the torque (in newton metres) that the engine is capable of providing at that speed.

Torque is part of the basic specification of an engine: the power output of an engine is expressed as its torque multiplied by its rotational speed of the axis. Internal-combustion engines produce useful torque only over a limited range of rotational speeds (typically from around 1,000–6,000 rpm for a small car). The varying torque output over that range can be measured with a dynamometer, and shown as a torque curve.

Steam engines and electric motors tend to produce maximum torque close to zero rpm, with the torque diminishing as rotational speed rises (due to increasing friction and other constraints). Reciprocating steam engines can start heavy loads from zero RPM without a clutch.

Relationship between torque, power, and energy

If a force is allowed to act through a distance, it is doing mechanical work. Similarly, if torque is allowed to act through a rotational distance, it is doing work. Mathematically, for rotation about a fixed axis through the center of mass,

${\displaystyle W=\int _{\theta _{1}}^{\theta _{2}}\tau \ \mathrm {d} \theta ,}$

where W is work, τ is torque, and θ₁ and θ₂ represent (respectively) the initial and final angular positions of the body.^[12]

Proof

The work done by a variable force acting over a finite linear displacement ${\displaystyle s}$ is given by integrating the force with respect to an elemental linear displacement ${\displaystyle \mathrm {d} {\vec {s}}}$

${\displaystyle W=\int _{s_{1}}^{s_{2}}{\vec {F}}\cdot \mathrm {d} {\vec {s}}}$

However, the infinitesimal linear displacement ${\displaystyle \mathrm {d} {\vec {s}}}$ is related to a corresponding angular displacement ${\displaystyle \mathrm {d} {\vec {\theta }}}$ and the radius vector ${\displaystyle {\vec {r}}}$ as

${\displaystyle \mathrm {d} {\vec {s}}=\mathrm {d} {\vec {\theta }}\times {\vec {r}}}$

Substitution in the above expression for work gives

${\displaystyle W=\int _{s_{1}}^{s_{2}}{\vec {F}}\cdot \mathrm {d} {\vec {\theta }}\times {\vec {r}}}$

The expression ${\displaystyle {\vec {F}}\cdot \mathrm {d} {\vec {\theta }}\times {\vec {r}}}$ is a scalar triple product given by ${\displaystyle \left[{\vec {F}}\,\mathrm {d} {\vec {\theta }}\,{\vec {r}}\right]}$. An alternate expression for the same scalar triple product is

${\displaystyle \left[{\vec {F}}\,\mathrm {d} {\vec {\theta }}\,{\vec {r}}\right]={\vec {r}}\times {\vec {F}}\cdot \mathrm {d} {\vec {\theta }}}$

But as per the definition of torque,

${\displaystyle {\vec {\tau }}={\vec {r}}\times {\vec {F}}}$

Corresponding substitution in the expression of work gives,

${\displaystyle W=\int _{s_{1}}^{s_{2}}{\vec {\tau }}\cdot \mathrm {d} {\vec {\theta }}}$

Since the parameter of integration has been changed from linear displacement to angular displacement, the limits of the integration also change correspondingly, giving

${\displaystyle W=\int _{\theta _{1}}^{\theta _{2}}{\vec {\tau }}\cdot \mathrm {d} {\vec {\theta }}}$

If the torque and the angular displacement are in the same direction, then the scalar product reduces to a product of magnitudes; i.e., ${\displaystyle {\vec {\tau }}\cdot \mathrm {d} {\vec {\theta }}=\left|{\vec {\tau }}\right|\left|\,\mathrm {d} {\vec {\theta }}\right|\cos 0=\tau \,\mathrm {d} \theta }$ giving

${\displaystyle W=\int _{\theta _{1}}^{\theta _{2}}\tau \,\mathrm {d} \theta }$

It follows from the work-energy theorem that W also represents the change in the rotational kinetic energy E_r of the body, given by

${\displaystyle E_{\mathrm {r} }={\tfrac {1}{2}}I\omega ^{2},}$

where I is the moment of inertia of the body and ω is its angular speed.^[12]

Power is the work per unit time, given by

${\displaystyle P={\boldsymbol {\tau }}\cdot {\boldsymbol {\omega }},}$

where P is power, τ is torque, ω is the angular velocity, and ⋅ represents the scalar product.

Algebraically, the equation may be rearranged to compute torque for a given angular speed and power output. Note that the power injected by the torque depends only on the instantaneous angular speed – not on whether the angular speed increases, decreases, or remains constant while the torque is being applied (this is equivalent to the linear case where the power injected by a force depends only on the instantaneous speed – not on the resulting acceleration, if any).

In practice, this relationship can be observed in bicycles: Bicycles are typically composed of two road wheels, front and rear gears (referred to as sprockets) meshing with a circular chain, and a derailleur mechanism if the bicycle's transmission system allows multiple gear ratios to be used (i.e. multi-speed bicycle), all of which attached to the frame. A cyclist, the person who rides the bicycle, provides the input power by turning pedals, thereby cranking the front sprocket (commonly referred to as chainring). The input power provided by the cyclist is equal to the product of cadence (i.e. the number of pedal revolutions per minute) and the torque on spindle of the bicycle's crankset. The bicycle's drivetrain transmits the input power to the road wheel, which in turn conveys the received power to the road as the output power of the bicycle. Depending on the gear ratio of the bicycle, a (torque, rpm)_input pair is converted to a (torque, rpm)_output pair. By using a larger rear gear, or by switching to a lower gear in multi-speed bicycles, angular speed of the road wheels is decreased while the torque is increased, product of which (i.e. power) does not change.

Consistent units must be used. For metric SI units, power is watts, torque is newton metres and angular speed is radians per second (not rpm and not revolutions per second).

Also, the unit newton metre is dimensionally equivalent to the joule, which is the unit of energy. However, in the case of torque, the unit is assigned to a vector, whereas for energy, it is assigned to a scalar.

Conversion to other units

A conversion factor may be necessary when using different units of power or torque. For example, if rotational speed (revolutions per time) is used in place of angular speed (radians per time), we multiply by a factor of 2π radians per revolution. In the following formulas, P is power, τ is torque, and ν (Greek letter nu) is rotational speed.

${\displaystyle P=\tau \cdot 2\pi \cdot \nu }$

Showing units:

${\displaystyle P({\rm {W}})=\tau {\rm {(N\cdot m)}}\cdot 2\pi {\rm {(rad/rev)}}\cdot \nu {\rm {(rev/sec)}}}$

Dividing by 60 seconds per minute gives us the following.

${\displaystyle P({\rm {W}})={\frac {\tau {\rm {(N\cdot m)}}\cdot 2\pi {\rm {(rad/rev)}}\cdot \nu {\rm {(rpm)}}}{60}}}$

where rotational speed is in revolutions per minute (rpm).

Some people (e.g., American automotive engineers) use horsepower (imperial mechanical) for power, foot-pounds (lbf⋅ft) for torque and rpm for rotational speed. This results in the formula changing to:

${\displaystyle P({\rm {hp}})={\frac {\tau {\rm {(lbf\cdot ft)}}\cdot 2\pi {\rm {(rad/rev)}}\cdot \nu ({\rm {rpm}})}{33,000}}.}$

The constant below (in foot pounds per minute) changes with the definition of the horsepower; for example, using metric horsepower, it becomes approximately 32,550.

Use of other units (e.g., BTU per hour for power) would require a different custom conversion factor.

Derivation

For a rotating object, the linear distance covered at the circumference of rotation is the product of the radius with the angle covered. That is: linear distance = radius × angular distance. And by definition, linear distance = linear speed × time = radius × angular speed × time.

By the definition of torque: torque = radius × force. We can rearrange this to determine force = torque ÷ radius. These two values can be substituted into the definition of power:

${\displaystyle {\begin{aligned}{\text{power}}&={\frac {{\text{force}}\cdot {\text{linear distance}}}{\text{time}}}\\[6pt]&={\frac {\left({\dfrac {\text{torque}}{r}}\right)\cdot (r\cdot {\text{angular speed}}\cdot t)}{t}}\\[6pt]&={\text{torque}}\cdot {\text{angular speed}}.\end{aligned}}}$

The radius r and time t have dropped out of the equation. However, angular speed must be in radians, by the assumed direct relationship between linear speed and angular speed at the beginning of the derivation. If the rotational speed is measured in revolutions per unit of time, the linear speed and distance are increased proportionately by 2π in the above derivation to give:

${\displaystyle {\text{power}}={\text{torque}}\cdot 2\pi \cdot {\text{rotational speed}}.\,}$

If torque is in newton metres and rotational speed in revolutions per second, the above equation gives power in newton metres per second or watts. If Imperial units are used, and if torque is in pounds-force feet and rotational speed in revolutions per minute, the above equation gives power in foot pounds-force per minute. The horsepower form of the equation is then derived by applying the conversion factor 33,000 ft⋅lbf/min per horsepower:

${\displaystyle {\begin{aligned}{\text{power}}&={\text{torque}}\cdot 2\pi \cdot {\text{rotational speed}}\cdot {\frac {{\text{ft}}\cdot {\text{lbf}}}{\text{min}}}\cdot {\frac {\text{horsepower}}{33,000\cdot {\frac {{\text{ft}}\cdot {\text{lbf}}}{\text{min}}}}}\\[6pt]&\approx {\frac {{\text{torque}}\cdot {\text{RPM}}}{5,252}}\end{aligned}}}$

because ${\displaystyle 5252.113122\approx {\frac {33,000}{2\pi }}.\,}$

Principle of moments

The Principle of Moments, also known as Varignon's theorem (not to be confused with the geometrical theorem of the same name) states that the sum of torques due to several forces applied to a single point is equal to the torque due to the sum (resultant) of the forces. Mathematically, this follows from:

${\displaystyle (\mathbf {r} \times \mathbf {F} _{1})+(\mathbf {r} \times \mathbf {F} _{2})+\cdots =\mathbf {r} \times (\mathbf {F} _{1}+\mathbf {F} _{2}+\cdots ).}$

Torque multiplier

A torque multiplier is a gear box with reduction ratios greater than 1. The given torque at the input gets multiplied as per the reduction ratio and transmitted to the output, thereby achieving greater torque, but with reduced rotational speed.