Neutron star

From Wikipedia, the free encyclopedia

Play media

The size of a neutron star compared to Manhattan

Radiation from the pulsar PSR B1509-58, a rapidly spinning neutron star, makes nearby gas glow in X-rays (gold, from Chandra) and illuminates the rest of the nebula, here seen in infrared (blue and red, from WISE).

A neutron star is a type of stellar remnant that can result from the gravitational collapse of a massive star after a supernova. Neutron stars are the densest and smallest stars known to exist in the universe; with a radius of only about 12–13 km (7 mi), they can have a mass of about two times that of the Sun.

Neutron stars are composed almost entirely of neutrons, which are subatomic particles without net electrical charge and with slightly larger mass than protons. Neutron stars are very hot and are supported against further collapse by quantum degeneracy pressure due to the phenomenon described by the Pauli exclusion principle, which states that no two neutrons (or any other fermionic particles) can occupy the same place and quantum state simultaneously.

A typical neutron star has a mass between ~1.4 and about 3 solar masses (M_☉) with a surface temperature of ~6×10⁵ K.^{[1][2][3][4][a]} Neutron stars have overall densities of 3.7×10¹⁷ to 5.9×10¹⁷ kg/m³ (2.6×10¹⁴ to 4.1×10¹⁴ times the density of the Sun),^[b] which is comparable to the approximate density of an atomic nucleus of 3×10¹⁷ kg/m³.^[5] The neutron star's density varies from below 1×10⁹ kg/m³ in the crust – increasing with depth – to above 6×10¹⁷ or 8×10¹⁷ kg/m³ deeper inside (denser than an atomic nucleus).^[6] A normal-sized matchbox containing neutron star material would have a mass of approximately 5 billion tonnes or ~1 km³ of Earth rock.^{[citation needed]}

In general, compact stars of less than 1.44 M_☉ (the Chandrasekhar limit) are white dwarfs while compact stars weighing between that and 3 M_☉ (the Tolman–Oppenheimer–Volkoff limit) should be neutron stars. The maximum observed mass of neutron stars is about 2 M_☉. Compact stars with more than 10 M_☉ will overcome the neutron degeneracy pressure and gravitational collapse will usually occur to produce a black hole.^[7] The smallest observed mass of a black hole is about 5 M_☉. Between these, hypothetical intermediate-mass stars such as quark stars and electroweak stars have been proposed, but none have been shown to exist. The equations of state of matter at such high densities are not precisely known because of the theoretical and empirical difficulties.

Some neutron stars rotate very rapidly (up to 716 times a second,^[8][9] or approximately 43,000 revolutions per minute) and emit beams of electromagnetic radiation as pulsars. Indeed, the discovery of pulsars in 1967 first suggested that neutron stars exist. Gamma-ray bursts may be produced from rapidly rotating, high-mass stars that collapse to form a neutron star, or from the merger of binary neutron stars. There are thought to be on the order of 10⁸ neutron stars in the galaxy, but they can only be easily detected in certain instances, such as if they are a pulsar or part of a binary system. Non-rotating and non-accreting neutron stars are virtually undetectable; however, the Hubble Space Telescope has observed one thermally radiating neutron star, called RX J185635-3754.

Formation

Any main sequence star with an initial mass of around 10 M_☉ or above has the potential to become a neutron star. As the star evolves away from the main sequence, subsequent nuclear burning produces an iron-rich core. When all nuclear fuel in the core has been exhausted, the core must be supported by degeneracy pressure alone. Further deposits of material from shell burning cause the core to exceed the Chandrasekhar limit. Electron degeneracy pressure is overcome and the core collapses further, sending temperatures soaring to over 5×10⁹ K. At these temperatures, photodisintegration (the breaking up of iron nuclei into alpha particles by high- energy gamma rays) occurs. As the temperature climbs even higher, electrons and protons combine to form neutrons, releasing a flood of neutrinos. When densities reach nuclear density of 4×10¹⁷ kg/m³, neutron degeneracy pressure halts the contraction. The infalling outer atmosphere of the star is flung outwards, becoming a Type II or Type Ib supernova.
The remnant left is a neutron star. If it has a mass greater than about 5 M_☉, it collapses further to become a black hole. Other neutron stars are formed within close binaries.

As the core of a massive star is compressed during a Type II, Type Ib or Type Ic supernova, and collapses into a neutron star, it retains most of its angular momentum. Since it has only a tiny fraction of its parent's radius (and therefore its moment of inertia is sharply reduced), a neutron star is formed with very high rotation speed, and then gradually slows down. Neutron stars are known that have rotation periods from about 1.4 ms to 30 s. The neutron star's density also gives it very high surface gravity, with typical values ranging from 10¹² to 10¹³ m/s² (more than 10¹¹ times of that of Earth).^[4] One measure of such immense gravity is the fact that neutron stars have an escape velocity ranging from 100,000 km/s to 150,000 km/s, that is, from a third to half the speed of light. Matter falling onto the surface of a neutron star would be accelerated to tremendous speed by the star's gravity. The force of impact would likely destroy the object's component atoms, rendering all its matter identical, in most respects, to the rest of the star.

Properties

Gravitational light deflection at a neutron star. Due to relativistic light deflection more than half of the surface is visible (each chequered patch here represents 30 degrees by 30 degrees).^[10] In natural units, the mass of the depicted star is 1 and its radius 4, or twice its Schwarzschild radius.^[10]

The gravitational field at the star's surface is about 2×10¹¹ times stronger than on Earth. Such a strong gravitational field acts as a gravitational lens and bends the radiation emitted by the star such that parts of the normally invisible rear surface become visible.^[10] If the radius of the neutron star is 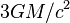or less, then the photons may be trapped in an orbit, thus making the whole surface of that neutron star visible, along with destabilizing orbits at that and less than that of the radius. A fraction of the mass of a star that collapses to form a neutron star is released in the supernova explosion from which it forms (from the law of mass-energy equivalence, E = mc²). The energy comes from the gravitational binding energy of a neutron star.

Neutron star relativistic equations of state provided by Jim Lattimer include a graph of radius vs. mass for various models.^[11] The most likely radii for a given neutron star mass are bracketed by models AP4 (smallest radius) and MS2 (largest radius). BE is the ratio of gravitational binding energy mass equivalent to observed neutron star gravitational mass of "M" kilograms with radius "R" meters,^[12]

      

Given current values

^[13]

and star masses "M" commonly reported as multiples of one solar mass,

then the relativistic fractional binding energy of a neutron star is

A 2 M_☉ neutron star would not be more compact than 10,970 meters radius (AP4 model). Its mass fraction gravitational binding energy would then be 0.187, −18.7% (exothermic). This is not near 0.6/2 = 0.3, −30%.

A neutron star is so dense that one teaspoon (5 milliliters) of its material would have a mass over 5.5×10¹² kg (that is 1100 tonnes per 1 nanolitre), about 900 times the mass of the Great Pyramid of Giza.^[c] Hence, the gravitational force of a typical neutron star is such that if an object were to fall from a height of one meter, it would only take one microsecond to hit the surface of the neutron star, and would do so at around 2000 kilometers per second, or 7.2 million kilometers per hour.^[14]

The temperature inside a newly formed neutron star is from around 10¹¹ to 10¹² kelvin.^[6] However, the huge number of neutrinos it emits carry away so much energy that the temperature falls within a few years to around 10⁶ kelvin.^[6] Even at 1 million kelvin, most of the light generated by a neutron star is in X-rays.

The pressure increases from 3×10³³ to 1.6×10³⁵ Pa from the inner crust to the center.^[15]
The equation of state for a neutron star is still not known. It is assumed that it differs significantly from that of a white dwarf, whose EOS is that of a degenerate gas which can be described in close agreement with special relativity. However, with a neutron star the increased effects of general relativity can no longer be ignored. Several EOS have been proposed (FPS, UU, APR, L, SLy, and others) and current research is still attempting to constrain the theories to make predictions of neutron star matter.^[4][16] This means that the relation between density and mass is not fully known, and this causes uncertainties in radius estimates. For example, a 1.5 M_☉ neutron star could have a radius of 10.7, 11.1, 12.1 or 15.1 kilometres (for EOS FPS, UU, APR or L respectively).^[16]

Structure

Cross-section of neutron star. Densities are in terms of ρ₀ the saturation nuclear matter density, where nucleons begin to touch.

Current understanding of the structure of neutron stars is defined by existing mathematical models, but it might be possible to infer through studies of neutron-star oscillations. Similar to asteroseismology for ordinary stars, the inner structure might be derived by analyzing observed frequency spectra of stellar oscillations.^[4]

On the basis of current models, the matter at the surface of a neutron star is composed of ordinary atomic nuclei crushed into a solid lattice with a sea of electrons flowing through the gaps between them. It is possible that the nuclei at the surface are iron, due to iron's high binding energy per nucleon.^[17] It is also possible that heavy element cores, such as iron, simply sink beneath the surface, leaving only light nuclei like helium and hydrogen cores.^[17] If the surface temperature exceeds 10⁶ kelvin (as in the case of a young pulsar), the surface should be fluid instead of the solid phase observed in cooler neutron stars (temperature <10 sup="">6

kelvin).^[17]
The "atmosphere" of the star is hypothesized to be at most several micrometers thick, and its dynamic is fully controlled by the star's magnetic field. Below the atmosphere one encounters a solid "crust". This crust is extremely hard and very smooth (with maximum surface irregularities of ~5 mm), because of the extreme gravitational field.^[18] The expected hierarchy of phases of nuclear matter in the inner crust has been characterized as nuclear pasta.^[19]

Proceeding inward, one encounters nuclei with ever increasing numbers of neutrons; such nuclei would decay quickly on Earth, but are kept stable by tremendous pressures. As this process continues at increasing depths, neutron drip becomes overwhelming, and the concentration of free neutrons increases rapidly. In this region, there are nuclei, free electrons, and free neutrons. The nuclei become increasingly small (gravity and pressure overwhelming the strong force) until the core is reached, by definition the point where they disappear altogether.

The composition of the superdense matter in the core remains uncertain. One model describes the core as superfluid neutron-degenerate matter (mostly neutrons, with some protons and electrons). More exotic forms of matter are possible, including degenerate strange matter (containing strange quarks in addition to up and down quarks), matter containing high-energy pions and kaons in addition to neutrons,^[4] or ultra-dense quark-degenerate matter.

History of discoveries

The first direct observation of a neutron star in visible light. The neutron star is RX J185635-3754.

In 1934, Walter Baade and Fritz Zwicky proposed the existence of the neutron star,^[20][d] only a year after the discovery of the neutron by Sir James Chadwick.^[23] In seeking an explanation for the origin of a supernova, they tentatively proposed that in supernova explosions ordinary stars are turned into stars that consist of extremely closely packed neutrons that they called neutron stars. Baade and Zwicky correctly proposed at that time that the release of the gravitational binding energy of the neutron stars powers the supernova: "In the supernova process, mass in bulk is annihilated". Neutron stars were thought to be too faint to be detectable and little work was done on them until November 1967, when Franco Pacini (1939–2012) pointed out that if the neutron stars were spinning and had large magnetic fields, then electromagnetic waves would be emitted. Unbeknown to him, radio astronomer Antony Hewish and his research assistant Jocelyn Bell at Cambridge were shortly to detect radio pulses from stars that are now believed to be highly magnetized, rapidly spinning neutron stars, known as pulsars.

In 1965, Antony Hewish and Samuel Okoye discovered "an unusual source of high radio brightness temperature in the Crab Nebula".^[24] This source turned out to be the Crab Nebula neutron star that resulted from the great supernova of 1054.

In 1967, Iosif Shklovsky examined the X-ray and optical observations of Scorpius X-1 and correctly concluded that the radiation comes from a neutron star at the stage of accretion.^[25]

In 1967, Jocelyn Bell and Antony Hewish discovered regular radio pulses from CP 1919. This pulsar was later interpreted as an isolated, rotating neutron star. The energy source of the pulsar is the rotational energy of the neutron star. The majority of known neutron stars (about 2000, as of 2010) have been discovered as pulsars, emitting regular radio pulses.

In 1971, Riccardo Giacconi, Herbert Gursky, Ed Kellogg, R. Levinson, E. Schreier, and H. Tananbaum discovered 4.8 second pulsations in an X-ray source in the constellation Centaurus, Cen X-3. They interpreted this as resulting from a rotating hot neutron star. The energy source is gravitational and results from a rain of gas falling onto the surface of the neutron star from a companion star or the interstellar medium.

In 1974, Antony Hewish was awarded the Nobel Prize in Physics "for his decisive role in the discovery of pulsars" without Jocelyn Bell who shared in the discovery.

In 1974, Joseph Taylor and Russell Hulse discovered the first binary pulsar, PSR B1913+16, which consists of two neutron stars (one seen as a pulsar) orbiting around their center of mass. Einstein's general theory of relativity predicts that massive objects in short binary orbits should emit gravitational waves, and thus that their orbit should decay with time. This was indeed observed, precisely as general relativity predicts, and in 1993, Taylor and Hulse were awarded the Nobel Prize in Physics for this discovery.

In 1982, Don Backer and colleagues discovered the first millisecond pulsar, PSR B1937+21. This objects spins 642 times per second, a value that placed fundamental constraints on the mass and radius of neutron stars. Many millisecond pulsars were later discovered, but PSR B1937+12 remained the fastest-spinning known pulsar for 24 years, until PSR J1748-2446ad was discovered.

In 2003, Marta Burgay and colleagues discovered the first double neutron star system where both components are detectable as pulsars, PSR J0737-3039. The discovery of this system allows a total of 5 different tests of general relativity, some of these with unprecedented precision.

In 2010, Paul Demorest and colleagues measured the mass of the millisecond pulsar PSR J1614–2230 to be 1.97±0.04 M_☉, using Shapiro delay.^[26] This was substantially higher than any previously measured neutron star mass (1.67 M_☉, see PSR J1903+0327), and places strong constraints on the interior composition of neutron stars.
In 2013, John Antoniadis and colleagues measured the mass of PSR J0348+0432 to be 2.01±0.04 M_☉, using white dwarf spectroscopy.^[27] This confirmed the existence of such massive stars using a different method. Furthermore, this allowed, for the first time, a test of general relativity using such a massive neutron star.

Rotation

Neutron stars rotate extremely rapidly after their creation due to the conservation of angular momentum; like spinning ice skaters pulling in their arms, the slow rotation of the original star's core speeds up as it shrinks. A newborn neutron star can rotate several times a second; sometimes, the neutron star absorbs orbiting matter from a companion star, increasing the rotation to several hundred times per second, reshaping the neutron star into an oblate spheroid.

Over time, neutron stars slow down (spin down) because their rotating magnetic fields radiate energy; older neutron stars may take several seconds for each revolution.

The rate at which a neutron star slows its rotation is usually constant and very small: the observed rates of decline are between 10⁻¹⁰ and 10⁻²¹ seconds for each rotation. Therefore, for a typical slow down rate of 10⁻¹⁵ seconds per rotation, a neutron star now rotating in 1 second will rotate in 1.000003 seconds after a century, or 1.03 seconds after 1 million years.

NASA artist's conception of a "starquake", or "stellar quake".

Sometimes a neutron star will spin up or undergo a glitch, a sudden small increase of its rotation speed. Glitches are thought to be the effect of a starquake — as the rotation of the star slows down, the shape becomes more spherical. Due to the stiffness of the "neutron" crust, this happens as discrete events when the crust ruptures, similar to tectonic earthquakes. After the starquake, the star will have a smaller equatorial radius, and since angular momentum is conserved, rotational speed increases. Recent work, however, suggests that a starquake would not release sufficient energy for a neutron star glitch; it has been suggested that glitches may instead be caused by transitions of vortices in the superfluid core of the star from one metastable energy state to a lower one.^[28]

Neutron stars have been observed to "pulse" radio and x-ray emissions believed to be caused by particle acceleration near the magnetic poles, which need not be aligned with the rotation axis of the star. Through mechanisms not yet entirely understood, these particles produce coherent beams of radio emission. External viewers see these beams as pulses of radiation whenever the magnetic pole sweeps past the line of sight. The pulses come at the same rate as the rotation of the neutron star, and thus, appear periodic. Neutron stars which emit such pulses are called pulsars.

The most rapidly rotating neutron star currently known, PSR J1748-2446ad, rotates at 716 rotations per second.^[29] A recent paper reported the detection of an X-ray burst oscillation (an indirect measure of spin) at 1122 Hz from the neutron star XTE J1739-285.^[30] However, at present, this signal has only been seen once, and should be regarded as tentative until confirmed in another burst from this star.

Population and distances

At present, there are about 2000 known neutron stars in the Milky Way and the Magellanic Clouds, the majority of which have been detected as radio pulsars. Neutron stars are mostly concentrated along the disk of the Milky Way although the spread perpendicular to the disk is large because the supernova explosion process can impart high speeds (400 km/s) to the newly created neutron star.

Some of the closest neutron stars are RX J1856.5-3754 about 400 light years away and PSR J0108-1431 at about 424 light years.^[31] RX J1856.5-3754 is a member of a close group of neutron stars called The Magnificent Seven. Another nearby neutron star that was detected transiting the backdrop of the constellation Ursa Minor has been nicknamed Calvera by its Canadian and American discoverers, after the villain in the 1960 film The Magnificent Seven. This rapidly moving object was discovered using the ROSAT/Bright Source Catalog.

Binary neutron stars

About 5% of all known neutron stars are members of a binary system. The formation and evolution scenario of binary neutron stars is a rather exotic and complicated process.^[32] The companion stars may be either ordinary stars, white dwarfs or other neutron stars. According to modern theories of binary evolution it is expected that neutron stars also exist in binary systems with black hole companions. Such binaries are expected to be prime sources for emitting gravitational waves. Neutron stars in binary systems often emit X-rays which is caused by the heating of material (gas) accreted from the companion star. Material from the outer layers of a (bloated) companion star is sucked towards the neutron star as a result of its very strong gravitational field. As a result of this process binary neutron stars may also coalesce into black holes if the accretion of mass takes place under extreme conditions.^[33] It has been proposed that coalescence of binaries consisting of two neutron stars may be responsible for producing short gamma-ray bursts. Such events may also be responsible for creating all chemical elements beyond iron,^[34] as opposed to the supernova nucleosynthesis theory.

Subtypes

• Neutron star
  • Protoneutron star (PNS), theorized.^[35]
  • Radio-quiet neutron stars
  • Radio loud neutron star
    • Single pulsars–general term for neutron stars that emit directed pulses of radiation towards us at regular intervals (due to their strong magnetic fields).
      • Rotation-powered pulsar ("radio pulsar")
        • Magnetar–a neutron star with an extremely strong magnetic field (1000 times more than a regular neutron star), and long rotation periods (5 to 12 seconds).
          • Soft gamma repeater (SGR)
          • Anomalous X-ray pulsar (AXP)
    • Binary pulsars
      • Low-mass X-ray binaries (LMXB)
      • Intermediate-mass X-ray binaries (IMXB)
      • High-mass X-ray binaries (HMXB)
      • Accretion-powered pulsar ("X-ray pulsar")
        • X-ray burster–a neutron star with a low mass binary companion from which matter is accreted resulting in irregular bursts of energy from the surface of the neutron star.
        • Millisecond pulsar (MSP) ("recycled pulsar")
          • Sub-millisecond pulsar^[36]
  • Exotic star
    • Quark star–currently a hypothetical type of neutron star composed of quark matter, or strange matter. As of 2008, there are three candidates.
    • Electroweak star–currently a hypothetical type of extremely heavy neutron star, in which the quarks are converted to leptons through the electroweak force, but the gravitational collapse of the star is prevented by radiation pressure. As of 2010, there is no evidence for their existence.
    • Preon star–currently a hypothetical type of neutron star composed of preon matter. As of 2008, there is no evidence for the existence of preons.

Giant nucleus

A neutron star has some of the properties of an atomic nucleus, including density (within an order of magnitude) and being composed of nucleons. In popular scientific writing, neutron stars are therefore sometimes described as giant nuclei. However, in other respects, neutron stars and atomic nuclei are quite different. In particular, a nucleus is held together by the strong interaction, whereas a neutron star is held together by gravity, and thus the density and structure of neutron stars is more variable. It is generally more useful to consider such objects as stars.

Examples of neutron stars

• PSR J0108-1431 – closest neutron star
• LGM-1 – the first recognized radio-pulsar
• PSR B1257+12 – the first neutron star discovered with planets (a millisecond pulsar)
• SWIFT J1756.9-2508 – a millisecond pulsar with a stellar-type companion with planetary range mass (below brown dwarf)
• PSR B1509-58 source of the "Hand of God" photo shot by the Chandra X-ray Observatory.
• PSR J0348+0432 - the most massive neutron star with a well-constrained mass, .

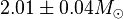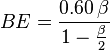
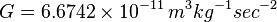
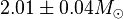Solar Roadways

From Wikipedia, the free encyclopedia

Solar Roadways Inc

Type	Startup
Founded	2006 (2006)
Founder	Scott Brusaw Julie Brusaw
Headquarters	721 Pine Street, Sandpoint, Idaho 83864, United States [1]
Website	solarroadways.com

Solar Roadways Incorporated is a startup company based in Sandpoint, Idaho, that is developing solar powered road panels to form a smart highway. Their technology combines a transparent driving surface with underlying solar cells, electronics and sensors to act as a solar array with programmable capability. Solar Roadways Inc is working to develop and commercially produce road panels which are made from recycled materials and incorporate photovoltaic cells. ^[2]

History

In 2006, the company was founded by Scott and Julie Brusaw, with Scott as President and CEO. The company envisioned replacing asphalt surfaces with structurally-engineered solar panels capable of withstanding vehicular traffic."^[3] The proposed system would require the development of strong, transparent, and self-cleaning glass that has the necessary traction and impact-resistance properties. ^[4]

In 2009, Solar Roadways received a $100,000 Small Business Innovation Research (SBIR) grant from the Department of Transportation (DOT) for Phase I to develop and build a solar parking lot.^[5] In 2011, Solar Roadways received $750,000 SBIR grant from the DOT for Phase II to develop and build a solar parking lot.^[6] The DOT distinguishes the technology proposed by Solar Roadways Inc. as "Solar Power Applications in the Roadway," as compared to a number of other solar technologies categorized by the DOT as "Solar Applications along the Roadway."^[7] From SBIR grant money, Solar Roadways has built a 12-by-36-foot (3.7 by 11.0 m) parking lot covered with hexagonal glass-covered solar panels sitting on top of a concrete base, which are heated to help remove snow and ice, and also include LEDs to display messages. The hexagonal shape allows for better coverage on curves and hills. According to the Brusaws, the panels can sustain a 250,000 lb (110,000 kg) load. ^[8]

In April 2014, Solar Roadways started a crowdfunding drive at Indiegogo to raise money so they can get the product into production. In May, it was extended by another 30 days. The campaign raised 2.2 million dollars, exceeding its target of 1 million dollars.^[9] The drive became Indiegogo’s most popular campaign ever in terms of the number of backers it has attracted.^[10] The success was attributed in part to a Tweet made by George Takei, who played Sulu on Star Trek, due to his more than 8 million followers.^[11][12] One of the Brusaws’ videos went viral, with nearly 15 million views as of June 2014.^[12]

In 2014, doubt was expressed regarding the political feasibility of the project on a national scale by Jonathan Levine, a professor of urban planning at the University of Michigan. He suggested, however, that a single town might be able to deploy the concept in a limited test case such as a parking lot.^[13]

List of awards and honors

• 2009 EE Times Annual Creativity in Electronics (ACE) Awards "Best Enabler Award for Green Engineering" category finalist.^[14]
• 2010 EE Times Annual Creativity in Electronics (ACE) Awards "Most Promising Renewable Energy Award" category finalist.^[15]
• 2010 General Electric Ecoimagination Community Award of $50,000.^[16]
• 2013 World Technology Award finalist.^[17]
• 2014 Popular Science. One of 7 "Best of What's New" Engineering category.^[18]

Numerical cognition

From Wikipedia, the free encyclopedia

Numerical cognition is a subdiscipline of cognitive science that studies the cognitive, developmental and neural bases of numbers and mathematics. As with many cognitive science endeavors, this is a highly interdisciplinary topic, and includes researchers in cognitive psychology, developmental psychology, neuroscience and cognitive linguistics. This discipline, although it may interact with questions in the philosophy of mathematics is primarily concerned with empirical questions.

Topics included in the domain of numerical cognition include:

• How do non-human animals process numerosity?
• How do infants acquire an understanding of numbers (and how much is inborn)?
• How do humans associate linguistic symbols with numerical quantities?
• How do these capacities underlie our ability to perform complex calculations?
• What are the neural bases of these abilities, both in humans and in non-humans?
• What metaphorical capacities and processes allow us to extend our numerical understanding into complex domains such as the concept of infinity, the infinitesimal or the concept of the limit in calculus?

Comparative studies

A variety of research has demonstrated that non-human animals, including rats, lions and various species of primates have an approximate sense of number (referred to as "numerosity") (for a review, see Dehaene 1997). For example, when a rat is trained to press a bar 8 or 16 times to receive a food reward, the number of bar presses will approximate a Gaussian or Normal distribution with peak around 8 or 16 bar presses. When rats are more hungry, their bar pressing behavior is more rapid, so by showing that the peak number of bar presses is the same for either well-fed or hungry rats, it is possible to disentangle time and number of bar presses.

Similarly, researchers have set up hidden speakers in the African savannah to test natural (untrained) behavior in lions (McComb, Packer & Pusey 1994). These speakers can play a number of lion calls, from 1 to 5. If a single lioness hears, for example, three calls from unknown lions, she will leave, while if she is with four of her sisters, they will go and explore. This suggests that not only can lions tell when they are "outnumbered" but that they can do this on the basis of signals from different sensory modalities, suggesting that numerosity is a multisensory concept.

Developmental studies

Developmental psychology studies have shown that human infants, like non-human animals, have an approximate sense of number. For example, in one study, infants were repeatedly presented with arrays of (in one block) 16 dots. Careful controls were in place to eliminate information from "non-numerical" parameters such as total surface area, luminance, circumference, and so on. After the infants had been presented with many displays containing 16 items, they habituated, or stopped looking as long at the display. Infants were then presented with a display containing 8 items, and they looked longer at the novel display.

Because of the numerous controls that were in place to rule out non-numerical factors, the experimenters infer that six-month-old infants are sensitive to differences between 8 and 16. Subsequent experiments, using similar methodologies showed that 6-month-old infants can discriminate numbers differing by a 2:1 ratio (8 vs. 16 or 16 vs. 32) but not by a 3:2 ratio (8 vs. 12 or 16 vs. 24). However, 10-month-old infants succeed both at the 2:1 and the 3:2 ratio, suggesting an increased sensitivity to numerosity differences with age (for a review of this literature see Feigenson, Dehaene & Spelke 2004).

In another series of studies, Karen Wynn showed that infants as young as five months are able to do very simple additions (e.g., 1 + 1 = 2) and subtractions (3 - 1 = 2). To demonstrate this, Wynn used a "violation of expectation" paradigm, in which infants were shown (for example) one Mickey Mouse doll going behind a screen, followed by another. If, when the screen was lowered, infants were presented with only one Mickey (the "impossible event") they looked longer than if they were shown two Mickeys (the "possible" event). Further studies by Karen Wynn and Koleen McCrink found that although infants' ability to compute exact outcomes only holds over small numbers, infants can compute approximate outcomes of larger addition and subtraction events (e.g., "5+5" and "10-5" events).

There is debate about how much these infant systems actually contain in terms of number concepts, harkening to the classic nature versus nurture debate. Gelman & Gallistel 1978 suggested that a child innately has the concept of natural number, and only has to map this onto the words used in her language. Carey 2004, Carey 2009 disagreed, saying that these systems can only encode large numbers in an approximate way, where language-based natural numbers can be exact. One promising approach is to see if cultures that lack number words can deal with natural numbers. The results so far are mixed (e.g., Pica et al. 2004); Butterworth & Reeve 2008, Butterworth, Reeve & Lloyd 2008.

Neuroimaging and neurophysiological studies

Human neuroimaging studies have demonstrated that regions of the parietal lobe, including the intraparietal sulcus (IPS) and the inferior parietal lobule (IPL) are activated when subjects are asked to perform calculation tasks. Based on both human neuroimaging and neuropsychology, Stanislas Dehaene and colleagues have suggested that these two parietal structures play complementary roles. The IPS is thought to house the circuitry that is fundamentally involved in numerical estimation (Piazza et al. 2004), number comparison (Pinel et al. 2001; Pinel et al. 2004) and on-line calculation (often tested with subtraction) while the IPL is thought to be involved in overlearned tasks, such as multiplication (see Dehaene 1997). Thus, a patient with a lesion to the IPL may be able to subtract, but not multiply, and vice versa for a patient with a lesion to the IPS. In addition to these parietal regions, regions of the frontal lobe are also active in calculation tasks. These activations overlap with regions involved in language processing such as Broca's area and regions involved in working memory and attention. Future research will be needed to disentangle the complex influences of language, working memory and attention on numerical processes.
Single-unit neurophysiology in monkeys has also found neurons in the frontal cortex and in the intraparietal sulcus that respond to numbers. Andreas Nieder (Nieder 2005; Nieder, Freedman & Miller 2002; Nieder & Miller 2004) trained monkeys to perform a "delayed match-to-sample" task. For example, a monkey might be presented with a field of four dots, and is required to keep that in memory after the display is taken away. Then, after a delay period of several seconds, a second display is presented. If the number on the second display match that from the first, the monkey has to release a lever. If it is different, the monkey has to hold the lever. Neural activity recorded during the delay period showed that neurons in the intraparietal sulcus and the frontal cortex had a "preferred numerosity", exactly as predicted by behavioral studies. That is, a certain number might fire strongly for four, but less strongly for three or five, and even less for two or six. Thus, we say that these neurons were "tuned" for specific quantities. Note that these neuronal responses followed Weber's law, as has been demonstrated for other sensory dimensions, and consistent with the ratio dependence observed for non-human animals' and infants' numerical behavior (Nieder & Miller 2003).

Relations between number and other cognitive processes

There is evidence that numerical cognition is intimately related to other aspects of thought – particularly spatial cognition.^[1] One line of evidence comes from studies performed on number-form synaesthetes.^[2] Such individuals report that numbers are mentally represented with a particular spatial layout; others experience numbers as perceivable objects that can be visually manipulated to facilitate calculation. Behavioral studies further reinforce the connection between numerical and spatial cognition. For instance, participants respond quicker to larger numbers if they are responding on the right side of space, and quicker to smaller numbers when on the left—the so-called "Spatial-Numerical Association of Response Codes" or SNARC effect.^[3] This effect varies across culture and context,^[4] however, and some research has even begun to question whether the SNARC reflects an inherent number-space association,^[5] instead invoking strategic problem solving or a more general cognitive mechanism like conceptual metaphor.^[6][7] Moreover, neuroimaging studies reveal that the association between number and space also shows up in brain activity. Regions of the parietal cortex, for instance, show shared activation for both spatial and numerical processing.^[8] These various lines of research suggest a strong, but flexible, connection between numerical and spatial cognition.

Modification of the usual decimal representation was advocated by John Colson. The sense of complementation, missing in the usual decimal system, is expressed by signed-digit representation.

Ethnolinguistic variance

The numeracy of indigenous peoples is studied to identify universal aspects of numerical cognition in humans. Notable examples include the Pirahã people who have no words for specific numbers and the Munduruku people who only have number words up to five. Pirahã adults are unable to mark an exact number of tallies for a pile of nuts containing fewer than ten items. Anthropologist Napoleon Chagnon spent several decades studying the Yanomami in the field. He concluded that they have no need for counting in their everyday lives. Their hunters keep track of individual arrows with the same mental faculties that they use to recognize their family members. There are no known hunter-gatherer cultures that have a counting system in their language. The mental and lingual capabilities for numeracy are tied to the development of agriculture and with it large numbers of indistinguishable items.^[9]

Numeracy

From Wikipedia, the free encyclopedia

Children in Laos have fun as they improve numeracy with "Number Bingo." They roll three dice, construct an equation from the numbers to produce a new number, then cover that number on the board, trying to get 4 in a row.

Numeracy is the ability to reason and to apply simple numerical concepts.^[1] Basic numeracy skills consist of comprehending fundamental arithmetics like addition, subtraction, multiplication, and division. For example, if one can understand simple mathematical equations such as, 2 + 2 = 4, then one would be considered possessing at least basic numeric knowledge. Substantial aspects of numeracy also include number sense, operation sense, computation, measurement, geometry, probability and statistics. A numerically literate person can manage and respond to the mathematical demands of life.^[2] By contrast, innumeracy (the lack of numeracy) can have a negative impact. Numeracy has an influence on career professions, literacy, and risk perception towards health decisions. Low numeracy distorts risk perception towards health decisions^[3] and may negatively affect economic choices.^[4][5] "Greater numeracy has been associated with reduced susceptibility to framing effects, less influence of nonnumerical information such as mood states, and greater sensitivity to different levels of numerical risk".^[6]

§Representation of numbers

Humans have evolved to mentally represent numbers in two major ways from observation (not formal math).^[7] These representations are often thought to be innate^[8] (see Numerical cognition), to be shared across human cultures,^[9] to be common to multiple species,^[10] and not to be the result of individual learning or cultural transmission. They are:

1. Approximate representation of numerical magnitude, and
2. Precise representation of the quantity of individual items.

Approximate representations of numerical magnitude imply that one can relatively estimate and comprehend an amount if the number is large (see Approximate number system). For example, one experiment showed children and adults arrays of many dots.^[7] After briefly observinging them, both groups could accurately estimate the approximate number of dots. However, distinguishing differences between large numbers of dots proved to be more challenging.^[7]

Precise representations of distinct individuals demonstrate that people are more accurate in estimating amounts and distinguishing differences when the numbers are relatively small (see Subitizing).^[7] For example, in one experiment, an experimenter presented an infant with two piles of crackers, one with two crackers the other with three. The experimenter then covered each pile with a cup. When allowed to choose a cup, the infant always chose the cup with more crackers because the infant could distinguish the difference.^[7]

Both systems—approximate representation of magnitude and precise representation quantity of individual items—have limited power. For example, neither allows representations of fractions or negative numbers. More complex representations require education. However, achievement in school mathematics correlates with an individual's unlearned approximate number sense).^[11]

§Definitions and assessment

Fundamental (or rudimentary) numeracy skills include understanding of the real number line, time, measurement, and estimation.^[3] Fundamental skills include basic skills (the ability to identify and understand numbers) and computational skills (the ability to perform simple arithmetical operations and compare numerical magnitudes).

More sophisticated numeracy skills include understanding of ratio concepts (notably fractions, proportions, percentages, and probabilities), and knowing when and how to perform multistep operations.^[3] Two categories of skills are included at the higher levels: the analytical skills (the ability to understand numerical information, such as required to interpret graphs and charts) and the statistical skills (the ability to apply higher probabilistic and statistical computation, such as conditional probabilities).

A variety of tests have been developed for assessing numeracy and health numeracy. ^{[3][6][12][13] [14] [15] [16]}

§Childhood influences

The first couple of years of childhood are considered to be a vital part of life for the development of numeracy and literacy.^[17] There are many components that play key roles in the development of numeracy at a young age, such as Socioeconomic Status (SES), parenting, Home Learning Environment (HLE), and age.^[17]

§Socioeconomic status

Children who are brought up in families with high SES tend to be more engaged in developmentally enhancing activities.^[17] These children are more likely to develop the necessary abilities to learn and to become more motivated to learn.^[17] More specifically, a mother's education level is considered to have an effect on the child's ability to achieve in numeracy. That is, mothers with a high level of education will tend to have children who succeed more in numeracy.^[17]

§Parenting

Parents are suggested to collaborate with their child in simple learning exercises, such as reading a book, painting, drawing, and playing with numbers. On a more expressive note, the act of using complex language, being more responsive towards the child, and establishing warm interactions are recommended to parents with the confirmation of positive numeracy outcomes.^[17] When discussing beneficial parenting behaviors, a feedback loop is formed because pleased parents are more willing to interact with their child, which in essence promotes better development in the child.^[17]

§Home-learning environment

Along with parenting and SES, a strong home-learning environment increases the likelihood of the child being prepared for comprehending complex mathematical schooling.^[18] For example, if a child is influenced by many learning activities in the household, such as puzzles, coloring books, mazes, or books with picture riddles, then they will be more prepared to face school activities.^[18]

§Age

Age is accounted for when discussing the development of numeracy in children.^[18] Children under the age of 5 have the best opportunity to absorb basic numeracy skills.^[18] After the age of 7, achievement of basic numeracy skills become less influential.^[18] For example, a study was conducted to compare the reading and mathematic abilities between children, ages 5 and 7, each in three different mental capacity groups (underachieving, average, and overachieving). The differences in the amount of knowledge retained were greater between the three different groups at age 5, than between the groups at age 7. This reveals that the younger you are the greater chance you have to retain more information, like numeracy.

§Literacy

There seems to be a relationship between literacy and numeracy,^{[19] [20]} which can be seen in young children. Depending on the level of literacy or numeracy at a young age, one can predict the growth of literacy and/ or numeracy skills in future development.^[21] There is some evidence that humans may have an inborn sense of number. In one study for example, five-month-old infants were shown two dolls, which were then hidden with a screen. The babies saw the experimenter pull one doll from behind the screen. Without the child's knowledge, a second experimenter could remove, or add dolls, unseen behind the screen. When the screen was removed, the infants showed more surprise at an unexpected number (for example, if there were still two dolls). Some researchers have concluded that the babies were able to count, although others doubt this and claim the infants noticed surface area rather than number.^[22]

§Employment

Numeracy has a huge impact on employment.^[23] In a work environment, numeracy can be a controlling factor affecting career achievements and failures.^[23] Many professions require individuals to have a well-developed sense of numeracy, for example: mathematician, physicist, accountant, actuary, Risk Analyst, financial analyst, engineer, and architect. Even outside these specialized areas, the lack of proper numeracy skills can reduce employment opportunities and promotions, resulting in unskilled manual careers, low-paying jobs, and even unemployment.^[24]
For example, carpenters and interior designers need to be able to measure, use fractions, and handle budgets.^[25] Another example pertaining to numeracy influencing employment was demonstrated at the Poynter Institute. The Poynter Institute has recently included numeracy as one of the skills required by competent journalists. Max Frankel, former executive editor of the New York Times, argues that "deploying numbers skillfully is as important to communication as deploying verbs". Unfortunately, it is evident that journalists often show poor numeracy skills. In a study by the Society of Professional Journalists, 58% of job applicants interviewed by broadcast news directors lacked an adequate understanding of statistical materials.^[26]

With regards to assessing applicants for an employment position, psychometric numerical reasoning tests have been created by occupational psychologists, who are involved in the study of numeracy. These psychometric numerical reasoning tests are used to assess an applicants' ability to comprehend and apply numbers. These tests are sometimes administered with a time limit, resulting in the need for the test-taker to think quickly and concisely. Research has shown that these tests are very useful in evaluating potential applicants because they do not allow the applicants to prepare for the test, unlike interview questions. This suggests that an applicant's results are reliable and accurate.^[27]

These psychometric numerical reasoning tests first became prevalent during the 1980s, following the pioneering work of psychologists, such as P. Kline. In 1986 P. Kline's published a book entitled, "A handbook of test construction: Introduction to psychometric design", which explained that psychometric testing could provide reliable and objective results. These findings could then be used to effectively assess a candidate's abilities in numeracy. In the future, psychometric numerical reasoning tests will continue to be used in employment assessments to fairly and accurately differentiate and evaluate possible employment applicants.

§Innumeracy and dyscalculia

Innumeracy is a neologism coined by an analogue with illiteracy. Innumeracy refers to a lack of ability to reason with numbers. The term innumeracy was coined by cognitive scientist Douglas Hofstadter. However, this term was popularized in 1989 by mathematician John Allen Paulos in his book entitled, Innumeracy: Mathematical Illiteracy and its Consequences.

Developmental dyscalculia refers to a persistent and specific impairment of basic numerical-arithmetical skills learning in the context of normal intelligence.

§Patterns and differences

The root cause of innumeracy varies. Innumeracy has been seen in those suffering from poor education and childhood deprivation of numeracy.^[28] Innumeracy is apparent in children during the transition of numerical skills obtained before schooling and the new skills taught in the education departments because of their memory capacity to comprehend the material.^[28] Patterns of innumeracy have also been observed depending on age, gender, and race.^[29] Older adults have been associated with lower numeracy skills than younger adults.^[29] Men have been identified to have higher numeracy skills than women.^[23] Some studies seem to indicate young people of African heritage tend to have lower numeracy skills.^[29] The Trends in International Mathematics and Science Study (TIMSS) in which children at fourth-grade (average 10 to 11 years) and eighth-grade (average 14 to 15 years) from 49 countries were tested on mathematical comprehension. The assessment included tests for number, algebra (also called patterns and relationships at fourth grade), measurement, geometry, and data. The latest study, in 2003 found that children from Singapore at both grade levels had the highest performance. Countries like Hong Kong SAR, Japan, and Taiwan also shared high levels of numeracy. The lowest scores were found in countries like South Africa, Ghana, and Saudi Arabia. Another finding showed a noticeable difference between boys and girls with some exceptions. For example, girls performed significantly better in Singapore, and boys performed significantly better in the United States.^[7]

§Theory

There is a theory that innumeracy is more common than illiteracy when dividing cognitive abilities into two separate categories. David C. Geary, a notable cognitive developmental and evolutionary psychologist from the University of Missouri, created the terms "biological primary abilities" and "biological secondary abilities".^[28] Biological primary abilities evolve over time and are necessary for survival. Such abilities include speaking a common language or knowledge of simple mathematics.^[28] Biological secondary abilities are attained through personal experiences and cultural customs, such as reading or high level mathematics learned through schooling.^[28] Literacy and numeracy are similar in the sense that they are both important skills used in life. However, they differ in the sorts of mental demands each makes. Literacy consists of acquiring vocabulary and grammatical sophistication, which seem to be more closely related to memorization, whereas numeracy involves manipulating concepts, such as in calculus or geometry, and builds from basic numeracy skills.^[28] This could be a potential explanation of the challenge of being numerate.^[28]

§Innumeracy and risk perception in health decision-making

Health numeracy has been defined as "the degree to which individuals have the capacity to access, process, interpret, communicate, and act on numerical, quantitative, graphical, biostatistical, and probabilistic health information needed to make effective health decisions".^[30] The concept of health numeracy is a component of the concept of health literacy. Health numeracy and health literacy can be thought of as the combination of skills needed for understanding risk and making good choices in health-related behavior.

Health numeracy requires basic numeracy but also more advanced analytical and statistical skills. For instance, health numeracy also requires the ability to understand probabilities or relative frequencies in various numerical and graphical formats, and to engage in Bayesian inference, while avoiding errors sometimes associated with Bayesian reasoning (see Base rate fallacy, Conservatism (Bayesian)). Health numeracy also requires understanding terms with definitions that are specific to the medical context. For instance, although 'survival' and 'mortality' are complementary in common usage, these terms are not complementary in medicine (see five-year survival rate).^[31][32] Innumeracy is also a very common problem when dealing with risk perception in health-related behavior; it is associated with patients, physicians, journalists and policymakers.^[29][32] Those who lack or have limited health numeracy skills run the risk of making poor health-related decisions because of an inaccurate perception of information.^[17] For example, if a patient has been diagnosed with breast cancer, being innumerate may hinder her ability to comprehend her physician's recommendations or even the severity of the health concern. One study found that people tended to overestimate their chances of survival or even to choose lower quality hospitals.^[23]
Innumeracy also makes it difficult or impossible for some patients to read medical graphs correctly.^[33] Some authors have distinguished graph literacy from numeracy.^[34] Indeed, many doctors exhibit innumeracy when attempting to explain a graph or statistics to a patient. Once again, a misunderstanding between a doctor and patient due to either the doctor, patient, or both being unable to comprehend numbers effectively could result in serious health consequences.

Different presentation formats of numerical information, for instance natural frequency icon arrays, have been evaluated to assist both low numeracy and high numeracy individuals.^{[29][35][36][37][38]}