Pulsed nuclear thermal rocket

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Pulsed_nuclear_thermal_rocket

 

A sequence for a stationary-pulsed-stationary maneuver for a pulsed thermal nuclear rocket. During the stationary mode (working at constant nominal power), the fuel temperature is always constant (solid black line), and the propellant is coming cold (blue dotted lines) heated in the chamber and exhausted in the nozzle (red dotted line). When amplification in thrust or specific impulse is required, the nuclear core is "switched on" to a pulsed mode. In this mode, the fuel in continuously quenched and instantaneously heated by the pulses. Once the requirements for high thrust and specific impulse are not required, the nuclear core is "switched on" to the initial stationary mode.

 

A pulsed nuclear thermal rocket is a type of nuclear thermal rocket (NTR) concept developed at the Polytechnic University of Catalonia, Spain and presented at the 2016 AIAA/SAE/ASEE Propulsion Conference for thrust and specific impulse (I_sp) amplification in a conventional nuclear thermal rocket.

The pulsed nuclear thermal rocket is a bimodal rocket able to work in a stationary (at constant nominal power as in a conventional NTR), and as well as a pulsed mode as a TRIGA-like reactor, making possible the production of high power and an intensive neutron flux in short time intervals. In contrast to nuclear reactors where velocities of the coolant are no larger than a few meter per second and thus, typical residence time is on seconds, however, in rockets chambers with subsonic velocities of the propellant around hundreds of meters per second, residence time are around ${\displaystyle 10^{-2}s}$ to :${\displaystyle 10^{-3}s}$ and then a long power pulse translates into an important gain in energy in comparison with the stationary mode. The gained energy -by pulsing the nuclear core, can be used for thrust amplification by increasing the propellant mass flow, or using the intensive neutron flux to produce a very high specific impulse amplification – even higher than the fission-fragment rocket, where in the pulsed rocket the final propellant temperature is only limited by the radiative cooling after the pulsation. 

Statement of the concept

A rough calculation for the energy gain by using a pulsed thermal nuclear rocket in comparison with the conventional stationary mode, is as follows. The energy stored into the fuel after a pulsation, is the sensible heat stored because the fuel temperature increase. This energy may be written as

${\displaystyle E_{\text{pulse}}=c_{f}M_{f}\Delta T}$

where:

${\displaystyle E_{\text{pulse}}}$ is the sensible heat stored after pulsation,

${\displaystyle c_{f}}$ is the fuel heat capacity,

${\displaystyle M_{\text{f}}}$ is the fuel mass,

${\displaystyle \Delta T}$ is the temperature increase between pulsations.

On the other hand, the energy generated in the stationary mode, i.e., when the nuclear core operates at nominal constant power is given by

${\displaystyle E_{\text{stationary}}=\chi _{l}lt}$

where:

${\displaystyle \chi _{l}}$ is the linear power of the fuel (power per length of fuel),

${\displaystyle l}$ is the length of the fuel,

${\displaystyle t}$ is the residence time of the propellant in the chamber.

Also, for the case of cylindrical geometries for the nuclear fuel we have

${\displaystyle M_{f}=\pi R_{f}^{2}l\rho _{f}}$

and the linear power given by 

${\displaystyle \chi _{l}=4\pi \kappa _{f}(T_{f}-T_{s})}$

Where:

${\displaystyle R_{f}}$ is the radius of the cylindrical fuel,

${\displaystyle \rho _{f}}$ the fuel density,

${\displaystyle \kappa _{f}}$ the fuel thermal conductivity,

${\displaystyle T_{f}}$ is the fuel temperature at the center line,

${\displaystyle T_{s}}$ is the surface or cladding temperature.

Therefore, the energy ratio between the pulsed mode and the stationary mode, ${\displaystyle N={\frac {E_{\text{pulse}}}{E_{\text{stationary}}}}}$ yields

${\displaystyle N={\frac {c_{f}\rho _{f}R_{f}^{2}}{4\pi \kappa _{f}(T_{f}-T_{s})}}\left[{\frac {\Delta T}{t}}\right]}$

Where the term inside the bracket, ${\displaystyle \left[{\frac {\Delta T}{t}}\right]}$ is the quenching rate. 

Typical average values of the parameters for common nuclear fuels as MOX fuel or uranium dioxide are: heat capacities, thermal conductivity and densities around ${\displaystyle c_{f}\simeq 300J/(mol\cdot K)}$,${\displaystyle \kappa _{f}\simeq 6W/(K\cdot m^{2})}$ and ${\displaystyle \rho _{f}\simeq 10^{4}kg/(m^{3})}$, respectively., with radius close to ${\displaystyle R_{f}\simeq 10^{-2}m}$, and the temperature drop between the center line and the cladding on ${\displaystyle T_{f}-T_{s}=600K}$ or less (which result in linear power on ${\displaystyle \chi _{l}\simeq 45000W/m)}$. With these values the gain in energy is approximately given by:

${\displaystyle N\simeq 6\times 10^{-3}\left[{\frac {\Delta T}{t}}\right]}$

where ${\displaystyle \left[{\frac {\Delta T}{t}}\right]}$ is given in ${\displaystyle K/s}$. Because the residence time of the propellant in the chamber is on ${\displaystyle 10^{-3}s}$ to ${\displaystyle 10^{-2}s}$ considering subsonic velocities of the propellant of hundreds of meters per second and meter chambers, then, with temperatures differences on ${\displaystyle \Delta T\simeq 10^{3}K}$ or quenching rates on ${\displaystyle \left[{\frac {\Delta T}{t}}\right]\simeq 10^{6}K/s}$ energy amplification by pulsing the core could be thousands times larger than the stationary mode. More rigorous calculations considering the transient heat transfer theory shows energy gains around hundreds or thousands times, i.e., ${\displaystyle 10^{2}\leq N\leq 10^{3}}$. 

Quenching rates on ${\displaystyle \left[{\frac {\Delta T}{t}}\right]\geq 10^{6}K/s}$ are typical in the technology for production of amorphous metal, where extremely rapid cooling in the order of ${\displaystyle 10^{6}K/s\leq \left[{\frac {\Delta T}{t}}\right]\leq 10^{7}K/s}$ are required.

Direct thrust amplification

The most direct way to harness the amplified energy by pulsing the nuclear core is by increasing the thrust via increasing the propellant mass flow. 

Increasing the thrust in the stationary mode -where power is fixed by thermodynamic constraints, is only possible by sacrificing exhaust velocity. In fact, the power is given by

${\displaystyle P={\frac {1}{2}}Fv_{\text{e}}}$

where ${\displaystyle P}$ is the power, ${\displaystyle F}$ is the thrust and ${\displaystyle v_{\text{e}}}$ the exhaust velocity. On the other hand, thrust is given by

${\displaystyle F={\dot {m}}_{\text{p}}v_{\text{e}}}$

where ${\displaystyle {\dot {m}}_{\text{p}}}$ is the propellant mass flow. Thus, if it is desired to increase the thrust, say, n-times in the stationary mode, it will be necessary to increase ${\displaystyle n^{2}}$-times the propellant mass flow, and decreasing ${\displaystyle {\frac {1}{n}}}$-times the exhaust velocity. However, if the nuclear core is pulsed, thrust may be amplified ${\displaystyle n}$-times by amplifying the power ${\displaystyle n}$-times and the propellant mass flow ${\displaystyle n}$-times and keeping constant the exhaust velocity. 

I_sp amplification

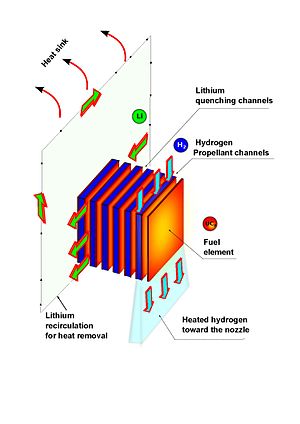
Pulsed nuclear thermal rocket unit cell concept for I_sp amplification. In this cell, hydrogen-propellant is heated by the continuous intense neutronic pulses in the propellant channels. At the same time, the unwanted energy from the fission fragments is removed by a solidary cooling channel with lithium or other liquid metal.

 

The attainment of high exhaust velocity or specific impulse (I_sp) is the first concern. The most general expression for the I_sp is given by 

${\displaystyle I_{\text{sp}}\simeq c{\sqrt {T}}}$

being ${\displaystyle c}$ a constant, and ${\displaystyle T}$ the temperature of the propellant before expansion. However, the temperature of the propellant is related directly with the energy as ${\displaystyle E\simeq kT}$, where ${\displaystyle k}$ is the Boltzmann constant. Thus,

${\displaystyle I_{\text{sp}}\simeq c'{\sqrt {E}}}$

being ${\displaystyle c'}$ a constant. 

In a conventional stationary NTR, the energy ${\displaystyle E}$ for heating the propellant is almost from the fission fragments which encompass almost the 95% of the total energy, and the faction of energy from prompt neutrons ${\displaystyle f_{\text{n}}}$ is only around 5%, and therefore, in comparison, is almost negligible. However, if the nuclear core is pulsed -as previously discussed, it is able to produce ${\displaystyle N}$ times more energy than the stationary mode, and then the fraction of prompt neutrons or ${\displaystyle f_{\text{n}}N}$ could be equal or larger than the total energy in the stationary mode; and because this neutron energy is directly transported from the fuel into the propellant as kinetic energy -unlike the energy from fission fragments which is transported as heat from the fuel into the propellant, then is not constrained by the second law of thermodynamics, meaning that there is no impediment to transport this energy from the fuel to the propellant even if the fuel is colder than the propellant, in other words, it is possible a " propellant hotter than the fuel" which is the very limit for specific impulse enhancement in classics NTRs. 

In summary, if the pulse generates ${\displaystyle N}$ times more energy than the stationary mode, the I_sp amplification is given by

${\displaystyle I_{\text{sp}}\simeq I_{\text{sp,o}}{\sqrt {f_{\text{n}}N+1}}}$

Where:

${\displaystyle I_{\text{sp}}}$ is the amplified specific impulse,

${\displaystyle I_{\text{sp,o}}}$ the specific impulse in the stationary mode,

${\displaystyle f_{\text{n}}}$ the fraction of prompt neutrons,

${\displaystyle N}$ the energy amplification by pulsing the nuclear core.

With values of ${\displaystyle N}$ between ${\displaystyle 10^{2}}$ to ${\displaystyle 10^{3}}$ and prompt neutron fractions around ${\displaystyle f_{\text{n}}\simeq {\frac {1}{20}}}$, the hypothetical ${\displaystyle I_{\text{sp}}}$ amplification attainable makes the concept specially interesting for interplanetary spaceflight. 

Advantages of the design

There are several advantages relative to conventional stationary NTR designs. Because the neutron energy is transported as kinetic energy from the fuel into the propellant, then a propellant hotter than the fuel is possible and therefore the ${\displaystyle I_{\text{sp}}}$ is not limited to the maximum temperature permissible by the fuel, i.e., its melting temperature.

The other nuclear rocket concept which allows a propellant hotter than the fuel is the fission fragment rocket. Because it directly uses the fission fragments as propellant, it can also achieve a very high specific impulse.

Other considerations

For ${\displaystyle I_{\text{sp}}}$ amplification, only the energy from prompt neutrons, and some prompt gamma energy, is used for this purpose. The rest of the energy, i.e., the almost ${\displaystyle 95\%}$ from fission fragments is unwanted energy and must be continuously evacuated by a heat removal auxiliary system using a suitable coolant. Liquid metals, and particularly lithium, can provide the fast quenching rates required. One aspect to be considered is the large amount of energy which must be evacuated as residual heat (almost 95% of the total energy). This implies a large dedicated heat transfer surface.

As regards to the mechanism for pulsing the core, the pulsed mode can be produced using a variety of configurations depending on the desired frequency of the pulsations. For instance, the use of standard control rods in a single or banked configuration with motor driving mechanism or the use of standard pneumatically operated pulsing mechanisms are suitable for generating up to 10 pulses per minute. For the production of pulses at rates up to 50 pulsations per second, the use of rotating wheels introducing alternately neutron poison and fuel or neutron poison and non-neutron poison can be considered. However, for pulsations ranking the thousands of pulses per second (kHz), optical choppers or modern wheels employing magnetic bearings allow to revolve at 10 kHz. If even faster pulsations are desired it would be necessary to make use of a new type of pulsing mechanism that does not involve mechanical motion, for example, lasers (based in the 3He polarization) as early proposed by Bowman, or proton and neutron beams. Frequencies on the order of 1 kHz to 10 kHz are likely choices.

Nuclear pulse propulsion

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Nuclear_pulse_propulsion

 

An artist's conception of the Project Orion "basic" spacecraft, powered by nuclear pulse propulsion.

 

Nuclear pulse propulsion or external pulsed plasma propulsion is a hypothetical method of spacecraft propulsion that uses nuclear explosions for thrust. It was first developed as Project Orion by DARPA, after a suggestion by Stanislaw Ulam in 1947. Newer designs using inertial confinement fusion have been the baseline for most post-Orion designs, including Project Daedalus and Project Longshot. 

Project Orion

A nuclear pulse propulsion unit. The explosive charge ablatively vaporizes the propellant, propelling it away from the charge, and simultaneously creating a plasma out of the propellant. The propellant then goes on to impact the pusher plate at the bottom of the Orion spacecraft, imparting a pulse of 'pushing' energy.

 

Project Orion was the first serious attempt to design a nuclear pulse rocket. The design effort was carried out at General Atomics in the late 1950s and early 1960s. The idea of Orion was to react small directional nuclear explosives utilizing a variant of the Teller-Ulam two-stage bomb design against a large steel pusher plate attached to the spacecraft with shock absorbers. Efficient directional explosives maximized the momentum transfer, leading to specific impulses in the range of 6,000 seconds, or about thirteen times that of the Space Shuttle main engine. With refinements a theoretical maximum of 100,000 seconds (1 MN·s/kg) might be possible. Thrusts were in the millions of tons, allowing spacecraft larger than 8×10⁶ tons to be built with 1958 materials.

The reference design was to be constructed of steel using submarine-style construction with a crew of more than 200 and a vehicle takeoff weight of several thousand tons. This low-tech single-stage reference design would reach Mars and back in four weeks from the Earth's surface (compared to 12 months for NASA's current chemically powered reference mission). The same craft could visit Saturn's moons in a seven-month mission (compared to chemically powered missions of about nine years). 

A number of engineering problems were found and solved over the course of the project, notably related to crew shielding and pusher-plate lifetime. The system appeared to be entirely workable when the project was shut down in 1965, the main reason being given that the Partial Test Ban Treaty made it illegal (however, before the treaty, the US and Soviet Union had already detonated at least nine nuclear bombs, including thermonuclear bombs, in space, i.e., at altitudes over 100 km: see high-altitude nuclear explosions). There were also ethical issues with launching such a vehicle within the Earth's magnetosphere: calculations using the now disputed linear no-threshold model of radiation damage showed that the fallout from each takeoff would kill between 1 and 10 people. In a threshold model, such extremely low levels of thinly distributed radiation would have no associated ill-effects, while under hormesis models, such tiny doses would be negligibly beneficial. With the possible use of less efficient clean nuclear bombs for achieving orbit and then more efficient higher yield dirty bombs for travel would bring down the amount of fallout caused from an Earth-based launch by a significant factor. 

One useful mission for this near-term technology would be to deflect an asteroid that could collide with the Earth, depicted dramatically in the 1998 film Deep Impact, even though it was a comet in that particular film. The extremely high performance would permit even a late launch to succeed, and the vehicle could effectively transfer a large amount of kinetic energy to the asteroid by simple impact, and in the event of an imminent asteroid impact a few predicted deaths from fallout would probably not be considered prohibitive. Also, an automated mission would eliminate the most problematic issues of the design: the shock absorbers.

Orion is one of very few interstellar space drives that could theoretically be constructed with available technology, as discussed in a 1968 paper, Interstellar Transport by Freeman Dyson. 

Project Daedalus

Project Daedalus was a study conducted between 1973 and 1978 by the British Interplanetary Society (BIS) to design a plausible interstellar unmanned spacecraft that could reach a nearby star within one human scientist's working lifetime or about 50 years. A dozen scientists and engineers led by Alan Bond worked on the project. At the time fusion research appeared to be making great strides, and in particular, inertial confinement fusion (ICF) appeared to be adaptable as a rocket engine. 

ICF uses small pellets of fusion fuel, typically lithium deuteride (⁶Li²H) with a small deuterium/tritium trigger at the center. The pellets are thrown into a reaction chamber where they are hit on all sides by lasers or another form of beamed energy. The heat generated by the beams explosively compresses the pellet, to the point where fusion takes place. The result is a hot plasma, and a very small "explosion" compared to the minimum size bomb that would be required to instead create the necessary amount of fission. 

For Daedalus, this process was run within a large electromagnet which formed the rocket engine. After the reaction, ignited by electron beams in this case, the magnet funnelled the hot gas to the rear for thrust. Some of the energy was diverted to run the ship's systems and engine. In order to make the system safe and energy efficient, Daedalus was to be powered by a helium-3 fuel that would have had to be collected from Jupiter. 

Medusa

Conceptual diagram of a Medusa propulsion spacecraft, showing: (A) the payload capsule, (B) the winch mechanism, (C) the optional main tether cable, (D) riser tethers, and (E) the parachute mechanism.

 

Operating sequence of the Medusa propulsion system. This diagram shows the operating sequence of a Medusa propulsion spacecraft (1) Starting at moment of explosive-pulse unit firing, (2) As the explosive pulse reaches the parachute canopy, (3) Pushes the canopy, accelerating it away from the explosion as the spacecraft plays out the main tether with the winch, generating electricity as it extends, and accelerating the spacecraft, (4) And finally winches the spacecraft forward to the canopy and uses excess electricity for other purposes.

 

The Medusa design is a type of nuclear pulse propulsion which has more in common with solar sails than with conventional rockets. It was envisioned by Johndale Solem in the 1990s and published in the Journal of the British Interplanetary Society (JBIS).

A Medusa spacecraft would deploy a large "spinnaker" sail ahead of it, attached by separate independent cables, and then launch nuclear explosives forward to detonate between itself and its sail. The sail would be accelerated by the plasma and photonic impulse, running out the tethers as when a fish flees the fisherman, and generating electricity at the "reel". The spacecraft would then use some of the generated electricity to reel itself up towards the sail, constantly smoothly accelerating as it goes.

In the original design, multiple tethers connected to multiple motor generators. The advantage over the single tether is to increase the distance between the explosion and the tethers, thus reducing damage to the tethers.

For heavy payloads, performance could be improved by taking advantage of lunar materials, for example, wrapping the explosive with lunar rock or water, likely stored previously at a stable Earth-Moon Lagrange point to be subsequently acquired by the Medusa spacecraft.

Medusa performs better than the classical Orion design because its sail intercepts more of the explosive impulse, its shock-absorber stroke is much longer, and all its major structures are in tension and hence can be quite lightweight. Medusa-type ships would be capable of a specific impulse between 50,000 and 100,000 seconds (500 to 1000 kN·s/kg).

Medusa is widely known to the public in the BBC documentary film To Mars By A-Bomb: The Secret History of Project Orion. A short film shows an artist's conception of how the Medusa spacecraft works "by throwing bombs into a sail that's ahead of it".

Project Longshot

Project Longshot was a NASA-sponsored research project carried out in conjunction with the US Naval Academy in the late 1980s. Longshot was in some ways a development of the basic Daedalus concept, in that it used magnetically funneled ICF as a rocket. The key difference was that they felt that the reaction could not power both the rocket and the systems, and instead included a 300 kW conventional nuclear reactor for running the ship. The added weight of the reactor reduced performance somewhat, but even using LiD fuel it would be able to reach Alpha Centauri, the closest solar system to our own, in 100 years (approx. velocity of 13,411 km/s, at a distance of 4.5 light years - equivalent to 4.5% of light speed). 

Antimatter-catalyzed nuclear pulse propulsion

In the mid-1990s research at the Pennsylvania State University led to the concept of using antimatter to catalyze nuclear reactions. In short, antiprotons would react inside the nucleus of uranium, causing a release of energy that breaks the nucleus apart as in conventional nuclear reactions. Even a small number of such reactions can start the chain reaction that would otherwise require a much larger volume of fuel to sustain. Whereas the "normal" critical mass for plutonium is about 11.8 kilograms (for a sphere at standard density), with antimatter catalyzed reactions this could be well under one gram. 

Several rocket designs using this reaction were proposed, some which would use all-fission reactions for interplanetary missions, and others using fission-fusion (effectively a very small version of Orion's bombs) for interstellar missions. 

MSNW magneto-inertial fusion driven rocket

MSNW magneto-inertial fusion driven rocket

Concept graphic of a fusion-driven rocket powered spacecraft arriving at Mars
Designer	MSNW LLC
Application	Interplanetary
Status	Theoretical
Performance
Specific impulse	1,606 s to 5,722 s (depending on fusion gain)
Burn time	1 day to 90 days (10 days optimal with gain of 40)
References
References
Notes	Fuel: Deuterium-tritium cryogenic pellet Propellent: Lithium or aluminum Power requirements: 100 kW to 1,000 kW

NASA funded MSNW LLC and the University of Washington in 2011 to study and develop a fusion rocket through the NASA Innovative Advanced Concepts NIAC Program.

The rocket uses a form of magneto-inertial fusion to produce a direct thrust fusion rocket. Powerful magnetic fields cause large metal rings (likely made of lithium, where a set for one pulse has a total mass of 365 grams) to collapse around the deuterium-tritium plasma, compressing it to a fusion state. Energy from these fusion reactions heats and ionizes the shell of metal formed by the crushed rings. The hot, ionized metal is shot out of a magnetic rocket nozzle at a high speed (up to 30 km/s). Repeating this process roughly every minute would propel the spacecraft. The fusion reaction is not self-sustaining and requires electrical energy to induce fusion. With electrical requirements estimated to be between 100 kW to 1,000 kW (300 kW average), spacecraft designs incorporate solar panels to produce the electrical energy needed for the fusion engine.

This approach uses Foil Liner Compression to create a fusion reaction of the proper energy scale to be used for space propulsion. The proof of concept experiment in Redmond, Washington, will use aluminum liners for compression. However, the actual rocket design will run with lithium liners.

The performance characteristics of the engine are highly dependent on the Fusion energy gain factor achieved by the reactor. Gains are expected to be between a factor of 20 and 200, with an estimated average of 40. With higher fusions gains comes higher exhaust velocity, higher specific impulse and lower electrical power requirements. The table below summarizes different performance characteristics for a theoretical 90-day Mars transfer at gains of 20, 40 and 200.

FDR parameters for 90 Mars transfer burn

Total gain	Gain 20	Gain 40	Gain 200
Liner mass (kg)	0.365	0.365	0.365
Specific impulse (s)	1,606	2,435	5,722
Mass fraction	0.33	0.47	0.68
Specific mass (kg/kW)	0.8	0.53	0.23
Mass propellant (kg)	110,000	59,000	20,000
Mass initial (kg)	184,000	130,000	90,000
Electrical power required (kW)	1,019	546	188

By April 2013, MSNW had demonstrated subcomponents of the systems: heating deuterium plasma up to fusion temperatures and have concentrated the magnetic fields needed to create fusion. They planned to put the two technologies together for a test before the end of 2013.

They could later be scaled up in power and plan to add the necessary fusion fuel (deuterium) by the end (Sept 2014) of the NIAC Study.