Lagrange point

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Lagrange_point 

Small-mass objects (green) at the Lagrange points are in equilibrium with one massive body (blue) orbiting another (yellow). At all other points, the gravitational forces due to the two massive bodies are non-equilibria.

 

Lagrange points in the Sun–Earth system (not to scale). A small object at L4 or L5 will hold its relative position. A small object at L1, L2, or L3 will hold its relative position until deflected slightly radially, after which it will diverge from its original position.

 

An example of a spacecraft at Sun–Earth L2
  WMAP ·   Earth

In celestial mechanics, the Lagrange points /ləˈɡrɑːndʒ/ (also Lagrangian points, L-points, or libration points) are points of equilibrium for small-mass objects under the influence of two massive orbiting bodies. Mathematically, this involves the solution of the restricted three-body problem in which two bodies are very much more massive than the third.

Normally, the two massive bodies exert an unbalanced gravitational force at a point, altering the orbit of whatever is at that point. At the Lagrange points, the gravitational forces of the two large bodies and the centrifugal force balance each other. This can make Lagrange points an excellent location for satellites, as few orbit corrections are needed to maintain the desired orbit. Small objects placed in orbit at Lagrange points are in equilibrium in at least two directions relative to the center of mass of the large bodies.

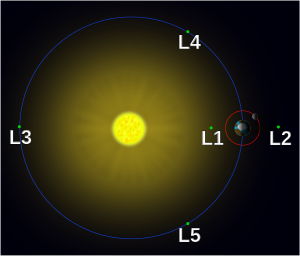
There are five such points, labelled L₁ to L₅, all in the orbital plane of the two large bodies, for each given combination of two orbital bodies. For instance, there are five Lagrangian points L₁ to L₅ for the Sun–Earth system, and in a similar way there are five different Lagrangian points for the Earth–Moon system. L₁, L₂, and L₃ are on the line through the centres of the two large bodies, while L₄ and L₅ each act as the third vertex of an equilateral triangle formed with the centres of the two large bodies. L₄ and L₅ are stable, which implies that objects can orbit around them in a rotating coordinate system tied to the two large bodies.

The L₄ and L₅ points are stable points and have a tendency to pull objects into them. Several planets have trojan asteroids near their L₄ and L₅ points with respect to the Sun. Jupiter has more than a million of these trojans. Artificial satellites have been placed at L₁ and L₂ with respect to the Sun and Earth, and with respect to the Earth and the Moon. The Lagrangian points have been proposed for uses in space exploration.

History

The three collinear Lagrange points (L₁, L₂, L₃) were discovered by Leonhard Euler a few years before Joseph-Louis Lagrange discovered the remaining two.

In 1772, Lagrange published an "Essay on the three-body problem". In the first chapter he considered the general three-body problem. From that, in the second chapter, he demonstrated two special constant-pattern solutions, the collinear and the equilateral, for any three masses, with circular orbits.

Lagrange points

The five Lagrange points are labelled and defined as follows:

L₁ point

The L₁ point lies on the line defined between the two large masses M₁ and M₂. It is the point where the gravitational attraction of M₂ and that of M₁ combine to produce an equilibrium. An object that orbits the Sun more closely than Earth would normally have a shorter orbital period than Earth, but that ignores the effect of Earth's own gravitational pull. If the object is directly between Earth and the Sun, then Earth's gravity counteracts some of the Sun's pull on the object, and therefore increases the orbital period of the object. The closer to Earth the object is, the greater this effect is. At the L₁ point, the orbital period of the object becomes exactly equal to Earth's orbital period. L₁ is about 1.5 million kilometers from Earth, or 0.01 au, 1/100th the distance to the Sun.

L₂ point

The L₂ point lies on the line through the two large masses, beyond the smaller of the two. Here, the gravitational forces of the two large masses balance the centrifugal effect on a body at L₂. On the opposite side of Earth from the Sun, the orbital period of an object would normally be greater than that of Earth. The extra pull of Earth's gravity decreases the orbital period of the object, and at the L₂ point that orbital period becomes equal to Earth's. Like L₁, L₂ is about 1.5 million kilometers or 0.01 au from Earth.

A notable example of an artificial satellite at L2 is the James Webb Space Telescope, designed to operate near the Earth–Sun L₂. See other spacecraft at Sun–Earth L₂.

L₃ point

The L₃ point lies on the line defined by the two large masses, beyond the larger of the two. Within the Sun–Earth system, the L₃ point exists on the opposite side of the Sun, a little outside Earth's orbit and slightly closer to the center of the Sun than Earth is. This placement occurs because the Sun is also affected by Earth's gravity and so orbits around the two bodies' barycentre, which is well inside the body of the Sun. An object at Earth's distance from the Sun would have an orbital period of one year if only the Sun's gravity is considered. But an object on the opposite side of the Sun from Earth and directly in line with both "feels" Earth's gravity adding slightly to the Sun's and therefore must orbit a little farther from the barycentre of Earth and Sun in order to have the same 1-year period. It is at the L₃ point that the combined pull of Earth and Sun causes the object to orbit with the same period as Earth, in effect orbiting an Earth+Sun mass with the Earth-Sun barycentre at one focus of its orbit.

L₄ and L₅ points

Gravitational accelerations at L₄

The L₄ and L₅ points lie at the third corners of the two equilateral triangles in the plane of orbit whose common base is the line between the centres of the two masses, such that the point lies behind (L₅) or ahead (L₄) of the smaller mass with regard to its orbit around the larger mass.

Stability

The triangular points (L₄ and L₅) are stable equilibria, provided that the ratio of M₁/M₂ is greater than 24.96. This is the case for the Sun–Earth system, the Sun–Jupiter system, and, by a smaller margin, the Earth–Moon system. When a body at these points is perturbed, it moves away from the point, but the factor opposite of that which is increased or decreased by the perturbation (either gravity or angular momentum-induced speed) will also increase or decrease, bending the object's path into a stable, kidney bean-shaped orbit around the point (as seen in the corotating frame of reference).

The points L₁, L₂, and L₃ are positions of unstable equilibrium. Any object orbiting at L₁, L₂, or L₃ will tend to fall out of orbit; it is therefore rare to find natural objects there, and spacecraft inhabiting these areas must employ station keeping in order to maintain their position.

Put Simply:

A Lagrange (Lagrangian) Point is one of five infinitely small points where, in relation to two mutually orbiting bodies, the forces due to the gravity of each body in opposition to each other and to the centrifugal force, mutually exactly oppose each other.

Lagrange Point 1 (L₁) is found along a line drawn between the centres of gravity of the two objects, at a distance from one and the other which is proportional to their masses.

L₂ is a point along the same line extended beyond the smaller body to a distance where the same counterbalance of forces holds true.

L₃ is a corresponding point along that line extended beyond the larger body.

L₄ and L₅ are found at the opposite vertices of the equilateral triangles whose common base is the line drawn between the centers of gravity the of the larger and smaller masses.

Infinitely small items placed at these points will, in the absence of random instabilities, stay there. If displaced, they will, without any other force, move away at ever increasing speeds. However, at L₄ and L₅, the Coriolis force will act upon an item to tend to return it to that point.

Natural objects at Lagrange points

Main article: List of objects at Lagrangian points

Due to the natural stability of L₄ and L₅, it is common for natural objects to be found orbiting in those Lagrange points of planetary systems. Objects that inhabit those points are generically referred to as 'trojans' or 'trojan asteroids'. The name derives from the names that were given to asteroids discovered orbiting at the Sun–Jupiter L₄ and L₅ points, which were taken from mythological characters appearing in Homer's Iliad, an epic poem set during the Trojan War. Asteroids at the L₄ point, ahead of Jupiter, are named after Greek characters in the Iliad and referred to as the "Greek camp". Those at the L₅ point are named after Trojan characters and referred to as the "Trojan camp". Both camps are considered to be types of trojan bodies.

As the Sun and Jupiter are the two most massive objects in the Solar System, there are more Sun-Jupiter trojans than for any other pair of bodies. However, smaller numbers of objects are known at the Lagrange points of other orbital systems:

• The Sun–Earth L₄ and L₅ points contain interplanetary dust and at least two asteroids, 2010 TK7 and 2020 XL5.
• The Earth–Moon L₄ and L₅ points contain concentrations of interplanetary dust, known as Kordylewski clouds. Stability at these specific points is greatly complicated by solar gravitational influence.
• The Sun–Neptune L₄ and L₅ points contain several dozen known objects, the Neptune trojans.
• Mars has four accepted Mars trojans: 5261 Eureka, 1999 UJ7, 1998 VF31, and 2007 NS2.
• Saturn's moon Tethys has two smaller moons of Saturn in its L₄ and L₅ points, Telesto and Calypso. Another Saturn moon, Dione also has two Lagrangian co-orbitals, Helene at its L₄ point and Polydeuces at L₅. The moons wander azimuthally about the Lagrangian points, with Polydeuces describing the largest deviations, moving up to 32° away from the Saturn–Dione L₅ point.
• One version of the giant impact hypothesis postulates that an object named Theia formed at the Sun–Earth L₄ or L₅ point and crashed into Earth after its orbit destabilized, forming the Moon.
• In binary stars, the Roche lobe has its apex located at L₁; if one of the stars expands past its Roche lobe, then it will lose matter to its companion star, known as Roche lobe overflow.

Objects which are on horseshoe orbits are sometimes erroneously described as trojans, but do not occupy Lagrange points. Known objects on horseshoe orbits include 3753 Cruithne with Earth, and Saturn's moons Epimetheus and Janus.

Physical and mathematical details

A contour plot of the effective potential due to gravity and the centrifugal force of a two-body system in a rotating frame of reference. The arrows indicate the gradients of the potential around the five Lagrange points—downhill toward them (red) or away from them (blue). Counterintuitively, the L₄ and L₅ points are the high points of the potential. At the points themselves these forces are balanced.

 

Visualisation of the relationship between the Lagrangian points (red) of a planet (blue) orbiting a star (yellow) counterclockwise, and the effective potential in the plane containing the orbit (grey rubber-sheet model with purple contours of equal potential).
Click for animation.

Lagrangian points are the constant-pattern solutions of the restricted three-body problem. For example, given two massive bodies in orbits around their common barycenter, there are five positions in space where a third body, of comparatively negligible mass, could be placed so as to maintain its position relative to the two massive bodies. As seen in a rotating reference frame that matches the angular velocity of the two co-orbiting bodies, the gravitational fields of two massive bodies combined providing the centripetal force at the Lagrangian points, allowing the smaller third body to be relatively stationary with respect to the first two.

L₁

The location of L₁ is the solution to the following equation, gravitation providing the centripetal force:

$${\displaystyle {\frac {M_{1}}{(R-r)^{2}}}-{\frac {M_{2}}{r^{2}}}=\left({\frac {M_{1}}{M_{1}+M_{2}}}R-r\right){\frac {M_{1}+M_{2}}{R^{3}}}}$$

where r is the distance of the L₁ point from the smaller object, R is the distance between the two main objects, and M₁ and M₂ are the masses of the large and small object, respectively. (The quantity in parentheses on the right is the distance of L₁ from the center of mass.) Solving this for r involves solving a quintic function, but if the mass of the smaller object (M₂) is much smaller than the mass of the larger object (M₁) then L₁ and L₂ are at approximately equal distances r from the smaller object, equal to the radius of the Hill sphere, given by:

$${\displaystyle r\approx R{\sqrt[{3}]{\frac {M_{2}}{3M_{1}}}}}$$

We may also write this as:

$${\displaystyle {\frac {M_{2}}{r^{3}}}\approx 3{\frac {M_{1}}{R^{3}}}}$$

Since the tidal effect of a body is proportional to its mass divided by the distance cubed, this means that the tidal effect of the smaller body at the L₁ or at the L₂ point is about three times of that body. We may also write:

$${\displaystyle \rho _{2}(d_{2}/r)^{3}\approx 3\rho _{1}(d_{1}/R)^{3}}$$

where ρ₁ and ρ₂ are the average densities of the two bodies and ${\displaystyle d_{1}}$ and ${\displaystyle d_{2}}$ are their diameters. The ratio of diameter to distance gives the angle subtended by the body, showing that viewed from these two Lagrange points, the apparent sizes of the two bodies will be similar, especially if the density of the smaller one is about thrice that of the larger, as in the case of the earth and the sun.

This distance can be described as being such that the orbital period, corresponding to a circular orbit with this distance as radius around M₂ in the absence of M₁, is that of M₂ around M₁, divided by √3 ≈ 1.73:

$${\displaystyle T_{s,M_{2}}(r)={\frac {T_{M_{2},M_{1}}(R)}{\sqrt {3}}}.}$$

L₂

The Lagrangian L₂ point for the Sun–Earth system.

The location of L₂ is the solution to the following equation, gravitation providing the centripetal force:

$${\displaystyle {\frac {M_{1}}{(R+r)^{2}}}+{\frac {M_{2}}{r^{2}}}=\left({\frac {M_{1}}{M_{1}+M_{2}}}R+r\right){\frac {M_{1}+M_{2}}{R^{3}}}}$$

with parameters defined as for the L₁ case. Again, if the mass of the smaller object (M₂) is much smaller than the mass of the larger object (M₁) then L₂ is at approximately the radius of the Hill sphere, given by:

$${\displaystyle r\approx R{\sqrt[{3}]{\frac {M_{2}}{3M_{1}}}}}$$

The same remarks about tidal influence and apparent size apply as for the L₁ point. For example, the angular radius of the sun as viewed from L₂ is arcsin(695.5×10³/151.1×10⁶) ≈ 0.264°, whereas that of the earth is arcsin(6371/1.5×10⁶) ≈ 0.242°. Looking toward the sun from L₂ one sees an annular eclipse. It is necessary for a spacecraft, like Gaia, to follow a Lissajous orbit or a halo orbit around L₂ in order for its solar panels to get full sun.

L₃

The location of L₃ is the solution to the following equation, gravitation providing the centripetal force:

$${\displaystyle {\frac {M_{1}}{\left(R-r\right)^{2}}}+{\frac {M_{2}}{\left(2R-r\right)^{2}}}=\left({\frac {M_{2}}{M_{1}+M_{2}}}R+R-r\right){\frac {M_{1}+M_{2}}{R^{3}}}}$$

with parameters M₁, M₂ and R defined as for the L₁ and L₂ cases, and r now indicates the distance of L₃ from the position of the smaller object, if it were rotated 180 degrees about the larger object, while positive r implying L₃ is closer to the larger object than the smaller object. If the mass of the smaller object (M₂) is much smaller than the mass of the larger object (M₁) then:

$${\displaystyle r\approx R{\frac {7M_{2}}{12M_{1}}}}$$

L₄ and L₅

Further information: Trojan (celestial body)

The reason these points are in balance is that, at L₄ and L₅, the distances to the two masses are equal. Accordingly, the gravitational forces from the two massive bodies are in the same ratio as the masses of the two bodies, and so the resultant force acts through the barycenter of the system; additionally, the geometry of the triangle ensures that the resultant acceleration is to the distance from the barycenter in the same ratio as for the two massive bodies. The barycenter being both the center of mass and center of rotation of the three-body system, this resultant force is exactly that required to keep the smaller body at the Lagrange point in orbital equilibrium with the other two larger bodies of the system (indeed, the third body needs to have negligible mass). The general triangular configuration was discovered by Lagrange working on the three-body problem.

Net radial acceleration of a point orbiting along the Earth–Moon line

Radial acceleration

The radial acceleration a of an object in orbit at a point along the line passing through both bodies is given by:

$${\displaystyle a=-{\frac {GM_{1}}{r^{2}}}\operatorname {sgn}(r)+{\frac {GM_{2}}{(R-r)^{2}}}\operatorname {sgn}(R-r)+{\frac {G{\bigl (}(M_{1}+M_{2})r-M_{2}R{\bigr )}}{R^{3}}}}$$

where r is the distance from the large body M₁, R is the distance between the two main objects and sgn(x) is the sign function of x. The terms in this function represent respectively: force from M₁; force from M₂; and centripetal force. The points L₃, L₁, L₂ occur where the acceleration is zero — see chart at right. Positive acceleration is acceleration towards the right of the chart and negative acceleration is towards the left; that is why acceleration has opposite signs on opposite sides of the gravity wells.

Stability

Although the L₁, L₂, and L₃ points are nominally unstable, there are quasi-stable periodic orbits called halo orbits around these points in a three-body system. A full n-body dynamical system such as the Solar System does not contain these periodic orbits, but does contain quasi-periodic (i.e. bounded but not precisely repeating) orbits following Lissajous-curve trajectories. These quasi-periodic Lissajous orbits are what most of Lagrangian-point space missions have used until now. Although they are not perfectly stable, a modest effort of station keeping keeps a spacecraft in a desired Lissajous orbit for a long time.

For Sun–Earth-L₁ missions, it is preferable for the spacecraft to be in a large-amplitude (100,000–200,000 km or 62,000–124,000 mi) Lissajous orbit around L₁ than to stay at L₁, because the line between Sun and Earth has increased solar interference on Earth–spacecraft communications. Similarly, a large-amplitude Lissajous orbit around L₂ keeps a probe out of Earth's shadow and therefore ensures continuous illumination of its solar panels.

The L₄ and L₅ points are stable provided that the mass of the primary body (e.g. the Earth) is at least 25 times the mass of the secondary body (e.g. the Moon), and the mass of the secondary is at least 10 times that of the tertiary (e.g. the satellite). The Earth is over 81 times the mass of the Moon (the Moon is 1.23% of the mass of the Earth). Although the L₄ and L₅ points are found at the top of a "hill", as in the effective potential contour plot above, they are nonetheless stable. The reason for the stability is a second-order effect: as a body moves away from the exact Lagrange position, Coriolis acceleration (which depends on the velocity of an orbiting object and cannot be modeled as a contour map) curves the trajectory into a path around (rather than away from) the point. Because the source of stability is the Coriolis force, the resulting orbits can be stable, but generally are not planar, but "three-dimensional": they lie on a warped surface intersecting the ecliptic plane. The kidney-shaped orbits typically shown nested around L₄ and L₅ are the projections of the orbits on a plane (e.g. the ecliptic) and not the full 3-D orbits.

Solar System values

Sun-planet Lagrange points to scale (click for clearer points)

This table lists sample values of L₁, L₂, and L₃ within the Solar System. Calculations assume the two bodies orbit in a perfect circle with separation equal to the semimajor axis and no other bodies are nearby. Distances are measured from the larger body's center of mass with L₃ showing a negative location. The percentage columns show how the distances compare to the semimajor axis. E.g. for the Moon, L₁ is located 326400 km from Earth's center, which is 84.9% of the Earth–Moon distance or 15.1% in front of the Moon; L₂ is located 448900 km from Earth's center, which is 116.8% of the Earth–Moon distance or 16.8% beyond the Moon; and L₃ is located −381700 km from Earth's center, which is 99.3% of the Earth–Moon distance or 0.7084% in front of the Moon's 'negative' position.

Lagrangian points in Solar System

Body pair	Semimajor axis, SMA (×109 m)	L1 (×109 m)	1 − L1/SMA (%)	L2 (×109 m)	L2/SMA − 1 (%)	L3 (×109 m)	1 + L3/SMA (%)
Earth–Moon	0.3844	0.32639	15.09	0.4489	16.78	−0.38168	0.7084
Sun–Mercury	57.909	57.689	0.3806	58.13	0.3815	−57.909	0.000009683
Sun–Venus	108.21	107.2	0.9315	109.22	0.9373	−108.21	0.0001428
Sun–Earth	149.6	148.11	0.997	151.1	1.004	−149.6	0.0001752
Sun–Mars	227.94	226.86	0.4748	229.03	0.4763	−227.94	0.00001882
Sun–Jupiter	778.34	726.45	6.667	832.65	6.978	−777.91	0.05563
Sun–Saturn	1426.7	1362.5	4.496	1492.8	4.635	−1426.4	0.01667
Sun–Uranus	2870.7	2801.1	2.421	2941.3	2.461	−2870.6	0.002546
Sun–Neptune	4498.4	4383.4	2.557	4615.4	2.602	−4498.3	0.003004

Spaceflight applications

Sun–Earth

The satellite ACE in an orbit around Sun–Earth L₁

Sun–Earth L₁ is suited for making observations of the Sun–Earth system. Objects here are never shadowed by Earth or the Moon and, if observing Earth, always view the sunlit hemisphere. The first mission of this type was the 1978 International Sun Earth Explorer 3 (ISEE-3) mission used as an interplanetary early warning storm monitor for solar disturbances. Since June 2015, DSCOVR has orbited the L₁ point. Conversely it is also useful for space-based solar telescopes, because it provides an uninterrupted view of the Sun and any space weather (including the solar wind and coronal mass ejections) reaches L₁ up to an hour before Earth. Solar and heliospheric missions currently located around L₁ include the Solar and Heliospheric Observatory, Wind, and the Advanced Composition Explorer. Planned missions include the Interstellar Mapping and Acceleration Probe (IMAP) and the NEO Surveyor.

Sun–Earth L₂ is a good spot for space-based observatories. Because an object around L₂ will maintain the same relative position with respect to the Sun and Earth, shielding and calibration are much simpler. It is, however, slightly beyond the reach of Earth's umbra, so solar radiation is not completely blocked at L₂. Spacecraft generally orbit around L₂, avoiding partial eclipses of the Sun to maintain a constant temperature. From locations near L₂, the Sun, Earth and Moon are relatively close together in the sky; this means that a large sunshade with the telescope on the dark-side can allow the telescope to cool passively to around 50 K – this is especially helpful for infrared astronomy and observations of the cosmic microwave background. The James Webb Space Telescope has been positioned at L₂ (12/25/21).

Sun–Earth L₃ was a popular place to put a "Counter-Earth" in pulp science fiction and comic books. Once space-based observation became possible via satellites and probes, it was shown to hold no such object. The Sun–Earth L₃ is unstable and could not contain a natural object, large or small, for very long. This is because the gravitational forces of the other planets are stronger than that of Earth (Venus, for example, comes within 0.3 AU of this L₃ every 20 months).

A spacecraft orbiting near Sun–Earth L₃ would be able to closely monitor the evolution of active sunspot regions before they rotate into a geoeffective position, so that a seven-day early warning could be issued by the NOAA Space Weather Prediction Center. Moreover, a satellite near Sun–Earth L₃ would provide very important observations not only for Earth forecasts, but also for deep space support (Mars predictions and for crewed mission to near-Earth asteroids). In 2010, spacecraft transfer trajectories to Sun–Earth L₃ were studied and several designs were considered.

Missions to Lagrangian points generally orbit the points rather than occupy them directly.

Another interesting and useful property of the collinear Lagrangian points and their associated Lissajous orbits is that they serve as "gateways" to control the chaotic trajectories of the Interplanetary Transport Network.

Earth–Moon

Earth–Moon L₁ allows comparatively easy access to Lunar and Earth orbits with minimal change in velocity and this has as an advantage to position a habitable space station intended to help transport cargo and personnel to the Moon and back.

Earth–Moon L₂ has been used for a communications satellite covering the Moon's far side, for example, Queqiao, launched in 2018, and would be an ideal location for a propellant depot as part of the proposed depot-based space transportation architecture.

Sun–Venus

Scientists at the B612 Foundation were planning to use Venus's L₃ point to position their planned Sentinel telescope, which aimed to look back towards Earth's orbit and compile a catalogue of near-Earth asteroids.

Sun–Mars

In 2017, the idea of positioning a magnetic dipole shield at the Sun–Mars L₁ point for use as an artificial magnetosphere for Mars was discussed at a NASA conference. The idea is that this would protect the planet's atmosphere from the Sun's radiation and solar winds.

Lagrangian spacecraft and missions

Main article: List of objects at Lagrangian points

Spacecraft at Sun–Earth L₁

International Sun Earth Explorer 3 (ISEE-3) began its mission at the Sun–Earth L₁ before leaving to intercept a comet in 1982. The Sun–Earth L₁ is also the point to which the Reboot ISEE-3 mission was attempting to return the craft as the first phase of a recovery mission (as of September 25, 2014 all efforts have failed and contact was lost).

Solar and Heliospheric Observatory (SOHO) is stationed in a halo orbit at L₁, and the Advanced Composition Explorer (ACE) in a Lissajous orbit. WIND is also at L₁. Currently slated for launch in late 2024, the Interstellar Mapping and Acceleration Probe will be placed near L₁.

Deep Space Climate Observatory (DSCOVR), launched on 11 February 2015, began orbiting L₁ on 8 June 2015 to study the solar wind and its effects on Earth. DSCOVR is unofficially known as GORESAT, because it carries a camera always oriented to Earth and capturing full-frame photos of the planet similar to the Blue Marble. This concept was proposed by then-Vice President of the United States Al Gore in 1998 and was a centerpiece in his 2006 film An Inconvenient Truth.

LISA Pathfinder (LPF) was launched on 3 December 2015, and arrived at L₁ on 22 January 2016, where, among other experiments, it tested the technology needed by (e)LISA to detect gravitational waves. LISA Pathfinder used an instrument consisting of two small gold alloy cubes.

After ferrying lunar samples back to Earth, the transport module of Chang'e 5 was sent to L1 with its remaining fuel as part of the Chinese Lunar Exploration Program on 16 December 2020 where it is permanently stationed to conduct limited Earth-Sun observations.

Spacecraft at Sun–Earth L₂

Spacecraft at the Sun–Earth L₂ point are in a Lissajous orbit until decommissioned, when they are sent into a heliocentric graveyard orbit.

• 1 October 2001 – October 2010: Wilkinson Microwave Anisotropy Probe
• November 2003 – April 2004: WIND, then it returned to Earth orbit before going to L₁ where it still remains
• July 2009 – 29 April 2013: Herschel Space Telescope
• 3 July 2009 – 21 October 2013: Planck Space Observatory
• 25 August 2011 – April 2012: Chang'e 2, from where it travelled to 4179 Toutatis and then into deep space
• January 2014: Gaia Space Observatory
• 2019: Spektr-RG X-Ray Observatory
• 2021: James Webb Space Telescope uses a halo orbit
• 2022: Euclid Space Telescope
• 2024: Nancy Grace Roman Space Telescope (WFIRST) will use a halo orbit
• 2031: Advanced Telescope for High-Energy Astrophysics (ATHENA) will use a halo orbit

Spacecraft at Earth–Moon L₂

• Chang'e 5-T1 experimental spacecraft DFH-3A "service module" was sent to the Earth-Moon L₂ lunar Lissajous orbit on 13 January 2015, where it used the remaining 800 kg of fuel to test maneuvers key to future lunar missions.
• Queqiao entered orbit around the Earth–Moon L₂ on 14 June 2018. It serves as a relay satellite for the Chang'e 4 lunar far-side lander, which cannot communicate directly with Earth.

Overpopulation

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Overpopulation 

 

This article is about overpopulation in biology in general. For the human overpopulation, see human overpopulation.

Overpopulation or overabundance is a phenomenon that occurs when a species’ population becomes larger than the carrying capacity of its environment. This may occur from increased birth rates, less predation or lower mortality rates, and large scale migration. As a result, the overpopulated species as well as other animals in the ecosystem begin to compete for food, space, and resources. The animals in an overpopulated area may then be forced to migrate to areas not typically inhabited, or die off without access to necessary resources.

Background

In ecology, overpopulation is a concept used primarily in wildlife management. Typically, an overpopulation causes the entire population of the species in question to become weaker, as no single individual is able to find enough food or shelter. As such, overpopulation is thus characterized by an increase in the diseases and parasite-load which live upon the species in question, as the entire population is weaker. Other characteristics of overpopulation are lower fecundity, adverse effects on the environment (soil, vegetation or fauna) and lower average body weights. Especially the worldwide increase of deer populations, which usually show irruptive growth, is proving to be of ecological concern. Ironically, where ecologists were preoccupied with conserving or augmenting deer populations only a century ago, the focus has now shifted in the direct opposite, and ecologists are now more concerned with limiting the populations of such animals.

Supplemental feeding of charismatic species or interesting game species is a major problem in causing overpopulation, as is too little hunting or trapping of such species. Management solutions are increasing hunting by making it easier or cheaper for (foreign) hunters to hunt, banning supplemental feeding, awarding bounties, forcing landowners to hunt or contract professional hunters, using immunocontraception, promoting the harvest of venison or other wild meats, introducing large predators (rewilding), poisonings or introducing diseases.

A useful tool in wildlife culling is the use of mobile freezer trailers in which to store carcasses. The harvest of meat from wild animals is a sustainable method of creating a circular economy.

Immunocontraception is more ethical for animal rights activists, but it has proved completely impractical.

Well studied species

Deer

In Scotland the program of having landowners privately cull the overpopulation of red deer in the highlands has proved an abject failure. Scotland's deer are stunted, emaciated, and frequently starve in the Spring. As of 2016 the population is now so high, that 100,000 deer would need to be culled each year only to maintain the current population. A number of landowners have proven unwilling to accede to the law, requiring government intervention anyway. It has been necessary to contract professional hunters in order to satisfy landowner legislation regarding the annual cull. Millions of pounds of taxpayers' cash is spent on the annual cull. As of 2020, 100,000 deer are shot each year. Compounding the problem, some landowners have used supplemental feeding at certain shooting blinds in order to ease sport hunting.

Overpopulation can have effects on forage plants, eventually causing a species to thus alter the greater environment. Natural ecosystems are extremely complex. The overpopulation of deer in Britain has been caused by legislation making hunting more difficult, but another reason may be the proliferation of forests, used by different deer species to breed and shelter. Forests and parks have caused Britain to be much more forested than it was in recent history, and may thus perversely be causing biodiversity loss, conversion of heath habitat to grassland, extirpation of grassland and woodland plants due to overgrazing and the changing of the habitat structure. Examples are bluebells and primroses. Deer open up the forest and reduce the amount of brambles, which then has knock-on effects on dormice and certain birds which nest near the ground, such as the capercaillie, dunnock, nightingale, song thrush, willow warbler, marsh tit, willow tit and bullfinch. Populations of the nightingale and the European turtle dove are believed to be primarily impacted by muntjac. Grouse populations suffer due to smashing into the fencing needed to protect against deer. On the other hand common redstart and wood warblers may benefit from the more open understory created by the deer.

A significant amount of the environmental destruction in Britain is caused by an overabundance of deer. Besides ecological effects, overpopulation of deer causes economic effects due to browsing on crops, expensive fencing needed to combat this and protect new afforestation planting and coppice growth, and increasing numbers of road traffic incidents. Deer are in fact the most lethal animals of Britain, killing approximately 20 people a year from road accidents. In Scotland, the cost of road accidents due to these animals is estimated to be £7million, and such collisions cause injuries to 50 to 100 people a year. High populations cause stripping of the bark of trees, eventually destroying forests. Protecting forests from deer costs on average three times as much as planting the forest in the first place. The NGO Trees for Life spent weeks planting native trees in Scotland, aiming to rebuild the ancient Caledonian Forest. After winter snowdrifts in 2014/2015 flattened the deer fences, more than a decade's growth was lost in a matter of weeks. In 2009 – 2010 the cost of forest protection in Scotland ran to £10.5m.

Some animals, such as muntjac, are too small and boring for most hunters to shoot, which poses additional management problems.

In the United States the exact same problem is seen with white-tailed deer, where populations have exploded and become invasive species in some areas. In continental Europe roe deer pose a similar problem, although the populations were formerly much less, they have swelled in the 20th century so that although two and a half million are shot each year by hunters in Western Europe alone, as of 1998, the population still appears to be increasing, causing problems for forestry and traffic. In an experiment where roe deer on a Norwegian island was freed from human harvest and predators, the deer showed a doubling of the population each year or two. In the Netherlands and southern England roe deer were extirpated from the entirety of the country except for a few small areas around 1875. In the 1970s the species was still completely absent from Wales, but as of 2013, it has colonized the entire country. As new forests were planted in the Netherlands in the 20th century, the population began to expand rapidly. As of 2016 there are some 110,000 deer in the country.

Birds

Aquaculture operations, recreation angling and populations of endangered fish such as the schelly are impacted by cormorant populations. Open aquaculture ponds provide winter or year-round homes and food for cormorants. Cormorants' effect on the aquaculture industry is significant, with a dense flock capable of consuming an entire harvest. Cormorants are estimated to cost the catfish industry in Mississippi alone between $10 million and $25 million annually. Cormorant culling is commonly achieved by sharp-shooting, nest destruction, roost dispersal and oiling the eggs.

Geese numbers have also been called overpopulated. In the Canadian Arctic region, snow geese, Ross's geese, greater white-fronted geese and some populations of Canada geese have been increasing significantly over the past decades. Lesser snow geese populations have increased to over three million, and continue to increase by some 5% per year. Giant Canada geese have grown from near extinction to nuisance levels. Average body sizes have decreased and parasite loads are higher. Before the 1980s, Arctic geese populations had boom and bust cycles (see above) thought to be based on food availability, although there are still some bust years, this no longer seems the case.

It is difficult to know what the numbers of geese were before the 20th century, before human impact presumably altered them. There are a few anecdotal claims from that time of two or three million, but these are likely exaggerations, as that would imply a massive die-off or vast amounts harvested, for which there is no evidence. More likely estimates from the period of 1500 to 1900 are a few hundred thousand animals, which implies that with the exception of Ross's geese, modern populations of geese are many millions more than in pre-industrial levels.

Humans are blamed as the ultimate cause for the increase, directly and indirectly, due to management legislation limiting hunting introduced specifically in order to protect bird populations, but most importantly due to the increase in agriculture and large parks, which has had the effect of creating vast amounts of unintentional sanctuaries filled with food. Urban geese flocks have increased enormously. City ordinances generally prohibit discharging firearms, keeping such flocks safe, and there is abundant food. Geese profit from agricultural grain crops, and seem to be shifting their habitat preferences to such farmlands. Ironically, the creation of wildlife refuges may have exacerbated this: as geese overpopulations destroyed the Scirpus salt marsh habitats that they were originally restricted to, this has speeded their conversion to adopt new feeding habitats, while maintaining roosting sites in refuges. The creation of wildlife refuges to protect wetland habitats in the continental United States from the 1930s to 1950s seems to have had the effect of disrupting the migration routes, as geese no longer fly as far south to Texas and Louisiana as they once did. Reduction of goose hunting in the US since the 1970s seems to have further had the effect of protecting populations. In Canada hunting has also decreased dramatically, from 43.384% harvest rates in the 1960s to 8% in the 1990s. Nonetheless, when kill rates were compared to populations, hunting alone does not seems to be solely responsible for the increase -weather or a not yet completed shift in habitat preference to agricultural land may also be factors. Although hunting may have formerly been the main factor in maintaining stable populations, ecologists no longer consider it a practical management solution, as public interest in the practice has continued to wane, and the population is now so large that the massive culls needed are unrealistic to ask from the public. Climate change in the Arctic would appear to be an obvious cause for the increase, but when subpopulations are correlated with local climatic increases, this does not seem to hold true, and furthermore, breeding regions seem to be shifting southwards anyway, irrespective of climate change.

The nutrient subsidy provided by foraging in agricultural land has made the overall landscape use by geese unsustainable. Where such geese congregate local plant communities have been substantially altered; these chronic effects are cumulative, and have been considered a threat to the Arctic ecosystems, due to knock-off effects on native ducks, shorebirds and passerines. Grubbing and overgrazing by geese completely denudes the tundra and marshland, in combination with abiotic processes, this creates large desert expanses of hypersaline, anoxic mud which continue to increase each year -eventually these habitat changes become irreversible remaining in this state for decades. Biodiversity drops to only one or two species which are inedible for geese, such as Senecio congestus, Salicornia borealis and Atriplex hastata. Because grazing occurs in serial stages, with biodiversity decreasing at each stage, floral composition may be used as an indicator of the degree of goose foraging at a site. Other effects are destruction of the vegetation holding dunes in place, the shift from sedge meadows and grassy swards with herbaceous plants to moss fields, which can eventually give way to bare ground called 'peat barrens', and the erosion of this bare peat until glacial gravel and till is bared. In the High Arctic research is less developed: Eriophorum scheuchzeri and E. angustifolium fens appear to be affected, and are being replaced by carpets of moss, whereas meadows covered in Dupontia fisheri appear to be escaping destruction. There does not appear to be the damage found at lower latitudes in the Arctic. There is little proper research in effects on other birds. The yellow rail (Coturnicops noveboracensis) appears to be extirpated from areas of Manitoba due habitat loss caused by the geese, whereas on the other hand the semipalmated plover (Charadrius semipalmatus) appears to be taking advantage of the large areas of dead willows as a breeding ground.

In the wintering grounds in continental USA, effects are much less pronounced. Experimentally excluding geese by means of fencing in North Carolina has found heavily affected areas can regenerate after only two years. Bulrush stands (Schoenoplectus americanus) are still an important component of the diet, but there are indications the bulrush is being impacted, with soft mudflats gradually replacing areas where it grows.

Damage to agriculture is primarily to seedlings, winter wheat and hay production. Changing the species composition to species less palatable to geese, such as Lotus may alleviate losses in hay operations. Geese also feed on agricultural land without causing economic loss, gleaning seeds from corn, soya or other grains and feeding on wheat, potato and corn stubble. In Québec crop damage insurance for the hay industry began in 1992 and claims increased yearly; actual compensation paid by the government, including administrative costs, amount to some half a million dollars a year.

The fact that Arctic regions are remote, there is little public understanding for combatting the problem, and ecologists as yet do not have any effective solutions for combatting the problem anyway. In Canada, the most important hunters of geese are the Cree people around Hudson Bay, members of the Mushkegowuk Harvesters Association, with an average kill rate of up to 60.75 birds per species per hunter in the 1970s. Kill rates have dropped, with hunters taking only half as much in the 1990s. However, total numbers of kills have increased, i.e. there are more hunters, but they are killing less per person. Nonetheless, per household the kills are approximately the same, at 100 birds. This indicates that stimulating an increase in native hunting might be difficult to achieve. The Cree population has increased. Elders say the taste of the birds has gotten worse, and they are thinner, both possibly effects due to the overpopulation. Elders also say that hunting has gotten more difficult, because there are less young and goslings, which are more likely to fall for decoys. Inuit peoples and other peoples to the north do much less hunting of geese, with kill rates of 1 to 24 per species per hunter. Per kilogram, hunters save some $8.14 to $11.40 from buying poultry at stores. Total kill numbers from hunters elsewhere in the USA and southern Canada has been falling steadily. This is blamed on a decline in people interested in hunting, more feeding areas for the birds, and larger flocks with more experienced adult birds which makes decoying difficult. Individual hunters are bagging higher numbers, compensating lower hunter numbers.

Management strategies in the USA include increasing the bag limit and the number of open hunting days, goose egg addling, trapping and relocation, and egg and nest destruction, managing habitat to make it less attractive to geese, harassment and direct culling. In Denver, Colorado, during moulting season biologists rounded up 300Canada geese (of 5,000 in the city), ironically on Canada Day, killing them and distributing the meat to needy families (as opposed to sending it to a landfill), to try to curb the number of geese, following such programs in New York, Pennsylvania, Oregon and Maryland. Complaints about the birds were that they had taken over the golf courses, pooped all over the place, devoured native plants and scared citizens. Such culls have proven socially controversial, with intense backlash by some citizens. Park officials had tried dipping eggs in oil, using noise-makers and planting tall plants, but this was not sufficient.

In Russia, the problem does not seem to exist, likely due to human harvest and local long-term cooling climate trends in the Russian Far East and Wrangel Island.

It is also possible that the population growth is completely natural, and that when the carrying capacity of the environment is reached the population will stop growing. For organisations such as Ducks Unlimited, the resurgence of goose populations in North America can be called one of the greatest success story in wildlife management. By 2003 the US goose harvest was approaching 4 million, three times the numbers 30 years ago.

Pets

Overpopulation in domestic pets is the surplus of pets, such as cats, dogs, and exotic animals. In the United States, six to eight million animals are brought to shelters each year, of which an estimated three to four million are subsequently euthanized, including 2.7 million considered healthy and adoptable. Euthanasia numbers have declined since the 1970s, when U.S. shelters euthanized an estimated 12 to 20 million animals. Most humane societies, animal shelters and rescue groups urge animal caregivers to have their animals spayed or neutered to prevent the births of unwanted and accidental litters that could contribute to this dynamic.

In the United States, over half of the households own a dog or a cat. Even with so much pet ownership there is still an issue with pet overpopulation, especially seen in shelters. Because of this problem it is estimated that between 10 and 25 percent of dogs and cats are killed yearly. The animals are killed humanely, but the goal is to greatly lower and eventually completely avoid this. Estimating the overpopulation of pets, especially cats and dogs, is a difficult task, but it has been a continuous problem. It has been hard to determine the number of shelters and animals in each shelter around even just the US. Animals are constantly being moved around or euthanized, so it is difficult to keep track of those numbers across the country. It is becoming universally agreed upon that sterilization is a tool that can help reduce population size so that less offspring are produced in the future With less offspring, pet populations can start to decrease which reduces the amount that get killed each year.

Population cycles

Main articles: Population cycle and Lotka–Volterra equations

In the wild, rampant population growth of prey species often causes growth in the populations of predators. Such predator-prey relationships can form cycles, which are usually mathematically modelled as Lotka–Volterra equations.

In natural ecosystems, predator population growth lags just behind the prey populations. After the prey population crashes, the overpopulation of predators causes the entire population to be subjected to mass starvation. The population of the predator drops, as less young are able to survive into adulthood. This could be considered a perfect time for wildlife managers to allow hunters or trappers to harvest as much of these animals as necessary, for example lynx in Canada, although on the other hand this may impact the ability of the predator to rebound when the prey population begins to exponentially increase again. Such mathematical models are also crucial in determining the amount of fish which may be sustainably harvested in fisheries, this is known as the maximum sustainable yield.

Predator population growth has the effect of controlling the prey population, and can result in the evolution of prey species in favour of genetic characteristics that render it less vulnerable to predation (and the predator may co-evolve, in response).

In the absence of predators, species are bound by the resources they can find in their environment, but this does not necessarily control overpopulation, at least in the short term. An abundant supply of resources can produce a population boom followed by a population crash. Rodents such as lemmings and voles have such population cycles of rapid growth and subsequent decrease. Snowshoe hares populations similarly cycle dramatically, as did those of one of their predators, the lynx. Another example is the cycles among populations of grey wolves and moose in Isle Royale National Park. For some still unexplained reason, such patterns in mammal population dynamics are more prevalent in ecosystems found at more arctic latitudes.

Some species such as locusts experience large natural cyclic variations, experienced by farmers as plagues.

Determining population size/density

When  determining whether a population is overpopulated a variety of factors must be looked at. Given complexity of the issue, often it is determined by scientist and wildlife management as to what constitutes such a claim. In many cases scientists will look to food sources and living space to gauge the abundance of a species in a particular area. National parks collect extensive data on the activities and quality of the environment they are established in. This data can be used to track whether a specific species is consuming larger amounts of their desired food source over time.

This is done typically in four ways, the first being “total counting”. Researchers will use aerial photography to count large populations in a specific area such as deer, waterfowl, and other “flocking” or “herd” animals. Incomplete counts involve counting a small subsection of a population and extrapolating the data across the whole area. This method will take into account the behavior of the animals such as how much territory a herd may cover, the density of the population, and other potential factors that may come into question.

The third method is “indirect counts” ; this is done by looking at the environment for signs of animal presence. Typically done by counting fecal matter or dens/nesting of a particular animal. This method is not an accurate method, but gives general counts of a population in a specific locale.

Lastly the method of mark-recapture is used extensively to determine general population sizes. This method involves the trapping of animals after which some form of tag is placed on the animal and it is released back into the wild. After which, other trappings will determine population size based on the number of marked versus unmarked animals.

Fish populations

similar methods can be used to determine the population of fish however some key differences arise in the extrapolation of data. Unlike many land animals in-land fish populations are divided into smaller population sizes. Factors such as migration may not be relevant when determining population in a specific locales while more important for others such as the many species of salmon or trout. Monitoring of waterways and isolated bodies of water provide more frequently updated information on the populations in specific areas. This is done using similar methods to the mark-recapture methods of many land animals.

Introduced species

Main articles: Introduced species and Invasive species

The introduction of a foreign species has often caused ecological disturbance, such as when deer and trout were introduced into Argentina, or when rabbits were introduced to Australia and when predators were introduced in turn to attempt to control the rabbits.

When an introduced species is so successful that its population begins to increase exponentially and causes deleterious effects to farmers, fisheries or the natural environment, these introduced species are called invasive species.

In the case of the Mute swan, Cygnus olor, their population has rapidly spread across much of North America as well as parts of Canada and western Europe. This species of swan has caused much concern for wildlife management as they damage aquatic vegetation, and harass other waterfowl, dislocating them. The population of the Mute swan has seen an average increase of around 10-18% per year which further threatens to impact the areas they inhabit. Management of the species comes in a variety of ways. Similar to overpopulated or invasive species, hunting is one of the most effective methods of population control. Other methods may involve trapping, relocation, or euthanasia.

Criticism

In natural ecosystems, populations naturally expand until they reach the carrying capacity of the environment; if the resources on which they depend are exhausted, they naturally collapse. According to the animal rights movement, calling this an 'overpopulation' is more an ethics question than a scientific fact. Animal rights organisations are commonly critics of ecological systems and wildlife management. Animal rights activists and locals earning income from commercial hunts counter that scientists are outsiders who do not know wildlife issues, and that any slaughter of animals is evil.

Various case studies indicate that use of cattle as 'natural grazers' in many European nature parks due to absence of hunting, culling or natural predators (such as wolves),may cause an overpopulation because the cattle do not migrate. This has the effect of reducing plant biodiversity, as the cattle consume native plants. Because such cattle populations begin to starve and die in the winter as available forage drops, this has caused animal rights activists to advocate supplemental feeding, which has the effect of exacerbating the ecological effects, causing nitrification and eutrophication due to excess faeces, deforestation as trees are destroyed, and biodiversity loss.

Despite the ecological effects of overpopulation, wildlife managers may want such high populations in order to satisfy public enjoyment of seeing wild animals. Others contend that introducing large predators such as lynx and wolves may have similar economic benefits, even if tourists rarely actually catch glimpses of such creatures.

In regards to population size, most of the methods used give estimates that vary in accuracy to the actual size and density of the population. Criticisms of theses methods generally fall onto the efficacy of methods used.

Human overpopulation

Main article: Human overpopulation

Overpopulation can result from an increase in births, a decline in mortality rates against the background of high fertility rates. It is possible for very sparsely populated areas to be overpopulated if the area has a meagre or non-existent capability to sustain life (e.g. a desert). Advocates of population moderation cite issues like quality of life and risk of starvation and disease as a basis to argue against continuing high human population growth and for population decline.