Cryogenics

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Cryogenics

Nitrogen is a liquid under −195.8 °C (77.3 K).

In physics, cryogenics is the production and behaviour of materials at very low temperatures.

The 13th International Institute of Refrigeration's (IIR) International Congress of Refrigeration (held in Washington DC in 1971) endorsed a universal definition of "cryogenics" and "cryogenic" by accepting a threshold of 120 K (−153 °C) to distinguish these terms from conventional refrigeration. This is a logical dividing line, since the normal boiling points of the so-called permanent gases (such as helium, hydrogen, neon, nitrogen, oxygen, and normal air) lie below 120 K, while the Freon refrigerants, hydrocarbons, and other common refrigerants have boiling points above 120 K.

Discovery of superconducting materials with critical temperatures significantly above the boiling point of nitrogen has provided new interest in reliable, low-cost methods of producing high-temperature cryogenic refrigeration. The term "high temperature cryogenic" describes temperatures ranging from above the boiling point of liquid nitrogen, −195.79 °C (77.36 K; −320.42 °F), up to −50 °C (223 K; −58 °F).^[7] The discovery of superconductive properties is first attributed to Heike Kamerlingh Onnes on July 10, 1908. The discovery came after the ability to reach a temperature of 2 K. These first superconductive properties were observed in mercury at a temperature of 4.2 K.

Cryogenicists use the Kelvin or Rankine temperature scale, both of which measure from absolute zero, rather than more usual scales such as Celsius which measures from the freezing point of water at sea level or Fahrenheit which measures from the freezing point of a particular brine solution at sea level.

Definitions and distinctions

Cryogenics

The branches of engineering that involve the study of very low temperatures (ultra low temperature i.e. below 123 K), how to produce them, and how materials behave at those temperatures.

Cryobiology

The branch of biology involving the study of the effects of low temperatures on organisms (most often for the purpose of achieving cryopreservation). Other applications include Lyophilization (freeze-drying) of pharmaceutical components and medicine.

Cryoconservation of animal genetic resources

The conservation of genetic material with the intention of conserving a breed. The conservation of genetic material is not limited to non-humans. Many services provide genetic storage or the preservation of stem cells at birth. They may be used to study the generation of cell lines or for stem-cell therapy.

Cryosurgery

The branch of surgery applying cryogenic temperatures to destroy and kill tissue, e.g. cancer cells. Commonly referred to as Cryoablation.

Cryoelectronics

The study of electronic phenomena at cryogenic temperatures. Examples include superconductivity and variable-range hopping.

Cryonics

Cryopreserving humans and animals with the intention of future revival. "Cryogenics" is sometimes erroneously used to mean "Cryonics" in popular culture and the press.

Etymology

The word cryogenics stems from Greek κρύος (cryos) – "cold" + γενής (genis) – "generating".

Cryogenic fluids

This is a diagram of an infrared space telescope that needs a cold mirror and instruments. One instrument needs to be even colder, and it has a cryocooler. The instrument is in region 1 and its cryocooler is in region 3 in a warmer region of the spacecraft (see MIRI (Mid-Infrared Instrument) or James Webb Space Telescope).

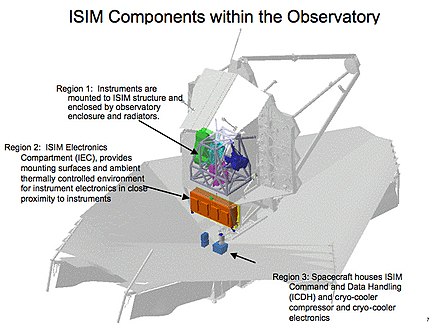
Cryogenic fluids with their boiling point in Kelvin and degree Celsius.

Fluid	Boiling point (K)	Boiling point (°C)
Helium-3	3.19	−269.96
Helium-4	4.214	−268.936
Hydrogen	20.27	−252.88
Neon	27.09	−246.06
Nitrogen	77.09	−196.06
Air	78.8	−194.35
Fluorine	85.24	−187.91
Argon	87.24	−185.91
Oxygen	90.18	−182.97
Methane	111.7	−161.45
Krypton	119.93	−153.415

Industrial applications

A medium-sized dewar is being filled with liquid nitrogen by a larger cryogenic storage tank.

Catalogue image of a cryogenic valve

 

Cryogenic valves in situ, heavily frozen from condensed atmospheric humidity

 

Further information: Low-temperature technology timeline

Liquefied gases, such as liquid nitrogen and liquid helium, are used in many cryogenic applications. Liquid nitrogen is the most commonly used element in cryogenics and is legally purchasable around the world. Liquid helium is also commonly used and allows for the lowest attainable temperatures to be reached.

These liquids may be stored in Dewar flasks, which are double-walled containers with a high vacuum between the walls to reduce heat transfer into the liquid. Typical laboratory Dewar flasks are spherical, made of glass and protected in a metal outer container. Dewar flasks for extremely cold liquids such as liquid helium have another double-walled container filled with liquid nitrogen. Dewar flasks are named after their inventor, James Dewar, the man who first liquefied hydrogen. Thermos bottles are smaller vacuum flasks fitted in a protective casing.

Cryogenic barcode labels are used to mark Dewar flasks containing these liquids, and will not frost over down to −195 degrees Celsius.

Cryogenic transfer pumps are the pumps used on LNG piers to transfer liquefied natural gas from LNG carriers to LNG storage tanks, as are cryogenic valves.

Cryogenic processing

The field of cryogenics advanced during World War II when scientists found that metals frozen to low temperatures showed more resistance to wear. Based on this theory of cryogenic hardening, the commercial cryogenic processing industry was founded in 1966 by Bill and Ed Busch. With a background in the heat treating industry, the Busch brothers founded a company in Detroit called CryoTech in 1966. Busch originally experimented with the possibility of increasing the life of metal tools to anywhere between 200% and 400% of the original life expectancy using cryogenic tempering instead of heat treating.^{[citation needed]} This evolved in the late 1990s into the treatment of other parts.

Cryogens, such as liquid nitrogen, are further used for specialty chilling and freezing applications. Some chemical reactions, like those used to produce the active ingredients for the popular statin drugs, must occur at low temperatures of approximately −100 °C (−148 °F). Special cryogenic chemical reactors are used to remove reaction heat and provide a low temperature environment. The freezing of foods and biotechnology products, like vaccines, requires nitrogen in blast freezing or immersion freezing systems. Certain soft or elastic materials become hard and brittle at very low temperatures, which makes cryogenic milling (cryomilling) an option for some materials that cannot easily be milled at higher temperatures.

Cryogenic processing is not a substitute for heat treatment, but rather an extension of the heating–quenching–tempering cycle. Normally, when an item is quenched, the final temperature is ambient. The only reason for this is that most heat treaters do not have cooling equipment. There is nothing metallurgically significant about ambient temperature. The cryogenic process continues this action from ambient temperature down to −320 °F (140 °R; 78 K; −196 °C). In most instances the cryogenic cycle is followed by a heat tempering procedure. As all alloys do not have the same chemical constituents, the tempering procedure varies according to the material's chemical composition, thermal history and/or a tool's particular service application.

The entire process takes 3–4 days.

Fuels

Another use of cryogenics is cryogenic fuels for rockets with liquid hydrogen as the most widely used example. Liquid oxygen (LOX) is even more widely used but as an oxidizer, not a fuel. NASA's workhorse Space Shuttle used cryogenic hydrogen/oxygen propellant as its primary means of getting into orbit. LOX is also widely used with RP-1 kerosene, a non-cryogenic hydrocarbon, such as in the rockets built for the Soviet space program by Sergei Korolev.

Russian aircraft manufacturer Tupolev developed a version of its popular design Tu-154 with a cryogenic fuel system, known as the Tu-155. The plane uses a fuel referred to as liquefied natural gas or LNG, and made its first flight in 1989.

Other applications

Astronomical instruments on the Very Large Telescope are equipped with continuous-flow cooling systems.

Some applications of cryogenics:

• Nuclear magnetic resonance (NMR) is one of the most common methods to determine the physical and chemical properties of atoms by detecting the radio frequency absorbed and subsequent relaxation of nuclei in a magnetic field. This is one of the most commonly used characterization techniques and has applications in numerous fields. Primarily, the strong magnetic fields are generated by supercooling electromagnets, although there are spectrometers that do not require cryogens. In traditional superconducting solenoids, liquid helium is used to cool the inner coils because it has a boiling point of around 4 K at ambient pressure. Inexpensive metallic superconductors can be used for the coil wiring. So-called high-temperature superconducting compounds can be made to super conduct with the use of liquid nitrogen, which boils at around 77 K.
• Magnetic resonance imaging (MRI) is a complex application of NMR where the geometry of the resonances is deconvoluted and used to image objects by detecting the relaxation of protons that have been perturbed by a radio-frequency pulse in the strong magnetic field. This is most commonly used in health applications.
• Cryogenic electron microscopy (cryoEM) is a popular method in structural biology for elucidating the structures of proteins, cells, and other biological systems. Samples are plunge-frozen into a cryogen such as liquid ethane cooled by liquid nitrogen, and are then kept at liquid nitrogen temperature as they are inserted into an electron microscope for imaging. Electron microscopes are also themselves cooled by liquid nitrogen.
• In large cities, it is difficult to transmit power by overhead cables, so underground cables are used. But underground cables get heated and the resistance of the wire increases, leading to waste of power. Superconductors could be used to increase power throughput, although they would require cryogenic liquids such as nitrogen or helium to cool special alloy-containing cables to increase power transmission. Several feasibility studies have been performed and the field is the subject of an agreement within the International Energy Agency.

Cryogenic gases delivery truck at a supermarket, Ypsilanti, Michigan

• Cryogenic gases are used in transportation and storage of large masses of frozen food. When very large quantities of food must be transported to regions like war zones, earthquake hit regions, etc., they must be stored for a long time, so cryogenic food freezing is used. Cryogenic food freezing is also helpful for large scale food processing industries.
• Many infrared (forward looking infrared) cameras require their detectors to be cryogenically cooled.
• Certain rare blood groups are stored at low temperatures, such as −165°C, at blood banks.
• Cryogenics technology using liquid nitrogen and CO₂ has been built into nightclub effect systems to create a chilling effect and white fog that can be illuminated with colored lights.
• Cryogenic cooling is used to cool the tool tip at the time of machining in manufacturing process. It increases the tool life. Oxygen is used to perform several important functions in the steel manufacturing process.
• Many rockets and lunar landers use cryogenic gases as propellants. These include liquid oxygen, liquid hydrogen, and liquid methane.
• By freezing an automobile or truck tire in liquid nitrogen, the rubber is made brittle and can be crushed into small particles. These particles can be used again for other items.
• Experimental research on certain physics phenomena, such as spintronics and magnetotransport properties, requires cryogenic temperatures for the effects to be observable.
• Certain vaccines must be stored at cryogenic temperatures. For example, the Pfizer–BioNTech COVID-19 vaccine must be stored at temperatures of −90 to −60 °C (−130 to −76 °F). (See cold chain.)

Production

This section does not cite any sources. Please help improve this section by adding citations to reliable sources. Unsourced material may be challenged and removed. (March 2023) (Learn how and when to remove this message)

Cryogenic cooling of devices and material is usually achieved via the use of liquid nitrogen, liquid helium, or a mechanical cryocooler (which uses high-pressure helium lines). Gifford-McMahon cryocoolers, pulse tube cryocoolers and Stirling cryocoolers are in wide use with selection based on required base temperature and cooling capacity. The most recent development in cryogenics is the use of magnets as regenerators as well as refrigerators. These devices work on the principle known as the magnetocaloric effect.

Detectors

There are various cryogenic detectors which are used to detect particles.

For cryogenic temperature measurement down to 30 K, Pt100 sensors, a resistance temperature detector (RTD), are used. For temperatures lower than 30 K, it is necessary to use a silicon diode for accuracy.

Squaring the circle

From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Squaring_the_circle

Squaring the circle: the areas of this square and this circle are both equal to π. In 1882, it was proven that this figure cannot be constructed in a finite number of steps with an idealized compass and straightedge.

Squaring the circle is a problem in geometry first proposed in Greek mathematics. It is the challenge of constructing a square with the area of a given circle by using only a finite number of steps with a compass and straightedge. The difficulty of the problem raised the question of whether specified axioms of Euclidean geometry concerning the existence of lines and circles implied the existence of such a square.

In 1882, the task was proven to be impossible, as a consequence of the Lindemann–Weierstrass theorem, which proves that pi (${\displaystyle \pi }$) is a transcendental number. That is, ${\displaystyle \pi }$ is not the root of any polynomial with rational coefficients. It had been known for decades that the construction would be impossible if ${\displaystyle \pi }$ were transcendental, but that fact was not proven until 1882. Approximate constructions with any given non-perfect accuracy exist, and many such constructions have been found.

Despite the proof that it is impossible, attempts to square the circle have been common in pseudomathematics (i.e. the work of mathematical cranks). The expression "squaring the circle" is sometimes used as a metaphor for trying to do the impossible.

The term quadrature of the circle is sometimes used as a synonym for squaring the circle. It may also refer to approximate or numerical methods for finding the area of a circle. In general, quadrature or squaring may also be applied to other plane figures.

History

Methods to calculate the approximate area of a given circle, which can be thought of as a precursor problem to squaring the circle, were known already in many ancient cultures. These methods can be summarized by stating the approximation to π that they produce. In around 2000 BCE, the Babylonian mathematicians used the approximation ${\displaystyle \pi \approx \frac{25}{8}=3.125}$, and at approximately the same time the ancient Egyptian mathematicians used ${\displaystyle \pi \approx \frac{256}{81}\approx 3.16}$. Over 1000 years later, the Old Testament Books of Kings used the simpler approximation ${\displaystyle \pi \approx 3}$. Ancient Indian mathematics, as recorded in the Shatapatha Brahmana and Shulba Sutras, used several different approximations to ${\displaystyle \pi }$. Archimedes proved a formula for the area of a circle, according to which ${\displaystyle 3\frac{10}{71}\approx 3.141<\pi <3\frac{1}{7}\approx 3.143}$. In Chinese mathematics, in the third century CE, Liu Hui found even more accurate approximations using a method similar to that of Archimedes, and in the fifth century Zu Chongzhi found ${\displaystyle \pi \approx 355/113\approx 3.141593}$, an approximation known as Milü.

The problem of constructing a square whose area is exactly that of a circle, rather than an approximation to it, comes from Greek mathematics. Greek mathematicians found compass and straightedge constructions to convert any polygon into a square of equivalent area. They used this construction to compare areas of polygons geometrically, rather than by the numerical computation of area that would be more typical in modern mathematics. As Proclus wrote many centuries later, this motivated the search for methods that would allow comparisons with non-polygonal shapes:

Having taken their lead from this problem, I believe, the ancients also sought the quadrature of the circle. For if a parallelogram is found equal to any rectilinear figure, it is worthy of investigation whether one can prove that rectilinear figures are equal to figures bound by circular arcs.

Some apparent partial solutions gave false hope for a long time. In this figure, the shaded figure is the lune of Hippocrates. Its area is equal to the area of the triangle ABC (found by Hippocrates of Chios).

The first known Greek to study the problem was Anaxagoras, who worked on it while in prison. Hippocrates of Chios attacked the problem by finding a shape bounded by circular arcs, the lune of Hippocrates, that could be squared. Antiphon the Sophist believed that inscribing regular polygons within a circle and doubling the number of sides would eventually fill up the area of the circle (this is the method of exhaustion). Since any polygon can be squared, he argued, the circle can be squared. In contrast, Eudemus argued that magnitudes can be divided up without limit, so the area of the circle would never be used up. Contemporaneously with Antiphon, Bryson of Heraclea argued that, since larger and smaller circles both exist, there must be a circle of equal area; this principle can be seen as a form of the modern intermediate value theorem. The more general goal of carrying out all geometric constructions using only a compass and straightedge has often been attributed to Oenopides, but the evidence for this is circumstantial.

The problem of finding the area under an arbitrary curve, now known as integration in calculus, or quadrature in numerical analysis, was known as squaring before the invention of calculus. Since the techniques of calculus were unknown, it was generally presumed that a squaring should be done via geometric constructions, that is, by compass and straightedge. For example, Newton wrote to Oldenburg in 1676 "I believe M. Leibnitz will not dislike the theorem towards the beginning of my letter pag. 4 for squaring curve lines geometrically". In modern mathematics the terms have diverged in meaning, with quadrature generally used when methods from calculus are allowed, while squaring the curve retains the idea of using only restricted geometric methods.

A 1647 attempt at squaring the circle, Opus geometricum quadraturae circuli et sectionum coni decem libris comprehensum by Grégoire de Saint-Vincent, was heavily criticized by Vincent Léotaud. Nevertheless, de Saint-Vincent succeeded in his quadrature of the hyperbola, and in doing so was one of the earliest to develop the natural logarithm. James Gregory, following de Saint-Vincent, attempted another proof of the impossibility of squaring the circle in Vera Circuli et Hyperbolae Quadratura (The True Squaring of the Circle and of the Hyperbola) in 1667. Although his proof was faulty, it was the first paper to attempt to solve the problem using algebraic properties of ${\displaystyle \pi }$. Johann Heinrich Lambert proved in 1761 that ${\displaystyle \pi }$ is an irrational number. It was not until 1882 that Ferdinand von Lindemann succeeded in proving more strongly that π is a transcendental number, and by doing so also proved the impossibility of squaring the circle with compass and straightedge.

After Lindemann's impossibility proof, the problem was considered to be settled by professional mathematicians, and its subsequent mathematical history is dominated by pseudomathematical attempts at circle-squaring constructions, largely by amateurs, and by the debunking of these efforts. As well, several later mathematicians including Srinivasa Ramanujan developed compass and straightedge constructions that approximate the problem accurately in few steps.

Two other classical problems of antiquity, famed for their impossibility, were doubling the cube and trisecting the angle. Like squaring the circle, these cannot be solved by compass and straightedge. However, they have a different character than squaring the circle, in that their solution involves the root of a cubic equation, rather than being transcendental. Therefore, more powerful methods than compass and straightedge constructions, such as neusis construction or mathematical paper folding, can be used to construct solutions to these problems.

Impossibility

The solution of the problem of squaring the circle by compass and straightedge requires the construction of the number ${\displaystyle \sqrt{\pi }}$, the length of the side of a square whose area equals that of a unit circle. If ${\displaystyle \sqrt{\pi }}$ were a constructible number, it would follow from standard compass and straightedge constructions that ${\displaystyle \pi }$ would also be constructible. In 1837, Pierre Wantzel showed that lengths that could be constructed with compass and straightedge had to be solutions of certain polynomial equations with rational coefficients. Thus, constructible lengths must be algebraic numbers. If the circle could be squared using only compass and straightedge, then ${\displaystyle \pi }$ would have to be an algebraic number. It was not until 1882 that Ferdinand von Lindemann proved the transcendence of ${\displaystyle \pi }$ and so showed the impossibility of this construction. Lindemann's idea was to combine the proof of transcendence of Euler's number ${\displaystyle e}$, shown by Charles Hermite in 1873, with Euler's identity $${\displaystyle e^{i\pi }=-1.}$$ This identity immediately shows that ${\displaystyle \pi }$ is an irrational number, because a rational power of a transcendental number remains transcendental. Lindemann was able to extend this argument, through the Lindemann–Weierstrass theorem on linear independence of algebraic powers of ${\displaystyle e}$, to show that ${\displaystyle \pi }$ is transcendental and therefore that squaring the circle is impossible.

Bending the rules by introducing a supplemental tool, allowing an infinite number of compass-and-straightedge operations or by performing the operations in certain non-Euclidean geometries makes squaring the circle possible in some sense. For example, Dinostratus' theorem uses the quadratrix of Hippias to square the circle, meaning that if this curve is somehow already given, then a square and circle of equal areas can be constructed from it. The Archimedean spiral can be used for another similar construction. Although the circle cannot be squared in Euclidean space, it sometimes can be in hyperbolic geometry under suitable interpretations of the terms. The hyperbolic plane does not contain squares (quadrilaterals with four right angles and four equal sides), but instead it contains regular quadrilaterals, shapes with four equal sides and four equal angles sharper than right angles. There exist in the hyperbolic plane (countably) infinitely many pairs of constructible circles and constructible regular quadrilaterals of equal area, which, however, are constructed simultaneously. There is no method for starting with an arbitrary regular quadrilateral and constructing the circle of equal area. Symmetrically, there is no method for starting with an arbitrary circle and constructing a regular quadrilateral of equal area, and for sufficiently large circles no such quadrilateral exists.

Approximate constructions

Although squaring the circle exactly with compass and straightedge is impossible, approximations to squaring the circle can be given by constructing lengths close to ${\displaystyle \pi }$. It takes only elementary geometry to convert any given rational approximation of ${\displaystyle \pi }$ into a corresponding compass and straightedge construction, but such constructions tend to be very long-winded in comparison to the accuracy they achieve. After the exact problem was proven unsolvable, some mathematicians applied their ingenuity to finding approximations to squaring the circle that are particularly simple among other imaginable constructions that give similar precision.

Construction by Kochański

Kochański's approximate construction

 

Continuation with equal-area circle and square; ${\displaystyle r}$ denotes the initial radius

One of many early historical approximate compass-and-straightedge constructions is from a 1685 paper by Polish Jesuit Adam Adamandy Kochański, producing an approximation diverging from ${\displaystyle \pi }$ in the 5th decimal place. Although much more precise numerical approximations to ${\displaystyle \pi }$ were already known, Kochański's construction has the advantage of being quite simple. In the left diagram $${\displaystyle |P_3{P}_9|=|P_1{P}_2|\sqrt{\frac{40}{3}-2\sqrt{3}}\approx 3.141533338\cdot |P_1{P}_2|\approx \pi r.}$$ In the same work, Kochański also derived a sequence of increasingly accurate rational approximations for ${\displaystyle \pi }$.

Constructions using 355/113

Jacob de Gelder's 355/113 construction

Ramanujan's 355/113 construction

Jacob de Gelder published in 1849 a construction based on the approximation $${\displaystyle \pi \approx \frac{355}{113}=3.141592920\dots }$$ This value is accurate to six decimal places and has been known in China since the 5th century as Milü, and in Europe since the 17th century.

Gelder did not construct the side of the square; it was enough for him to find the value $${\displaystyle \overline{AH}=\frac{4^2}{7^2+8^2}.}$$ The illustration shows de Gelder's construction.

In 1914, Indian mathematician Srinivasa Ramanujan gave another geometric construction for the same approximation.

Constructions using the golden ratio

Hobson's golden ratio construction

 

Dixon's golden ratio construction

 

Beatrix's 13-step construction

An approximate construction by E. W. Hobson in 1913 is accurate to three decimal places. Hobson's construction corresponds to an approximate value of $${\displaystyle \frac{6}{5}\cdot \left(1+\phi \right)=3.141640\dots ,}$$ where ${\displaystyle \phi }$ is the golden ratio, ${\displaystyle \phi =(1+\sqrt{5})/2}$.

The same approximate value appears in a 1991 construction by Robert Dixon. In 2022 Frédéric Beatrix presented a geometrographic construction in 13 steps.

Second construction by Ramanujan

Squaring the circle, approximate construction according to Ramanujan of 1914, with continuation of the construction (dashed lines, mean proportional red line), see animation.

 

Sketch of "Manuscript book 1 of Srinivasa Ramanujan" p. 54, Ramanujan's 355/113 construction

In 1914, Ramanujan gave a construction which was equivalent to taking the approximate value for ${\displaystyle \pi }$ to be $${\displaystyle {\left(9^2+\frac{{19}^2}{22}\right)}^{\frac{1}{4}}=\sqrt[4]{\frac{2143}{22}}=3.141592652582\dots }$$ giving eight decimal places of ${\displaystyle \pi }$. He describes the construction of line segment OS as follows.

Let AB (Fig.2) be a diameter of a circle whose centre is O. Bisect the arc ACB at C and trisect AO at T. Join BC and cut off from it CM and MN equal to AT. Join AM and AN and cut off from the latter AP equal to AM. Through P draw PQ parallel to MN and meeting AM at Q. Join OQ and through T draw TR, parallel to OQ and meeting AQ at R. Draw AS perpendicular to AO and equal to AR, and join OS. Then the mean proportional between OS and OB will be very nearly equal to a sixth of the circumference, the error being less than a twelfth of an inch when the diameter is 8000 miles long.

Incorrect constructions

In his old age, the English philosopher Thomas Hobbes convinced himself that he had succeeded in squaring the circle, a claim refuted by John Wallis as part of the Hobbes–Wallis controversy. During the 18th and 19th century, the false notions that the problem of squaring the circle was somehow related to the longitude problem, and that a large reward would be given for a solution, became prevalent among would-be circle squarers. In 1851, John Parker published a book Quadrature of the Circle in which he claimed to have squared the circle. His method actually produced an approximation of ${\displaystyle \pi }$ accurate to six digits.

The Victorian-age mathematician, logician, and writer Charles Lutwidge Dodgson, better known by his pseudonym Lewis Carroll, also expressed interest in debunking illogical circle-squaring theories. In one of his diary entries for 1855, Dodgson listed books he hoped to write, including one called "Plain Facts for Circle-Squarers". In the introduction to "A New Theory of Parallels", Dodgson recounted an attempt to demonstrate logical errors to a couple of circle-squarers, stating:

The first of these two misguided visionaries filled me with a great ambition to do a feat I have never heard of as accomplished by man, namely to convince a circle squarer of his error! The value my friend selected for Pi was 3.2: the enormous error tempted me with the idea that it could be easily demonstrated to BE an error. More than a score of letters were interchanged before I became sadly convinced that I had no chance.

A ridiculing of circle squaring appears in Augustus De Morgan's book A Budget of Paradoxes, published posthumously by his widow in 1872. Having originally published the work as a series of articles in The Athenæum, he was revising it for publication at the time of his death. Circle squaring declined in popularity after the nineteenth century, and it is believed that De Morgan's work helped bring this about.

Heisel's 1934 book

Even after it had been proved impossible, in 1894, amateur mathematician Edwin J. Goodwin claimed that he had developed a method to square the circle. The technique he developed did not accurately square the circle, and provided an incorrect area of the circle which essentially redefined ${\displaystyle \pi }$ as equal to 3.2. Goodwin then proposed the Indiana pi bill in the Indiana state legislature allowing the state to use his method in education without paying royalties to him. The bill passed with no objections in the state house, but the bill was tabled and never voted on in the Senate, amid increasing ridicule from the press.

The mathematical crank Carl Theodore Heisel also claimed to have squared the circle in his 1934 book, "Behold! : the grand problem no longer unsolved: the circle squared beyond refutation." Paul Halmos referred to the book as a "classic crank book."

In literature

The problem of squaring the circle has been mentioned over a wide range of literary eras, with a variety of metaphorical meanings. Its literary use dates back at least to 414 BC, when the play The Birds by Aristophanes was first performed. In it, the character Meton of Athens mentions squaring the circle, possibly to indicate the paradoxical nature of his utopian city.

Vitruvian Man

Dante's Paradise, canto XXXIII, lines 133–135, contain the verse:

As the geometer his mind applies
To square the circle, nor for all his wit
Finds the right formula, howe'er he tries

Qual è ’l geométra che tutto s’affige
per misurar lo cerchio, e non ritrova,
pensando, quel principio ond’elli indige,

For Dante, squaring the circle represents a task beyond human comprehension, which he compares to his own inability to comprehend Paradise. Dante's image also calls to mind a passage from Vitruvius, famously illustrated later in Leonardo da Vinci's Vitruvian Man, of a man simultaneously inscribed in a circle and a square. Dante uses the circle as a symbol for God, and may have mentioned this combination of shapes in reference to the simultaneous divine and human nature of Jesus. Earlier, in canto XIII, Dante calls out Greek circle-squarer Bryson as having sought knowledge instead of wisdom.

Several works of 17th-century poet Margaret Cavendish elaborate on the circle-squaring problem and its metaphorical meanings, including a contrast between unity of truth and factionalism, and the impossibility of rationalizing "fancy and female nature". By 1742, when Alexander Pope published the fourth book of his Dunciad, attempts at circle-squaring had come to be seen as "wild and fruitless":

Mad Mathesis alone was unconfined,
Too mad for mere material chains to bind,
Now to pure space lifts her ecstatic stare,
Now, running round the circle, finds it square.

Similarly, the Gilbert and Sullivan comic opera Princess Ida features a song which satirically lists the impossible goals of the women's university run by the title character, such as finding perpetual motion. One of these goals is "And the circle – they will square it/Some fine day."

The sestina, a poetic form first used in the 12th century by Arnaut Daniel, has been said to metaphorically square the circle in its use of a square number of lines (six stanzas of six lines each) with a circular scheme of six repeated words. Spanos (1978) writes that this form invokes a symbolic meaning in which the circle stands for heaven and the square stands for the earth. A similar metaphor was used in "Squaring the Circle", a 1908 short story by O. Henry, about a long-running family feud. In the title of this story, the circle represents the natural world, while the square represents the city, the world of man.

In later works, circle-squarers such as Leopold Bloom in James Joyce's novel Ulysses and Lawyer Paravant in Thomas Mann's The Magic Mountain are seen as sadly deluded or as unworldly dreamers, unaware of its mathematical impossibility and making grandiose plans for a result they will never attain.