Symmetry in biology

From Wikipedia, the free encyclopedia

 

A selection of animals showing a range of possible body symmetries, including both asymmetry, radial and bilateral body plans

Symmetry in biology is the balanced distribution of duplicate body parts or shapes within the body of an organism. In nature and biology, symmetry is always approximate. For example, plant leaves – while considered symmetrical – rarely match up exactly when folded in half. Symmetry creates a class of patterns in nature, where the near-repetition of the pattern element is by reflection or rotation.

The body plans of most multicellular organisms exhibit some form of symmetry, whether radial, bilateral, or spherical. A small minority, notably among the sponges, exhibit no symmetry (i.e., are asymmetric). Symmetry was once important in animal taxonomy; the Radiata, animals with radial symmetry, formed one of the four branches of Georges Cuvier's classification of the animal kingdom.

Radial symmetry

These sea anemones have been painted to emphasize their radial symmetry. (Plate from Ernst Haeckel's Kunstformen der Natur).

Radially symmetric organisms resemble a pie where several cutting planes produce roughly identical pieces. Such an organism exhibits no left or right sides. They have a top and a bottom surface, or a front and a back.

Symmetry has been important historically in the taxonomy of animals; Georges Cuvier classified animals with radial symmetry in the taxon Radiata (Zoophytes),^[1][2] which is now generally accepted to be a polyphyletic assemblage of different phyla of the Animal kingdom.^[3] Most radially symmetric animals are symmetrical about an axis extending from the center of the oral surface, which contains the mouth, to the center of the opposite, aboral, end. Radial symmetry is especially suitable for sessile animals such as the sea anemone, floating animals such as jellyfish, and slow moving organisms such as starfish. Animals in the phyla Cnidaria and Echinodermata are radially symmetric,^[4] although many sea anemones and some corals have bilateral symmetry defined by a single structure, the siphonoglyph.^[5]

Lilium bulbiferum displays typical floral symmetry with repeated parts arranged around the axis of the flower.

Many flowers are radially symmetric or actinomorphic. Roughly identical flower parts – petals, sepals, and stamens – occur at regular intervals around the axis of the flower, which is often the female part, with the carpel, style and stigma.^[6]

Gastroenteritis viruses have radial symmetry, being icosahedral: A rotavirus, B adenovirus, C norovirus, D astrovirus.

Many viruses have radial symmetries, their coats being composed of a relatively small number of protein molecules arranged in a regular pattern to form polyhedrons, spheres, or ovoids. Most are icosahedrons.^[7]

Special forms of radial symmetry

Tetramerism is a variant of radial symmetry found in jellyfish, which have four canals in an otherwise radial body plan.

Apple cut horizontally, showing pentamerism

Pentamerism (also called pentaradial and pentagonal symmetry) means the organism is in five parts around a central axis, 72° apart. Among animals, only the echinoderms such as sea stars, sea urchins, and sea lilies are pentamerous as adults, with five arms arranged around the mouth. Being bilaterian animals, however, they initially develop with mirror symmetry as larvae, then gain pentaradial symmetry later.^[8]

Flowering plants show fivefold symmetry in many flowers and in various fruits. This is well seen in the arrangement of the five carpels (the botanical fruits containing the seeds) in an apple cut transversely.

Hexamerism is found in the corals and sea anemones (class Anthozoa) which are divided into two groups based on their symmetry. The most common corals in the subclass Hexacorallia have a hexameric body plan; their polyps have sixfold internal symmetry and the number of their tentacles is a multiple of six.

Octamerism is found in corals of the subclass Octocorallia. These have polyps with eight tentacles and octameric radial symmetry. The octopus, however, has bilateral symmetry, despite its eight arms.

Spherical symmetry

Volvox is a microscopic green freshwater alga with spherical symmetry. Young colonies can be seen inside the larger ones.

Spherical symmetry occurs in an organism if it is able to be cut into two identical halves through any cut that runs through the organism's center. Organisms which show approximate spherical symmetry include the freshwater green alga Volvox.^[4]

Bilateral symmetry

In bilateral symmetry (also called plane symmetry), only one plane, called the sagittal plane, divides an organism into roughly mirror image halves. Thus there is approximate reflection symmetry. Internal organs are however not necessarily symmetric.

The small emperor moth, Saturnia pavonia, displays a deimatic pattern with bilateral symmetry.

Animals that are bilaterally symmetric have mirror symmetry in the sagittal plane, which divides the body vertically into left and right halves, with one of each sense organ and limb group on either side. At least 99% of animals are bilaterally symmetric, including humans,^[9][10][11] where facial symmetry influences people's judgements of attractiveness.^[12]

When an organism normally moves in one direction, it inevitably has a front or head end. This end encounters the environment before the rest of the body as the organism moves along, so sensory organs such as eyes tend to be clustered there, and similarly it is the likely site for a mouth as food is encountered.^[11] A distinct head, with sense organs connected to a central nervous system, therefore (on this view) tends to develop (cephalization). Given a direction of travel which creates a front/back difference, and gravity which creates a dorsal/ventral difference, left and right are unavoidably distinguished, so a bilaterally symmetric body plan is widespread and found in most animal phyla.^[11][13] Bilateral symmetry also permits streamlining to reduce drag, and on a traditional view in zoology facilitates locomotion.^[11] However, in the Cnidaria, different symmetries exist, and bilateral symmetry is not necessarily aligned with the direction of locomotion, so another mechanism such as internal transport may be needed to explain the origin of bilateral symmetry in animals.^[11][14]

Starfish larvae are bilaterally symmetric, whereas the adults have fivefold symmetry.

The phylum Echinodermata, which includes starfish, sea urchins and sand dollars, is unique among animals in having bilateral symmetry at the larval stage, but pentamerism (fivefold symmetry) as adults.^[15]

Bilateral symmetry is not easily broken. In experiments using the fruit fly, Drosophila, in contrast to other traits (where laboratory selection experiments always yield a change), right- or left-sidedness in eye size, or eye facet number, wing-folding behavior (left over right) show a lack of response.^[16]

Females of some species select for symmetry, presumed by biologists to be a mark (technically a "cue") of fitness. Female barn swallows, a species where adults have long tail streamers, prefer to mate with males that have the most symmetrical tails.^[17]

Flower of bee orchid (Ophrys apifera) is bilaterally symmetrical (zygomorphic). The lip of the flower resembles the (bilaterally symmetric) abdomen of a female bee; pollination occurs when a male bee attempts to mate with it.

Flowers in some families of flowering plants, such as the orchid and pea families, and also most of the figwort family,^[18] are bilaterally symmetric (zygomorphic).^[19]

Biradial symmetry

Biradial symmetry is a combination of radial and bilateral symmetry, as in the ctenophores. Here, the body components are arranged with similar parts on either side of a central axis, and each of the four sides of the body is identical to the opposite side but different from the adjacent side. This may represent a stage in the evolution of bilateral symmetry "from a presumably radially symmetrical ancestor."^[14]

Asymmetry

Not all animals are symmetric. Many members of the phylum Porifera (sponges) have no symmetry, though some are radially symmetric.^[20]
It is normal for essentially symmetric animals to show some measure of asymmetry. Usually in humans the left brain is structured differently to the right; the heart is positioned towards the left; and the right hand functions better than the left hand.^[21] The scale-eating cichlid Perissodus microlepis develops left or right asymmetries in their mouths and jaws that allow them to be more effective when removing scales from the left or right flank of their prey.^[22]

The approximately 400 species of flatfish also lack symmetry as adults, though the larvae are bilaterally symmetrical. Adult flatfish rest on one side, and the eye that was on that side has migrated round to the other (top) side of the body.^[23]

Essential Science: Methane ice dunes found on Pluto

By Tim Sandle     Jun 4, 2018 in Science

Original link:  http://www.digitaljournal.com/tech-and-science/science/essential-science-methane-ice-dunes-found-on-pluto/article/523756 

 

Houston - Methane ice dunes have been detected on the surface of Pluto, by NASA spacecraft. The structures offer a new insight into the dwarf planet and with the differences in planetary structures within our solar system.

The new findings point to evidence of dunes of frozen methane on Pluto. The news of interest since it indicates that the distant dwarf planet has a far more dynamic planetary ecosystem than previously thought. Prior to the finding, it had been thought that Pluto's atmosphere (mainly nitrogen with minor amounts of methane and carbon monoxide) was far too thin to be able to shape, through the presence of wind, the types of features common to deserts on Earth. The findings into the methane dunes therefore came as a surprise to NASA researchers.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

New Horizons NASA spacecraft

NASA

The data comes from NASA's New Horizons mission, which flew close to Pluto in July 2015. The data from this mission continues to be analysed. The space probe sped past Pluto at a rate of 58,536 kilometers per hour (36,373 miles per hour), collecting valuable data as moved further out into space. New Horizons New Horizons is an interplanetary space probe that was launched as a part of NASA's New Frontiers program. The main mission to perform a flyby study of the Pluto, followed by a secondary mission to fly close to and study other Kuiper belt objects. In doing so the craft became the fifth artificial object to leave the Solar System.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

CLOSEUP: The dwarf planet Pluto as photographed by the LORRI and Ralph instruments aboard the New Horizons spacecraft on July 13.

NASA-JHUAPL-SWRI/Wikimedia Commons

Pluto (or '134340 Pluto') is a dwarf planet located in the Kuiper belt, which refs to the ring of bodies beyond Neptune. It was the first Kuiper belt object to be discovered, originally classified as a planet and then downgraded to dwarf planet status. Pluto is primarily made of ice and rock and is relatively small—about one-sixth the mass of the Moon. However, the object continues to fascinate scientists interested in the origins of the Solar System. New insights into Pluto The New Horizons data into Pluto relates to a plain in the surface called Sputnik Planitia. Earlier image shad suggested that parts of the plain are covered with fields of dunes. These are, as the new data reveals, dues of methane located close to a range of mountains composed of water ice, which stand 5 kilometers tall. The newly detected methane dunes a situated between 0.4 and 1 kilometer apart, where the surface temperatures are minus 230 degrees Celsius (minus 382 Fahrenheit). The dunes appear to be composed of and that they are made up of particles of methane ice, which are some 200-300 micrometers in diameter. In other words, the methane particles are around the size of grains of sand.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

The Pluto system as we know it today. Four small moons--Styx, Nix, Kerberos and Hydra, orbit the central "binary planet" comprising Pluto and its large, nearby moon Charon.

NASA/STScI/Showalter

According to Jani Radebaugh, a planetary scientist at Brigham Young University, speaking with The Guardian: “Pluto, even though it’s so far away from Earth and so very cold, has a riot of processes we never expected to see. It is far more interesting than any of us dreamed, and tells us that these very distant bodies are well worth visiting.” Researchers have compared Pluto's dunes to those in California's Death Valley and China's Taklamakan desert, albeit with a very different composition. While dunes have been detected elsewhere in solar system: on Mars, Venus, and Saturn's moon Titan, Pluto's are the only dunes to be found to be composed of methane.

 

 

 

 

 

 

 

 

 

 

 

 

 

Pluto By Moonlight: On July 14, New Horizons mission scientists will soon obtain the first images of the night region of Pluto, using only the light from Charon, itself softly illuminated by a Sun 1,000 times dimmer than it is at Earth.

JHUAPL / SwRI

The findings have been published in the journal Science. The research paper succinctly is titled “Dunes on Pluto.”

Polar coordinate system

From Wikipedia, the free encyclopedia

 

Points in the polar coordinate system with pole O and polar axis L. In green, the point with radial coordinate 3 and angular coordinate 60 degrees or (3, 60°). In blue, the point (4, 210°).

In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction.

The reference point (analogous to the origin of a Cartesian coordinate system) is called the pole, and the ray from the pole in the reference direction is the polar axis. The distance from the pole is called the radial coordinate or radius, and the angle is called the angular coordinate, polar angle, or azimuth.^[1]

History

Hipparchus

The concepts of angle and radius were already used by ancient peoples of the first millennium BC. The Greek astronomer and astrologer Hipparchus (190–120 BC) created a table of chord functions giving the length of the chord for each angle, and there are references to his using polar coordinates in establishing stellar positions.^[2] In On Spirals, Archimedes describes the Archimedean spiral, a function whose radius depends on the angle. The Greek work, however, did not extend to a full coordinate system.

From the 8th century AD onward, astronomers developed methods for approximating and calculating the direction to Mecca (qibla)—and its distance—from any location on the Earth.^[3] From the 9th century onward they were using spherical trigonometry and map projection methods to determine these quantities accurately. The calculation is essentially the conversion of the equatorial polar coordinates of Mecca (i.e. its longitude and latitude) to its polar coordinates (i.e. its qibla and distance) relative to a system whose reference meridian is the great circle through the given location and the Earth's poles, and whose polar axis is the line through the location and its antipodal point.^[4]

There are various accounts of the introduction of polar coordinates as part of a formal coordinate system. The full history of the subject is described in Harvard professor Julian Lowell Coolidge's Origin of Polar Coordinates.^[5] Grégoire de Saint-Vincent and Bonaventura Cavalieri independently introduced the concepts in the mid-seventeenth century. Saint-Vincent wrote about them privately in 1625 and published his work in 1647, while Cavalieri published his in 1635 with a corrected version appearing in 1653. Cavalieri first used polar coordinates to solve a problem relating to the area within an Archimedean spiral. Blaise Pascal subsequently used polar coordinates to calculate the length of parabolic arcs.

In Method of Fluxions (written 1671, published 1736), Sir Isaac Newton examined the transformations between polar coordinates, which he referred to as the "Seventh Manner; For Spirals", and nine other coordinate systems.^[6] In the journal Acta Eruditorum (1691), Jacob Bernoulli used a system with a point on a line, called the pole and polar axis respectively. Coordinates were specified by the distance from the pole and the angle from the polar axis. Bernoulli's work extended to finding the radius of curvature of curves expressed in these coordinates.

The actual term polar coordinates has been attributed to Gregorio Fontana and was used by 18th-century Italian writers. The term appeared in English in George Peacock's 1816 translation of Lacroix's Differential and Integral Calculus.^[7][8] Alexis Clairaut was the first to think of polar coordinates in three dimensions, and Leonhard Euler was the first to actually develop them.^[5]

Conventions

A polar grid with several angles labelled in degrees

The radial coordinate is often denoted by r or ρ, and the angular coordinate by ϕ, θ, or t. The angular coordinate is specified as ϕ by ISO standard 31-11.

Angles in polar notation are generally expressed in either degrees or radians (2π rad being equal to 360°). Degrees are traditionally used in navigation, surveying, and many applied disciplines, while radians are more common in mathematics and mathematical physics.^[9]

In many contexts, a positive angular coordinate means that the angle ϕ is measured counterclockwise from the axis.

In mathematical literature, the polar axis is often drawn horizontal and pointing to the right, and the angle is denoted by θ instead of ϕ.

Uniqueness of polar coordinates

Adding any number of full turns (360°) to the angular coordinate does not change the corresponding direction. Also, a negative radial coordinate is best interpreted as the corresponding positive distance measured in the opposite direction. Therefore, the same point can be expressed with an infinite number of different polar coordinates (r, ϕ ± n×360°) or (−r, ϕ ± (2n + 1)180°), where n is any integer.^[10] Moreover, the pole itself can be expressed as (0, ϕ) for any angle ϕ.^[11]

Where a unique representation is needed for any point, it is usual to limit r to non-negative numbers (r ≥ 0) and ϕ to the interval [0, 360°) or (−180°, 180°] (in radians, [0, 2π) or (−π, π]).^[12] One must also choose a unique azimuth for the pole, e.g., ϕ = 0.

Converting between polar and Cartesian coordinates

A diagram illustrating the relationship between polar and Cartesian coordinates.

 

A curve on the Cartesian plane can be mapped into polar coordinates. In this animation, ${\displaystyle y=\sin(6x)+2}$ is mapped onto ${\displaystyle r=\sin(6\varphi )+2}$. Click on image for details.

The polar coordinates r and ϕ can be converted to the Cartesian coordinates x and y by using the trigonometric functions sine and cosine:

${\displaystyle x=r\cos \varphi }$

${\displaystyle y=r\sin \varphi }$

The Cartesian coordinates x and y can be converted to polar coordinates r and ϕ with r ≥ 0 and ϕ in the interval (−π, π] by:^[13]

${\displaystyle r={\sqrt {x^{2}+y^{2}}}\quad }$ (as in the Pythagorean theorem or the Euclidean norm), and

${\displaystyle \varphi =\operatorname {atan2} (y,x)\quad }$,

where atan2 is a common variation on the arctangent function defined as

${\displaystyle \operatorname {atan2} (y,x)={\begin{cases}\arctan({\frac {y}{x}})&{\mbox{if }}x>0\\\arctan({\frac {y}{x}})+\pi &{\mbox{if }}x<0 0="" amp="" and="" arctan="" frac="" geq="" if="" mbox="" pi="" x="0{\mbox{" y="">0\\-{\frac {\pi }{2}}&{\mbox{if }}x=0{\mbox{ and }}y<0 amp="" and="" if="" mbox="" text="" undefined="" x="0{\mbox{" y="0\end{cases}}}</annotation">$

If r is calculated first as above, then this formula for ϕ may be stated a little more simply using the standard arccosine function:

${\displaystyle \varphi ={\begin{cases}\arccos({\frac {x}{r}})&{\mbox{if }}y\geq 0{\mbox{ and }}r\neq 0\\-\arccos({\frac {x}{r}})&{\mbox{if }}y<0 amp="" if="" mbox="" r="0\end{cases}}}</annotation" text="" undefined="">$

The value of ϕ above is the principal value of the complex number function arg applied to x+iy. An angle in the range [0, 2π) may be obtained by adding 2π to the value in case it is negative (in other words when y is negative).

Polar equation of a curve

The equation defining an algebraic curve expressed in polar coordinates is known as a polar equation. In many cases, such an equation can simply be specified by defining r as a function of ϕ. \The resulting curve then consists of points of the form (r(ϕ), ϕ) and can be regarded as the graph of the polar function r. Note that, in contrast to Cartesian coordinates, the independent variable is the second number in the ordered pair, ϕ.

Different forms of symmetry can be deduced from the equation of a polar function r. If r(−ϕ) = r(ϕ) the curve will be symmetrical about the horizontal (0°/180°) ray, if r(π − ϕ) = r(ϕ) it will be symmetric about the vertical (90°/270°) ray, and if r(ϕ − α) = r(ϕ) it will be rotationally symmetric by α clockwise and counterclockwise about the pole.

Because of the circular nature of the polar coordinate system, many curves can be described by a rather simple polar equation, whereas their Cartesian form is much more intricate. Among the best known of these curves are the polar rose, Archimedean spiral, lemniscate, limaçon, and cardioid.

For the circle, line, and polar rose below, it is understood that there are no restrictions on the domain and range of the curve.

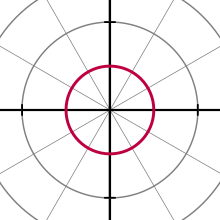
Circle

A circle with equation r(ϕ) = 1

The general equation for a circle with a center at (r₀, ${\displaystyle \gamma }$) and radius a is

${\displaystyle r^{2}-2rr_{0}\cos(\varphi -\gamma )+r_{0}^{2}=a^{2}.}$

This can be simplified in various ways, to conform to more specific cases, such as the equation

${\displaystyle r(\varphi )=a}$

for a circle with a center at the pole and radius a.^[14]

When r₀ = a, or when the origin lies on the circle, the equation becomes

${\displaystyle r=2a\cos(\varphi -\gamma )}$.

In the general case, the equation can be solved for r, giving

${\displaystyle r=r_{0}\cos(\varphi -\gamma )+{\sqrt {a^{2}-r_{0}^{2}\sin ^{2}(\varphi -\gamma )}}}$,

the solution with a minus sign in front of the square root gives the same curve.

Line

A polar rose with equation r(ϕ) = 2 sin 4ϕ

Radial lines (those running through the pole) are represented by the equation

${\displaystyle \varphi =\gamma }$,

where ɣ is the angle of elevation of the line; that is, ɣ = arctan m where m is the slope of the line in the Cartesian coordinate system. The non-radial line that crosses the radial line ϕ = ɣ perpendicularly at the point (r₀, ɣ) has the equation

${\displaystyle r(\varphi )={r_{0}}\sec(\varphi -\gamma ).}$

Otherwise stated (r₀, ɣ) is the point in which the tangent intersects the imaginary circle of radius r₀.

Polar rose

A polar rose is a famous mathematical curve that looks like a petaled flower, and that can be expressed as a simple polar equation,

${\displaystyle r(\varphi )=a\cos(k\varphi +\gamma _{0})}$

for any constant ɣ₀ (including 0). If k is an integer, these equations will produce a k-petaled rose if k is odd, or a 2k-petaled rose if k is even. If k is rational but not an integer, a rose-like shape may form but with overlapping petals. Note that these equations never define a rose with 2, 6, 10, 14, etc. petals. The variable a represents the length of the petals of the rose.

Archimedean spiral

One arm of an Archimedean spiral with equation r(ϕ) = ϕ / 2π for 0 < ϕ < 6π

The Archimedean spiral is a famous spiral that was discovered by Archimedes, which can also be expressed as a simple polar equation. It is represented by the equation

${\displaystyle r(\varphi )=a+b\varphi .}$

Changing the parameter a will turn the spiral, while b controls the distance between the arms, which for a given spiral is always constant. The Archimedean spiral has two arms, one for ϕ > 0 and one for ϕ < 0. The two arms are smoothly connected at the pole. Taking the mirror image of one arm across the 90°/270° line will yield the other arm. This curve is notable as one of the first curves, after the conic sections, to be described in a mathematical treatise, and as being a prime example of a curve that is best defined by a polar equation.

Conic sections

Ellipse, showing semi-latus rectum

A conic section with one focus on the pole and the other somewhere on the 0° ray (so that the conic's major axis lies along the polar axis) is given by:

${\displaystyle r={\ell \over {1-e\cos \varphi }}}$

where e is the eccentricity and ${\displaystyle \ell }$ is the semi-latus rectum (the perpendicular distance at a focus from the major axis to the curve). If e > 1, this equation defines a hyperbola; if e = 1, it defines a parabola; and if e < 1, it defines an ellipse. The special case e = 0 of the latter results in a circle of the radius ${\displaystyle \ell }$.

Intersection of two polar curves

The graphs of two polar functions ${\displaystyle r=f(\theta )}$ and ${\displaystyle r=g(\theta )}$ have possible intersections of three types:

1. In the origin if the equations ${\displaystyle f(\theta )=0}$ and ${\displaystyle g(\theta )=0}$ have at least one solution each.
2. All the points ${\displaystyle [g(\theta _{i}),\theta _{i}]}$ where ${\displaystyle \theta _{i}}$ are the solutions to the equation ${\displaystyle f(\theta )=g(\theta )}$.
3. All the points ${\displaystyle [g(\theta _{i}),\theta _{i}]}$ where ${\displaystyle \theta _{i}}$ are the solutions to the equation ${\displaystyle f(\theta +(2k+1)\pi )=-g(\theta )}$ where ${\displaystyle k}$ is an integer.

Complex numbers

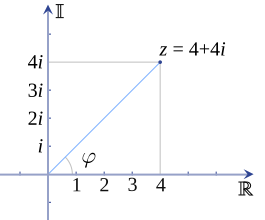
An illustration of a complex number z plotted on the complex plane

 

An illustration of a complex number plotted on the complex plane using Euler's formula

Every complex number can be represented as a point in the complex plane, and can therefore be expressed by specifying either the point's Cartesian coordinates (called rectangular or Cartesian form) or the point's polar coordinates (called polar form). The complex number z can be represented in rectangular form as

${\displaystyle z=x+iy}$

where i is the imaginary unit, or can alternatively be written in polar form (via the conversion formulae given above) as

${\displaystyle z=r\cdot (\cos \varphi +i\sin \varphi )}$

and from there as

${\displaystyle z=re^{i\varphi }}$

where e is Euler's number, which are equivalent as shown by Euler's formula.^[15] (Note that this formula, like all those involving exponentials of angles, assumes that the angle ϕ is expressed in radians.) To convert between the rectangular and polar forms of a complex number, the conversion formulae given above can be used.

For the operations of multiplication, division, and exponentiation of complex numbers, it is generally much simpler to work with complex numbers expressed in polar form rather than rectangular form. From the laws of exponentiation:

• Multiplication:

${\displaystyle r_{0}e^{i\varphi _{0}}\cdot r_{1}e^{i\varphi _{1}}=r_{0}r_{1}e^{i(\varphi _{0}+\varphi _{1})}}$

• Division:

${\displaystyle {\frac {r_{0}e^{i\varphi _{0}}}{r_{1}e^{i\varphi _{1}}}}={\frac {r_{0}}{r_{1}}}e^{i(\varphi _{0}-\varphi _{1})}}$

• Exponentiation (De Moivre's formula):

${\displaystyle (re^{i\varphi })^{n}=r^{n}e^{in\varphi }}$

Calculus

Calculus can be applied to equations expressed in polar coordinates.^[16][17]

The angular coordinate ϕ is expressed in radians throughout this section, which is the conventional choice when doing calculus.

Differential calculus

Using x = r cos ϕ and y = r sin ϕ , one can derive a relationship between derivatives in Cartesian and polar coordinates. For a given function, u(x,y), it follows that (by computing its total derivatives)

${\displaystyle {\begin{aligned}r{\frac {\partial u}{\partial r}}&=r{\frac {\partial u}{\partial x}}{\frac {\partial x}{\partial r}}+r{\frac {\partial u}{\partial y}}{\frac {\partial y}{\partial r}},\\{\frac {\partial u}{\partial \varphi }}&={\frac {\partial u}{\partial x}}{\frac {\partial x}{\partial \varphi }}+{\frac {\partial u}{\partial y}}{\frac {\partial y}{\partial \varphi }},\end{aligned}}}$

or

${\displaystyle {\begin{aligned}r{\frac {\partial u}{\partial r}}&=r{\frac {\partial u}{\partial x}}\cos \varphi +r{\frac {\partial u}{\partial y}}\sin \varphi =x{\frac {\partial u}{\partial x}}+y{\frac {\partial u}{\partial y}},\\{\frac {\partial u}{\partial \varphi }}&=-{\frac {\partial u}{\partial x}}r\sin \varphi +{\frac {\partial u}{\partial y}}r\cos \varphi =-y{\frac {\partial u}{\partial x}}+x{\frac {\partial u}{\partial y}}.\end{aligned}}}$

Hence, we have the following formulae:

${\displaystyle {\begin{aligned}r{\frac {\partial }{\partial r}}&=x{\frac {\partial }{\partial x}}+y{\frac {\partial }{\partial y}}\\{\frac {\partial }{\partial \varphi }}&=-y{\frac {\partial }{\partial x}}+x{\frac {\partial }{\partial y}}.\end{aligned}}}$

Using the inverse coordinates transformation, an analogous reciprocal relationship can be derived between the derivatives. Given a function u(r,ϕ), it follows that

${\displaystyle {\begin{aligned}{\frac {\partial u}{\partial x}}&={\frac {\partial u}{\partial r}}{\frac {\partial r}{\partial x}}+{\frac {\partial u}{\partial \varphi }}{\frac {\partial \varphi }{\partial x}},\\{\frac {\partial u}{\partial y}}&={\frac {\partial u}{\partial r}}{\frac {\partial r}{\partial y}}+{\frac {\partial u}{\partial \varphi }}{\frac {\partial \varphi }{\partial y}},\end{aligned}}}$

or

${\displaystyle {\begin{aligned}{\frac {\partial u}{\partial x}}&={\frac {\partial u}{\partial r}}{\frac {x}{\sqrt {x^{2}+y^{2}}}}-{\frac {\partial u}{\partial \varphi }}{\frac {y}{x^{2}+y^{2}}}\\&=\cos \varphi {\frac {\partial u}{\partial r}}-{\frac {1}{r}}\sin \varphi {\frac {\partial u}{\partial \varphi }},\\{\frac {\partial u}{\partial y}}&={\frac {\partial u}{\partial r}}{\frac {y}{\sqrt {x^{2}+y^{2}}}}+{\frac {\partial u}{\partial \varphi }}{\frac {x}{x^{2}+y^{2}}}\\&=\sin \varphi {\frac {\partial u}{\partial r}}+{\frac {1}{r}}\cos \varphi {\frac {\partial u}{\partial \varphi }}.\end{aligned}}}$

Hence, we have the following formulae:

${\displaystyle {\begin{aligned}{\frac {\partial }{\partial x}}&=\cos \varphi {\frac {\partial }{\partial r}}-{\frac {1}{r}}\sin \varphi {\frac {\partial }{\partial \varphi }}\\{\frac {\partial }{\partial y}}&=\sin \varphi {\frac {\partial }{\partial r}}+{\frac {1}{r}}\cos \varphi {\frac {\partial }{\partial \varphi }}.\end{aligned}}}$

To find the Cartesian slope of the tangent line to a polar curve r(ϕ) at any given point, the curve is first expressed as a system of parametric equations.

${\displaystyle {\begin{aligned}x&=r(\varphi )\cos \varphi \\y&=r(\varphi )\sin \varphi \end{aligned}}}$

Differentiating both equations with respect to ϕ yields

${\displaystyle {\begin{aligned}{\frac {dx}{d\varphi }}&=r'(\varphi )\cos \varphi -r(\varphi )\sin \varphi \\{\frac {dy}{d\varphi }}&=r'(\varphi )\sin \varphi +r(\varphi )\cos \varphi .\end{aligned}}}$

Dividing the second equation by the first yields the Cartesian slope of the tangent line to the curve at the point (r(ϕ), ϕ):

${\displaystyle {\frac {dy}{dx}}={\frac {r'(\varphi )\sin \varphi +r(\varphi )\cos \varphi }{r'(\varphi )\cos \varphi -r(\varphi )\sin \varphi }}.}$

For other useful formulas including divergence, gradient, and Laplacian in polar coordinates, see curvilinear coordinates.

Integral calculus (arc length)

The arc length (length of a line segment) defined by a polar function is found by the integration over the curve r(ϕ). Let L denote this length along the curve starting from points A through to point B, where these points correspond to ϕ = a and ϕ = b such that 0 < b − a < 2π. The length of L is given by the following integral

${\displaystyle L=\int _{a}^{b}{\sqrt {\left[r(\varphi )\right]^{2}+\left[{\tfrac {dr(\varphi )}{d\varphi }}\right]^{2}}}d\varphi }$

Integral calculus (area)

The integration region R is bounded by the curve r(ϕ) and the rays ϕ = a and ϕ = b.

Let R denote the region enclosed by a curve r(ϕ) and the rays ϕ = a and ϕ = b, where 0 < b − a ≤ 2π. Then, the area of R is

${\displaystyle {\frac {1}{2}}\int _{a}^{b}\left[r(\varphi )\right]^{2}\,d\varphi .}$

The region R is approximated by n sectors (here, n = 5).

 

A planimeter, which mechanically computes polar integrals

This result can be found as follows. First, the interval [a, b] is divided into n subintervals, where n is an arbitrary positive integer. Thus Δϕ, the length of each subinterval, is equal to b − a (the total length of the interval), divided by n, the number of subintervals. For each subinterval i = 1, 2, …, n, let ϕ_i be the midpoint of the subinterval, and construct a sector with the center at the pole, radius r(ϕ_i), central angle Δϕ and arc length r(ϕ_i)Δϕ. The area of each constructed sector is therefore equal to

${\displaystyle \left[r(\varphi _{i})\right]^{2}\pi \cdot {\frac {\Delta \varphi }{2\pi }}={\frac {1}{2}}\left[r(\varphi _{i})\right]^{2}\Delta \varphi .}$

Hence, the total area of all of the sectors is

${\displaystyle \sum _{i=1}^{n}{\tfrac {1}{2}}r(\varphi _{i})^{2}\,\Delta \varphi .}$

As the number of subintervals n is increased, the approximation of the area continues to improve. In the limit as n → ∞, the sum becomes the Riemann sum for the above integral.

A mechanical device that computes area integrals is the planimeter, which measures the area of plane figures by tracing them out: this replicates integration in polar coordinates by adding a joint so that the 2-element linkage effects Green's theorem, converting the quadratic polar integral to a linear integral.

Generalization

Using Cartesian coordinates, an infinitesimal area element can be calculated as dA = dx dy. The substitution rule for multiple integrals states that, when using other coordinates, the Jacobian determinant of the coordinate conversion formula has to be considered:

${\displaystyle J=\det {\frac {\partial (x,y)}{\partial (r,\varphi )}}={\begin{vmatrix}{\frac {\partial x}{\partial r}}&{\frac {\partial x}{\partial \varphi }}\\[8pt]{\frac {\partial y}{\partial r}}&{\frac {\partial y}{\partial \varphi }}\end{vmatrix}}={\begin{vmatrix}\cos \varphi &-r\sin \varphi \\\sin \varphi &r\cos \varphi \end{vmatrix}}=r\cos ^{2}\varphi +r\sin ^{2}\varphi =r.}$

Hence, an area element in polar coordinates can be written as

${\displaystyle dA=dx\,dy\ =J\,dr\,d\varphi =r\,dr\,d\varphi .}$

Now, a function, that is given in polar coordinates, can be integrated as follows:

${\displaystyle \iint _{R}f(x,y)\,dA=\int _{a}^{b}\int _{0}^{r(\varphi )}f(r,\varphi )\,r\,dr\,d\varphi .}$

Here, R is the same region as above, namely, the region enclosed by a curve r(ϕ) and the rays ϕ = a and ϕ = b.

The formula for the area of R mentioned above is retrieved by taking f identically equal to 1. A more surprising application of this result yields the Gaussian integral

${\displaystyle \int _{-\infty }^{\infty }e^{-x^{2}}\,dx={\sqrt {\pi }}.}$

Vector calculus

Vector calculus can also be applied to polar coordinates. For a planar motion, let ${\displaystyle \mathbf {r} }$ be the position vector (r cos(ϕ), r sin(ϕ)), with r and ϕ depending on time t.

We define the unit vectors

${\displaystyle {\hat {\mathbf {r} }}=(\cos(\varphi ),\sin(\varphi ))}$

in the direction of r and

${\displaystyle {\hat {\boldsymbol {\varphi }}}=(-\sin(\varphi ),\cos(\varphi ))={\hat {\mathbf {k} }}\times {\hat {\mathbf {r} }}\ ,}$

in the plane of the motion perpendicular to the radial direction, where ${\displaystyle {\hat {\mathbf {k} }}}$ is a unit vector normal to the plane of the motion.

Then

${\displaystyle {\begin{aligned}\mathbf {r} &=(x,\ y)=r(\cos \varphi ,\ \sin \varphi )=r{\hat {\mathbf {r} }}\ ,\\{\dot {\mathbf {r} }}&=({\dot {x}},\ {\dot {y}})={\dot {r}}(\cos \varphi ,\ \sin \varphi )+r{\dot {\varphi }}(-\sin \varphi ,\ \cos \varphi )={\dot {r}}{\hat {\mathbf {r} }}+r{\dot {\varphi }}{\hat {\boldsymbol {\varphi }}}\ ,\\{\ddot {\mathbf {r} }}&=({\ddot {x}},\ {\ddot {y}})\\&={\ddot {r}}(\cos \varphi ,\ \sin \varphi )+2{\dot {r}}{\dot {\varphi }}(-\sin \varphi ,\ \cos \varphi )+r{\ddot {\varphi }}(-\sin \varphi ,\ \cos \varphi )-r{\dot {\varphi }}^{2}(\cos \varphi ,\ \sin \varphi )\\&=\left({\ddot {r}}-r{\dot {\varphi }}^{2}\right){\hat {\mathbf {r} }}+\left(r{\ddot {\varphi }}+2{\dot {r}}{\dot {\varphi }}\right){\hat {\boldsymbol {\varphi }}}\\&=\left({\ddot {r}}-r{\dot {\varphi }}^{2}\right){\hat {\mathbf {r} }}+{\frac {1}{r}}\;{\frac {d}{dt}}\left(r^{2}{\dot {\varphi }}\right){\hat {\boldsymbol {\varphi }}}\end{aligned}}}$.

Centrifugal and Coriolis terms

Position vector r, always points radially from the origin.

Velocity vector v, always tangent to the path of motion.

Acceleration vector a, not parallel to the radial motion but offset by the angular and Coriolis accelerations, nor tangent to the path but offset by the centripetal and radial accelerations.

 

Kinematic vectors in plane polar coordinates. Notice the setup is not restricted to 2d space, but a plane in any higher dimension.

The term ${\displaystyle r{\dot {\varphi }}^{2}}$ is sometimes referred to as the centripetal acceleration, and the term ${\displaystyle 2{\dot {r}}{\dot {\varphi }}}$ as the Coriolis acceleration. For example, see Shankar.^[18]

Note: these terms, that appear when acceleration is expressed in polar coordinates, are a mathematical consequence of differentiation; they appear whenever polar coordinates are used. In planar particle dynamics these accelerations appear when setting up Newton's second law of motion in a rotating frame of reference. Here these extra terms are often called fictitious forces; fictitious because they are simply a result of a change in coordinate frame. That does not mean they do not exist, rather they exist only in the rotating frame.

Inertial frame of reference S and instantaneous non-inertial co-rotating frame of reference S′. The co-rotating frame rotates at angular rate Ω equal to the rate of rotation of the particle about the origin of S′ at the particular moment t. Particle is located at vector position r(t) and unit vectors are shown in the radial direction to the particle from the origin, and also in the direction of increasing angle ϕ normal to the radial direction. These unit vectors need not be related to the tangent and normal to the path. Also, the radial distance r need not be related to the radius of curvature of the path.

Co-rotating frame

For a particle in planar motion, one approach to attaching physical significance to these terms is based on the concept of an instantaneous co-rotating frame of reference.^[19] To define a co-rotating frame, first an origin is selected from which the distance r(t) to the particle is defined. An axis of rotation is set up that is perpendicular to the plane of motion of the particle, and passing through this origin. Then, at the selected moment t, the rate of rotation of the co-rotating frame Ω is made to match the rate of rotation of the particle about this axis, dϕ/dt. Next, the terms in the acceleration in the inertial frame are related to those in the co-rotating frame. Let the location of the particle in the inertial frame be (r(t), ϕ(t)), and in the co-rotating frame be (r(t), ϕ′(t)). Because the co-rotating frame rotates at the same rate as the particle, dϕ′/dt = 0. The fictitious centrifugal force in the co-rotating frame is mrΩ², radially outward. The velocity of the particle in the co-rotating frame also is radially outward, because dϕ′/dt = 0. The fictitious Coriolis force therefore has a value −2m(dr/dt)Ω, pointed in the direction of increasing ϕ only. Thus, using these forces in Newton's second law we find:

${\displaystyle {\boldsymbol {F}}+{\boldsymbol {F_{cf}}}+{\boldsymbol {F_{Cor}}}=m{\ddot {\boldsymbol {r}}}\ ,}$

where over dots represent time differentiations, and F is the net real force (as opposed to the fictitious forces). In terms of components, this vector equation becomes:

${\displaystyle F_{r}+mr\Omega ^{2}=m{\ddot {r}}}$

${\displaystyle F_{\varphi }-2m{\dot {r}}\Omega =mr{\ddot {\varphi }}\ ,}$

which can be compared to the equations for the inertial frame:

${\displaystyle F_{r}=m{\ddot {r}}-mr{\dot {\varphi }}^{2}}$

${\displaystyle F_{\varphi }=mr{\ddot {\varphi }}+2m{\dot {r}}{\dot {\varphi }}\ .}$

This comparison, plus the recognition that by the definition of the co-rotating frame at time t it has a rate of rotation Ω = dϕ/dt, shows that we can interpret the terms in the acceleration (multiplied by the mass of the particle) as found in the inertial frame as the negative of the centrifugal and Coriolis forces that would be seen in the instantaneous, non-inertial co-rotating frame.

For general motion of a particle (as opposed to simple circular motion), the centrifugal and Coriolis forces in a particle's frame of reference commonly are referred to the instantaneous osculating circle of its motion, not to a fixed center of polar coordinates. For more detail, see centripetal force.

Connection to spherical and cylindrical coordinates

The polar coordinate system is extended into three dimensions with two different coordinate systems, the cylindrical and spherical coordinate system.

Applications

Polar coordinates are two-dimensional and thus they can be used only where point positions lie on a single two-dimensional plane. They are most appropriate in any context where the phenomenon being considered is inherently tied to direction and length from a center point. For instance, the examples above show how elementary polar equations suffice to define curves—such as the Archimedean spiral—whose equation in the Cartesian coordinate system would be much more intricate. Moreover, many physical systems—such as those concerned with bodies moving around a central point or with phenomena originating from a central point—are simpler and more intuitive to model using polar coordinates. The initial motivation for the introduction of the polar system was the study of circular and orbital motion.

Position and navigation

Polar coordinates are used often in navigation as the destination or direction of travel can be given as an angle and distance from the object being considered. For instance, aircraft use a slightly modified version of the polar coordinates for navigation. In this system, the one generally used for any sort of navigation, the 0° ray is generally called heading 360, and the angles continue in a clockwise direction, rather than counterclockwise, as in the mathematical system. Heading 360 corresponds to magnetic north, while headings 90, 180, and 270 correspond to magnetic east, south, and west, respectively.^[20] Thus, an aircraft traveling 5 nautical miles due east will be traveling 5 units at heading 90 (read zero-niner-zero by air traffic control).^[21]

Modeling

Systems displaying radial symmetry provide natural settings for the polar coordinate system, with the central point acting as the pole. A prime example of this usage is the groundwater flow equation when applied to radially symmetric wells. Systems with a radial force are also good candidates for the use of the polar coordinate system. These systems include gravitational fields, which obey the inverse-square law, as well as systems with point sources, such as radio antennas.

Radially asymmetric systems may also be modeled with polar coordinates. For example, a microphone's pickup pattern illustrates its proportional response to an incoming sound from a given direction, and these patterns can be represented as polar curves. The curve for a standard cardioid microphone, the most common unidirectional microphone, can be represented as r = 0.5 + 0.5sin(ϕ) at its target design frequency.^[22] The pattern shifts toward omnidirectionality at lower frequencies.