Good ideas start with a question. Great ideas start with a question that comes back to you. One such question that has haunted scientists and philosophers for thousands of years is whether there is a smallest unit of length, a shortest distance below which we cannot resolve structures. Can we forever look closer and ever closer into space, time, and matter? Or is there a fundamental limit, and if so, what is it, and what is it that dictates its nature?
I
picture our foreign ancestors sitting in their cave watching the world
in amazement, wondering what the stones, the trees and they themselves
are made of — and then starving to death. Luckily, those smart enough to
hunt down the occasional bear eventually gave rise to a human
civilization that was sheltered enough from the harshness of life to let
the survivors get back to watching and wondering what we are made of.
Science and philosophy in earnest is only a few thousand years old, but
the question whether there is smallest unit has been a driving force in
our studies of the natural world for all of recorded history.
The
Ancient Greeks invented atomism: the idea that there is an ultimate and
smallest element of matter that everything is made of dates back to
Democritus of Abdera. Zeno’s famous paradoxa sought to shed light on the
possibility of infinite divisibility. The question returned in the
modern age with the advent of quantum mechanics, with Heisenberg’s
uncertainty principle fundamentally limiting the precision to which we
can measure. It became only more pressing with the divergences inherent
to quantum field theory, due to the necessary inclusion of infinitely
short distances.

It
was in fact Heisenberg who first suggested that the divergences in
quantum field theory might be cured by the existence of a fundamentally
minimal length, and he introduced it by making position operators
non-commuting among themselves. Just as the non-commutativity of
momentum and position operators leads to an uncertainty principle, the
non-commutativity of position operators limits how well distances can be
measured.
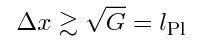
Heisenberg’s
main worry, which the minimal length was supposed to deal with, was the
non-renormalizability of Fermi’s theory of beta-decay. This theory,
however, turned out to be only an approximation to the renormalizable
electro-weak interaction, so he had to worry no more.
Heisenberg’s
idea was forgotten for some decades, then picked up again and
eventually grew into the area of non-commutative geometries. Meanwhile,
the problem of quantizing gravity appeared on stage and with it, again,
non-renormalizability.
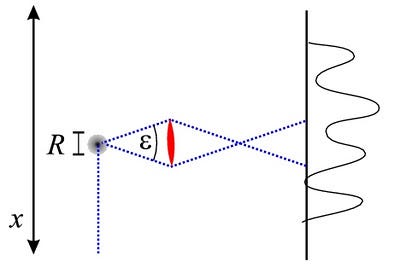
In the mid-1960s, Alden Mead reinvestigated Heisenberg’s microscope,
the argument that lead to the uncertainty principle, with
(non-quantized) gravity taken into account. He showed that gravity
amplifies the uncertainty inherent to position so that it becomes
impossible to measure distances below the Planck length: about 10^-33
cm. Mead’s argument was forgotten, then rediscovered in the 1990s by
string theorists who had noticed that using strings to prevent
divergences (by avoiding point-interactions) also implies a finite resolution, if in a technically somewhat different way than Mead’s.

Since
then, the idea that the Planck length may be a fundamental length
beyond which there is nothing new to find, ever, appeared in other
approaches towards quantum gravity, such as Loop Quantum Gravity and
Asymptotically Safe Gravity. It has also been studied as an effective
theory by modifying quantum field theory to include a minimal length
from scratch, and often runs under the name “generalized uncertainty”.
One
of the main difficulties with these theories is that a minimal length,
if interpreted as the length of a ruler, would not be invariant under
Lorentz-transformations due to length contraction. In other words, the
idea of a “minimum length” would suddenly imply that different observers
(i.e., people moving at different velocities) would measure different
fundamental minimum lengths from one another! This problem is easy to
overcome in momentum space, where it is a maximal energy that has to be
made Lorentz-invariant, because momentum space is not translationally
invariant. But in position space, one either has to break
Lorentz-invariance or deform it and give up locality, which has
observable consequences, and not always desired ones. Personally, I
think it is a mistake to interpret the minimal length as the length of a
ruler (a component of a Lorentz-vector), and it should instead be
interpreted as a Lorentz-invariant scalar to begin with, but opinions on
that matter differ.
The science and history of the physical idea of a minimal length has now been covered in a recent book by Amit Hagar.
Amit
is a philosopher but he certainly knows his math and physics. Indeed, I
suspect the book would be quite hard to understand for a reader without
at least some background knowledge in those two subjects. Amit has made
a considerable effort to address the topic of a fundamental length from
as many perspectives as possible, and he covers a lot of scientific
history and philosophical
considerations that I had not previously been
aware of. The book is also noteworthy for including a chapter on quantum
gravity phenomenology.
My
only complaint about the book is its title, because the question of
“discrete vs. continuous” is not the same as the question of “finite vs.
infinite resolution.” One can have a continuous structure and yet be
unable to resolve it beyond some limit, such as would be the case when
the limit makes itself noticeable as a blur rather than a
discretization. On the other hand, one can have a discrete structure
that does not prevent arbitrarily sharp resolution, which can happen
when localization on a single base-point of the discrete structure is
possible.
(Amit’s book is admittedly quite pricey,
so let me add that he said should sales numbers reach 500, Cambridge
University Press will put a considerably less expensive paperback
version on offer. So tell your library to get a copy and let’s hope
we’ll make it to 500 so it becomes affordable for more of the interested
readers.)
Every
once in a while I think that there maybe is no fundamentally smallest
unit of length; that all these arguments for its existence are wrong. I
like to think that we can look infinitely close into structures and will
never find a final theory, turtles upon turtles, or that structures are
ultimately self-similar and repeat. Alas, it is hard to make sense of
the romantic idea of universes in universes in universes mathematically,
not that I didn’t try, and so the minimal length keeps coming back to
me.
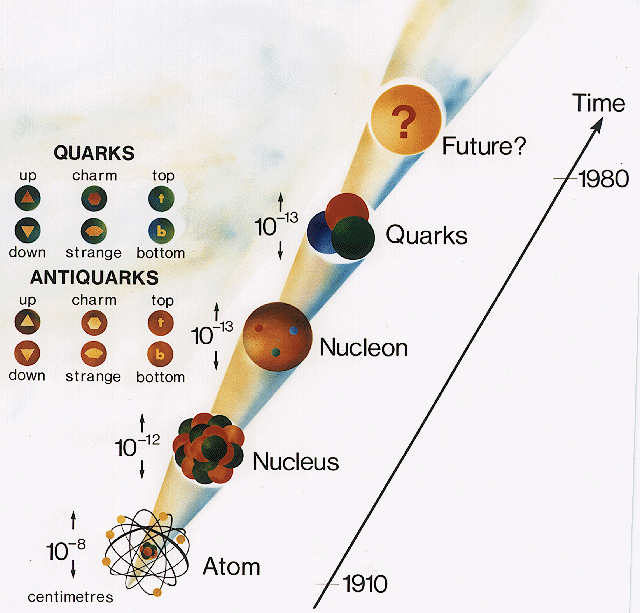
Many
(if not most) endeavors to find observational evidence for quantum
gravity today look for manifestations of a minimal length in one way or
the other, such as modifications of the dispersion relation, modifications of the commutation-relations, or Bekenstein’s tabletop search for quantum gravity.
The question of whether there is a smallest possible scale in the
Universe is today a very active area of research. We’ve come a long way,
but we’re still out to answer the same questions that people asked
themselves thousands of years ago. Although we’ve certainly made a lot
of progress, the ultimate answer is still beyond our abilities to
resolve.
This post was written by Sabine Hossenfelder, assistant professor of physics at Nordita. You can read her (more technical) paper on a fundamental minimum length here, and follow her tweets at @skdh.

